|
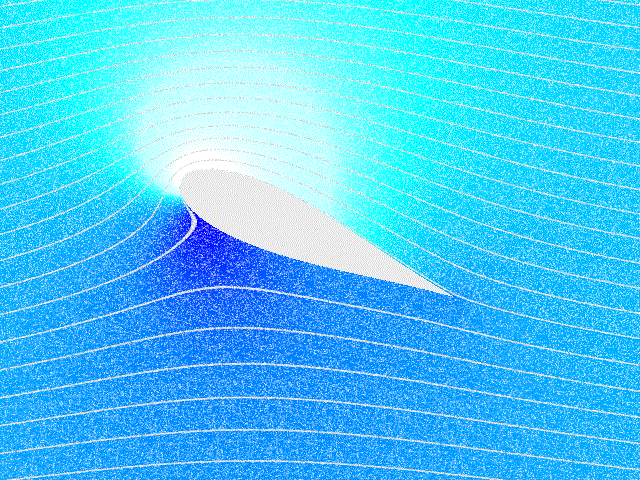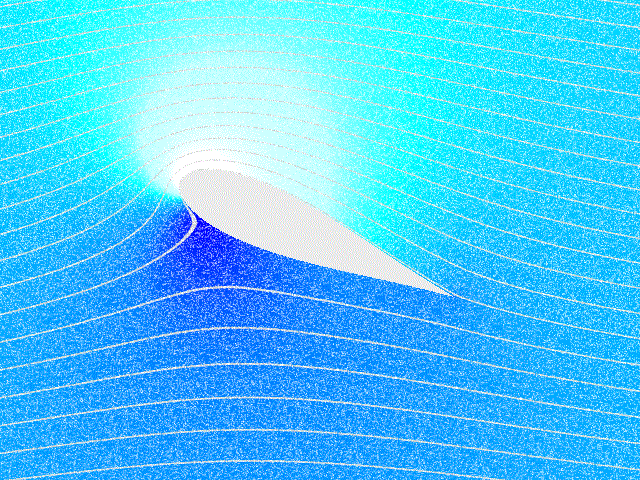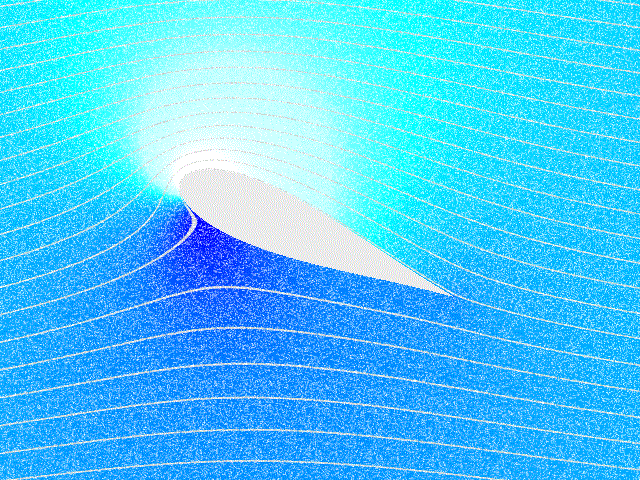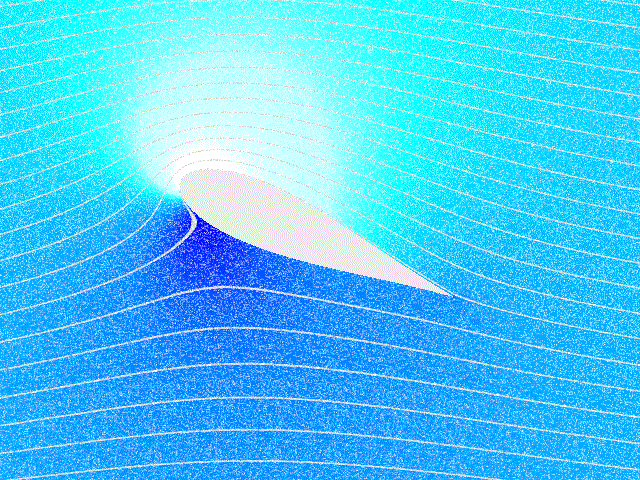
Vorticity
In continuum mechanics, vorticity is a pseudovector (or axial vector) field that describes the local spinning motion of a continuum near some point (the tendency of something to rotate), as would be seen by an observer located at that point and traveling along with the flow. It is an important quantity in the dynamical theory of fluids and provides a convenient framework for understanding a variety of complex flow phenomena, such as the formation and motion of vortex rings. Mathematically, the vorticity \boldsymbol is the curl of the flow velocity \mathbf v: :\boldsymbol \equiv \nabla \times \mathbf v\,, where \nabla is the nabla operator. Conceptually, \boldsymbol could be determined by marking parts of a continuum in a small neighborhood of the point in question, and watching their ''relative'' displacements as they move along the flow. The vorticity \boldsymbol would be twice the mean angular velocity vector of those particles relative to their center of mass, orie ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
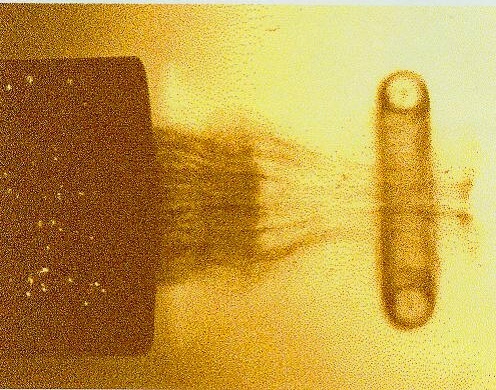
Vortex Ring
A vortex ring, also called a toroidal vortex, is a torus-shaped vortex in a fluid; that is, a region where the fluid mostly spins around an imaginary axis line that forms a closed loop. The dominant flow in a vortex ring is said to be toroidal, more precisely poloidal. Vortex rings are plentiful in turbulent flows of liquids and gases, but are rarely noticed unless the motion of the fluid is revealed by suspended particles—as in the smoke rings which are often produced intentionally or accidentally by smokers. Fiery vortex rings are also a commonly produced trick by fire eaters. Visible vortex rings can also be formed by the firing of certain artillery, in mushroom clouds, in microbursts, and rarely in volcanic eruptions. A vortex ring usually tends to move in a direction that is perpendicular to the plane of the ring and such that the inner edge of the ring moves faster forward than the outer edge. Within a stationary body of fluid, a vortex ring can travel for relative ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Circulation (fluid Dynamics)
In physics, circulation is the line integral of a vector field around a closed curve embedded in the field. In fluid dynamics, the field is the fluid velocity field. In electrodynamics, it can be the electric or the magnetic field. In aerodynamics, it finds applications in the calculation of lift, for which circulation was first used independently by Frederick Lanchester, Ludwig Prandtl, Martin Kutta and Nikolay Zhukovsky. It is usually denoted (uppercase gamma). Definition and properties If is a vector field and is a vector representing the differential length of a small element of a defined curve, the contribution of that differential length to circulation is : \mathrm\Gamma = \mathbf \cdot \mathrm\mathbf = \left, \mathbf\ \left, \mathrm\mathbf\ \cos \theta. Here, is the angle between the vectors and . The circulation of a vector field around a closed curve is the line integral: \Gamma = \oint_\mathbf \cdot \mathrm d \mathbf. In a conservative vector field ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Rankine Vortex
The Rankine vortex is a simple mathematical model of a vortex in a viscous fluid. It is named after its discoverer, William John Macquorn Rankine. The vortices observed in nature are usually modelled with an irrotational (potential or free) vortex. However, in a potential vortex, the velocity becomes infinite at the vortex center. In reality, very close to the origin, the motion resembles a solid body rotation. The Rankine vortex model assumes a solid-body rotation inside a cylinder of radius a and a potential vortex outside the cylinder. The radius a is referred to as the vortex-core radius. The velocity components (v_r,v_\theta,v_z) of the Rankine vortex, expressed in terms of the cylindrical-coordinate system (r,\theta,z) are given by :v_r=0,\quad v_\theta(r) = \frac\begin r/a^2 & r \le a, \\ 1/ r & r > a \end, \quad v_z = 0 where \Gamma is the circulation strength of the Rankine vortex. Since solid-body rotation is characterized by an azimuthal velocity \Omega r, where \O ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Flow Velocity
In continuum mechanics the flow velocity in fluid dynamics, also macroscopic velocity in statistical mechanics, or drift velocity in electromagnetism, is a vector field used to mathematically describe the motion of a continuum. The length of the flow velocity vector is scalar, the ''flow speed''. It is also called velocity field; when evaluated along a line, it is called a velocity profile (as in, e.g., law of the wall). Definition The flow velocity ''u'' of a fluid is a vector field : \mathbf=\mathbf(\mathbf,t), which gives the velocity of an '' element of fluid'' at a position \mathbf\, and time t.\, The flow speed ''q'' is the length of the flow velocity vector :q = \, \mathbf \, and is a scalar field. Uses The flow velocity of a fluid effectively describes everything about the motion of a fluid. Many physical properties of a fluid can be expressed mathematically in terms of the flow velocity. Some common examples follow: Steady flow The flow of a fluid is sai ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Fluid Dynamics
In physics, physical chemistry and engineering, fluid dynamics is a subdiscipline of fluid mechanics that describes the flow of fluids – liquids and gases. It has several subdisciplines, including (the study of air and other gases in motion) and (the study of water and other liquids in motion). Fluid dynamics has a wide range of applications, including calculating forces and moment (physics), moments on aircraft, determining the mass flow rate of petroleum through pipeline transport, pipelines, weather forecasting, predicting weather patterns, understanding nebulae in interstellar space, understanding large scale Geophysical fluid dynamics, geophysical flows involving oceans/atmosphere and Nuclear weapon design, modelling fission weapon detonation. Fluid dynamics offers a systematic structure—which underlies these practical disciplines—that embraces empirical and semi-empirical laws derived from flow measurement and used to solve practical problems. The solution to a fl ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Solenoidal
In vector calculus a solenoidal vector field (also known as an incompressible vector field, a divergence-free vector field, or a transverse vector field) is a vector field v with divergence zero at all points in the field: \nabla \cdot \mathbf = 0. A common way of expressing this property is to say that the field has no sources or sinks.This statement does not mean that the field lines of a solenoidal field must be closed, neither that they cannot begin or end. For a detailed discussion of the subject, see J. Slepian: "Lines of Force in Electric and Magnetic Fields", American Journal of Physics, vol. 19, pp. 87-90, 1951, and L. Zilberti: "The Misconception of Closed Magnetic Flux Lines", IEEE Magnetics Letters, vol. 8, art. 1306005, 2017. Properties The divergence theorem gives an equivalent integral definition of a solenoidal field; namely that for any closed surface, the net total flux through the surface must be zero: where d\mathbf is the outward normal to each surface el ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Curl (mathematics)
In vector calculus, the curl, also known as rotor, is a vector operator that describes the Differential (infinitesimal), infinitesimal Circulation (physics), circulation of a vector field in three-dimensional Euclidean space. The curl at a point in the field is represented by a vector (geometry), vector whose length and direction denote the Magnitude (mathematics), magnitude and axis of the maximum circulation. The curl of a field is formally defined as the circulation density at each point of the field. A vector field whose curl is zero is called irrotational. The curl is a form of derivative, differentiation for vector fields. The corresponding form of the fundamental theorem of calculus is Kelvin–Stokes theorem, Stokes' theorem, which relates the surface integral of the curl of a vector field to the line integral of the vector field around the boundary curve. The notation is more common in North America. In the rest of the world, particularly in 20th century scientific li ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Right-hand Rule
In mathematics and physics, the right-hand rule is a Convention (norm), convention and a mnemonic, utilized to define the orientation (vector space), orientation of Cartesian coordinate system, axes in three-dimensional space and to determine the direction of the cross product of two Euclidean vector, vectors, as well as to establish the direction of the force on a Electric current, current-carrying conductor in a magnetic field. The various right- and left-hand rules arise from the fact that the three axes of three-dimensional space have two possible orientations. This can be seen by holding your hands together with palms up and fingers curled. If the curl of the fingers represents a movement from the first or x-axis to the second or y-axis, then the third or z-axis can point along either right thumb or left thumb. History The right-hand rule dates back to the 19th century when it was implemented as a way for identifying the positive direction of coordinate axes in three dime ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Normal (geometry)
In geometry, a normal is an object (e.g. a line, ray, or vector) that is perpendicular to a given object. For example, the normal line to a plane curve at a given point is the infinite straight line perpendicular to the tangent line to the curve at the point. A normal vector is a vector perpendicular to a given object at a particular point. A normal vector of length one is called a unit normal vector or normal direction. A curvature vector is a normal vector whose length is the curvature of the object. Multiplying a normal vector by results in the opposite vector, which may be used for indicating sides (e.g., interior or exterior). In three-dimensional space, a surface normal, or simply normal, to a surface at point is a vector perpendicular to the tangent plane of the surface at . The vector field of normal directions to a surface is known as '' Gauss map''. The word "normal" is also used as an adjective: a line ''normal'' to a plane, the ''normal'' component of ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Infinitesimal
In mathematics, an infinitesimal number is a non-zero quantity that is closer to 0 than any non-zero real number is. The word ''infinitesimal'' comes from a 17th-century Modern Latin coinage ''infinitesimus'', which originally referred to the "infinity- th" item in a sequence. Infinitesimals do not exist in the standard real number system, but they do exist in other number systems, such as the surreal number system and the hyperreal number system, which can be thought of as the real numbers augmented with both infinitesimal and infinite quantities; the augmentations are the reciprocals of one another. Infinitesimal numbers were introduced in the development of calculus, in which the derivative was first conceived as a ratio of two infinitesimal quantities. This definition was not rigorously formalized. As calculus developed further, infinitesimals were replaced by limits, which can be calculated using the standard real numbers. In the 3rd century BC Archimedes used what ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Differential (infinitesimal)
In mathematics, differential refers to several related notions derived from the early days of calculus, put on a rigorous footing, such as infinitesimal differences and the derivatives of functions. The term is used in various branches of mathematics such as calculus, differential geometry, algebraic geometry and algebraic topology. Introduction The term differential is used nonrigorously in calculus to refer to an infinitesimal ("infinitely small") change in some varying quantity. For example, if ''x'' is a variable, then a change in the value of ''x'' is often denoted Δ''x'' (pronounced ''delta x''). The differential ''dx'' represents an infinitely small change in the variable ''x''. The idea of an infinitely small or infinitely slow change is, intuitively, extremely useful, and there are a number of ways to make the notion mathematically precise. Using calculus, it is possible to relate the infinitely small changes of various variables to each other mathematically using ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Continuum Mechanics
Continuum mechanics is a branch of mechanics that deals with the deformation of and transmission of forces through materials modeled as a ''continuous medium'' (also called a ''continuum'') rather than as discrete particles. Continuum mechanics deals with ''deformable bodies'', as opposed to rigid bodies. A continuum model assumes that the substance of the object completely fills the space it occupies. While ignoring the fact that matter is made of atoms, this provides a sufficiently accurate description of matter on length scales much greater than that of inter-atomic distances. The concept of a continuous medium allows for intuitive analysis of bulk matter by using differential equations that describe the behavior of such matter according to physical laws, such as mass conservation, momentum conservation, and energy conservation. Information about the specific material is expressed in constitutive relationships. Continuum mechanics treats the physical properties of ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |