|
Ursescu Theorem
In mathematics, particularly in functional analysis and convex analysis, the Ursescu theorem is a theorem that generalizes the closed graph theorem, the open mapping theorem, and the uniform boundedness principle. Ursescu Theorem The following notation and notions are used, where \mathcal : X \rightrightarrows Y is a set-valued function and S is a non-empty subset of a topological vector space X: * the affine span of S is denoted by \operatorname S and the linear span is denoted by \operatorname S. * S^ := \operatorname_X S denotes the algebraic interior of S in X. * ^S:= \operatorname_ S denotes the relative algebraic interior of S (i.e. the algebraic interior of S in \operatorname(S - S)). * ^S := ^S if \operatorname \left(S - s_0\right) is barreled for some/every s_0 \in S while ^S := \varnothing otherwise. ** If S is convex then it can be shown that for any x \in X, x \in ^ S if and only if the cone generated by S - x is a barreled linear subspace of X or equivalently, ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Functional Analysis
Functional analysis is a branch of mathematical analysis, the core of which is formed by the study of vector spaces endowed with some kind of limit-related structure (e.g. Inner product space#Definition, inner product, Norm (mathematics)#Definition, norm, Topological space#Definition, topology, etc.) and the linear transformation, linear functions defined on these spaces and respecting these structures in a suitable sense. The historical roots of functional analysis lie in the study of function space, spaces of functions and the formulation of properties of transformations of functions such as the Fourier transform as transformations defining continuous function, continuous, unitary operator, unitary etc. operators between function spaces. This point of view turned out to be particularly useful for the study of differential equations, differential and integral equations. The usage of the word ''functional (mathematics), functional'' as a noun goes back to the calculus of variati ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
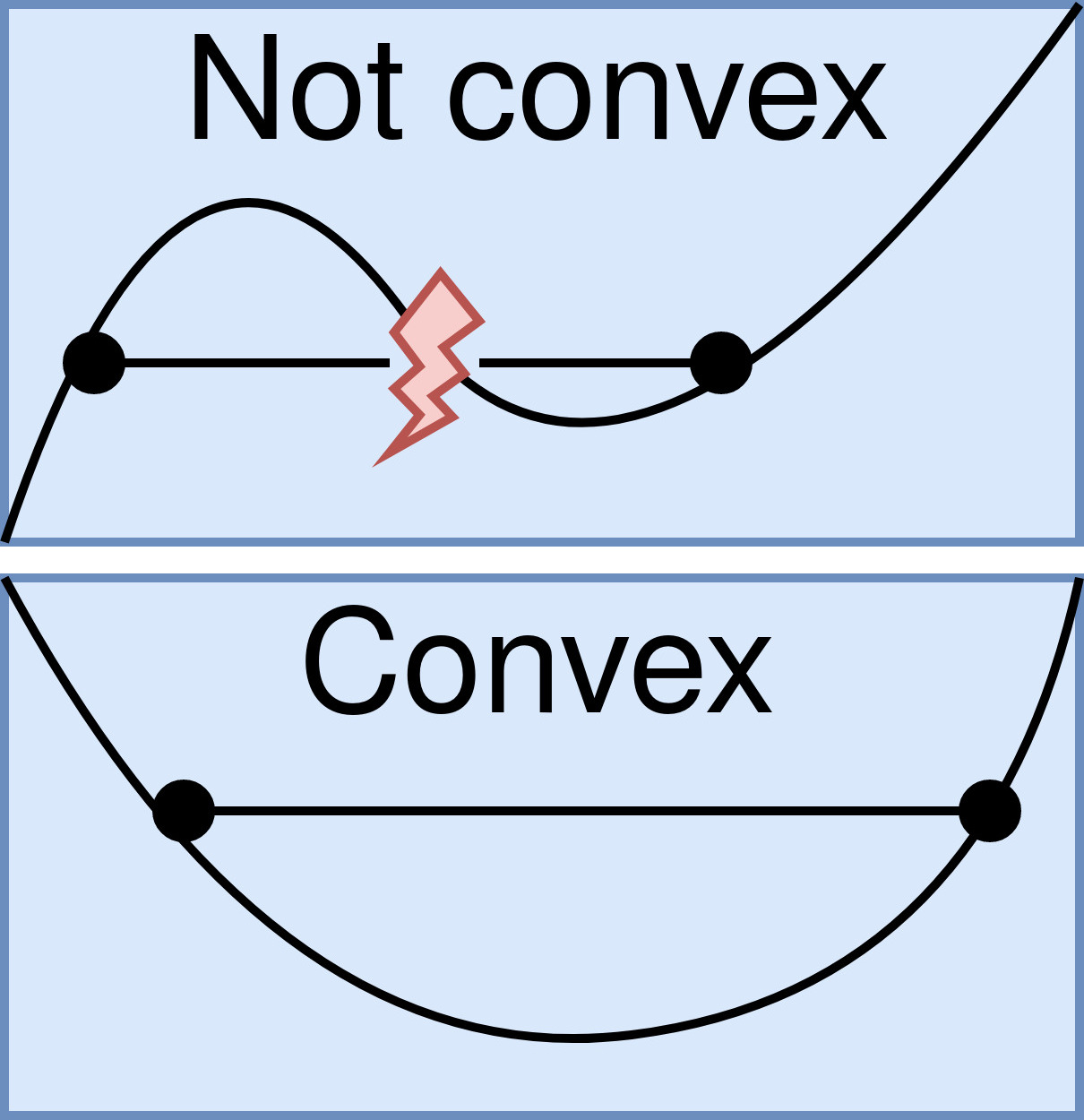
Convex Function
In mathematics, a real-valued function is called convex if the line segment between any two points on the graph of a function, graph of the function lies above the graph between the two points. Equivalently, a function is convex if its epigraph (mathematics), epigraph (the set of points on or above the graph of the function) is a convex set. A twice-differentiable function of a single variable is convex if and only if its second derivative is nonnegative on its entire domain. Well-known examples of convex functions of a single variable include the quadratic function x^2 and the exponential function e^x. In simple terms, a convex function refers to a function whose graph is shaped like a cup \cup, while a concave function's graph is shaped like a cap \cap. Convex functions play an important role in many areas of mathematics. They are especially important in the study of optimization problems where they are distinguished by a number of convenient properties. For instance, a st ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Barreled Space
In functional analysis and related areas of mathematics, a barrelled space (also written barreled space) is a topological vector space (TVS) for which every barrelled set in the space is a neighbourhood for the zero vector. A barrelled set or a barrel in a topological vector space is a set that is convex, balanced, absorbing, and closed. Barrelled spaces are studied because a form of the Banach–Steinhaus theorem still holds for them. Barrelled spaces were introduced by . Barrels A convex and balanced subset of a real or complex vector space is called a and it is said to be , , or . A or a in a topological vector space (TVS) is a subset that is a closed absorbing disk; that is, a barrel is a convex, balanced, closed, and absorbing subset. Every barrel must contain the origin. If \dim X \geq 2 and if S is any subset of X, then S is a convex, balanced, and absorbing set of X if and only if this is all true of S \cap Y in Y for every 2-dimensional vector subspace Y; ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Normed Space
In mathematics, a normed vector space or normed space is a vector space over the real or complex numbers, on which a norm is defined. A norm is the formalization and the generalization to real vector spaces of the intuitive notion of "length" in the real (physical) world. A norm is a real-valued function defined on the vector space that is commonly denoted x\mapsto \, x\, , and has the following properties: #It is nonnegative, meaning that \, x\, \geq 0 for every vector x. #It is positive on nonzero vectors, that is, \, x\, = 0 \text x = 0. # For every vector x, and every scalar \alpha, \, \alpha x\, = , \alpha, \, \, x\, . # The triangle inequality holds; that is, for every vectors x and y, \, x+y\, \leq \, x\, + \, y\, . A norm induces a distance, called its , by the formula d(x,y) = \, y-x\, . which makes any normed vector space into a metric space and a topological vector space. If this metric space is complete then the normed space is a Banach space. Every normed vec ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Convex Series
In mathematics, particularly in functional analysis and convex analysis, a is a series of the form \sum_^ r_i x_i where x_1, x_2, \ldots are all elements of a topological vector space X, and all r_1, r_2, \ldots are non-negative real numbers that sum to 1 (that is, such that \sum_^ r_i = 1). Types of Convex series Suppose that S is a subset of X and \sum_^ r_i x_i is a convex series in X. * If all x_1, x_2, \ldots belong to S then the convex series \sum_^ r_i x_i is called a with elements of S. * If the set \left\ is a (von Neumann) bounded set then the series called a . * The convex series \sum_^ r_i x_i is said to be a if the sequence of partial sums \left(\sum_^n r_i x_i\right)_^ converges in X to some element of X, which is called the . * The convex series is called if \sum_^ r_i x_i is a Cauchy series, which by definition means that the sequence of partial sums \left(\sum_^n r_i x_i\right)_^ is a Cauchy sequence. Types of subsets Convex series allow for the de ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
First Countable
In topology, a branch of mathematics, a first-countable space is a topological space satisfying the "first axiom of countability". Specifically, a space X is said to be first-countable if each point has a countable neighbourhood basis (local base). That is, for each point x in X there exists a sequence N_1, N_2, \ldots of neighbourhoods of x such that for any neighbourhood N of x there exists an integer i with N_i contained in N. Since every neighborhood of any point contains an open neighborhood of that point, the neighbourhood basis can be chosen without loss of generality to consist of open neighborhoods. Examples and counterexamples The majority of 'everyday' spaces in mathematics are first-countable. In particular, every metric space is first-countable. To see this, note that the set of open balls centered at x with radius 1/n for integers form a countable local base at x. An example of a space which is not first-countable is the cofinite topology on an uncountable set ( ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Jim Simons (mathematician)
James Harris Simons (; born 25 April 1938) is an American mathematician, billionaire hedge fund manager, and philanthropist. He is the founder of Renaissance Technologies, a quantitative hedge fund based in East Setauket, New York. He and his fund are known to be quantitative investors, using mathematical models and algorithms to make investment gains from market inefficiencies. Due to the long-term aggregate investment returns of Renaissance and its Medallion Fund, Simons is described as the "greatest investor on Wall Street," and more specifically "the most successful hedge fund manager of all time." As reported by ''Bloomberg Billionaires Index'', Simons' net worth is estimated to be $25.2 billion, making him the 66th-richest person in the world. Simons is known for his studies on pattern recognition. He developed the Chern–Simons form (with Shiing-Shen Chern), and contributed to the development of string theory by providing a theoretical framework to combine geometry ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
First Countable Space
In topology, a branch of mathematics, a first-countable space is a topological space satisfying the "first axiom of countability". Specifically, a space X is said to be first-countable if each point has a countable neighbourhood basis (local base). That is, for each point x in X there exists a sequence N_1, N_2, \ldots of neighbourhoods of x such that for any neighbourhood N of x there exists an integer i with N_i contained in N. Since every neighborhood of any point contains an open neighborhood of that point, the neighbourhood basis can be chosen without loss of generality to consist of open neighborhoods. Examples and counterexamples The majority of 'everyday' spaces in mathematics are first-countable. In particular, every metric space is first-countable. To see this, note that the set of open balls centered at x with radius 1/n for integers form a countable local base at x. An example of a space which is not first-countable is the cofinite topology on an uncountable set ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Series (mathematics)
In mathematics, a series is, roughly speaking, a description of the operation of adding infinitely many quantities, one after the other, to a given starting quantity. The study of series is a major part of calculus and its generalization, mathematical analysis. Series are used in most areas of mathematics, even for studying finite structures (such as in combinatorics) through generating functions. In addition to their ubiquity in mathematics, infinite series are also widely used in other quantitative disciplines such as physics, computer science, statistics and finance. For a long time, the idea that such a potentially infinite summation could produce a finite result was considered paradoxical. This paradox was resolved using the concept of a limit during the 17th century. Zeno's paradox of Achilles and the tortoise illustrates this counterintuitive property of infinite sums: Achilles runs after a tortoise, but when he reaches the position of the tortoise at the beginning of ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Convex Series
In mathematics, particularly in functional analysis and convex analysis, a is a series of the form \sum_^ r_i x_i where x_1, x_2, \ldots are all elements of a topological vector space X, and all r_1, r_2, \ldots are non-negative real numbers that sum to 1 (that is, such that \sum_^ r_i = 1). Types of Convex series Suppose that S is a subset of X and \sum_^ r_i x_i is a convex series in X. * If all x_1, x_2, \ldots belong to S then the convex series \sum_^ r_i x_i is called a with elements of S. * If the set \left\ is a (von Neumann) bounded set then the series called a . * The convex series \sum_^ r_i x_i is said to be a if the sequence of partial sums \left(\sum_^n r_i x_i\right)_^ converges in X to some element of X, which is called the . * The convex series is called if \sum_^ r_i x_i is a Cauchy series, which by definition means that the sequence of partial sums \left(\sum_^n r_i x_i\right)_^ is a Cauchy sequence. Types of subsets Convex series allow for the de ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Open Map
In mathematics, more specifically in topology, an open map is a function between two topological spaces that maps open sets to open sets. That is, a function f : X \to Y is open if for any open set U in X, the image f(U) is open in Y. Likewise, a closed map is a function that maps closed sets to closed sets. A map may be open, closed, both, or neither; in particular, an open map need not be closed and vice versa. Open and closed maps are not necessarily continuous. Further, continuity is independent of openness and closedness in the general case and a continuous function may have one, both, or neither property; this fact remains true even if one restricts oneself to metric spaces. Although their definitions seem more natural, open and closed maps are much less important than continuous maps. Recall that, by definition, a function f : X \to Y is continuous if the preimage of every open set of Y is open in X. (Equivalently, if the preimage of every closed set of Y is closed in ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Uniform Boundedness Principle
In mathematics, the uniform boundedness principle or Banach–Steinhaus theorem is one of the fundamental results in functional analysis. Together with the Hahn–Banach theorem and the open mapping theorem, it is considered one of the cornerstones of the field. In its basic form, it asserts that for a family of continuous linear operators (and thus bounded operators) whose domain is a Banach space, pointwise boundedness is equivalent to uniform boundedness in operator norm. The theorem was first published in 1927 by Stefan Banach and Hugo Steinhaus, but it was also proven independently by Hans Hahn. Theorem The completeness of X enables the following short proof, using the Baire category theorem. There are also simple proofs not using the Baire theorem . Corollaries The above corollary does claim that T_n converges to T in operator norm, that is, uniformly on bounded sets. However, since \left\ is bounded in operator norm, and the limit operator T is continuous ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |