|
Tate Curve
In mathematics, the Tate curve is a curve defined over the ring of formal power series \mathbb q with integer coefficients. Over the open subscheme where ''q'' is invertible, the Tate curve is an elliptic curve. The Tate curve can also be defined for ''q'' as an element of a complete field of norm less than 1, in which case the formal power series converge. The Tate curve was introduced by in a 1959 manuscript originally titled "Rational Points on Elliptic Curves Over Complete Fields"; he did not publish his results until many years later, and his work first appeared in . Definition The Tate curve is the projective plane curve over the ring Z of formal power series with integer coefficients given (in an affine open subset of the projective plane) by the equation : y^2+xy=x^3+a_4x+a_6 where :-a_4=5\sum_n \frac = 5q+45q^2+140q^3+\cdots :-a_6=\sum_\frac\times\frac = q+23q^2+154q^3+\cdots are power series with integer coefficients. The Tate curve over a complete field Suppose that ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Ring Of Formal Power Series
In mathematics, a formal series is an infinite sum that is considered independently from any notion of convergence, and can be manipulated with the usual algebraic operations on series (addition, subtraction, multiplication, division, partial sums, etc.). A formal power series is a special kind of formal series, whose terms are of the form a x^n where x^n is the nth power of a variable x (n is a non-negative integer), and a is called the coefficient. Hence, power series can be viewed as a generalization of polynomials, where the number of terms is allowed to be infinite, with no requirements of convergence. Thus, the series may no longer represent a function of its variable, merely a formal sequence of coefficients, in contrast to a power series, which defines a function by taking numerical values for the variable within a radius of convergence. In a formal power series, the x^n are used only as position-holders for the coefficients, so that the coefficient of x^5 is the fifth ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Elliptic Curve
In mathematics, an elliptic curve is a smooth, projective, algebraic curve of genus one, on which there is a specified point . An elliptic curve is defined over a field and describes points in , the Cartesian product of with itself. If the field's characteristic is different from 2 and 3, then the curve can be described as a plane algebraic curve which consists of solutions for: :y^2 = x^3 + ax + b for some coefficients and in . The curve is required to be non-singular, which means that the curve has no cusps or self-intersections. (This is equivalent to the condition , that is, being square-free in .) It is always understood that the curve is really sitting in the projective plane, with the point being the unique point at infinity. Many sources define an elliptic curve to be simply a curve given by an equation of this form. (When the coefficient field has characteristic 2 or 3, the above equation is not quite general enough to include all non-singular cub ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
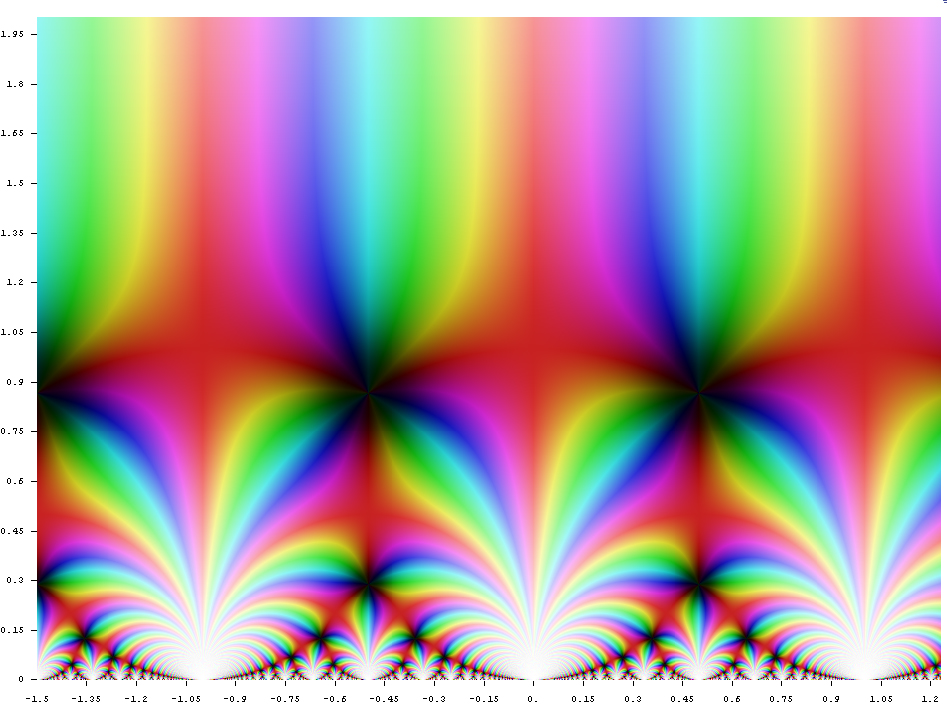
J-invariant
In mathematics, Felix Klein's -invariant or function, regarded as a function of a complex variable , is a modular function of weight zero for defined on the upper half-plane of complex numbers. It is the unique such function which is holomorphic away from a simple pole at the cusp such that :j\left(e^\right) = 0, \quad j(i) = 1728 = 12^3. Rational functions of are modular, and in fact give all modular functions. Classically, the -invariant was studied as a parameterization of elliptic curves over , but it also has surprising connections to the symmetries of the Monster group (this connection is referred to as monstrous moonshine). Definition The -invariant can be defined as a function on the upper half-plane :j(\tau) = 1728 \frac = 1728 \frac = 1728 \frac with the third definition implying j(\tau) can be expressed as a cube, also since 1728 = 12^3. The given functions are the modular discriminant \Delta(\tau) = g_2(\tau)^3 - 27g_3(\tau)^2 = (2\pi)^\,\eta^(\tau) ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Local Field
In mathematics, a field ''K'' is called a (non-Archimedean) local field if it is complete with respect to a topology induced by a discrete valuation ''v'' and if its residue field ''k'' is finite. Equivalently, a local field is a locally compact topological field with respect to a non-discrete topology. Sometimes, real numbers R, and the complex numbers C (with their standard topologies) are also defined to be local fields; this is the convention we will adopt below. Given a local field, the valuation defined on it can be of either of two types, each one corresponds to one of the two basic types of local fields: those in which the valuation is Archimedean and those in which it is not. In the first case, one calls the local field an Archimedean local field, in the second case, one calls it a non-Archimedean local field. Local fields arise naturally in number theory as completions of global fields. While Archimedean local fields have been quite well known in mathematics for a ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Semistable Abelian Variety
In algebraic geometry, a semistable abelian variety is an abelian variety defined over a global or local field, which is characterized by how it reduces at the primes of the field. For an abelian variety A defined over a field F with ring of integers R, consider the Néron model of A, which is a 'best possible' model of A defined over R. This model may be represented as a scheme over \mathrm(R) (cf. spectrum of a ring) for which the generic fibre constructed by means of the morphism \mathrm(F) \to \mathrm(R) gives back A. The Néron model is a smooth group scheme, so we can consider A^0, the connected component of the Néron model which contains the identity for the group law. This is an open subgroup scheme of the Néron model. For a residue field k, A^0_k is a group variety over k, hence an extension of an abelian variety by a linear group. If this linear group is an algebraic torus, so that A^0_k is a semiabelian variety, then A has ''semistable reduction'' at the prime ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Quadratic Twist
In the mathematical field of algebraic geometry, an elliptic curve E over a field K has an associated quadratic twist, that is another elliptic curve which is isomorphic to E over an algebraic closure of K. In particular, an isomorphism between elliptic curves is an isogeny of degree 1, that is an invertible isogeny. Some curves have higher order twists such as cubic and quartic twists. The curve and its twists have the same j-invariant. Applications of twists include cryptography, the solution of Diophantine equations, and when generalized to hyperelliptic curves, the study of the Sato–Tate conjecture. Quadratic twist First assume K is a field of characteristic different from 2. Let E be an elliptic curve over K of the form: : y^2 = x^3 + a_2 x^2 +a_4 x + a_6. \, Given d\neq 0 not a square in K, the quadratic twist of E is the curve E^d, defined by the equation: : dy^2 = x^3 + a_2 x^2 + a_4 x + a_6. \, or equivalently : y^2 = x^3 + d a_2 x^2 + d^2 a_4 x + d^3 a_6 ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Springer-Verlag
Springer Science+Business Media, commonly known as Springer, is a German multinational publishing company of books, e-books and peer-reviewed journals in science, humanities, technical and medical (STM) publishing. Originally founded in 1842 in Berlin, it expanded internationally in the 1960s, and through mergers in the 1990s and a sale to venture capitalists it fused with Wolters Kluwer and eventually became part of Springer Nature in 2015. Springer has major offices in Berlin, Heidelberg, Dordrecht, and New York City. History Julius Springer founded Springer-Verlag in Berlin in 1842 and his son Ferdinand Springer grew it from a small firm of 4 employees into Germany's then second largest academic publisher with 65 staff in 1872.Chronology ". Springer Science+Business Media. In 1964, Springer expanded its business internationall ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Graduate Texts In Mathematics
Graduate Texts in Mathematics (GTM) ( ISSN 0072-5285) is a series of graduate-level textbooks in mathematics published by Springer-Verlag. The books in this series, like the other Springer-Verlag mathematics series, are yellow books of a standard size (with variable numbers of pages). The GTM series is easily identified by a white band at the top of the book. The books in this series tend to be written at a more advanced level than the similar Undergraduate Texts in Mathematics series, although there is a fair amount of overlap between the two series in terms of material covered and difficulty level. List of books #''Introduction to Axiomatic Set Theory'', Gaisi Takeuti, Wilson M. Zaring (1982, 2nd ed., ) #''Measure and Category – A Survey of the Analogies between Topological and Measure Spaces'', John C. Oxtoby (1980, 2nd ed., ) #''Topological Vector Spaces'', H. H. Schaefer, M. P. Wolff (1999, 2nd ed., ) #''A Course in Homological Algebra'', Peter Hilton, Urs Stamm ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |