|
Seasonality
In time series data, seasonality is the presence of variations that occur at specific regular intervals less than a year, such as weekly, monthly, or quarterly. Seasonality may be caused by various factors, such as weather, vacation, and holidays and consists of periodic, repetitive, and generally regular and predictable patterns in the levels of a time series. Seasonal fluctuations in a time series can be contrasted with cyclical patterns. The latter occur when the data exhibits rises and falls that are not of a fixed period. Such non-seasonal fluctuations are usually due to economic conditions and are often related to the "business cycle"; their period usually extends beyond a single year, and the fluctuations are usually of at least two years. Organisations facing seasonal variations, such as ice-cream vendors, are often interested in knowing their performance relative to the normal seasonal variation. Seasonal variations in the labour market can be attributed to the entrance of ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Seasonal Subseries Plot
Seasonal subseries plots are a graphical tool to visualize and detect seasonality in a time series. Seasonal subseries plots involves the extraction of the seasons from a time series into a subseries. Based on a selected periodicity, it is an alternative plot that emphasizes the seasonal patterns are where the data for each season are collected together in separate mini time plots. Seasonal subseries plots enables the underlying seasonal pattern to be seen clearly, and also shows the changes in seasonality over time. Especially, it allows to detect changes between different seasons, changes within a particular season over time. However, this plot is only useful if the Periodic point, period of the seasonality is already known. In many cases, this will in fact be known. For example, monthly data typically has a period of 12. If the period is not known, an autocorrelation plot or spectral plot can be used to determine it. If there is a large number of observations, then a box plot m ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Seasonal Adjustment
Seasonal adjustment or deseasonalization is a statistical method for removing the seasonal component of a time series. It is usually done when wanting to analyse the trend, and cyclical deviations from trend, of a time series independently of the seasonal components. Many economic phenomena have seasonal cycles, such as agricultural production, (crop yields fluctuate with the seasons) and consumer consumption (increased personal spending leading up to Christmas). It is necessary to adjust for this component in order to understand underlying trends in the economy, so official statistics are often adjusted to remove seasonal components. Typically, seasonally adjusted data is reported for unemployment rates to reveal the underlying trends and cycles in labor markets. Time series components The investigation of many economic time series becomes problematic due to seasonal fluctuations. Time series are made up of four components: *S_t: The seasonal component *T_t: The trend component ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Time Series
In mathematics, a time series is a series of data points indexed (or listed or graphed) in time order. Most commonly, a time series is a sequence taken at successive equally spaced points in time. Thus it is a sequence of discrete-time data. Examples of time series are heights of ocean tides, counts of sunspots, and the daily closing value of the Dow Jones Industrial Average. A time series is very frequently plotted via a run chart (which is a temporal line chart). Time series are used in statistics, signal processing, pattern recognition, econometrics, mathematical finance, weather forecasting, earthquake prediction, electroencephalography, control engineering, astronomy, communications engineering, and largely in any domain of applied science and engineering which involves temporal measurements. Time series ''analysis'' comprises methods for analyzing time series data in order to extract meaningful statistics and other characteristics of the data. Time series ''forecasting' ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Time-series
In mathematics, a time series is a series of data points indexed (or listed or graphed) in time order. Most commonly, a time series is a sequence taken at successive equally spaced points in time. Thus it is a sequence of discrete-time data. Examples of time series are heights of ocean tides, counts of sunspots, and the daily closing value of the Dow Jones Industrial Average. A time series is very frequently plotted via a run chart (which is a temporal line chart). Time series are used in statistics, signal processing, pattern recognition, econometrics, mathematical finance, weather forecasting, earthquake prediction, electroencephalography, control engineering, astronomy, communications engineering, and largely in any domain of applied science and engineering which involves temporal measurements. Time series ''analysis'' comprises methods for analyzing time series data in order to extract meaningful statistics and other characteristics of the data. Time series ''forecasting'' ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Time Series Analysis
In mathematics, a time series is a series of data points indexed (or listed or graphed) in time order. Most commonly, a time series is a sequence taken at successive equally spaced points in time. Thus it is a sequence of discrete-time data. Examples of time series are heights of ocean tides, counts of sunspots, and the daily closing value of the Dow Jones Industrial Average. A time series is very frequently plotted via a run chart (which is a temporal line chart). Time series are used in statistics, signal processing, pattern recognition, econometrics, mathematical finance, weather forecasting, earthquake prediction, electroencephalography, control engineering, astronomy, communications engineering, and largely in any domain of applied science and engineering which involves temporal measurements. Time series ''analysis'' comprises methods for analyzing time series data in order to extract meaningful statistics and other characteristics of the data. Time series ''forecasting'' ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Decomposition Of Time Series
The decomposition of time series is a statistical task that deconstructs a time series into several components, each representing one of the underlying categories of patterns. There are two principal types of decomposition, which are outlined below. Decomposition based on rates of change This is an important technique for all types of time series analysis, especially for seasonal adjustment. It seeks to construct, from an observed time series, a number of component series (that could be used to reconstruct the original by additions or multiplications) where each of these has a certain characteristic or type of behavior. For example, time series are usually decomposed into: *T_t, the trend component at time ''t'', which reflects the long-term progression of the series (secular variation). A trend exists when there is a persistent increasing or decreasing direction in the data. The trend component does not have to be linear. *C_t, the cyclical component at time ''t'', which refle ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
X-12-ARIMA
X-13ARIMA-SEATS, successor to X-12-ARIMA and X-11, is a set of statistical methods for seasonal adjustment and other descriptive analysis of time series data that are implemented in the U.S. Census Bureau's software package. These methods are or have been used by Statistics Canada, Australian Bureau of Statistics, and the statistical offices of many other countries. X-12-ARIMA can be used together with many statistical packages, such as SAS in its econometric and time series (ETS) package, R in its (seasonal) package, Gretl or EViews which provides a graphical user interface for X-12-ARIMA, and NumXL which avails X-12-ARIMA functionality in Microsoft Excel. There is also a version for Matlab. Notable statistical agencies presently using X-12-ARIMA for seasonal adjustment include Statistics Canada, the U.S. Bureau of Labor Statistics and Census and Statistics Department (Hong Kong). The Brazilian Institute of Geography and Statistics uses X-13-ARIMA. X-12-ARIMA was the successo ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
ARIMA
Arima, officially The Royal Chartered Borough of Arima is the easternmost and second largest in area of the three boroughs of Trinidad and Tobago. It is geographically adjacent to Sangre Grande and Arouca at the south central foothills of the Northern Range. To the south is the Caroni–Arena Dam. Coterminous with Town of Arima since 1888, the borough of Arima is the fourth-largest municipality in population in the country (after Port of Spain, Chaguanas and San Fernando). The census estimated it had 33,606 residents in 2011. In 1887, the town petitioned Queen Victoria for municipal status as part of her Golden Jubilee celebration. This was granted in the following year, and Arima became a Royal Borough on 1 August 1888. Historically the third-largest town of Trinidad and Tobago, Arima is fourth since Chaguanas became the largest town in the country. Geography Climate The borough has a tropical rainforest climate (Köppen ''Af''), bordering on a tropical monsoon climate, ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Cyclostationary Process
A cyclostationary process is a signal having statistical properties that vary cyclically with time. A cyclostationary process can be viewed as multiple interleaved stationary processes. For example, the maximum daily temperature in New York City can be modeled as a cyclostationary process: the maximum temperature on July 21 is statistically different from the temperature on December 20; however, it is a reasonable approximation that the temperature on December 20 of different years has identical statistics. Thus, we can view the random process composed of daily maximum temperatures as 365 interleaved stationary processes, each of which takes on a new value once per year. Definition There are two differing approaches to the treatment of cyclostationary processes. The probabilistic approach is to view measurements as an instance of a stochastic process. As an alternative, the deterministic approach is to view the measurements as a single time series, from which a probability distri ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Dependent Variable
Dependent and independent variables are variables in mathematical modeling, statistical modeling and experimental sciences. Dependent variables receive this name because, in an experiment, their values are studied under the supposition or demand that they depend, by some law or rule (e.g., by a mathematical function), on the values of other variables. Independent variables, in turn, are not seen as depending on any other variable in the scope of the experiment in question. In this sense, some common independent variables are time, space, density, mass, fluid flow rate, and previous values of some observed value of interest (e.g. human population size) to predict future values (the dependent variable). Of the two, it is always the dependent variable whose variation is being studied, by altering inputs, also known as regressors in a statistical context. In an experiment, any variable that can be attributed a value without attributing a value to any other variable is called an in ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
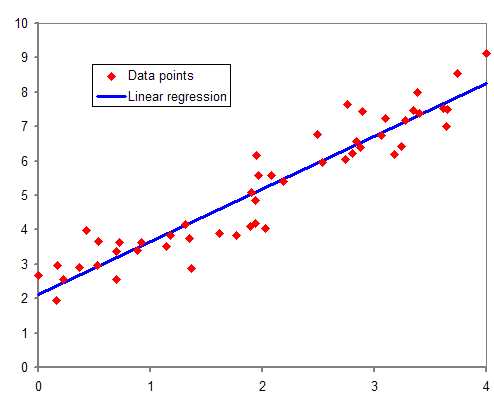
Regression Analysis
In statistical modeling, regression analysis is a set of statistical processes for estimating the relationships between a dependent variable (often called the 'outcome' or 'response' variable, or a 'label' in machine learning parlance) and one or more independent variables (often called 'predictors', 'covariates', 'explanatory variables' or 'features'). The most common form of regression analysis is linear regression, in which one finds the line (or a more complex linear combination) that most closely fits the data according to a specific mathematical criterion. For example, the method of ordinary least squares computes the unique line (or hyperplane) that minimizes the sum of squared differences between the true data and that line (or hyperplane). For specific mathematical reasons (see linear regression), this allows the researcher to estimate the conditional expectation (or population average value) of the dependent variable when the independent variables take on a given ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Ordinary Least Squares
In statistics, ordinary least squares (OLS) is a type of linear least squares method for choosing the unknown parameters in a linear regression model (with fixed level-one effects of a linear function of a set of explanatory variables) by the principle of least squares: minimizing the sum of the squares of the differences between the observed dependent variable (values of the variable being observed) in the input dataset and the output of the (linear) function of the independent variable. Geometrically, this is seen as the sum of the squared distances, parallel to the axis of the dependent variable, between each data point in the set and the corresponding point on the regression surface—the smaller the differences, the better the model fits the data. The resulting estimator can be expressed by a simple formula, especially in the case of a simple linear regression, in which there is a single regressor on the right side of the regression equation. The OLS estimator is consiste ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |