|
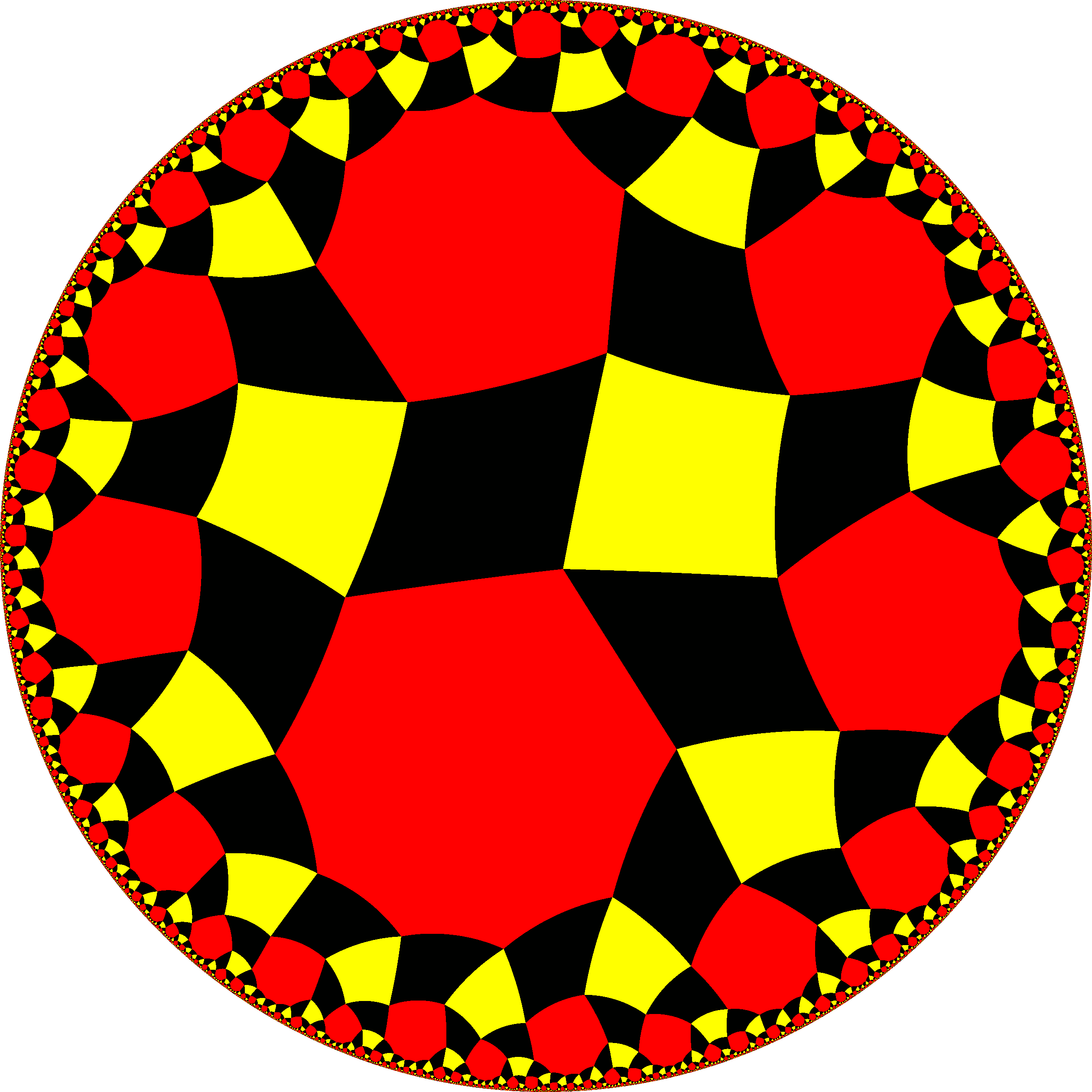
Snub Tetraoctagonal Tiling
In geometry, the snub tetraoctagonal tiling is a uniform tiling of the hyperbolic plane. It has Schläfli symbol of sr. Images Drawn in chiral pairs, with edges missing between black triangles: : Related polyhedra and tiling The ''snub tetraoctagonal tiling '' is seventh in a series of snub polyhedra and tilings with vertex figure 3.3.4.3.''n''. References * John H. Conway, Heidi Burgiel, Chaim Goodman-Strass, ''The Symmetries of Things'' 2008, (Chapter 19, The Hyperbolic Archimedean Tessellations) * See also *Square tiling *Tilings of regular polygons *List of uniform planar tilings *List of regular polytopes This article lists the regular polytopes and regular polytope compounds in Euclidean geometry, Euclidean, spherical geometry, spherical and hyperbolic geometry, hyperbolic spaces. The Schläfli symbol describes every regular tessellation of an ' ... External links * * Hyperbolic and Spherical Tiling Gallery* ttp://www.plunk.org/~hatch/HyperbolicTesselation ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Geometry
Geometry (; ) is, with arithmetic, one of the oldest branches of mathematics. It is concerned with properties of space such as the distance, shape, size, and relative position of figures. A mathematician who works in the field of geometry is called a ''geometer''. Until the 19th century, geometry was almost exclusively devoted to Euclidean geometry, which includes the notions of point, line, plane, distance, angle, surface, and curve, as fundamental concepts. During the 19th century several discoveries enlarged dramatically the scope of geometry. One of the oldest such discoveries is Carl Friedrich Gauss' ("remarkable theorem") that asserts roughly that the Gaussian curvature of a surface is independent from any specific embedding in a Euclidean space. This implies that surfaces can be studied ''intrinsically'', that is, as stand-alone spaces, and has been expanded into the theory of manifolds and Riemannian geometry. Later in the 19th century, it appeared that geometries ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Hyperbolic Geometry
In mathematics, hyperbolic geometry (also called Lobachevskian geometry or Bolyai– Lobachevskian geometry) is a non-Euclidean geometry. The parallel postulate of Euclidean geometry is replaced with: :For any given line ''R'' and point ''P'' not on ''R'', in the plane containing both line ''R'' and point ''P'' there are at least two distinct lines through ''P'' that do not intersect ''R''. (Compare the above with Playfair's axiom, the modern version of Euclid's parallel postulate.) Hyperbolic plane geometry is also the geometry of pseudospherical surfaces, surfaces with a constant negative Gaussian curvature. Saddle surfaces have negative Gaussian curvature in at least some regions, where they locally resemble the hyperbolic plane. A modern use of hyperbolic geometry is in the theory of special relativity, particularly the Minkowski model. When geometers first realised they were working with something other than the standard Euclidean geometry, they described their geomet ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Schläfli Symbol
In geometry, the Schläfli symbol is a notation of the form \ that defines regular polytopes and tessellations. The Schläfli symbol is named after the 19th-century Swiss mathematician Ludwig Schläfli, who generalized Euclidean geometry to more than three dimensions and discovered all their convex regular polytopes, including the six that occur in four dimensions. Definition The Schläfli symbol is a recursive description, starting with for a ''p''-sided regular polygon that is convex. For example, is an equilateral triangle, is a square, a convex regular pentagon, etc. Regular star polygons are not convex, and their Schläfli symbols contain irreducible fractions ''p''/''q'', where ''p'' is the number of vertices, and ''q'' is their turning number. Equivalently, is created from the vertices of , connected every ''q''. For example, is a pentagram; is a pentagon. A regular polyhedron that has ''q'' regular ''p''-sided Face (geometry), polygon faces around each Verte ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
H2 Snub 248a
H, or h, is the eighth letter in the Latin alphabet, used in the modern English alphabet, the alphabets of other western European languages and others worldwide. Its name in English is ''aitch'' (pronounced , plural ''aitches''), or regionally ''haitch'' ."H" ''Oxford English Dictionary,'' 2nd edition (1989); ''Merriam-Webster's Third New International Dictionary of the English Language, Unabridged'' (1993); "aitch" or "haitch", op. cit. History The original Semitic letter Heth most likely represented the voiceless pharyngeal fricative (). The form of the letter probably stood for a fence or posts. The Greek Eta 'Η' in archaic Greek alphabets, before coming to represent a long vowel, , still represented a similar sound, the voiceless glottal fricative . In this context, the letter eta is also known as Heta to underline this fact. Thus, in the Old Italic alphabets, the letter Heta of the Euboean alphabet was adopted with its original sound value . While Etruscan and La ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
H2 Snub 248b
H, or h, is the eighth letter in the Latin alphabet, used in the modern English alphabet, the alphabets of other western European languages and others worldwide. Its name in English is ''aitch'' (pronounced , plural ''aitches''), or regionally ''haitch'' ."H" ''Oxford English Dictionary,'' 2nd edition (1989); ''Merriam-Webster's Third New International Dictionary of the English Language, Unabridged'' (1993); "aitch" or "haitch", op. cit. History The original Semitic letter Heth most likely represented the voiceless pharyngeal fricative (). The form of the letter probably stood for a fence or posts. The Greek Eta 'Η' in archaic Greek alphabets, before coming to represent a long vowel, , still represented a similar sound, the voiceless glottal fricative . In this context, the letter eta is also known as Heta to underline this fact. Thus, in the Old Italic alphabets, the letter Heta of the Euboean alphabet was adopted with its original sound value . While Etruscan and La ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Vertex Figure
In geometry, a vertex figure, broadly speaking, is the figure exposed when a corner of a polyhedron or polytope is sliced off. Definitions Take some corner or Vertex (geometry), vertex of a polyhedron. Mark a point somewhere along each connected edge. Draw lines across the connected faces, joining adjacent points around the face. When done, these lines form a complete circuit, i.e. a polygon, around the vertex. This polygon is the vertex figure. More precise formal definitions can vary quite widely, according to circumstance. For example Coxeter (e.g. 1948, 1954) varies his definition as convenient for the current area of discussion. Most of the following definitions of a vertex figure apply equally well to infinite tessellation, tilings or, by extension, to Honeycomb (geometry), space-filling tessellation with polytope Cell (geometry), cells and other higher-dimensional polytopes. As a flat slice Make a slice through the corner of the polyhedron, cutting through all the edges ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
John Horton Conway
John Horton Conway (26 December 1937 – 11 April 2020) was an English mathematician active in the theory of finite groups, knot theory, number theory, combinatorial game theory and coding theory. He also made contributions to many branches of recreational mathematics, most notably the invention of the cellular automaton called the Game of Life. Born and raised in Liverpool, Conway spent the first half of his career at the University of Cambridge before moving to the United States, where he held the John von Neumann Professorship at Princeton University for the rest of his career. On 11 April 2020, at age 82, he died of complications from COVID-19. Early life and education Conway was born on 26 December 1937 in Liverpool, the son of Cyril Horton Conway and Agnes Boyce. He became interested in mathematics at a very early age. By the time he was 11, his ambition was to become a mathematician. After leaving sixth form, he studied mathematics at Gonville and Caius College, Camb ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Square Tiling
In geometry, the square tiling, square tessellation or square grid is a regular tiling of the Euclidean plane. It has Schläfli symbol of meaning it has 4 squares around every vertex. Conway called it a quadrille. The internal angle of the square is 90 degrees so four squares at a point make a full 360 degrees. It is one of three regular tilings of the plane. The other two are the triangular tiling and the hexagonal tiling. Uniform colorings There are 9 distinct uniform colorings of a square tiling. Naming the colors by indices on the 4 squares around a vertex: 1111, 1112(i), 1112(ii), 1122, 1123(i), 1123(ii), 1212, 1213, 1234. (i) cases have simple reflection symmetry, and (ii) glide reflection symmetry. Three can be seen in the same symmetry domain as reduced colorings: 1112i from 1213, 1123i from 1234, and 1112ii reduced from 1123ii. Related polyhedra and tilings This tiling is topologically related as a part of sequence of regular polyhedra and tilings, extending ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Tilings Of Regular Polygons
Euclidean plane tilings by convex regular polygons have been widely used since antiquity. The first systematic mathematical treatment was that of Kepler in his ''Harmonices Mundi'' (Latin: ''The Harmony of the World'', 1619). Notation of Euclidean tilings Euclidean tilings are usually named after Cundy & Rollett’s notation. This notation represents (i) the number of vertices, (ii) the number of polygons around each vertex (arranged clockwise) and (iii) the number of sides to each of those polygons. For example: 36; 36; 34.6, tells us there are 3 vertices with 2 different vertex types, so this tiling would be classed as a ‘3-uniform (2-vertex types)’ tiling. Broken down, 36; 36 (both of different transitivity class), or (36)2, tells us that there are 2 vertices (denoted by the superscript 2), each with 6 equilateral 3-sided polygons (triangles). With a final vertex 34.6, 4 more contiguous equilateral triangles and a single regular hexagon. However, this notation has two ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
List Of Uniform Planar Tilings
This table shows the 11 convex uniform tilings (regular and semiregular) of the Euclidean plane, and their dual tilings. There are three regular and eight semiregular tilings in the plane. The semiregular tilings form new tilings from their duals, each made from one type of irregular face. John Conway calls these uniform duals ''Catalan tilings'', in parallel to the Catalan solid polyhedra. Uniform tilings are listed by their vertex configuration, the sequence of faces that exist on each vertex. For example ''4.8.8'' means one square and two octagons on a vertex. These 11 uniform tilings have 32 different ''uniform colorings''. A uniform coloring allows identical sided polygons at a vertex to be colored differently, while still maintaining vertex-uniformity and transformational congruence between vertices. (Note: Some of the tiling images shown below are ''not'' color-uniform) In addition to the 11 convex uniform tilings, there are also 14 known nonconvex tilings, using sta ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
List Of Regular Polytopes
This article lists the regular polytopes and regular polytope compounds in Euclidean geometry, Euclidean, spherical geometry, spherical and hyperbolic geometry, hyperbolic spaces. The Schläfli symbol describes every regular tessellation of an ''n''-sphere, Euclidean and hyperbolic spaces. A Schläfli symbol describing an ''n''-polytope equivalently describes a tessellation of an (''n'' − 1)-sphere. In addition, the symmetry of a regular polytope or tessellation is expressed as a Coxeter group, which Coxeter expressed identically to the Schläfli symbol, except delimiting by square brackets, a notation that is called Coxeter notation. Another related symbol is the Coxeter-Dynkin diagram which represents a symmetry group with no rings, and the represents regular polytope or tessellation with a ring on the first node. For example, the cube has Schläfli symbol , and with its octahedral symmetry, [4,3] or , it is represented by Coxeter diagram . The regular polytopes are ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Chiral Figures
Chirality is a property of asymmetry important in several branches of science. The word ''chirality'' is derived from the Greek language, Greek (''kheir''), "hand", a familiar chiral object. An object or a system is ''chiral'' if it is distinguishable from its mirror image; that is, it cannot be wikt:superimpose, superimposed onto it. Conversely, a mirror image of an ''achiral'' object, such as a sphere, cannot be distinguished from the object. A chiral object and its mirror image are called ''enantiomorphs'' (Greek, "opposite forms") or, when referring to molecules, ''enantiomers''. A non-chiral object is called ''achiral'' (sometimes also ''amphichiral'') and can be superposed on its mirror image. The term was first used by William Thomson, 1st Baron Kelvin, Lord Kelvin in 1893 in the second Robert Boyle Lecture at the Oxford University Junior Scientific Club which was published in 1894: Human hands are perhaps the most recognized example of chirality. The left hand is a ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |