|
Reciprocal Lattice
In physics, the reciprocal lattice represents the Fourier transform of another lattice (group) (usually a Bravais lattice). In normal usage, the initial lattice (whose transform is represented by the reciprocal lattice) is a periodic spatial function in real space known as the ''direct lattice''. While the direct lattice exists in real space and is commonly understood to be a physical lattice (such as the lattice of a crystal), the reciprocal lattice exists in the space of spatial frequencies known as reciprocal space or k space, where \mathbf refers to the wavevector. In quantum physics, reciprocal space is closely related to momentum space according to the proportionality \mathbf = \hbar \mathbf, where \mathbf is the momentum vector and \hbar is the Planck constant. The reciprocal lattice of a reciprocal lattice is equivalent to the original direct lattice, because the defining equations are symmetrical with respect to the vectors in real and reciprocal space. Mathematical ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Reciprocal Monoclinic Lattice
Reciprocal may refer to: In mathematics * Multiplicative inverse, in mathematics, the number 1/''x'', which multiplied by ''x'' gives the product 1, also known as a ''reciprocal'' * Reciprocal polynomial, a polynomial obtained from another polynomial by reversing its coefficients * Reciprocal rule, a technique in calculus for calculating derivatives of reciprocal functions * Hyperbolic spiral, Reciprocal spiral, a plane curve * Reciprocal averaging, a statistical technique for aggregating categorical data In science and technology * Reciprocal aircraft heading, 180 degrees (the opposite direction) from a stated heading * Reciprocal lattice, a basis for the dual space of covectors, in crystallography * Reciprocal length, a measurement used in science * Reciprocating engine or piston engine * Reciprocating oscillation in physical wave theory Life sciences and medicine * Hybrid (biology), in genetics, the result of a reciprocal pair of crossings, forming ''reciprocal hybrids'' ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Neutron Diffraction
Neutron diffraction or elastic neutron scattering is the application of neutron scattering to the determination of the atomic and/or magnetic structure of a material. A sample to be examined is placed in a beam of thermal or cold neutrons to obtain a diffraction pattern that provides information of the structure of the material. The technique is similar to X-ray diffraction but due to their different scattering properties, neutrons and X-rays provide complementary information: X-Rays are suited for superficial analysis, strong x-rays from synchrotron radiation are suited for shallow depths or thin specimens, while neutrons having high penetration depth are suited for bulk samples.Measurement of residual stress in materials using neutrons |
Wavefront
In physics, the wavefront of a time-varying ''wave field'' is the set ( locus) of all points having the same '' phase''. The term is generally meaningful only for fields that, at each point, vary sinusoidally in time with a single temporal frequency (otherwise the phase is not well defined). Wavefronts usually move with time. For waves propagating in a unidimensional medium, the wavefronts are usually single points; they are curves in a two dimensional medium, and surfaces in a three-dimensional one. For a sinusoidal plane wave, the wavefronts are planes perpendicular to the direction of propagation, that move in that direction together with the wave. For a sinusoidal spherical wave, the wavefronts are spherical surfaces that expand with it. If the speed of propagation is different at different points of a wavefront, the shape and/or orientation of the wavefronts may change by refraction. In particular, lenses can change the shape of optical wavefronts from planar to ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Angular Frequency
In physics, angular frequency "''ω''" (also referred to by the terms angular speed, circular frequency, orbital frequency, radian frequency, and pulsatance) is a scalar measure of rotation rate. It refers to the angular displacement per unit time (for example, in rotation) or the rate of change of the phase of a sinusoidal waveform (for example, in oscillations and waves), or as the rate of change of the argument of the sine function. Angular frequency (or angular speed) is the magnitude of the pseudovector quantity angular velocity.(UP1) One turn is equal to 2''π'' radians, hence \omega = \frac = , where: *''ω'' is the angular frequency (unit: radians per second), *''T'' is the period (unit: seconds), *''f'' is the ordinary frequency (unit: hertz) (sometimes ''ν''). Units In SI units, angular frequency is normally presented in radians per second, even when it does not express a rotational value. The unit hertz (Hz) is dimensionally equivalent, but by conven ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Angular Wavenumber
In the physical sciences, the wavenumber (also wave number or repetency) is the ''spatial frequency'' of a wave, measured in cycles per unit distance (ordinary wavenumber) or radians per unit distance (angular wavenumber). It is analogous to temporal frequency, which is defined as the number of wave cycles per unit time (''ordinary frequency'') or radians per unit time (''angular frequency''). In multidimensional systems, the wavenumber is the magnitude of the ''wave vector''. The space of wave vectors is called '' reciprocal space''. Wave numbers and wave vectors play an essential role in optics and the physics of wave scattering, such as X-ray diffraction, neutron diffraction, electron diffraction, and elementary particle physics. For quantum mechanical waves, the wavenumber multiplied by the reduced Planck's constant is the ''canonical momentum''. Wavenumber can be used to specify quantities other than spatial frequency. For example, in optical spectroscopy, it is often use ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
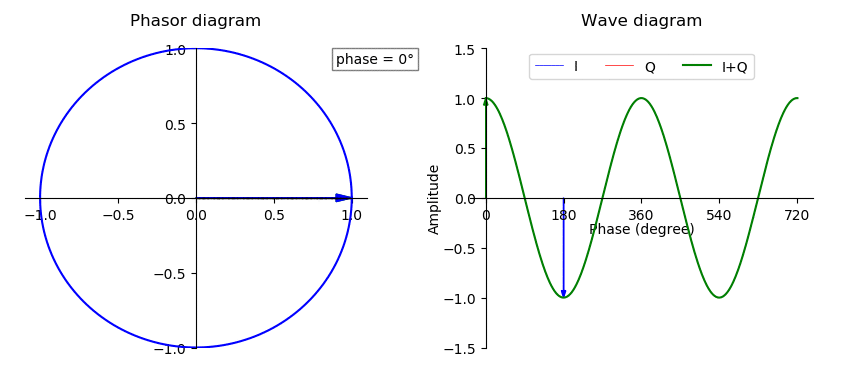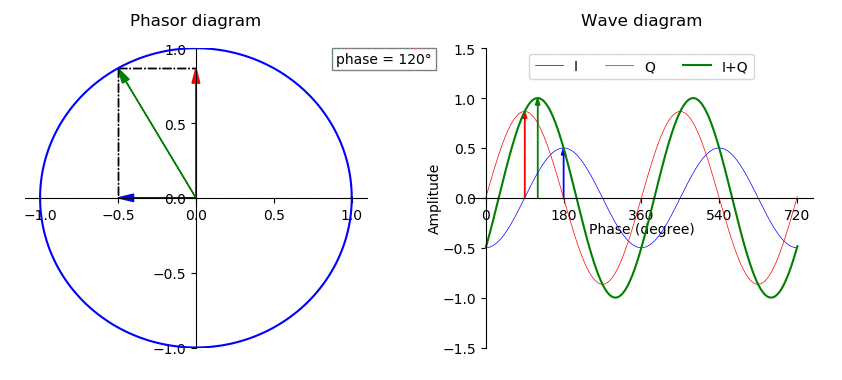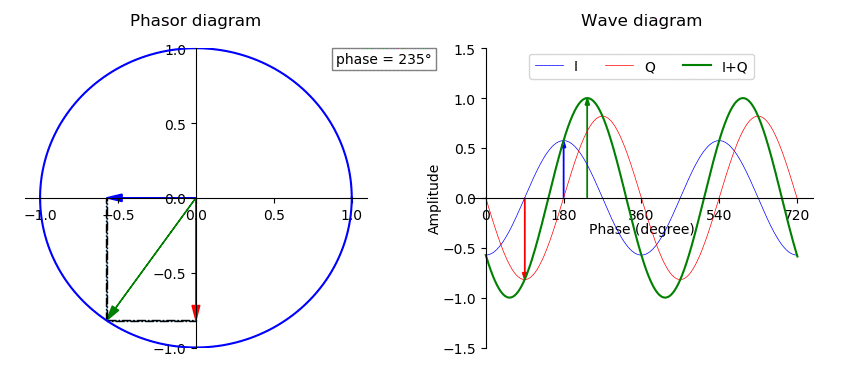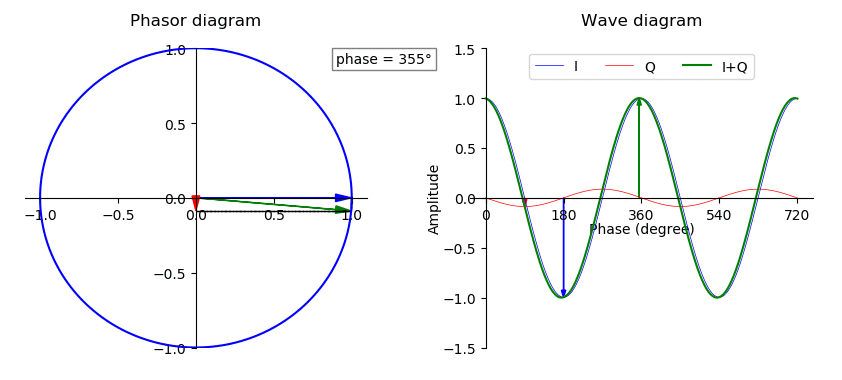
Phase (waves)
In physics and mathematics, the phase of a periodic function F of some real variable t (such as time) is an angle-like quantity representing the fraction of the cycle covered up to t. It is denoted \phi(t) and expressed in such a scale that it varies by one full turn as the variable t goes through each period (and F(t) goes through each complete cycle). It may be measured in any angular unit such as degrees or radians, thus increasing by 360° or 2\pi as the variable t completes a full period. This convention is especially appropriate for a sinusoidal function, since its value at any argument t then can be expressed as \phi(t), the sine of the phase, multiplied by some factor (the amplitude of the sinusoid). (The cosine may be used instead of sine, depending on where one considers each period to start.) Usually, whole turns are ignored when expressing the phase; so that \phi(t) is also a periodic function, with the same period as F, that repeatedly scans the same range ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Sinusoidal Plane Wave
In physics, a sinusoidal (or monochromatic) plane wave is a special case of plane wave: a field whose value varies as a sinusoidal function of time and of the distance from some fixed plane. For any position \vec x in space and any time t, the value of such a field can be written as :F(\vec x, t)=A \cos\left(2\pi \nu (\vec x \cdot \vec n - c t) + \varphi\right)\, where \vec n is a unit-length vector, the direction of propagation of the wave, and "\cdot" denotes the dot product of two vectors. The parameter A, which may be a scalar or a vector, is called the amplitude of the wave; the coefficient \nu, a positive scalar, its spatial frequency; and the adimensional scalar \varphi, an angle in radians, is its initial phase or phase shift. The scalar quantity d = \vec x \cdot \vec n gives the (signed) displacement of the point \vec x from the plane that is perpendicular to \vec n and goes through the origin of the coordinate system. This quantity is constant over each plane perpendi ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Frequency Domain
In physics, electronics, control systems engineering, and statistics, the frequency domain refers to the analysis of mathematical functions or signals with respect to frequency, rather than time. Put simply, a time-domain graph shows how a signal changes over time, whereas a frequency-domain graph shows how much of the signal lies within each given frequency band over a range of frequencies. A frequency-domain representation can also include information on the phase shift that must be applied to each sinusoid in order to be able to recombine the frequency components to recover the original time signal. A given function or signal can be converted between the time and frequency domains with a pair of mathematical operators called transforms. An example is the Fourier transform, which converts a time function into a complex valued sum or integral of sine waves of different frequencies, with amplitudes and phases, each of which represents a frequency component. The "spec ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Brillouin Zone
In mathematics and solid state physics, the first Brillouin zone is a uniquely defined primitive cell in reciprocal space. In the same way the Bravais lattice is divided up into Wigner–Seitz cells in the real lattice, the reciprocal lattice is broken up into Brillouin zones. The boundaries of this cell are given by planes related to points on the reciprocal lattice. The importance of the Brillouin zone stems from the description of waves in a periodic medium given by Bloch's theorem, in which it is found that the solutions can be completely characterized by their behavior in a single Brillouin zone. The first Brillouin zone is the locus of points in reciprocal space that are closer to the origin of the reciprocal lattice than they are to any other reciprocal lattice points (see the derivation of the Wigner–Seitz cell). Another definition is as the set of points in ''k''-space that can be reached from the origin without crossing any Bragg plane. Equivalently, this is the ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Laue Equations
In crystallography and solid state physics, the Laue equations relate incoming waves to outgoing waves in the process of elastic scattering, where the photon energy or light temporal frequency does not change by scattering, by a crystal lattice. They are named after physicist Max von Laue (1879–1960). The Laue equations can be written as \mathbf= \mathbf_ - \mathbf_ = \mathbf as the condition of elastic wave scattering by a crystal lattice, where \mathbf_, \mathbf k_, and \mathbf are an incoming (to the crystal) wavevector, an outgoing (from the crystal by scattering) wavevector, and a reciprocal lattice vector for the crystal respectively. Due to elastic scattering , \mathbf_, ^2=, \mathbf_, ^2, three vectors. \mathbf, \mathbf_, and -\mathbf_ , form a rhombus if the equation is satisfied. If the scattering satisfies this equation, all the crystal lattice points scatter the incoming wave toward the scattering direction (the direction along \mathbf k_). If the equation is not sati ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |

.jpg)

.gif)