|
Quillen's Q-construction
In algebra, Quillen's Q-construction associates to an exact category (e.g., an abelian category) an algebraic K-theory. More precisely, given an exact category ''C'', the construction creates a topological space B^+C so that \pi_0 (B^+C) is the Grothendieck group of ''C'' and, when ''C'' is the category of finitely generated projective modules over a ring ''R'', for i = 0, 1, 2, \pi_i (B^+C) is the ''i''-th K-group of ''R'' in the classical sense. (The notation "+" is meant to suggest the construction adds more to the classifying space ''BC''.) One puts :K_i(C) = \pi_i(B^+C) and call it the ''i''-th K-group of ''C''. Similarly, the ''i''-th K-group of ''C'' with coefficients in a group ''G'' is defined as the homotopy group with coefficients: :K_i(C; G) = \pi_i(B^+ C; G). The construction is widely applicable and is used to define an algebraic K-theory in a non-classical context. For example, one can define equivariant algebraic K-theory as \pi_* of B^+ of the category of equivari ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Daniel Quillen
Daniel Gray "Dan" Quillen (June 22, 1940 – April 30, 2011) was an American mathematician. He is known for being the "prime architect" of higher algebraic ''K''-theory, for which he was awarded the Cole Prize in 1975 and the Fields Medal in 1978. From 1984 to 2006, he was the Waynflete Professor of Pure Mathematics at Magdalen College, Oxford. Education and career Quillen was born in Orange, New Jersey, and attended Newark Academy. He entered Harvard University, where he earned both his AB, in 1961, and his PhD in 1964; the latter completed under the supervision of Raoul Bott, with a thesis in partial differential equations. He was a Putnam Fellow in 1959. Quillen obtained a position at the Massachusetts Institute of Technology after completing his doctorate. He also spent a number of years at several other universities. He visited France twice: first as a Sloan Fellow in Paris, during the academic year 1968–69, where he was greatly influenced by Grothendieck, and the ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
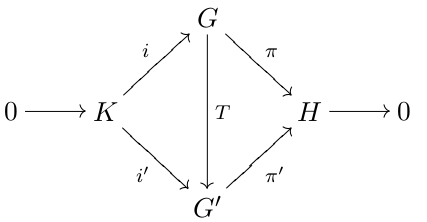
Classifying Space Of A Category
A nerve is an enclosed, cable-like bundle of nerve fibers (called axons) in the peripheral nervous system. Axons transmit electrical impulses. Nerves have historically been considered the basic units of the peripheral nervous system. A nerve provides a common pathway for the electrochemical nerve impulses called action potentials that are transmitted along each of the axons to peripheral organs or, in the case of sensory nerves, from the periphery back to the central nervous system. Each axon, within the nerve, is an extension of an individual neuron, along with other supportive cells such as some Schwann cells that coat the axons in myelin. Within a nerve, each axon is surrounded by a layer of connective tissue called the endoneurium. The axons are bundled together into groups called fascicles, and each fascicle is wrapped in a layer of connective tissue called the perineurium. Finally, the entire nerve is wrapped in a layer of connective tissue called the epineurium. Nerve c ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Serre Spectral Sequence
In mathematics, the Serre spectral sequence (sometimes Leray–Serre spectral sequence to acknowledge earlier work of Jean Leray in the Leray spectral sequence) is an important tool in algebraic topology. It expresses, in the language of homological algebra, the singular (co)homology of the total space ''X'' of a (Serre) fibration in terms of the (co)homology of the base space ''B'' and the fiber ''F''. The result is due to Jean-Pierre Serre in his doctoral dissertation. Cohomology spectral sequence Let f\colon X\to B be a Serre fibration of topological spaces, and let ''F'' be the (path-connected) fiber. The Serre cohomology spectral sequence is the following: : E_2^ = H^p(B, H^q(F)) \Rightarrow H^(X). Here, at least under standard simplifying conditions, the coefficient group in the E_2-term is the ''q''-th integral cohomology group of ''F'', and the outer group is the singular cohomology of ''B'' with coefficients in that group. Strictly speaking, what is meant is cohomology ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Local Coefficient System
In mathematics, a local system (or a system of local coefficients) on a topological space ''X'' is a tool from algebraic topology which interpolates between cohomology with coefficients in a fixed abelian group ''A'', and general sheaf cohomology in which coefficients vary from point to point. Local coefficient systems were introduced by Norman Steenrod in 1943. The category of perverse sheaves on a manifold is equivalent to the category of local systems on the manifold. Definition Let ''X'' be a topological space. A local system (of abelian groups/modules/...) on ''X'' is a locally constant sheaf (of abelian group In mathematics, an abelian group, also called a commutative group, is a group in which the result of applying the group operation to two group elements does not depend on the order in which they are written. That is, the group operation is commut ...s/Module over a ring, modules...) on ''X''. In other words, a sheaf \mathcal is a local system if every point has an ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Steinberg Group (K-theory)
In algebraic K-theory, a field of mathematics, the Steinberg group \operatorname(A) of a ring A is the universal central extension of the commutator subgroup of the stable general linear group of A . It is named after Robert Steinberg, and it is connected with lower K -groups, notably K_ and K_ . Definition Abstractly, given a ring A , the Steinberg group \operatorname(A) is the universal central extension of the commutator subgroup of the stable general linear group (the commutator subgroup is perfect and so has a universal central extension). Presentation using generators and relations A concrete presentation using generators and relations is as follows. Elementary matrices — i.e. matrices of the form (\lambda) := \mathbf + (\lambda) , where \mathbf is the identity matrix, (\lambda) is the matrix with \lambda in the (p,q) -entry and zeros elsewhere, and p \neq q — satisfy the following relations, called the Steinberg relations: : \begin e_(\lamb ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Universal Central Extension
In mathematical group theory, the Schur multiplier or Schur multiplicator is the second homology group H_2(G, \Z) of a group ''G''. It was introduced by in his work on projective representations. Examples and properties The Schur multiplier \operatorname(G) of a finite group ''G'' is a finite abelian group whose exponent divides the order of ''G''. If a Sylow ''p''-subgroup of ''G'' is cyclic for some ''p'', then the order of \operatorname(G) is not divisible by ''p''. In particular, if all Sylow ''p''-subgroups of ''G'' are cyclic, then \operatorname(G) is trivial. For instance, the Schur multiplier of the nonabelian group of order 6 is the trivial group since every Sylow subgroup is cyclic. The Schur multiplier of the elementary abelian group of order 16 is an elementary abelian group of order 64, showing that the multiplier can be strictly larger than the group itself. The Schur multiplier of the quaternion group is trivial, but the Schur multiplier of dihedral 2-groups ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Central Extension (mathematics)
In mathematics, a group extension is a general means of describing a group in terms of a particular normal subgroup and quotient group. If Q and N are two groups, then G is an extension of Q by N if there is a short exact sequence :1\to N\;\overset\;G\;\overset\;Q \to 1. If G is an extension of Q by N, then G is a group, \iota(N) is a normal subgroup of G and the quotient group G/\iota(N) is isomorphic to the group Q. Group extensions arise in the context of the extension problem, where the groups Q and N are known and the properties of G are to be determined. Note that the phrasing "G is an extension of N by Q" is also used by some. Since any finite group G possesses a maximal normal subgroup N with simple factor group G/N, all finite groups may be constructed as a series of extensions with finite simple groups. This fact was a motivation for completing the classification of finite simple groups. An extension is called a central extension if the subgroup N lies in the cente ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Eilenberg–MacLane Space
In mathematics, specifically algebraic topology, an Eilenberg–MacLane spaceSaunders Mac Lane originally spelt his name "MacLane" (without a space), and co-published the papers establishing the notion of Eilenberg–MacLane spaces under this name. (See e.g. ) In this context it is therefore conventional to write the name without a space. is a topological space with a single nontrivial homotopy group. Let ''G'' be a group and ''n'' a positive integer. A connected topological space ''X'' is called an Eilenberg–MacLane space of type K(G,n), if it has ''n''-th homotopy group \pi_n(X) isomorphic to ''G'' and all other homotopy groups trivial. If n > 1 then ''G'' must be abelian. Such a space exists, is a CW-complex, and is unique up to a weak homotopy equivalence, therefore any such space is often just called K(G,n). The name is derived from Samuel Eilenberg and Saunders Mac Lane, who introduced such spaces in the late 1940s. As such, an Eilenberg–MacLane space is a special k ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Homotopy Pullback
In mathematics, especially in algebraic topology, the homotopy limit and colimitpg 52 are variants of the notions of limit and colimit extended to the homotopy category \text(\textbf). The main idea is this: if we have a diagramF: I \to \textbfconsidered as an object in the homotopy category of diagrams F \in \text(\textbf^I), (where the homotopy equivalence of diagrams is considered pointwise), then the homotopy limit and colimits then correspond to the cone and cocone\begin \underset(F)&: * \to \textbf\\ \underset(F)&: * \to \textbf \endwhich are objects in the homotopy category \text(\textbf^*), where * is the category with one object and one morphism. Note this category is equivalent to the standard homotopy category \text(\textbf) since the latter homotopy functor category has functors which picks out an object in \text and a natural transformation corresponds to a continuous function of topological spaces. Note this construction can be generalized to model categories, which g ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Quillen's Theorem B
In topology, a branch of mathematics, Quillen's Theorem A gives a sufficient condition for the classifying spaces of two categories to be homotopy equivalent. Quillen's Theorem B gives a sufficient condition for a square consisting of classifying spaces of categories to be homotopy Cartesian. The two theorems play central roles in Quillen's Q-construction in algebraic K-theory and are named after Daniel Quillen Daniel Gray "Dan" Quillen (June 22, 1940 – April 30, 2011) was an American mathematician. He is known for being the "prime architect" of higher algebraic ''K''-theory, for which he was awarded the Cole Prize in 1975 and the Fields Medal in 197 .... The precise statements of the theorems are as follows. In general, the homotopy fiber of Bf: BC \to BD is not naturally the classifying space of a category: there is no natural category Ff such that FBf = BFf. Theorem B constructs Ff in a case when f is especially nice. References * * * * Theorems in topology ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Homotopy Fiber
In mathematics, especially homotopy theory, the homotopy fiber (sometimes called the mapping fiber)Joseph J. Rotman, ''An Introduction to Algebraic Topology'' (1988) Springer-Verlag ''(See Chapter 11 for construction.)'' is part of a construction that associates a fibration to an arbitrary continuous function of topological spaces f:A \to B. It acts as a homotopy theoretic kernel of a mapping of topological spaces due to the fact it yields a long exact sequence of homotopy groups\cdots \to \pi_(B) \to \pi_n(\text(f)) \to \pi_n(A) \to \pi_n(B) \to \cdotsMoreover, the homotopy fiber can be found in other contexts, such as homological algebra, where the distinguished triangleC(f)_\bullet 1\to A_\bullet \to B_\bullet \xrightarrowgives a long exact sequence analogous to the long exact sequence of homotopy groups. There is a dual construction called the homotopy cofiber. Construction The homotopy fiber has a simple description for a continuous map f:A \to B. If we replace f by a fibra ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Fibered Category
Fibred categories (or fibered categories) are abstract entities in mathematics used to provide a general framework for descent theory. They formalise the various situations in geometry and algebra in which ''inverse images'' (or ''pull-backs'') of objects such as vector bundles can be defined. As an example, for each topological space there is the category of vector bundles on the space, and for every continuous map from a topological space ''X'' to another topological space ''Y'' is associated the pullback functor taking bundles on ''Y'' to bundles on ''X''. Fibred categories formalise the system consisting of these categories and inverse image functors. Similar setups appear in various guises in mathematics, in particular in algebraic geometry, which is the context in which fibred categories originally appeared. Fibered categories are used to define stacks, which are fibered categories (over a site) with "descent". Fibrations also play an important role in categorical semantics ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |