|
Princeton Lectures In Analysis
The ''Princeton Lectures in Analysis'' is a series of four mathematics textbooks, each covering a different area of mathematical analysis. They were written by Elias M. Stein and Rami Shakarchi and published by Princeton University Press between 2003 and 2011. They are, in order, ''Fourier Analysis: An Introduction''; ''Complex Analysis''; ''Real Analysis: Measure Theory, Integration, and Hilbert Spaces''; and ''Functional Analysis: Introduction to Further Topics in Analysis''. Stein and Shakarchi wrote the books based on a sequence of intensive undergraduate courses Stein began teaching in the spring of 2000 at Princeton University. At the time Stein was a mathematics professor at Princeton and Shakarchi was a graduate student in mathematics. Though Shakarchi graduated in 2002, the collaboration continued until the final volume was published in 2011. The series emphasizes the unity among the branches of analysis and the applicability of analysis to other areas of mathematics. T ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Elias M
Elias is the Greek equivalent of Elijah ( he, אֵלִיָּהוּ ''ʾĒlīyyāhū''; Syriac language, Syriac: ܐܠܝܐ ''Eliyā''; Arabic language, Arabic: الیاس Ilyās, Ilyās/Elyās), a prophet in the Kingdom of Israel (Samaria), Northern Kingdom of Israel in the 9th century BC, mentioned in several holy books. Due to Elias' role in the scriptures and to many later associated traditions, the name is used as a personal name in numerous languages. Variants * Éilias Irish language, Irish * Elia Italian language, Italian, English language, English * Elias Norwegian language, Norwegian * Elías Icelandic language, Icelandic * Éliás Hungarian language, Hungarian * Elías Spanish language, Spanish * Eliáš, Elijáš Czech language, Czech * Elias, Eelis, Eljas Finnish language, Finnish * Elias Danish language, Danish, German language, German, Swedish language, Swedish * Elias Portuguese language, Portuguese * Elias, Iliya () Persian language, Persian * Elias, Elis Swedish l ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
London
London is the capital and largest city of England and the United Kingdom, with a population of just under 9 million. It stands on the River Thames in south-east England at the head of a estuary down to the North Sea, and has been a major settlement for two millennia. The City of London, its ancient core and financial centre, was founded by the Romans as '' Londinium'' and retains its medieval boundaries.See also: Independent city § National capitals The City of Westminster, to the west of the City of London, has for centuries hosted the national government and parliament. Since the 19th century, the name "London" has also referred to the metropolis around this core, historically split between the counties of Middlesex, Essex, Surrey, Kent, and Hertfordshire, which largely comprises Greater London, governed by the Greater London Authority.The Greater London Authority consists of the Mayor of London and the London Assembly. The London Mayor is distinguished fr ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
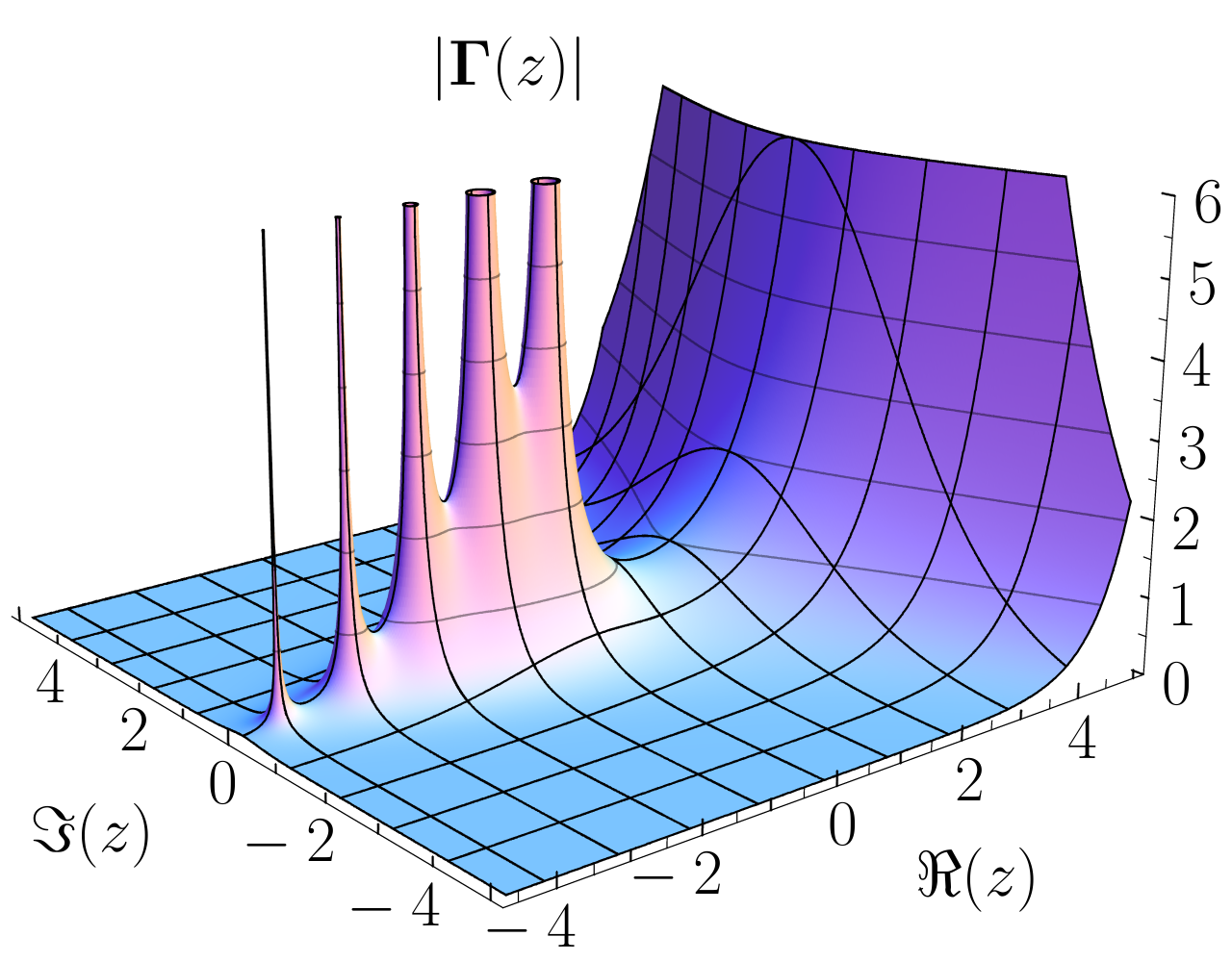
Meromorphic Function
In the mathematical field of complex analysis, a meromorphic function on an open subset ''D'' of the complex plane is a function that is holomorphic on all of ''D'' ''except'' for a set of isolated points, which are pole (complex analysis), poles of the function. The term comes from the Greek ''meros'' ( μέρος), meaning "part". Every meromorphic function on ''D'' can be expressed as the ratio between two holomorphic functions (with the denominator not constant 0) defined on ''D'': any pole must coincide with a zero of the denominator. Heuristic description Intuitively, a meromorphic function is a ratio of two well-behaved (holomorphic) functions. Such a function will still be well-behaved, except possibly at the points where the denominator of the fraction is zero. If the denominator has a zero at ''z'' and the numerator does not, then the value of the function will approach infinity; if both parts have a zero at ''z'', then one must compare the multiplicity of these zero ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Cauchy's Integral Theorem
In mathematics, the Cauchy integral theorem (also known as the Cauchy–Goursat theorem) in complex analysis, named after Augustin-Louis Cauchy (and Édouard Goursat), is an important statement about line integrals for holomorphic functions in the complex plane. Essentially, it says that if f(z) is holomorphic in a simply connected domain Ω, then for any simply closed contour C in Ω, that contour integral is zero. \int_C f(z)\,dz = 0. Statement Fundamental theorem for complex line integrals If is a holomorphic function on an open region , and \gamma is a curve in from z_0 to z_1 then, \int_f'(z) \, dz = f(z_1)-f(z_0). Also, when has a single-valued antiderivative in an open region , then the path integral \int_f'(z) \, dz is path independent for all paths in . Formulation on simply connected regions Let U \subseteq \Complex be a simply connected open set, and let f: U \to \Complex be a holomorphic function. Let \gamma: ,b\to U be a smooth closed curve. Then: \int_ ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Complex Plane
In mathematics, the complex plane is the plane formed by the complex numbers, with a Cartesian coordinate system such that the -axis, called the real axis, is formed by the real numbers, and the -axis, called the imaginary axis, is formed by the imaginary numbers. The complex plane allows a geometric interpretation of complex numbers. Under addition, they add like vectors. The multiplication of two complex numbers can be expressed more easily in polar coordinates—the magnitude or ''modulus'' of the product is the product of the two absolute values, or moduli, and the angle or ''argument'' of the product is the sum of the two angles, or arguments. In particular, multiplication by a complex number of modulus 1 acts as a rotation. The complex plane is sometimes known as the Argand plane or Gauss plane. Notational conventions Complex numbers In complex analysis, the complex numbers are customarily represented by the symbol ''z'', which can be separated into its real (''x'') and ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Riemann Integration
In the branch of mathematics known as real analysis, the Riemann integral, created by Bernhard Riemann, was the first rigorous definition of the integral of a function on an interval. It was presented to the faculty at the University of Göttingen in 1854, but not published in a journal until 1868. For many functions and practical applications, the Riemann integral can be evaluated by the fundamental theorem of calculus or approximated by numerical integration. Overview Let be a non-negative real-valued function on the interval , and let be the region of the plane under the graph of the function and above the interval . See the figure on the top right. This region can be expressed in set-builder notation as S = \left \. We are interested in measuring the area of . Once we have measured it, we will denote the area in the usual way by \int_a^b f(x)\,dx. The basic idea of the Riemann integral is to use very simple approximations for the area of . By taking better and be ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Lebesgue Integration
In mathematics, the integral of a non-negative function of a single variable can be regarded, in the simplest case, as the area between the graph of that function and the -axis. The Lebesgue integral, named after French mathematician Henri Lebesgue, extends the integral to a larger class of functions. It also extends the domains on which these functions can be defined. Long before the 20th century, mathematicians already understood that for non-negative functions with a smooth enough graph—such as continuous functions on closed bounded intervals—the ''area under the curve'' could be defined as the integral, and computed using approximation techniques on the region by polygons. However, as the need to consider more irregular functions arose—e.g., as a result of the limiting processes of mathematical analysis and the mathematical theory of probability—it became clear that more careful approximation techniques were needed to define a suitable integral. Also, one might ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Dirichlet's Theorem On Arithmetic Progressions
In number theory, Dirichlet's theorem, also called the Dirichlet prime number theorem, states that for any two positive coprime integers ''a'' and ''d'', there are infinitely many primes of the form ''a'' + ''nd'', where ''n'' is also a positive integer. In other words, there are infinitely many primes that are congruent to ''a'' modulo ''d''. The numbers of the form ''a'' + ''nd'' form an arithmetic progression :a,\ a+d,\ a+2d,\ a+3d,\ \dots,\ and Dirichlet's theorem states that this sequence contains infinitely many prime numbers. The theorem, named after Peter Gustav Lejeune Dirichlet, extends Euclid's theorem that there are infinitely many prime numbers. Stronger forms of Dirichlet's theorem state that for any such arithmetic progression, the sum of the reciprocals of the prime numbers in the progression diverges and that different such arithmetic progressions with the same modulus have approximately the same proportions of primes. Equivalently, the ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
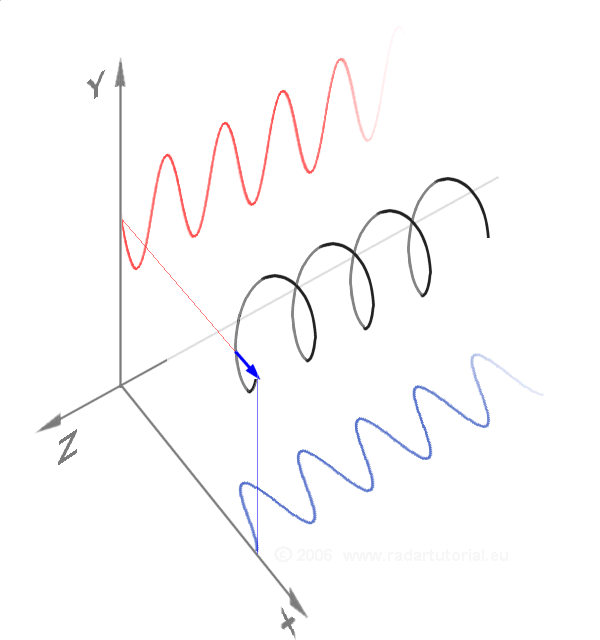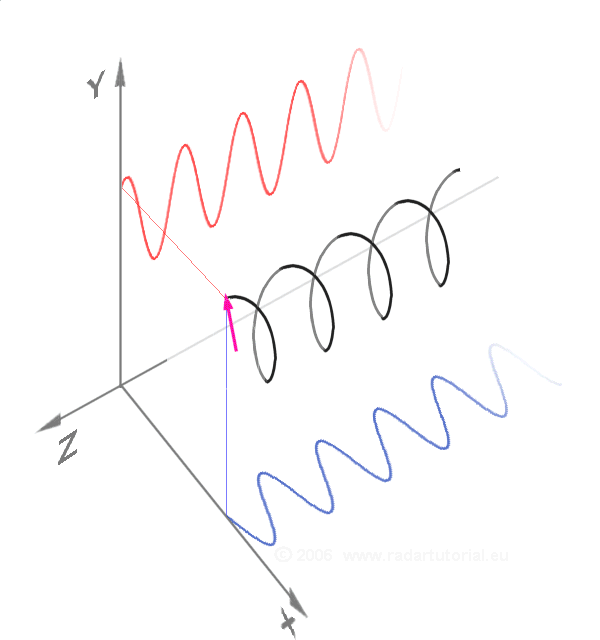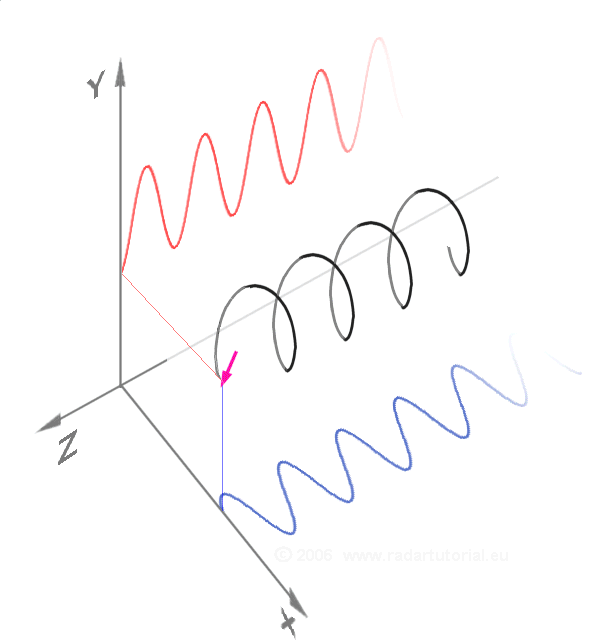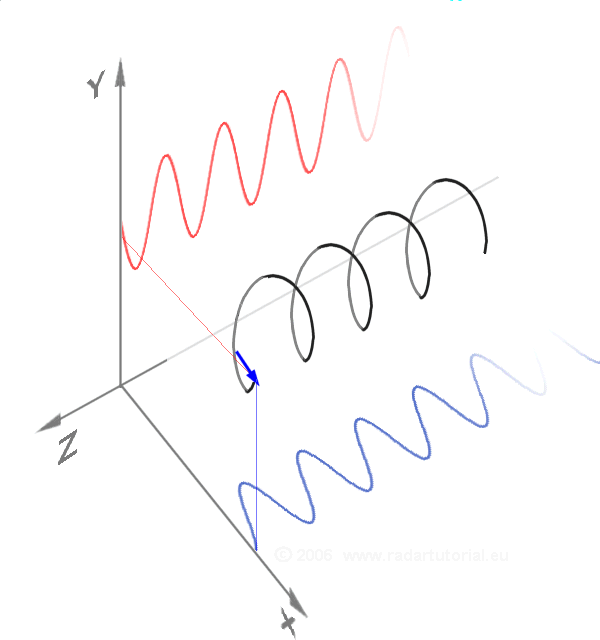
Fourier Transform
A Fourier transform (FT) is a mathematical transform that decomposes functions into frequency components, which are represented by the output of the transform as a function of frequency. Most commonly functions of time or space are transformed, which will output a function depending on temporal frequency or spatial frequency respectively. That process is also called ''analysis''. An example application would be decomposing the waveform of a musical chord into terms of the intensity of its constituent pitches. The term ''Fourier transform'' refers to both the frequency domain representation and the mathematical operation that associates the frequency domain representation to a function of space or time. The Fourier transform of a function is a complex-valued function representing the complex sinusoids that comprise the original function. For each frequency, the magnitude (absolute value) of the complex value represents the amplitude of a constituent complex sinusoid with that ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Continuous Fourier Transform
A Fourier transform (FT) is a mathematical transform that decomposes functions into frequency components, which are represented by the output of the transform as a function of frequency. Most commonly functions of time or space are transformed, which will output a function depending on temporal frequency or spatial frequency respectively. That process is also called ''analysis''. An example application would be decomposing the waveform of a musical chord into terms of the intensity of its constituent pitches. The term ''Fourier transform'' refers to both the frequency domain representation and the mathematical operation that associates the frequency domain representation to a function of space or time. The Fourier transform of a function is a complex-valued function representing the complex sinusoids that comprise the original function. For each frequency, the magnitude (absolute value) of the complex value represents the amplitude of a constituent complex sinusoid with that ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Discrete Fourier Transform
In mathematics, the discrete Fourier transform (DFT) converts a finite sequence of equally-spaced samples of a function into a same-length sequence of equally-spaced samples of the discrete-time Fourier transform (DTFT), which is a complex-valued function of frequency. The interval at which the DTFT is sampled is the reciprocal of the duration of the input sequence. An inverse DFT is a Fourier series, using the DTFT samples as coefficients of complex sinusoids at the corresponding DTFT frequencies. It has the same sample-values as the original input sequence. The DFT is therefore said to be a frequency domain representation of the original input sequence. If the original sequence spans all the non-zero values of a function, its DTFT is continuous (and periodic), and the DFT provides discrete samples of one cycle. If the original sequence is one cycle of a periodic function, the DFT provides all the non-zero values of one DTFT cycle. The DFT is the most important discret ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Number Theory
Number theory (or arithmetic or higher arithmetic in older usage) is a branch of pure mathematics devoted primarily to the study of the integers and arithmetic function, integer-valued functions. German mathematician Carl Friedrich Gauss (1777–1855) said, "Mathematics is the queen of the sciences—and number theory is the queen of mathematics."German original: "Die Mathematik ist die Königin der Wissenschaften, und die Arithmetik ist die Königin der Mathematik." Number theorists study prime numbers as well as the properties of mathematical objects made out of integers (for example, rational numbers) or defined as generalizations of the integers (for example, algebraic integers). Integers can be considered either in themselves or as solutions to equations (Diophantine geometry). Questions in number theory are often best understood through the study of Complex analysis, analytical objects (for example, the Riemann zeta function) that encode properties of the integers, primes ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
