|
Phase Constant
The propagation constant of a sinusoidal electromagnetic wave is a measure of the change undergone by the amplitude and phase of the wave as it propagates in a given direction. The quantity being measured can be the voltage, the current in a circuit, or a field vector such as electric field strength or flux density. The propagation constant itself measures the change per unit length, but it is otherwise dimensionless. In the context of two-port networks and their cascades, propagation constant measures the change undergone by the source quantity as it propagates from one port to the next. The propagation constant's value is expressed logarithmically, almost universally to the base '' e'', rather than the more usual base 10 that is used in telecommunications in other situations. The quantity measured, such as voltage, is expressed as a sinusoidal phasor. The phase of the sinusoid varies with distance which results in the propagation constant being a complex number, the imaginar ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Electromagnetic Wave
In physics, electromagnetic radiation (EMR) consists of waves of the electromagnetic (EM) field, which propagate through space and carry momentum and electromagnetic radiant energy. It includes radio waves, microwaves, infrared, (visible) light, ultraviolet, X-rays, and gamma rays. All of these waves form part of the electromagnetic spectrum. Classically, electromagnetic radiation consists of electromagnetic waves, which are synchronized oscillations of electric and magnetic fields. Depending on the frequency of oscillation, different wavelengths of electromagnetic spectrum are produced. In a vacuum, electromagnetic waves travel at the speed of light, commonly denoted ''c''. In homogeneous, isotropic media, the oscillations of the two fields are perpendicular to each other and perpendicular to the direction of energy and wave propagation, forming a transverse wave. The position of an electromagnetic wave within the electromagnetic spectrum can be characterized ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
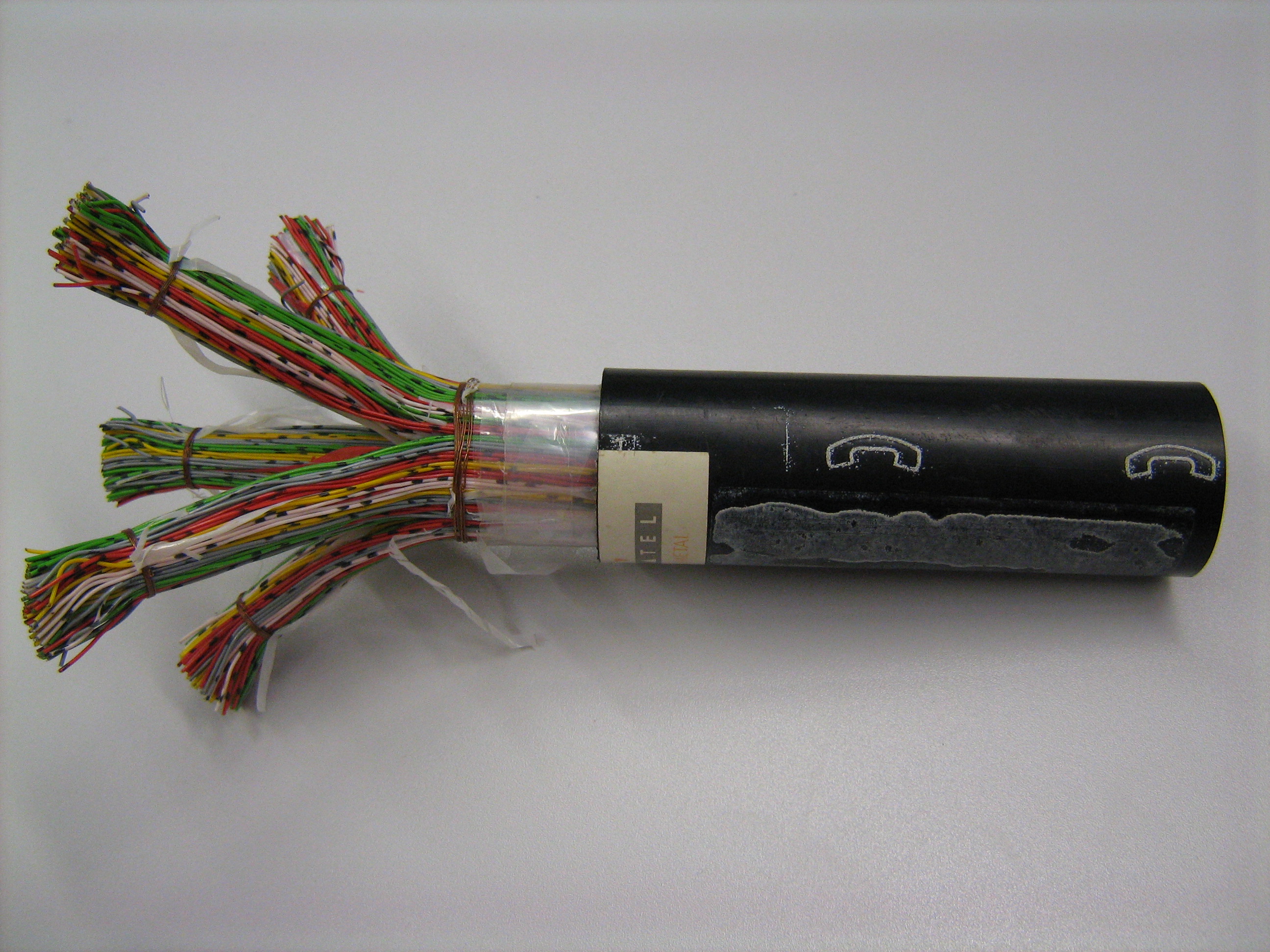
Primary Line Coefficients
The primary line constants are parameters that describe the characteristics of conductive transmission lines, such as pairs of copper wires, in terms of the physical electrical properties of the line. The primary line constants are only relevant to transmission lines and are to be contrasted with the secondary line constants, which can be derived from them, and are more generally applicable. The secondary line constants can be used, for instance, to compare the characteristics of a waveguide to a copper line, whereas the primary constants have no meaning for a waveguide. The constants are conductor resistance and inductance, and insulator capacitance and conductance, which are by convention given the symbols ''R'', ''L'', ''C'', and ''G'' respectively. The constants are enumerated in terms of per unit length. The circuit representation of these elements requires a distributed-element model and consequently calculus must be used to analyse the circuit. The analysis yields a s ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Radian
The radian, denoted by the symbol rad, is the unit of angle in the International System of Units (SI) and is the standard unit of angular measure used in many areas of mathematics. The unit was formerly an SI supplementary unit (before that category was abolished in 1995). The radian is defined in the SI as being a dimensionless unit, with 1 rad = 1. Its symbol is accordingly often omitted, especially in mathematical writing. Definition One radian is defined as the angle subtended from the center of a circle which intercepts an arc equal in length to the radius of the circle. More generally, the magnitude in radians of a subtended angle is equal to the ratio of the arc length to the radius of the circle; that is, \theta = \frac, where is the subtended angle in radians, is arc length, and is radius. A right angle is exactly \frac radians. The rotation angle (360°) corresponding to one complete revolution is the length of the circumference divided by the radius, whi ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Phase Constant
The propagation constant of a sinusoidal electromagnetic wave is a measure of the change undergone by the amplitude and phase of the wave as it propagates in a given direction. The quantity being measured can be the voltage, the current in a circuit, or a field vector such as electric field strength or flux density. The propagation constant itself measures the change per unit length, but it is otherwise dimensionless. In the context of two-port networks and their cascades, propagation constant measures the change undergone by the source quantity as it propagates from one port to the next. The propagation constant's value is expressed logarithmically, almost universally to the base '' e'', rather than the more usual base 10 that is used in telecommunications in other situations. The quantity measured, such as voltage, is expressed as a sinusoidal phasor. The phase of the sinusoid varies with distance which results in the propagation constant being a complex number, the imaginar ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Neper
The neper (symbol: Np) is a logarithmic unit for ratios of measurements of physical field and power quantities, such as gain and loss of electronic signals. The unit's name is derived from the name of John Napier, the inventor of logarithms. As is the case for the decibel and bel, the neper is a unit defined in the international standard ISO 80000. It is not part of the International System of Units (SI), but is accepted for use alongside the SI. Definition Like the decibel, the neper is a unit in a logarithmic scale. While the bel uses the decadic (base-10) logarithm to compute ratios, the neper uses the natural logarithm, based on Euler's number (). The level a ratio of two signal amplitudes or root-power quantities, with the unit neper, is given by : L = \ln\frac\mathrm, where x_1 and x_2 are the signal amplitudes, and is the natural logarithm. The level of a ratio of two power quantities, with the unit neper, is given by : L = \frac \ln\frac\mathrm, where p_1 ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Attenuation Constant
The propagation constant of a sinusoidal electromagnetic wave is a measure of the change undergone by the amplitude and phase of the wave as it propagates in a given direction. The quantity being measured can be the voltage, the current in a circuit, or a field vector such as electric field strength or flux density. The propagation constant itself measures the change per unit length, but it is otherwise dimensionless. In the context of two-port networks and their cascades, propagation constant measures the change undergone by the source quantity as it propagates from one port to the next. The propagation constant's value is expressed logarithmically, almost universally to the base '' e'', rather than the more usual base 10 that is used in telecommunications in other situations. The quantity measured, such as voltage, is expressed as a sinusoidal phasor. The phase of the sinusoid varies with distance which results in the propagation constant being a complex number, the imaginar ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Admittance
In electrical engineering, admittance is a measure of how easily a circuit or device will allow a current to flow. It is defined as the reciprocal of impedance, analogous to how conductance & resistance are defined. The SI unit of admittance is the siemens (symbol S); the older, synonymous unit is mho, and its symbol is ℧ (an upside-down uppercase omega Ω). Oliver Heaviside coined the term ''admittance'' in December 1887. Heaviside used ''Y'' to represent the magnitude of admittance, but it quickly became the conventional symbol for admittance itself through the publications of Charles Proteus Steinmetz. Heaviside probably chose ''Y'' simply because it is next to ''Z'' in the alphabet, the conventional symbol for impedance. Admittance is defined as :Y \equiv \frac \, where :''Y'' is the admittance, measured in siemens :''Z'' is the impedance, measured in ohms Resistance is a measure of the opposition of a circuit to the flow of a steady current, while impedanc ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Electrical Impedance
In electrical engineering, impedance is the opposition to alternating current presented by the combined effect of resistance and reactance in a circuit. Quantitatively, the impedance of a two-terminal circuit element is the ratio of the complex representation of the sinusoidal voltage between its terminals, to the complex representation of the current flowing through it. In general, it depends upon the frequency of the sinusoidal voltage. Impedance extends the concept of resistance to alternating current (AC) circuits, and possesses both magnitude and phase, unlike resistance, which has only magnitude. Impedance can be represented as a complex number, with the same units as resistance, for which the SI unit is the ohm (). Its symbol is usually , and it may be represented by writing its magnitude and phase in the polar form . However, Cartesian complex number representation is often more powerful for circuit analysis purposes. The notion of impedance is useful for ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Euler's Formula
Euler's formula, named after Leonhard Euler, is a mathematical formula in complex analysis that establishes the fundamental relationship between the trigonometric functions and the complex exponential function. Euler's formula states that for any real number : e^ = \cos x + i\sin x, where is the base of the natural logarithm, is the imaginary unit, and and are the trigonometric functions cosine and sine respectively. This complex exponential function is sometimes denoted ("cosine plus i sine"). The formula is still valid if is a complex number, and so some authors refer to the more general complex version as Euler's formula. Euler's formula is ubiquitous in mathematics, physics, and engineering. The physicist Richard Feynman called the equation "our jewel" and "the most remarkable formula in mathematics". When , Euler's formula may be rewritten as , which is known as Euler's identity. History In 1714, the English mathematician Roger Cotes presented a geometr ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Phase Constant
The propagation constant of a sinusoidal electromagnetic wave is a measure of the change undergone by the amplitude and phase of the wave as it propagates in a given direction. The quantity being measured can be the voltage, the current in a circuit, or a field vector such as electric field strength or flux density. The propagation constant itself measures the change per unit length, but it is otherwise dimensionless. In the context of two-port networks and their cascades, propagation constant measures the change undergone by the source quantity as it propagates from one port to the next. The propagation constant's value is expressed logarithmically, almost universally to the base '' e'', rather than the more usual base 10 that is used in telecommunications in other situations. The quantity measured, such as voltage, is expressed as a sinusoidal phasor. The phase of the sinusoid varies with distance which results in the propagation constant being a complex number, the imaginar ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Attenuation Constant
The propagation constant of a sinusoidal electromagnetic wave is a measure of the change undergone by the amplitude and phase of the wave as it propagates in a given direction. The quantity being measured can be the voltage, the current in a circuit, or a field vector such as electric field strength or flux density. The propagation constant itself measures the change per unit length, but it is otherwise dimensionless. In the context of two-port networks and their cascades, propagation constant measures the change undergone by the source quantity as it propagates from one port to the next. The propagation constant's value is expressed logarithmically, almost universally to the base '' e'', rather than the more usual base 10 that is used in telecommunications in other situations. The quantity measured, such as voltage, is expressed as a sinusoidal phasor. The phase of the sinusoid varies with distance which results in the propagation constant being a complex number, the imaginar ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Phasor
In physics and engineering, a phasor (a portmanteau of phase vector) is a complex number representing a sinusoidal function whose amplitude (''A''), angular frequency (''ω''), and initial phase (''θ'') are time-invariant. It is related to a more general concept called analytic representation,Bracewell, Ron. ''The Fourier Transform and Its Applications''. McGraw-Hill, 1965. p269 which decomposes a sinusoid into the product of a complex constant and a factor depending on time and frequency. The complex constant, which depends on amplitude and phase, is known as a phasor, or complex amplitude, and (in older texts) sinor or even complexor. A common situation in electrical networks powered by time varying current is the existence of multiple sinusoids all with the same frequency, but different amplitudes and phases. The only difference in their analytic representations is the complex amplitude (phasor). A linear combination of such functions can be represented as a linear co ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |