|
Modulus And Characteristic Of Convexity
In mathematics, the modulus of convexity and the characteristic of convexity are measures of "how convex" the unit ball in a Banach space is. In some sense, the modulus of convexity has the same relationship to the ''ε''-''δ'' definition of uniform convexity as the modulus of continuity does to the ''ε''-''δ'' definition of continuity. Definitions The modulus of convexity of a Banach space (''X'', , , ·, , ) is the function defined by :\delta (\varepsilon) = \inf \left\, where ''S'' denotes the unit sphere of (''X'', , , , , ). In the definition of ''δ''(''ε''), one can as well take the infimum over all vectors ''x'', ''y'' in ''X'' such that and . The characteristic of convexity of the space (''X'', , , , , ) is the number ''ε''0 defined by :\varepsilon_ = \sup \. These notions are implicit in the general study of uniform convexity by J. A. Clarkson (; this is the same paper containing the statements of Clark ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Mathematics
Mathematics is an area of knowledge that includes the topics of numbers, formulas and related structures, shapes and the spaces in which they are contained, and quantities and their changes. These topics are represented in modern mathematics with the major subdisciplines of number theory, algebra, geometry, and analysis, respectively. There is no general consensus among mathematicians about a common definition for their academic discipline. Most mathematical activity involves the discovery of properties of abstract objects and the use of pure reason to prove them. These objects consist of either abstractions from nature orin modern mathematicsentities that are stipulated to have certain properties, called axioms. A ''proof'' consists of a succession of applications of deductive rules to already established results. These results include previously proved theorems, axioms, andin case of abstraction from naturesome basic properties that are considered true starting points of ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
If And Only If
In logic and related fields such as mathematics and philosophy, "if and only if" (shortened as "iff") is a biconditional logical connective between statements, where either both statements are true or both are false. The connective is biconditional (a statement of material equivalence), and can be likened to the standard material conditional ("only if", equal to "if ... then") combined with its reverse ("if"); hence the name. The result is that the truth of either one of the connected statements requires the truth of the other (i.e. either both statements are true, or both are false), though it is controversial whether the connective thus defined is properly rendered by the English "if and only if"—with its pre-existing meaning. For example, ''P if and only if Q'' means that ''P'' is true whenever ''Q'' is true, and the only case in which ''P'' is true is if ''Q'' is also true, whereas in the case of ''P if Q'', there could be other scenarios where ''P'' is true and ''Q'' is ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Vitali Milman
Vitali Davidovich Milman ( he, ויטלי מילמן; russian: Виталий Давидович Мильман) (born 23 August 1939) is a mathematician specializing in analysis. He is a professor at the Tel Aviv University. In the past he was a President of the Israel Mathematical Union and a member of the “ Aliyah” committee of Tel Aviv University. Work Milman received his Ph.D. at Kharkiv State University in 1965 under the direction of Boris Levin. In a 1971 paper, Milman gave a new proof of Dvoretzky's theorem, stating that every convex body in dimension ''N'' has a section of dimension ''d(N)'', with ''d(N)'' tending to infinity with ''N'', that is arbitrarily close to being isometric to an ellipsoid. Milman's proof gives the optimal bound ''d(N)'' ≥ const log ''N''. In this proof, Milman put forth the concentration of measure phenomenon which has since found numerous applications. Milman made important contributions to the study of Banach spa ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Joram Lindenstrauss
Joram Lindenstrauss ( he, יורם לינדנשטראוס) (October 28, 1936 – April 29, 2012) was an Israeli mathematician working in functional analysis. He was a professor of mathematics at the Einstein Institute of Mathematics. Biography Joram Lindenstrauss was born in Tel Aviv. He was the only child of a pair of lawyers who immigrated to Israel from Berlin. He began to study mathematics at the Hebrew University of Jerusalem in 1954 while serving in the army. He became a full-time student in 1956 and received his master's degree in 1959. In 1962 Lindenstrauss earned his Ph.D. from the Hebrew University (dissertation: ''Extension of Compact Operators'', advisors: Aryeh Dvoretzky, Branko Grünbaum). He worked as a postdoc at Yale University and the University of Washington in Seattle from 1962 - 1965. He was appointed senior lecturer at the Hebrew University in 1965, associate professor on 1967 and full professor in 1969. He became the Leon H. and Ada G. Miller Memorial ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Uniformly Smooth Space
In mathematics, a uniformly smooth space is a normed vector space X satisfying the property that for every \epsilon>0 there exists \delta>0 such that if x,y\in X with \, x\, =1 and \, y\, \leq\delta then :\, x+y\, +\, x-y\, \le 2 + \epsilon\, y\, . The modulus of smoothness of a normed space ''X'' is the function ρ''X'' defined for every by the formula : \rho_X(t) = \sup \Bigl\. The triangle inequality yields that . The normed space ''X'' is uniformly smooth if and only if tends to 0 as ''t'' tends to 0. Properties * Every uniformly smooth Banach space is reflexive. * A Banach space X is uniformly smooth if and only if its continuous dual X^* is uniformly convex (and vice versa, via reflexivity). The moduli of convexity and smoothness are linked by ::\rho_(t) = \sup \, \quad t \ge 0, :and the maximal convex function majorated by the modulus of convexity δ''X'' is given by ::\tilde \delta_X(\varepsilon) = \sup \. :Furthermore, ::\delta_X(\varepsilon / 2) \ ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Israel Journal Of Mathematics
'' Israel Journal of Mathematics'' is a peer-reviewed mathematics journal published by the Hebrew University of Jerusalem (Magnes Press). Founded in 1963, as a continuation of the ''Bulletin of the Research Council of Israel'' (Section F), the journal publishes articles on all areas of mathematics. The journal is indexed by ''Mathematical Reviews'' and Zentralblatt MATH. Its 2009 MCQ was 0.70, and its 2009 impact factor The impact factor (IF) or journal impact factor (JIF) of an academic journal is a scientometric index calculated by Clarivate that reflects the yearly mean number of citations of articles published in the last two years in a given journal, as i ... was 0.754. External links * Mathematics journals Publications established in 1963 English-language journals Bimonthly journals Hebrew University of Jerusalem {{math-journal-stub ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Antipodal Point
In mathematics, antipodal points of a sphere are those diametrically opposite to each other (the specific qualities of such a definition are that a line drawn from the one to the other passes through the center of the sphere so forms a true diameter). This term applies to opposite points on a circle or any n-sphere. An antipodal point is sometimes called an antipode, a back-formation from the Greek loan word ''antipodes'', meaning "opposite (the) feet", as the true word singular is ''antipus''. Theory In mathematics, the concept of ''antipodal points'' is generalized to spheres of any dimension: two points on the sphere are antipodal if they are opposite ''through the centre''; for example, taking the centre as origin, they are points with related vectors v and −v. On a circle, such points are also called diametrically opposite. In other words, each line through the centre intersects the sphere in two points, one for each ray out from the centre, and these two poin ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Strictly Convex Space
In mathematics, a strictly convex space is a normed vector space (''X'', , , , , ) for which the closed unit ball is a strictly convex set. Put another way, a strictly convex space is one for which, given any two distinct points ''x'' and ''y'' on the unit sphere ∂''B'' (i.e. the boundary of the unit ball ''B'' of ''X''), the segment joining ''x'' and ''y'' meets ∂''B'' ''only'' at ''x'' and ''y''. Strict convexity is somewhere between an inner product space (all inner product spaces being strictly convex) and a general normed space in terms of structure. It also guarantees the uniqueness of a best approximation to an element in ''X'' (strictly convex) out of a convex subspace ''Y'', provided that such an approximation exists. If the normed space ''X'' is complete and satisfies the slightly stronger property of being uniformly convex (which implies strict convexity), then it is also reflexive by Milman-Pettis theorem. Properties The following properties are eq ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
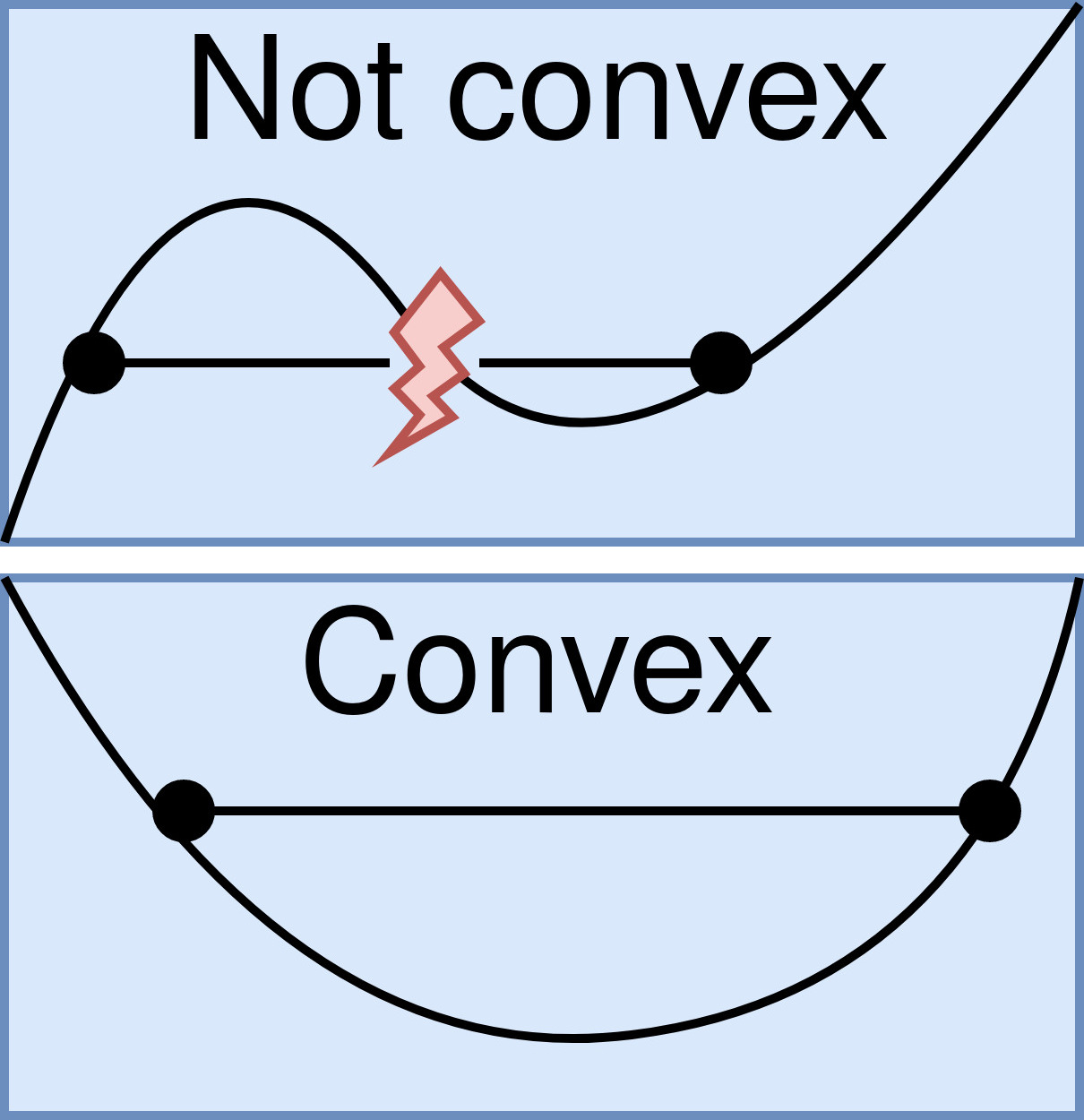
Convex Function
In mathematics, a real-valued function is called convex if the line segment between any two points on the graph of a function, graph of the function lies above the graph between the two points. Equivalently, a function is convex if its epigraph (mathematics), epigraph (the set of points on or above the graph of the function) is a convex set. A twice-differentiable function of a single variable is convex if and only if its second derivative is nonnegative on its entire domain. Well-known examples of convex functions of a single variable include the quadratic function x^2 and the exponential function e^x. In simple terms, a convex function refers to a function whose graph is shaped like a cup \cup, while a concave function's graph is shaped like a cap \cap. Convex functions play an important role in many areas of mathematics. They are especially important in the study of optimization problems where they are distinguished by a number of convenient properties. For instance, a st ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Convex Set
In geometry, a subset of a Euclidean space, or more generally an affine space over the reals, is convex if, given any two points in the subset, the subset contains the whole line segment that joins them. Equivalently, a convex set or a convex region is a subset that intersects every line into a single line segment (possibly empty). For example, a solid cube is a convex set, but anything that is hollow or has an indent, for example, a crescent shape, is not convex. The boundary of a convex set is always a convex curve. The intersection of all the convex sets that contain a given subset of Euclidean space is called the convex hull of . It is the smallest convex set containing . A convex function is a real-valued function defined on an interval with the property that its epigraph (the set of points on or above the graph of the function) is a convex set. Convex minimization is a subfield of optimization that studies the problem of minimizing convex functions over convex se ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Monotonic Function
In mathematics, a monotonic function (or monotone function) is a function between ordered sets that preserves or reverses the given order. This concept first arose in calculus, and was later generalized to the more abstract setting of order theory. In calculus and analysis In calculus, a function f defined on a subset of the real numbers with real values is called ''monotonic'' if and only if it is either entirely non-increasing, or entirely non-decreasing. That is, as per Fig. 1, a function that increases monotonically does not exclusively have to increase, it simply must not decrease. A function is called ''monotonically increasing'' (also ''increasing'' or ''non-decreasing'') if for all x and y such that x \leq y one has f\!\left(x\right) \leq f\!\left(y\right), so f preserves the order (see Figure 1). Likewise, a function is called ''monotonically decreasing'' (also ''decreasing'' or ''non-increasing'') if, whenever x \leq y, then f\!\left(x\right) \geq f\!\left(y\ri ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Clarkson's Inequalities
In mathematics, Clarkson's inequalities, named after James A. Clarkson, are results in the theory of ''L''''p'' spaces. They give bounds for the ''L''''p''-norms of the sum and difference of two measurable functions in ''L''''p'' in terms of the ''L''''p''-norms of those functions individually. Statement of the inequalities Let (''X'', Σ, ''μ'') be a measure space; let ''f'', ''g'' : ''X'' → R be measurable functions in ''L''''p''. Then, for 2 ≤ ''p'' < +∞, : For 1 < ''p'' < 2, : where : i.e., ''q'' = ''p'' ⁄ (''p'' − 1). The case ''p'' ≥ 2 is somewhat e ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |