|
Muoctahedron
In geometry, a regular skew apeirohedron is an infinite regular skew polyhedron, with either skew regular faces or skew regular vertex figures. History According to Coxeter, in 1926 John Flinders Petrie generalized the concept of regular skew polygons (nonplanar polygons) to finite regular skew polyhedra in 4-dimensions, and infinite regular skew apeirohedra in 3-dimensions (described here). Coxeter identified 3 forms, with planar faces and skew vertex figures, two are complements of each other. They are all named with a modified Schläfli symbol , where there are ''l''-gonal faces, ''m'' faces around each vertex, with ''holes'' identified as ''n''-gonal missing faces. Coxeter offered a modified Schläfli symbol for these figures, with implying the vertex figure, ''m'' l-gons around a vertex, and ''n''-gonal holes. Their vertex figures are skew polygons, zig-zagging between two planes. The regular skew polyhedra, represented by , follow this equation: * 2 sin(/''l'') · ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Muoctahedron
In geometry, a regular skew apeirohedron is an infinite regular skew polyhedron, with either skew regular faces or skew regular vertex figures. History According to Coxeter, in 1926 John Flinders Petrie generalized the concept of regular skew polygons (nonplanar polygons) to finite regular skew polyhedra in 4-dimensions, and infinite regular skew apeirohedra in 3-dimensions (described here). Coxeter identified 3 forms, with planar faces and skew vertex figures, two are complements of each other. They are all named with a modified Schläfli symbol , where there are ''l''-gonal faces, ''m'' faces around each vertex, with ''holes'' identified as ''n''-gonal missing faces. Coxeter offered a modified Schläfli symbol for these figures, with implying the vertex figure, ''m'' l-gons around a vertex, and ''n''-gonal holes. Their vertex figures are skew polygons, zig-zagging between two planes. The regular skew polyhedra, represented by , follow this equation: * 2 sin(/''l'') · ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Mucube
In geometry, a regular skew apeirohedron is an infinite regular skew polyhedron, with either skew regular faces or skew regular vertex figures. History According to Coxeter, in 1926 John Flinders Petrie generalized the concept of regular skew polygons (nonplanar polygons) to finite regular skew polyhedra in 4-dimensions, and infinite regular skew apeirohedra in 3-dimensions (described here). Coxeter identified 3 forms, with planar faces and skew vertex figures, two are complements of each other. They are all named with a modified Schläfli symbol , where there are ''l''-gonal faces, ''m'' faces around each vertex, with ''holes'' identified as ''n''-gonal missing faces. Coxeter offered a modified Schläfli symbol for these figures, with implying the vertex figure, ''m'' l-gons around a vertex, and ''n''-gonal holes. Their vertex figures are skew polygons, zig-zagging between two planes. The regular skew polyhedra, represented by , follow this equation: * 2 sin(/''l'') · ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
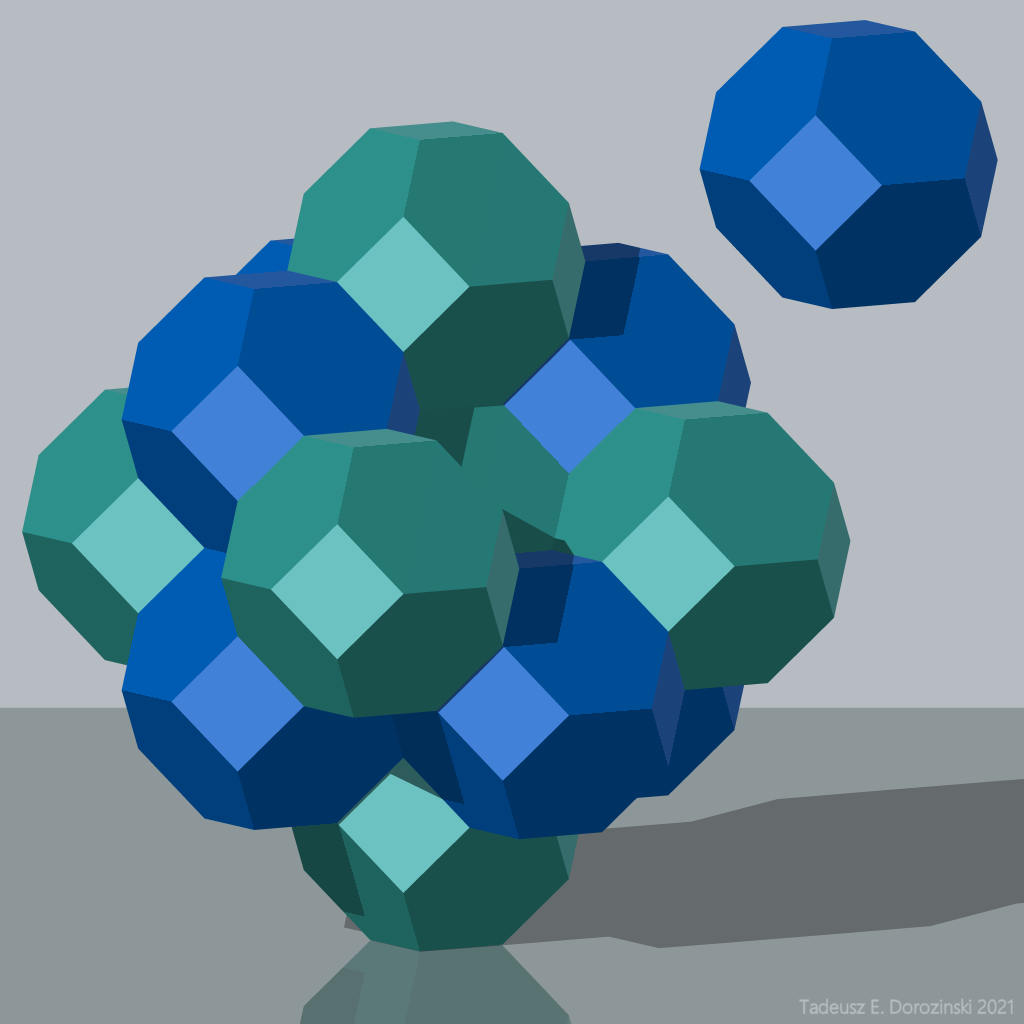
Bitruncated Cubic Honeycomb
The bitruncated cubic honeycomb is a space-filling tessellation (or honeycomb) in Euclidean 3-space made up of truncated octahedra (or, equivalently, bitruncated cubes). It has 4 truncated octahedra around each vertex. Being composed entirely of truncated octahedra, it is cell-transitive. It is also edge-transitive, with 2 hexagons and one square on each edge, and vertex-transitive. It is one of 28 uniform honeycombs. John Horton Conway calls this honeycomb a truncated octahedrille in his Architectonic and catoptric tessellation list, with its dual called an ''oblate tetrahedrille'', also called a disphenoid tetrahedral honeycomb. Although a regular tetrahedron can not tessellate space alone, this dual has identical disphenoid tetrahedron cells with isosceles triangle faces. Geometry It can be realized as the Voronoi tessellation of the body-centred cubic lattice. Lord Kelvin conjectured that a variant of the ''bitruncated cubic honeycomb'' (with curved faces and edges, but the ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Geometry
Geometry (; ) is, with arithmetic, one of the oldest branches of mathematics. It is concerned with properties of space such as the distance, shape, size, and relative position of figures. A mathematician who works in the field of geometry is called a ''geometer''. Until the 19th century, geometry was almost exclusively devoted to Euclidean geometry, which includes the notions of point, line, plane, distance, angle, surface, and curve, as fundamental concepts. During the 19th century several discoveries enlarged dramatically the scope of geometry. One of the oldest such discoveries is Carl Friedrich Gauss' ("remarkable theorem") that asserts roughly that the Gaussian curvature of a surface is independent from any specific embedding in a Euclidean space. This implies that surfaces can be studied ''intrinsically'', that is, as stand-alone spaces, and has been expanded into the theory of manifolds and Riemannian geometry. Later in the 19th century, it appeared that geometries ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
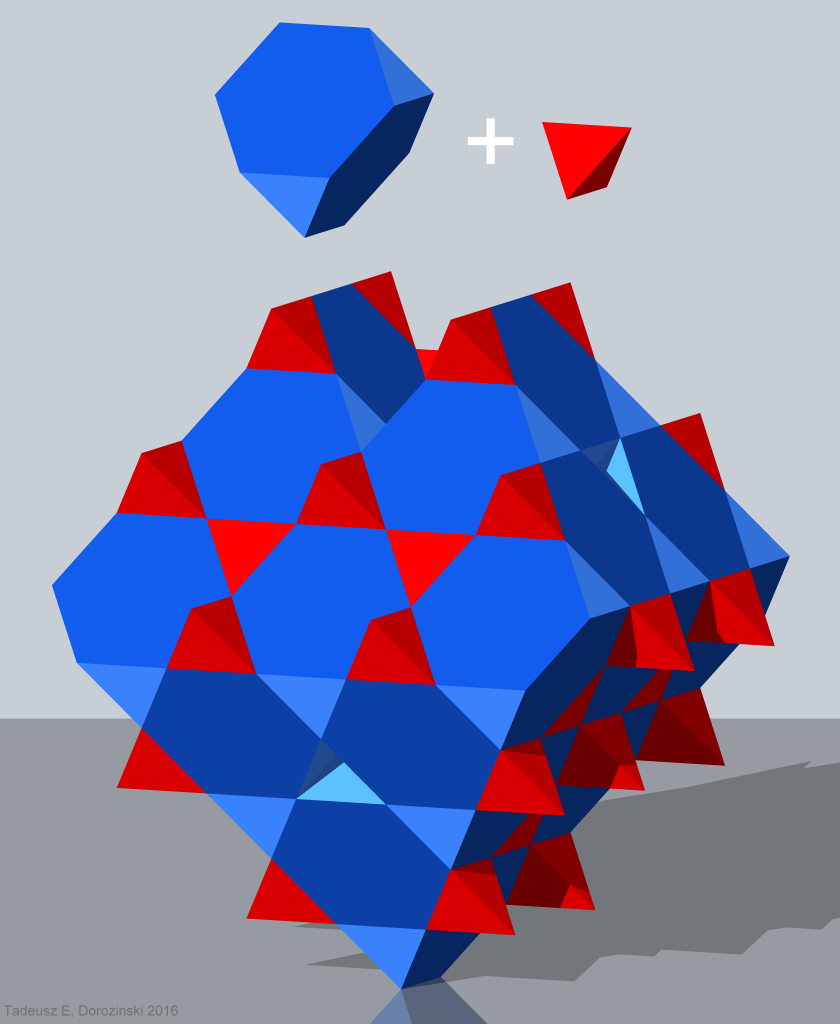
Quarter Cubic Honeycomb
The quarter cubic honeycomb, quarter cubic cellulation or bitruncated alternated cubic honeycomb is a space-filling tessellation (or honeycomb) in Euclidean 3-space. It is composed of tetrahedra and truncated tetrahedra in a ratio of 1:1. It is called "quarter-cubic" because its symmetry unit – the minimal block from which the pattern is developed by reflections – is four times that of the cubic honeycomb. It is vertex-transitive with 6 truncated tetrahedra and 2 tetrahedra around each vertex. It is one of the 28 convex uniform honeycombs. The faces of this honeycomb's cells form four families of parallel planes, each with a 3.6.3.6 tiling. Its vertex figure is an isosceles antiprism: two equilateral triangles joined by six isosceles triangles. John Horton Conway calls this honeycomb a truncated tetrahedrille, and its dual oblate cubille. The vertices and edges represent a Kagome lattice in three dimensions, which is the pyrochlore lattice. Construction The quarter ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
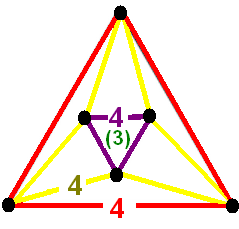
Regular Polygon 6 Annotated
The term regular can mean normal or in accordance with rules. It may refer to: People * Moses Regular (born 1971), America football player Arts, entertainment, and media Music * "Regular" (Badfinger song) * Regular tunings of stringed instruments, tunings with equal intervals between the paired notes of successive open strings Other uses in arts, entertainment, and media * Regular character, a main character who appears more frequently and/or prominently than a recurring character * Regular division of the plane, a series of drawings by the Dutch artist M. C. Escher which began in 1936 * ''Regular Show'', an animated television sitcom * '' The Regular Guys'', a radio morning show Language * Regular inflection, the formation of derived forms such as plurals in ways that are typical for the language ** Regular verb * Regular script, the newest of the Chinese script styles Mathematics There are an extremely large number of unrelated notions of "regularity" in mathematics. ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Runcinated Cubic Honeycomb
The cubic honeycomb or cubic cellulation is the only proper regular space-filling tessellation (or honeycomb) in Euclidean 3-space made up of cubic cells. It has 4 cubes around every edge, and 8 cubes around each vertex. Its vertex figure is a regular octahedron. It is a self-dual tessellation with Schläfli symbol . John Horton Conway called this honeycomb a cubille. Related honeycombs It is part of a multidimensional family of hypercube honeycombs, with Schläfli symbols of the form , starting with the square tiling, in the plane. It is one of 28 uniform honeycombs using convex uniform polyhedral cells. Isometries of simple cubic lattices Simple cubic lattices can be distorted into lower symmetries, represented by lower crystal systems: Uniform colorings There is a large number of uniform colorings, derived from different symmetries. These include: Projections The ''cubic honeycomb'' can be orthogonally projected into the euclidean plane with various symmetr ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Runcinated Cubic Honeycomb Verf
In geometry, runcination is an operation that cuts a regular polytope (or honeycomb) simultaneously along the faces, edges, and vertices, creating new facets in place of the original face, edge, and vertex centers. It is a higher order truncation operation, following cantellation, and truncation. It is represented by an extended Schläfli symbol t0,3. This operation only exists for 4-polytopes or higher. This operation is dual-symmetric for regular uniform 4-polytopes and 3-space convex uniform honeycombs. For a regular 4-polytope, the original cells remain, but become separated. The gaps at the separated faces become p-gonal prisms. The gaps between the separated edges become r-gonal prisms. The gaps between the separated vertices become cells. The vertex figure for a regular 4-polytope is an ''q''-gonal antiprism (called an ''antipodium'' if ''p'' and ''r'' are different). For regular 4-polytopes/honeycombs, this operation is also called expansion by Alicia Boole S ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Regular Polygon 4 Annotated
The term regular can mean normal or in accordance with rules. It may refer to: People * Moses Regular (born 1971), America football player Arts, entertainment, and media Music * "Regular" (Badfinger song) * Regular tunings of stringed instruments, tunings with equal intervals between the paired notes of successive open strings Other uses in arts, entertainment, and media * Regular character, a main character who appears more frequently and/or prominently than a recurring character * Regular division of the plane, a series of drawings by the Dutch artist M. C. Escher which began in 1936 * ''Regular Show'', an animated television sitcom * '' The Regular Guys'', a radio morning show Language * Regular inflection, the formation of derived forms such as plurals in ways that are typical for the language ** Regular verb * Regular script, the newest of the Chinese script styles Mathematics There are an extremely large number of unrelated notions of "regularity" in mathematics. ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Coxeter Group
In mathematics, a Coxeter group, named after H. S. M. Coxeter, is an abstract group that admits a formal description in terms of reflections (or kaleidoscopic mirrors). Indeed, the finite Coxeter groups are precisely the finite Euclidean reflection groups; the symmetry groups of regular polyhedra are an example. However, not all Coxeter groups are finite, and not all can be described in terms of symmetries and Euclidean reflections. Coxeter groups were introduced in 1934 as abstractions of reflection groups , and finite Coxeter groups were classified in 1935 . Coxeter groups find applications in many areas of mathematics. Examples of finite Coxeter groups include the symmetry groups of regular polytopes, and the Weyl groups of simple Lie algebras. Examples of infinite Coxeter groups include the triangle groups corresponding to regular tessellations of the Euclidean plane and the hyperbolic plane, and the Weyl groups of infinite-dimensional Kac–Moody algebras. Standard ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |