|
List Of Things Named After Jacques Hadamard
These are things named after Jacques Hadamard (1865–1963), a French mathematician. (For references, see the respective articles.) Theorems, lemmas, and conjectures Many of these results are sometimes called "Hadamard's theorem". * Cartan–Hadamard theorem, a statement in Riemannian geometry concerning the structure of complete Riemannian manifolds of non-positive sectional curvature ** Cartan–Hadamard conjecture, an open problem in Riemannian geometry and geometric measure theory concerning the generalization of the classical isoperimetric inequality to spaces of nonpositive sectional curvature * Cauchy–Hadamard theorem, a statement in complex analysis describing the radius of convergence of a power series * Hadamard's inequality, a bound on the determinant of a matrix whose entries are complex numbers in terms of the lengths of its column vectors * Hermite–Hadamard inequality, a bound on the mean value of a convex function in an interval in terms of the value it takes ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Jacques Hadamard
Jacques Salomon Hadamard (; 8 December 1865 – 17 October 1963) was a French mathematician who made major contributions in number theory, complex analysis, differential geometry and partial differential equations. Biography The son of a teacher, Amédée Hadamard, of Jewish descent, and Claire Marie Jeanne Picard, Hadamard was born in Versailles, France and attended the Lycée Charlemagne and Lycée Louis-le-Grand, where his father taught. In 1884 Hadamard entered the École Normale Supérieure, having placed first in the entrance examinations both there and at the École Polytechnique. His teachers included Tannery, Hermite, Darboux, Appell, Goursat and Picard. He obtained his doctorate in 1892 and in the same year was awarded the for his essay on the Riemann zeta function. In 1892 Hadamard married Louise-Anna Trénel, also of Jewish descent, with whom he had three sons and two daughters. The following year he took up a lectureship in the University of Bordeaux, where he ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
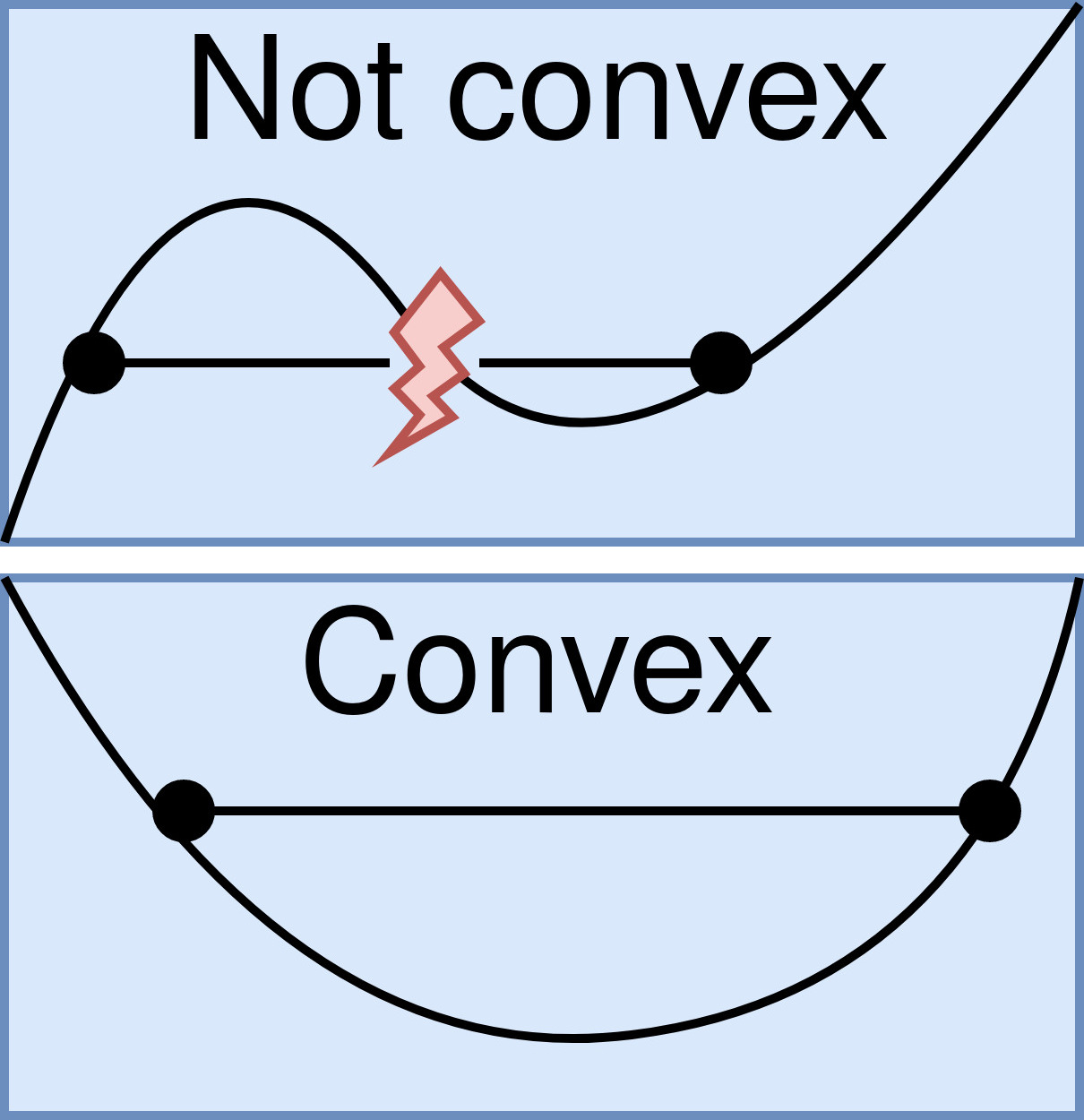
Convex Function
In mathematics, a real-valued function is called convex if the line segment between any two points on the graph of a function, graph of the function lies above the graph between the two points. Equivalently, a function is convex if its epigraph (mathematics), epigraph (the set of points on or above the graph of the function) is a convex set. A twice-differentiable function of a single variable is convex if and only if its second derivative is nonnegative on its entire domain. Well-known examples of convex functions of a single variable include the quadratic function x^2 and the exponential function e^x. In simple terms, a convex function refers to a function whose graph is shaped like a cup \cup, while a concave function's graph is shaped like a cap \cap. Convex functions play an important role in many areas of mathematics. They are especially important in the study of optimization problems where they are distinguished by a number of convenient properties. For instance, a st ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Hadamard Space
In geometry, an Hadamard space, named after Jacques Hadamard, is a non-linear generalization of a Hilbert space. In the literature they are also equivalently defined as complete CAT(0) spaces. A Hadamard space is defined to be a nonempty complete metric space such that, given any points x and y, there exists a point m such that for every point z, d(z, m)^2 + \leq . The point m is then the midpoint of x and y: d(x, m) = d(y, m) = d(x, y)/2. In a Hilbert space, the above inequality is equality (with m = (x+y)/2), and in general an Hadamard space is said to be if the above inequality is equality. A flat Hadamard space is isomorphic to a closed convex subset of a Hilbert space. In particular, a normed space is an Hadamard space if and only if it is a Hilbert space. The geometry of Hadamard spaces resembles that of Hilbert spaces, making it a natural setting for the study of rigidity theorems. In a Hadamard space, any two points can be joined by a unique geodesic between them; in p ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Hadamard Matrix
In mathematics, a Hadamard matrix, named after the French mathematician Jacques Hadamard, is a square matrix whose entries are either +1 or −1 and whose rows are mutually orthogonal. In geometric terms, this means that each pair of rows in a Hadamard matrix represents two perpendicular vectors, while in combinatorial terms, it means that each pair of rows has matching entries in exactly half of their columns and mismatched entries in the remaining columns. It is a consequence of this definition that the corresponding properties hold for columns as well as rows. The ''n''-dimensional parallelotope spanned by the rows of an ''n''×''n'' Hadamard matrix has the maximum possible ''n''-dimensional volume among parallelotopes spanned by vectors whose entries are bounded in absolute value by 1. Equivalently, a Hadamard matrix has maximal determinant among matrices with entries of absolute value less than or equal to 1 and so is an extremal solution of Hadamard's maximal determina ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Hadamard Manifold
In mathematics, a Hadamard manifold, named after Jacques Hadamard — more often called a Cartan–Hadamard manifold, after Élie Cartan — is a Riemannian manifold (M, g) that is complete and simply connected and has everywhere non-positive sectional curvature. By Cartan–Hadamard theorem all Cartan–Hadamard manifolds are diffeomorphic to the Euclidean space \R^n. Furthermore it follows from the Hopf–Rinow theorem that every pairs of points in a Cartan–Hadamard manifold may be connected by a unique geodesic segment. Thus Cartan–Hadamard manifolds are some of the closest relatives of \R^n. Examples The Euclidean space \R^n with its usual metric is a Cartan-Hadamard manifold with constant sectional curvature equal to 0. Standard n-dimensional hyperbolic space \mathbb^n is a Cartan-Hadamard manifold with constant sectional curvature equal to -1. Properties In Cartan-Hadamard manifolds, the map \exp_p : \operatornameM_p \to M is a covering map A covering of a topologic ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Hadamard's Dynamical System
In physics and mathematics, the Hadamard dynamical system (also called Hadamard's billiard or the Hadamard–Gutzwiller model) is a chaotic dynamical system, a type of dynamical billiards. Introduced by Jacques Hadamard in 1898, and studied by Martin Gutzwiller in the 1980s, it is the first dynamical system to be proven chaotic. The system considers the motion of a free (frictionless) particle on the Bolza surface, i.e, a two-dimensional surface of genus two (a donut with two holes) and constant negative curvature; this is a compact Riemann surface. Hadamard was able to show that every particle trajectory moves away from every other: that all trajectories have a positive Lyapunov exponent. Frank Steiner argues that Hadamard's study should be considered to be the first-ever examination of a chaotic dynamical system, and that Hadamard should be considered the first discoverer of chaos. He points out that the study was widely disseminated, and considers the impact of the ideas on the ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Hadamard Code
The Hadamard code is an error-correcting code named after Jacques Hadamard that is used for error detection and correction when transmitting messages over very noisy or unreliable channels. In 1971, the code was used to transmit photos of Mars back to Earth from the NASA space probe Mariner 9. Because of its unique mathematical properties, the Hadamard code is not only used by engineers, but also intensely studied in coding theory, mathematics, and theoretical computer science. The Hadamard code is also known under the names Walsh code, Walsh family, and Walsh–Hadamard code in recognition of the American mathematician Joseph Leonard Walsh. The Hadamard code is an example of a linear code of length 2^m over a binary alphabet. Unfortunately, this term is somewhat ambiguous as some references assume a message length k = m while others assume a message length of k = m+1. In this article, the first case is called the Hadamard code while the second is called the augmented Hadamar ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Riemann Zeta Function
The Riemann zeta function or Euler–Riemann zeta function, denoted by the Greek letter (zeta), is a mathematical function of a complex variable defined as \zeta(s) = \sum_^\infty \frac = \frac + \frac + \frac + \cdots for \operatorname(s) > 1 and its analytic continuation elsewhere. The Riemann zeta function plays a pivotal role in analytic number theory, and has applications in physics, probability theory, and applied statistics. Leonhard Euler first introduced and studied the function over the reals in the first half of the eighteenth century. Bernhard Riemann's 1859 article "On the Number of Primes Less Than a Given Magnitude" extended the Euler definition to a complex variable, proved its meromorphic continuation and functional equation, and established a relation between its zeros and the distribution of prime numbers. This paper also contained the Riemann hypothesis, a conjecture about the distribution of complex zeros of the Riemann zeta function that is consid ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Hadamard Product (matrices)
In mathematics, the Hadamard product (also known as the element-wise product, entrywise product or Schur product) is a binary operation that takes two matrices of the same dimensions and produces another matrix of the same dimension as the operands, where each element is the product of elements of the original two matrices. It is to be distinguished from the more common matrix product. It is attributed to, and named after, either French mathematician Jacques Hadamard or German Russian mathematician Issai Schur. The Hadamard product is associative and distributive. Unlike the matrix product, it is also commutative. Definition For two matrices and of the same dimension , the Hadamard product A \circ B (or A \odot B) is a matrix of the same dimension as the operands, with elements given by :(A \circ B)_ = (A \odot B)_ = (A)_ (B)_. For matrices of different dimensions ( and , where or ), the Hadamard product is undefined. Example For example, the Hadamard product for a 3 ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Hadamard Three-lines Theorem
In complex analysis, a branch of mathematics, the Hadamard three-lines theorem is a result about the behaviour of holomorphic functions defined in regions bounded by parallel lines in the complex plane. The theorem is named after the French mathematician Jacques Hadamard. Statement Define F(z) by : F(z)=f(z) M(a)^M(b)^ where , F(z), \leq 1 on the edges of the strip. The result follows once it is shown that the inequality also holds in the interior of the strip. After an affine transformation in the coordinate z, it can be assumed that a = 0 and b = 1. The function : F_n(z) = F(z) e^e^ tends to 0 as , z, tends to infinity and satisfies , F_n, \leq 1 on the boundary of the strip. The maximum modulus principle can therefore be applied to F_n in the strip. So , F_n(z), \leq 1. Because F_n(z) tends to F(z) as n tends to infinity, it follows that , F(z), \leq 1. ∎ Applications The three-line theorem can be used to prove the Hadamard three-circle theorem for a boun ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Hadamard Three-circle Theorem
In complex analysis, a branch of mathematics, the Hadamard three-circle theorem is a result about the behavior of holomorphic functions. Let f(z) be a holomorphic function on the annulus :r_1\leq\left, z\ \leq r_3. Let M(r) be the maximum of , f(z), on the circle , z, =r. Then, \log M(r) is a convex function of the logarithm \log (r). Moreover, if f(z) is not of the form cz^\lambda for some constants \lambda and c, then \log M(r) is strictly convex as a function of \log (r). The conclusion of the theorem can be restated as :\log\left(\frac\right)\log M(r_2)\leq \log\left(\frac\right)\log M(r_1) +\log\left(\frac\right)\log M(r_3) for any three concentric circles of radii r_1 History A statement and proof for the theorem was given by in 1912, but he attributes it to no one in particular, stating i ...[...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Analytic Continuation
In complex analysis, a branch of mathematics, analytic continuation is a technique to extend the domain of definition of a given analytic function. Analytic continuation often succeeds in defining further values of a function, for example in a new region where an infinite series representation in terms of which it is initially defined becomes divergent. The step-wise continuation technique may, however, come up against difficulties. These may have an essentially topological nature, leading to inconsistencies (defining more than one value). They may alternatively have to do with the presence of singularities. The case of several complex variables is rather different, since singularities then need not be isolated points, and its investigation was a major reason for the development of sheaf cohomology. Initial discussion Suppose ''f'' is an analytic function defined on a non-empty open subset ''U'' of the complex plane If ''V'' is a larger open subset of containing ''U'', and ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |