|
List Of Integrals Of Trigonometric Functions
The following is a list of integrals (antiderivative functions) of trigonometric functions. For antiderivatives involving both exponential and trigonometric functions, see List of integrals of exponential functions. For a complete list of antiderivative functions, see Lists of integrals. For the special antiderivatives involving trigonometric functions, see Trigonometric integral. Generally, if the function \sin x is any trigonometric function, and \cos x is its derivative, : \int a\cos nx\,dx = \frac\sin nx+C In all formulas the constant ''a'' is assumed to be nonzero, and ''C'' denotes the constant of integration. Integrands involving only sine : \int\sin ax\,dx = -\frac\cos ax+C : \int\sin^2 \,dx = \frac - \frac \sin 2ax +C= \frac - \frac \sin ax\cos ax +C : \int\sin^3 \,dx = \frac - \frac +C : \int x\sin^2 \,dx = \frac - \frac \sin 2ax - \frac \cos 2ax +C : \int x^2\sin^2 \,dx = \frac - \left( \frac - \frac \right) \sin 2ax - \frac \cos 2ax +C :\int x\sin ax\,dx = \fra ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Integral
In mathematics Mathematics is an area of knowledge that includes the topics of numbers, formulas and related structures, shapes and the spaces in which they are contained, and quantities and their changes. These topics are represented in modern mathematics ..., an integral assigns numbers to functions in a way that describes Displacement (geometry), displacement, area, volume, and other concepts that arise by combining infinitesimal data. The process of finding integrals is called integration. Along with Derivative, differentiation, integration is a fundamental, essential operation of calculus,Integral calculus is a very well established mathematical discipline for which there are many sources. See and , for example. and serves as a tool to solve problems in mathematics and physics involving the area of an arbitrary shape, the length of a curve, and the volume of a solid, among others. The integrals enumerated here are those termed definite integrals, which can be int ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Tangent (trigonometric Function)
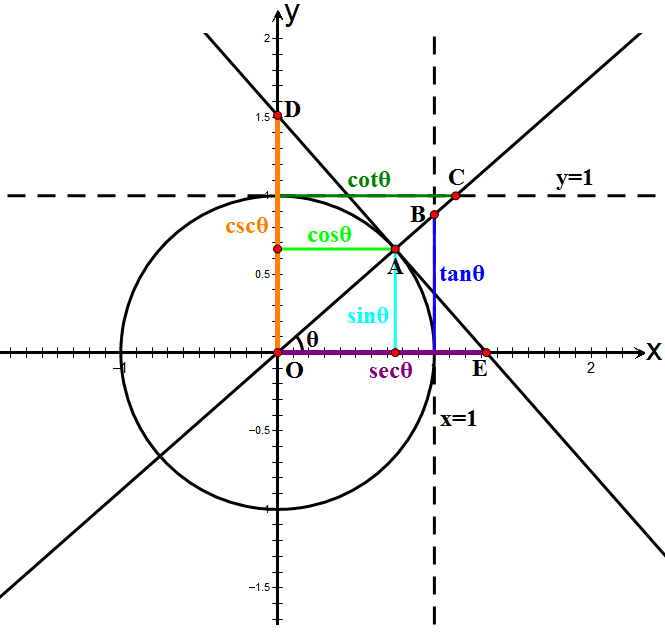
In mathematics, the trigonometric functions (also called circular functions, angle functions or goniometric functions) are real functions which relate an angle of a right-angled triangle to ratios of two side lengths. They are widely used in all sciences that are related to geometry, such as navigation, solid mechanics, celestial mechanics, geodesy, and many others. They are among the simplest periodic functions, and as such are also widely used for studying periodic phenomena through Fourier analysis. The trigonometric functions most widely used in modern mathematics are the sine, the cosine, and the tangent. Their multiplicative inverse, reciprocals are respectively the cosecant, the secant, and the cotangent, which are less used. Each of these six trigonometric functions has a corresponding Inverse trigonometric functions, inverse function, and an analog among the hyperbolic functions. The oldest definitions of trigonometric functions, related to right-angle triangles, define ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Tangent
In geometry, the tangent line (or simply tangent) to a plane curve at a given point is the straight line that "just touches" the curve at that point. Leibniz defined it as the line through a pair of infinitely close points on the curve. More precisely, a straight line is said to be a tangent of a curve at a point if the line passes through the point on the curve and has slope , where ''f'' is the derivative of ''f''. A similar definition applies to space curves and curves in ''n''-dimensional Euclidean space. As it passes through the point where the tangent line and the curve meet, called the point of tangency, the tangent line is "going in the same direction" as the curve, and is thus the best straight-line approximation to the curve at that point. The tangent line to a point on a differentiable curve can also be thought of as a '' tangent line approximation'', the graph of the affine function that best approximates the original function at the given point. Similarly, t ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Bioche's Rules
Bioche's rules, formulated by the French mathematician (1859–1949), are rules to aid in the computation of certain indefinite integrals in which the integrand contains sines and cosines. In the following, f(t) is a rational expression in \sin t and \cos t. In order to calculate \int f(t)\,dt, consider the integrand \omega(t)=f(t)\,dt. We consider the behavior of this entire integrand, including the dt, under translation and reflections of the ''t'' axis. The translations and reflections are ones that correspond to the symmetries and periodicities of the basic trigonometric functions. Bioche's rules state that: # If \omega(-t)=\omega(t), a good change of variables is u=\cos t. # If \omega(\pi-t)=\omega(t), a good change of variables is u=\sin t. # If \omega(\pi+t)=\omega(t), a good change of variables is u=\tan t. # If two of the preceding relations both hold, a good change of variables is u=\cos 2t. # In all other cases, use u=\tan(t/2). Because rules 1 and 2 involve flipp ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Cotangent
In mathematics, the trigonometric functions (also called circular functions, angle functions or goniometric functions) are real functions which relate an angle of a right-angled triangle to ratios of two side lengths. They are widely used in all sciences that are related to geometry, such as navigation, solid mechanics, celestial mechanics, geodesy, and many others. They are among the simplest periodic functions, and as such are also widely used for studying periodic phenomena through Fourier analysis. The trigonometric functions most widely used in modern mathematics are the sine, the cosine, and the tangent. Their reciprocals are respectively the cosecant, the secant, and the cotangent, which are less used. Each of these six trigonometric functions has a corresponding inverse function, and an analog among the hyperbolic functions. The oldest definitions of trigonometric functions, related to right-angle triangles, define them only for acute angles. To extend the sine and cosi ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Cosecant
In mathematics, the trigonometric functions (also called circular functions, angle functions or goniometric functions) are real functions which relate an angle of a right-angled triangle to ratios of two side lengths. They are widely used in all sciences that are related to geometry, such as navigation, solid mechanics, celestial mechanics, geodesy, and many others. They are among the simplest periodic functions, and as such are also widely used for studying periodic phenomena through Fourier analysis. The trigonometric functions most widely used in modern mathematics are the sine, the cosine, and the tangent. Their reciprocals are respectively the cosecant, the secant, and the cotangent, which are less used. Each of these six trigonometric functions has a corresponding inverse function, and an analog among the hyperbolic functions. The oldest definitions of trigonometric functions, related to right-angle triangles, define them only for acute angles. To extend the sine and cosi ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Integral Of The Secant Function
In calculus, the integral of the secant function can be evaluated using a variety of methods and there are multiple ways of expressing the antiderivative, all of which can be shown to be equivalent via trigonometric identities, : \int \sec \theta \, d\theta = \begin \dfrac12 \ln \left, \dfrac\ + C \\ 5pt\ln\left, \sec\theta + \tan\theta\ + C \\ 5pt\ln\left, \tan\left(\dfrac + \dfrac\right) \ + C\\ 5pt\end This formula is useful for evaluating various trigonometric integrals. In particular, it can be used to evaluate the integral of the secant cubed, which, though seemingly special, comes up rather frequently in applications. Proof that the different antiderivatives are equivalent Trigonometric forms : \int \sec \theta \, d\theta = \left\\text The second of these follows by first multiplying top and bottom of the interior fraction by . This gives in the denominator, and the result follows by moving the factor of into the logarithm as a square root. Leaving out the const ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Secant (trigonometric Function)
In mathematics, the trigonometric functions (also called circular functions, angle functions or goniometric functions) are real functions which relate an angle of a right-angled triangle to ratios of two side lengths. They are widely used in all sciences that are related to geometry, such as navigation, solid mechanics, celestial mechanics, geodesy, and many others. They are among the simplest periodic functions, and as such are also widely used for studying periodic phenomena through Fourier analysis. The trigonometric functions most widely used in modern mathematics are the sine, the cosine, and the tangent. Their reciprocals are respectively the cosecant, the secant, and the cotangent, which are less used. Each of these six trigonometric functions has a corresponding inverse function, and an analog among the hyperbolic functions. The oldest definitions of trigonometric functions, related to right-angle triangles, define them only for acute angles. To extend the sine and cosi ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Antiderivative
In calculus, an antiderivative, inverse derivative, primitive function, primitive integral or indefinite integral of a function is a differentiable function whose derivative is equal to the original function . This can be stated symbolically as . The process of solving for antiderivatives is called antidifferentiation (or indefinite integration), and its opposite operation is called ''differentiation'', which is the process of finding a derivative. Antiderivatives are often denoted by capital Roman letters such as and . Antiderivatives are related to definite integrals through the second fundamental theorem of calculus: the definite integral of a function over a closed interval In mathematics, a (real) interval is a set of real numbers that contains all real numbers lying between any two numbers of the set. For example, the set of numbers satisfying is an interval which contains , , and all numbers in between. Other ... where the function is Riemann integrable is eq ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Sine
In mathematics, sine and cosine are trigonometric functions of an angle. The sine and cosine of an acute angle are defined in the context of a right triangle: for the specified angle, its sine is the ratio of the length of the side that is opposite that angle to the length of the longest side of the triangle (the hypotenuse), and the cosine is the ratio of the length of the adjacent leg to that of the hypotenuse. For an angle \theta, the sine and cosine functions are denoted simply as \sin \theta and \cos \theta. More generally, the definitions of sine and cosine can be extended to any real value in terms of the lengths of certain line segments in a unit circle. More modern definitions express the sine and cosine as infinite series, or as the solutions of certain differential equations, allowing their extension to arbitrary positive and negative values and even to complex numbers. The sine and cosine functions are commonly used to model periodic phenomena such as sound and lig ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Constant Of Integration
In calculus, the constant of integration, often denoted by C (or c), is a constant term added to an antiderivative of a function f(x) to indicate that the indefinite integral of f(x) (i.e., the set of all antiderivatives of f(x)), on a connected domain, is only defined up to an additive constant. This constant expresses an ambiguity inherent in the construction of antiderivatives. More specifically, if a function f(x) is defined on an interval, and F(x) is an antiderivative of f(x), then the set of ''all'' antiderivatives of f(x) is given by the functions F(x) + C, where C is an arbitrary constant (meaning that ''any'' value of C would make F(x) + C a valid antiderivative). For that reason, the indefinite integral is often written as \int f(x) \, dx = F(x) + C, although the constant of integration might be sometimes omitted in lists of integrals for simplicity. Origin The derivative of any constant function is zero. Once one has found one antiderivative F(x) for a function f(x) ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
