|
Lehmer Pair
In the study of the Riemann hypothesis, a Lehmer pair is a pair of zeros of the Riemann zeta function that are unusually close to each other. They are named after Derrick Henry Lehmer, who discovered the pair of zeros : \begin & \tfrac 1 2 + i\,7005.06266\dots \\ pt& \tfrac 1 2 + i\,7005.10056\dots \end (the 6709th and 6710th zeros of the zeta function). More precisely, a Lehmer pair can be defined as having the property that their complex coordinates \gamma_n and \gamma_ obey the inequality :\frac \ge C\sum_ \left(\frac+\frac\right) for a constant C>5/4. It is an unsolved problem whether there exist infinitely many Lehmer pairs. If so, it would imply that the De Bruijn–Newman constant is non-negative, a fact that has been proven unconditionally by Brad Rodgers and Terence Tao. See also * Montgomery's pair correlation conjecture References {{reflist, refs= {{citation , last1 = Csordas , first1 = George , last2 = Smith , first2 = Wayne , last3 = Varga , first3 ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Riemann Hypothesis
In mathematics, the Riemann hypothesis is the conjecture that the Riemann zeta function has its zeros only at the negative even integers and complex numbers with real part . Many consider it to be the most important unsolved problem in pure mathematics. It is of great interest in number theory because it implies results about the distribution of prime numbers. It was proposed by , after whom it is named. The Riemann hypothesis and some of its generalizations, along with Goldbach's conjecture and the twin prime conjecture, make up Hilbert's eighth problem in David Hilbert's list of twenty-three unsolved problems; it is also one of the Clay Mathematics Institute's Millennium Prize Problems, which offers a million dollars to anyone who solves any of them. The name is also used for some closely related analogues, such as the Riemann hypothesis for curves over finite fields. The Riemann zeta function ζ(''s'') is a function whose argument ''s'' may be any complex number ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Zero Of A Function
In mathematics, a zero (also sometimes called a root) of a real-, complex-, or generally vector-valued function f, is a member x of the domain of f such that f(x) ''vanishes'' at x; that is, the function f attains the value of 0 at x, or equivalently, x is the solution to the equation f(x) = 0. A "zero" of a function is thus an input value that produces an output of 0. A root of a polynomial is a zero of the corresponding polynomial function. The fundamental theorem of algebra shows that any non-zero polynomial has a number of roots at most equal to its degree, and that the number of roots and the degree are equal when one considers the complex roots (or more generally, the roots in an algebraically closed extension) counted with their multiplicities. For example, the polynomial f of degree two, defined by f(x)=x^2-5x+6 has the two roots (or zeros) that are 2 and 3. f(2)=2^2-5\times 2+6= 0\textf(3)=3^2-5\times 3+6=0. If the function maps real numbers to real numbers, then it ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
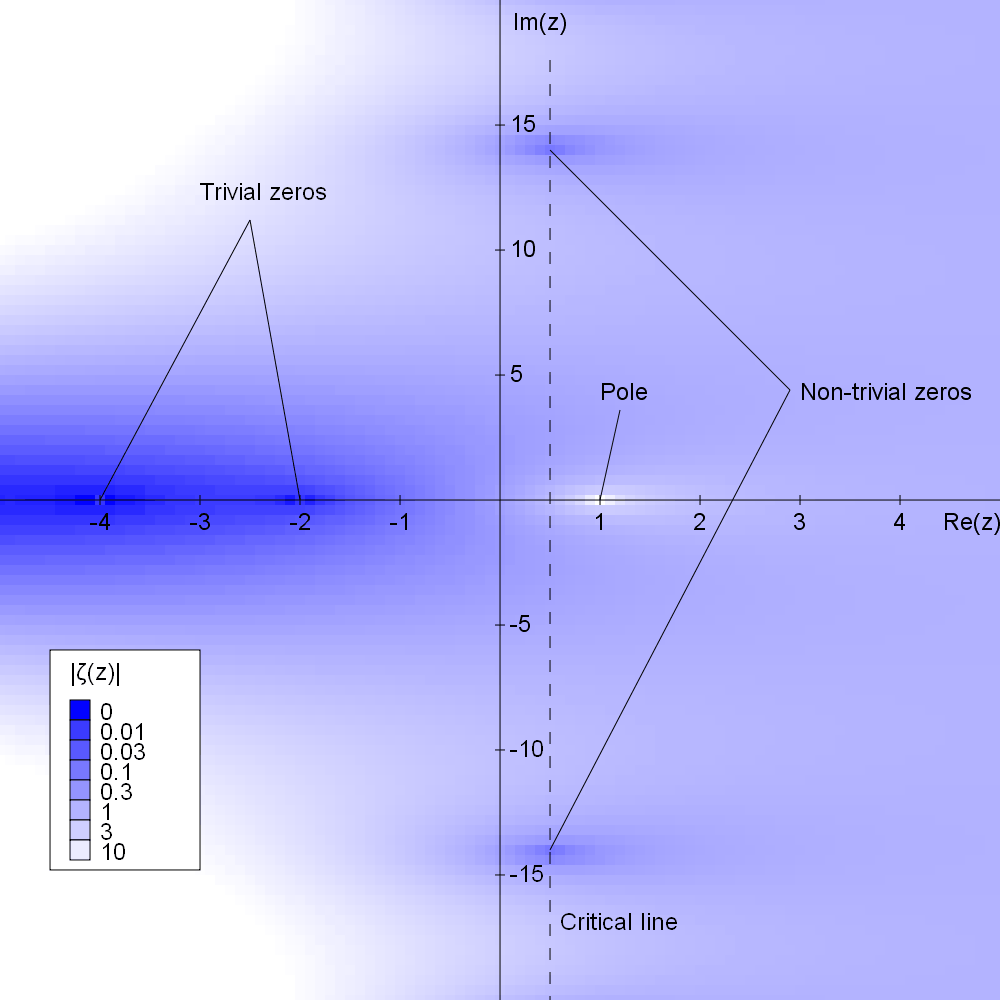
Riemann Zeta Function
The Riemann zeta function or Euler–Riemann zeta function, denoted by the Greek letter (zeta), is a mathematical function of a complex variable defined as \zeta(s) = \sum_^\infty \frac = \frac + \frac + \frac + \cdots for \operatorname(s) > 1 and its analytic continuation elsewhere. The Riemann zeta function plays a pivotal role in analytic number theory, and has applications in physics, probability theory, and applied statistics. Leonhard Euler first introduced and studied the function over the reals in the first half of the eighteenth century. Bernhard Riemann's 1859 article "On the Number of Primes Less Than a Given Magnitude" extended the Euler definition to a complex variable, proved its meromorphic continuation and functional equation, and established a relation between its zeros and the distribution of prime numbers. This paper also contained the Riemann hypothesis, a conjecture about the distribution of complex zeros of the Riemann zeta function that is consid ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Derrick Henry Lehmer
Derrick Henry "Dick" Lehmer (February 23, 1905 – May 22, 1991), almost always cited as D.H. Lehmer, was an American mathematician significant to the development of computational number theory. Lehmer refined Édouard Lucas' work in the 1930s and devised the Lucas–Lehmer primality test, Lucas–Lehmer test for Mersenne primes. His peripatetic career as a Number theory, number theorist, with him and his wife taking numerous types of work in the United States and abroad to support themselves during the Great Depression, fortuitously brought him into the center of research into early electronic computing. Early life Lehmer was born in Berkeley, California, to Derrick Norman Lehmer, a professor of mathematics at the University of California, Berkeley, and Clara Eunice Mitchell. He studied physics and earned a Bachelor degree from UC Berkeley, and continued with graduate studies at the University of Chicago. He and his father worked together on Lehmer sieves. Marriage During his s ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
De Bruijn–Newman Constant
The de Bruijn–Newman constant, denoted by Λ and named after Nicolaas Govert de Bruijn and Charles M. Newman, is a mathematical constant defined via the zero of a function, zeros of a certain function (mathematics), function ''H''(''λ'', ''z''), where ''λ'' is a real number, real parameter and ''z'' is a complex number, complex variable. More precisely, :H(\lambda, z):=\int_^ e^ \Phi(u) \cos (z u) d u, where \Phi is the super-exponential function, super-exponentially decaying function :\Phi(u) = \sum_^ (2\pi^2n^4e^ - 3 \pi n^2 e^ ) e^ and Λ is the unique real number with the property that ''H'' has only real zeros if and only if ''λ'' ≥ Λ. The constant is closely connected with Riemann hypothesis, Riemann's hypothesis concerning the zeros of the Riemann zeta function, Riemann zeta-function: since the Riemann hypothesis is equivalent to the claim that all the zeroes of ''H''(0, ''z'') are real, the Riemann hypothesis is equivalent to the conjecture tha ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Terence Tao
Terence Chi-Shen Tao (; born 17 July 1975) is an Australian-American mathematician. He is a professor of mathematics at the University of California, Los Angeles (UCLA), where he holds the James and Carol Collins chair. His research includes topics in harmonic analysis, partial differential equations, algebraic combinatorics, arithmetic combinatorics, geometric combinatorics, probability theory, compressed sensing and analytic number theory. Tao was born to ethnic Chinese immigrant parents and raised in Adelaide. Tao won the Fields Medal in 2006 and won the Royal Medal and Breakthrough Prize in Mathematics in 2014. He is also a 2006 MacArthur Fellow. Tao has been the author or co-author of over three hundred research papers. He is widely regarded as one of the greatest living mathematicians and has been referred to as the "Mozart of mathematics". Life and career Family Tao's parents are first-generation immigrants from Hong Kong to Australia.''Wen Wei Po'', Page A4, 24 Au ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Montgomery's Pair Correlation Conjecture
In mathematics, Montgomery's pair correlation conjecture is a conjecture made by that the pair correlation between pairs of zeros of the Riemann zeta function (normalized to have unit average spacing) is :1-\left(\frac\right)^ + \delta(u), which, as Freeman Dyson pointed out to him, is the same as the pair correlation function of random Hermitian matrices. Conjecture ''Under the assumption that the Riemann Hypothesis is true.'' Let \alpha\leq \beta be fixed, as T\to \infty'' : N(T;\alpha,\beta)\, := \, \sum_ 1 \, \sim \, \left( \int\limits_\alpha^\beta \left(1-\left(\frac\right)^2 \right) \mathrmu +\delta_( alpha,\beta\right)\frac\log\frac and we count over A = \, where each \gamma, \gamma' is the imaginary part of the non-trivial zeros of zeta function, that is \tfrac+i\gamma. Also \delta_0 denotes the delta measure supported at 0. Explanation Informally, this means that the chance of finding a zero in a very short interval of length 2π''L''/log(''T'') at a distanc ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Constructive Approximation
''Constructive Approximation'' is "an international mathematics journal dedicated to Approximations and Expansions and related research in computation, function theory, functional analysis, interpolation spaces and interpolation of operators, numerical analysis, space of functions, special functions, and applications." References External links Constructive Approximation web site Mathematics journals Approximation theory English-language journals Publications established in 1985 Springer Science+Business Media academic journals Bimonthly journals {{math-journal-stub ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Acta Mathematica
''Acta Mathematica'' is a peer-reviewed open-access scientific journal covering research in all fields of mathematics. According to Cédric Villani, this journal is "considered by many to be the most prestigious of all mathematical research journals".. According to the ''Journal Citation Reports'', the journal has a 2020 impact factor of 4.273, ranking it 5th out of 330 journals in the category "Mathematics". Publication history The journal was established by Gösta Mittag-Leffler in 1882 and is published by Institut Mittag-Leffler, a research institute for mathematics belonging to the Royal Swedish Academy of Sciences. The journal was printed and distributed by Springer from 2006 to 2016. Since 2017, Acta Mathematica has been published electronically and in print by International Press. Its electronic version is open access without publishing fees. Poincaré episode The journal's "most famous episode" (according to Villani) concerns Henri Poincaré, who won a prize offered ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Forum Of Mathematics
''Forum of Mathematics, Pi'' and ''Forum of Mathematics, Sigma'' are open-access peer-reviewed journals for mathematics published under a creative commons license by Cambridge University Press. The founding managing editor was Rob Kirby. He was succeeded by Robert Guralnick, who is currently the managing editor of both journals. ''Forum of Mathematics, Pi'' publishes articles of interest to a wide audience of mathematicians, while ''Forum of Mathematics, Sigma'' is intended for more specialized articles, with clusters of editors in different areas of mathematics. Abstracting and indexing Both journals are abstracted and indexed in Science Citation Index Expanded, MathSciNet, and Scopus Scopus is Elsevier's abstract and citation database launched in 2004. Scopus covers nearly 36,377 titles (22,794 active titles and 13,583 inactive titles) from approximately 11,678 publishers, of which 34,346 are peer-reviewed journals in top-l .... References External links A new open- ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
