|
Keith Briggs (mathematician)
Keith Briggs is a mathematician notable for several world-record achievements in the field of computational mathematics: *The most accurate calculation of the Feigenbaum constants, which was published in "A precise calculation of the Feigenbaum constants", ''Mathematics of Computation'', 57, 435–439. *The worst known badly approximable irrational pair ("Some explicit badly approximable pairs", ''Journal of Number Theory'', 103, 71). *The simplest known universal differential equation *The largest number of contributions in the last 5 years to Sloane's On-Line Encyclopedia of Integer Sequencessearch for briggs in OEIS. Many of these have involved major computations, such as the number of unlabelled graphs on up to 140 nodes. *The computation of the longest sequences of colossally abundant and superabundant numbers, and their application to a test of the Riemann Hypothesis (''Experimental Mathematics'', 15, 251–256). An article about him was in ''i-squared Magazine'', Issue 6 ( ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
World-record
A world record is usually the best global and most important performance that is ever recorded and officially verified in a specific skill, sport, or other kind of activity. The book ''Guinness World Records'' and other world records organizations collates and publishes notable records of many. One of them is the World Records Union that is the unique world records register organization recognized by the Council of the Notariats of the European Union. Terminology In the United States, the form World's Record was formerly more common. The term The World's Best was also briefly in use. The latter term is still used in athletics events, including track and field and road running to describe good and bad performances that are not recognized as an official world record: either because it is not an event where the IAAF tracks the record (e.g. the 150 m run or individual events in a decathlon), or because it does not fulfill other rigorous criteria of an otherwise qualifying event (e.g ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Feigenbaum Constants
In mathematics, specifically bifurcation theory, the Feigenbaum constants are two mathematical constants which both express ratios in a bifurcation diagram for a non-linear map. They are named after the physicist Mitchell J. Feigenbaum. History Feigenbaum originally related the first constant to the period-doubling bifurcations in the logistic map, but also showed it to hold for all one-dimensional maps with a single quadratic maximum. As a consequence of this generality, every chaotic system that corresponds to this description will bifurcate at the same rate. Feigenbaum made this discovery in 1975, and he officially published it in 1978. The first constant The first Feigenbaum constant is the limiting ratio of each bifurcation interval to the next between every period doubling, of a one-parameter map :x_ = f(x_i), where is a function parameterized by the bifurcation parameter . It is given by the limit :\delta = \lim_ \frac = 4.669\,201\,609\,\ldots, where are discret ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Universal Differential Equation
A universal differential equation (UDE) is a non-trivial differential algebraic equation with the property that its solutions can approximate any continuous function on any interval of the real line to any desired level of accuracy. Precisely, a (possibly implicit) differential equation ''P''(''y''', ''y'''', ''y'', ... , ''y''(''n'')) = 0 is a UDE if for any continuous real-valued function ''f'' and for any positive continuous function ''ε'' there exist a smooth solution ''y'' of ''P''(''y''', ''y'''', ''y'', ... , ''y''(''n'')) = 0 with , ''y''(''x'') − ''f''(''x''), 3. * Briggs proposed another family of UDEs whose construction is based on Jacobi elliptic functions: :y^ y^-3 y^ y^ y^+2\left(1-n^\right) y^=0, where ''n'' > 3. * Bournez and Pouly proved the existence of a fixed polynomial vector field ''p'' such that for any ''f'' and ''ε'' there exists some initial condition of the differential equation y' = p(y) that yields a unique and analytic solution satisfying , ''y ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
On-Line Encyclopedia Of Integer Sequences
The On-Line Encyclopedia of Integer Sequences (OEIS) is an online database of integer sequences. It was created and maintained by Neil Sloane while researching at AT&T Labs. He transferred the intellectual property and hosting of the OEIS to the OEIS Foundation in 2009. Sloane is chairman of the OEIS Foundation. OEIS records information on integer sequences of interest to both professional and amateur mathematicians, and is widely cited. , it contains over 350,000 sequences, making it the largest database of its kind. Each entry contains the leading terms of the sequence, keywords, mathematical motivations, literature links, and more, including the option to generate a graph or play a musical representation of the sequence. The database is searchable by keyword, by subsequence, or by any of 16 fields. History Neil Sloane started collecting integer sequences as a graduate student in 1965 to support his work in combinatorics. The database was at first stored on punche ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Abundant Numbers
In number theory, an abundant number or excessive number is a number for which the sum of its proper divisors is greater than the number. The integer 12 is the first abundant number. Its proper divisors are 1, 2, 3, 4 and 6 for a total of 16. The amount by which the sum exceeds the number is the abundance. The number 12 has an abundance of 4, for example. Definition A number ''n'' for which the ''sum'' ''of'' ''divisors'' ''σ''(''n'') > 2''n'', or, equivalently, the sum of proper divisors (or aliquot sum) ''s''(''n'') > ''n''. Abundance is the value ''σ''(''n'') − ''2n'' (or ''s''(''n'') − ''n''). Examples The first 28 abundant numbers are: :12, 18, 20, 24, 30, 36, 40, 42, 48, 54, 56, 60, 66, 70, 72, 78, 80, 84, 88, 90, 96, 100, 102, 104, 108, 112, 114, 120, ... . For example, the proper divisors of 24 are 1, 2, 3, 4, 6, 8, and 12, whose sum is 36. Because 36 is greater than 24, the number 24 is abundant. Its abundance is 36 − 24 = 12. Prope ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
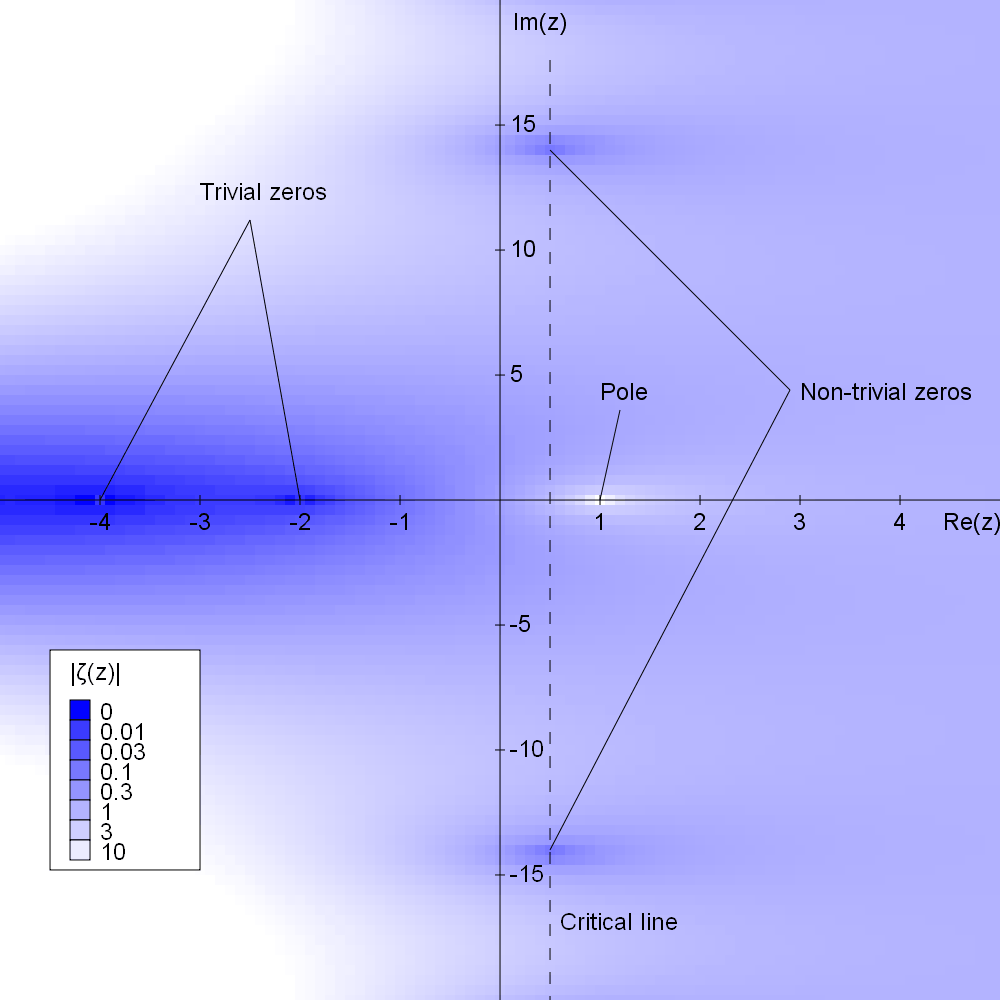
Riemann Hypothesis
In mathematics, the Riemann hypothesis is the conjecture that the Riemann zeta function has its zeros only at the negative even integers and complex numbers with real part . Many consider it to be the most important unsolved problem in pure mathematics. It is of great interest in number theory because it implies results about the distribution of prime numbers. It was proposed by , after whom it is named. The Riemann hypothesis and some of its generalizations, along with Goldbach's conjecture and the twin prime conjecture, make up Hilbert's eighth problem in David Hilbert's list of twenty-three unsolved problems; it is also one of the Clay Mathematics Institute's Millennium Prize Problems, which offers a million dollars to anyone who solves any of them. The name is also used for some closely related analogues, such as the Riemann hypothesis for curves over finite fields. The Riemann zeta function ζ(''s'') is a function whose argument ''s'' may be any complex number ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Erdős Number
The Erdős number () describes the "collaborative distance" between mathematician Paul Erdős and another person, as measured by authorship of mathematical papers. The same principle has been applied in other fields where a particular individual has collaborated with a large and broad number of peers. Overview Paul Erdős (1913–1996) was an influential Hungarian mathematician who in the latter part of his life spent a great deal of time writing papers with a large number of colleagues, working on solutions to outstanding mathematical problems. He published more papers during his lifetime (at least 1,525) than any other mathematician in history. (Leonhard Euler published more total pages of mathematics but fewer separate papers: about 800.) Erdős spent a large portion of his later life living out of a suitcase, visiting over 500 collaborators around the world. The idea of the Erdős number was originally created by the mathematician's friends as a tribute to his enormous o ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
George Szekeres
George Szekeres AM FAA (; 29 May 1911 – 28 August 2005) was a Hungarian–Australian mathematician. Early years Szekeres was born in Budapest, Hungary, as Szekeres György and received his degree in chemistry at the Technical University of Budapest. He worked six years in Budapest as an analytical chemist. He married Esther Klein in 1937.Obituary The Sydney Morning Herald Being , the family had to escape from the persecution so Szekeres took a job in Shanghai, China. There they lived through World War II, the Japanese occupation and the ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Place-name
Toponymy, toponymics, or toponomastics is the study of ''toponyms'' (proper names of places, also known as place names and geographic names), including their origins, meanings, usage and types. Toponym is the general term for a proper name of any geographical feature, and full scope of the term also includes proper names of all cosmographical features. In a more specific sense, the term ''toponymy'' refers to an inventory of toponyms, while the discipline researching such names is referred to as ''toponymics'' or ''toponomastics''. Toponymy is a branch of onomastics, the study of proper names of all kinds. A person who studies toponymy is called ''toponymist''. Etymology The term toponymy come from grc, τόπος / , 'place', and / , 'name'. The ''Oxford English Dictionary'' records ''toponymy'' (meaning "place name") first appearing in English in 1876. Since then, ''toponym'' has come to replace the term ''place-name'' in professional discourse among geographers. Toponym ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
21st-century English Mathematicians
The 1st century was the century spanning AD 1 ( I) through AD 100 ( C) according to the Julian calendar. It is often written as the or to distinguish it from the 1st century BC (or BCE) which preceded it. The 1st century is considered part of the Classical era, epoch, or historical period. The 1st century also saw the appearance of Christianity. During this period, Europe, North Africa and the Near East fell under increasing domination by the Roman Empire, which continued expanding, most notably conquering Britain under the emperor Claudius (AD 43). The reforms introduced by Augustus during his long reign stabilized the empire after the turmoil of the previous century's civil wars. Later in the century the Julio-Claudian dynasty, which had been founded by Augustus, came to an end with the suicide of Nero in AD 68. There followed the famous Year of Four Emperors, a brief period of civil war and instability, which was finally brought to an end by Vespasian, ninth Roman ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Toponymists
Toponymy, toponymics, or toponomastics is the study of '' toponyms'' (proper names of places, also known as place names and geographic names), including their origins, meanings, usage and types. Toponym is the general term for a proper name of any geographical feature, and full scope of the term also includes proper names of all cosmographical features. In a more specific sense, the term ''toponymy'' refers to an inventory of toponyms, while the discipline researching such names is referred to as ''toponymics'' or ''toponomastics''. Toponymy is a branch of onomastics, the study of proper names of all kinds. A person who studies toponymy is called ''toponymist''. Etymology The term toponymy come from grc, τόπος / , 'place', and / , 'name'. The ''Oxford English Dictionary'' records ''toponymy'' (meaning "place name") first appearing in English in 1876. Since then, ''toponym'' has come to replace the term ''place-name'' in professional discourse among geographers. Topon ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Year Of Birth Missing (living People)
A year or annus is the orbital period of a planetary body, for example, the Earth, moving in its orbit around the Sun. Due to the Earth's axial tilt, the course of a year sees the passing of the seasons, marked by change in weather, the hours of daylight, and, consequently, vegetation and soil fertility. In temperate and subpolar regions around the planet, four seasons are generally recognized: spring, summer, autumn and winter. In tropical and subtropical regions, several geographical sectors do not present defined seasons; but in the seasonal tropics, the annual wet and dry seasons are recognized and tracked. A calendar year is an approximation of the number of days of the Earth's orbital period, as counted in a given calendar. The Gregorian calendar, or modern calendar, presents its calendar year to be either a common year of 365 days or a leap year of 366 days, as do the Julian calendars. For the Gregorian calendar, the average length of the calendar yea ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |