|
Kaleidocycle With Scalene Triangles
A kaleidocycle or flextangle is a flexible polyhedron connecting six tetrahedra (or disphenoids) on opposite edges into a cycle. If the faces of the disphenoids are equilateral triangles, it can be constructed from a stretched triangular tiling net with four triangles in one direction and an even number in the other direction. The kaleidocycle has degenerate pairs of coinciding edges in transition, which function as hinges. The kaleidocycle has an additional property that it can be continuously twisted around a ring axis, showing 4 sets of 6 triangular faces. The kaleidocycle is invariant under twists about its ring axis by k\pi/2, where k is an integer, and can therefore be continuously twisted. Kaleidocycles can be constructed from a single piece of paper (with dimensions l \times 2l) without tearing or using adhesive. Because of this and their continuous twisting property, they are often given as examples of simple origami toys. The kaleidocycle is sometimes called a flexahedro ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Flexagon
In geometry, flexagons are flat models, usually constructed by folding strips of paper, that can be ''flexed'' or folded in certain ways to reveal faces besides the two that were originally on the back and front. Flexagons are usually square or rectangular (tetraflexagons) or hexagonal (hexaflexagons). A prefix can be added to the name to indicate the number of faces that the model can display, including the two faces (back and front) that are visible before flexing. For example, a hexaflexagon with a total of six faces is called a hexahexaflexagon. In hexaflexagon theory (that is, concerning flexagons with six sides), flexagons are usually defined in terms of ''pats''. Two flexagons are equivalent if one can be transformed to the other by a series of pinches and rotations. Flexagon equivalence is an equivalence relation. History Discovery and introduction The discovery of the first flexagon, a trihexaflexagon, is credited to the British mathematician Arthur H. Stone, whil ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Schwarz Lantern
In mathematics, the Schwarz lantern is a polyhedral approximation to a cylinder, used as a pathological example of the difficulty of defining the area of a smooth (curved) surface as the limit of the areas of polyhedra. It is formed by stacked rings of isosceles triangles, arranged within each ring in the same pattern as an antiprism. The resulting shape can be folded from paper, and is named after mathematician Hermann Schwarz and for its resemblance to a cylindrical paper lantern. It is also known as Schwarz's boot, Schwarz's polyhedron, or the Chinese lantern. As Schwarz showed, for the surface area of a polyhedron to converge to the surface area of a curved surface, it is not sufficient to simply increase the number of rings and the number of isosceles triangles per ring. Depending on the relation of the number of rings to the number of triangles per ring, the area of the lantern can converge to the area of the cylinder, to a limit arbitrarily larger than the area of the ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Flexible Polyhedron
In geometry, a flexible polyhedron is a polyhedral surface without any boundary edges, whose shape can be continuously changed while keeping the shapes of all of its faces unchanged. The Cauchy rigidity theorem shows that in dimension 3 such a polyhedron cannot be convex (this is also true in higher dimensions). The first examples of flexible polyhedra, now called Bricard octahedra, were discovered by . They are self-intersecting surfaces isometric to an octahedron. The first example of a flexible non-self-intersecting surface in \mathbb^3, the Connelly sphere, was discovered by . Steffen's polyhedron is another non-self-intersecting flexible polyhedron derived from Bricard's octahedra. Bellows conjecture In the late 1970s Connelly and D. Sullivan formulated the bellows conjecture stating that the volume of a flexible polyhedron is invariant under flexing. This conjecture was proved for polyhedra homeomorphic to a sphere by using elimination theory, and then pro ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Flexagon
In geometry, flexagons are flat models, usually constructed by folding strips of paper, that can be ''flexed'' or folded in certain ways to reveal faces besides the two that were originally on the back and front. Flexagons are usually square or rectangular (tetraflexagons) or hexagonal (hexaflexagons). A prefix can be added to the name to indicate the number of faces that the model can display, including the two faces (back and front) that are visible before flexing. For example, a hexaflexagon with a total of six faces is called a hexahexaflexagon. In hexaflexagon theory (that is, concerning flexagons with six sides), flexagons are usually defined in terms of ''pats''. Two flexagons are equivalent if one can be transformed to the other by a series of pinches and rotations. Flexagon equivalence is an equivalence relation. History Discovery and introduction The discovery of the first flexagon, a trihexaflexagon, is credited to the British mathematician Arthur H. Stone, whil ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
A Wrinkle In Time (2018 Film)
''A Wrinkle in Time'' is a 2018 American science fantasy adventure film directed by Ava DuVernay and written by Jennifer Lee and Jeff Stockwell, based on Madeleine L'Engle's 1962 novel of the same name. Produced by Walt Disney Pictures and Whitaker Entertainment, the story follows a young girl who, with the help of three astral travelers, sets off on a quest to find her missing father. The film stars Oprah Winfrey, Reese Witherspoon, Mindy Kaling, Levi Miller, Storm Reid, Gugu Mbatha-Raw, Michael Peña, Zach Galifianakis, and Chris Pine. It is Disney's second film adaptation of L'Engle's novel, following a 2003 television film. Development began in 2010, with DuVernay signing on to direct in February 2016. Principal photography began on November 2, 2016 in Los Angeles, California. Near the end of filming, production moved to New Zealand, where photography ended on February 25, 2017. With an estimated production budget of $103 million, the film became the first $100-million-bu ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Doris Schattschneider
Doris J. Schattschneider (née Wood) is an American mathematician, a retired professor of mathematics at Moravian College. She is known for writing about tessellations and about the art of M. C. Escher,.. for helping Martin Gardner validate and popularize the pentagon tiling discoveries of amateur mathematician Marjorie Rice, and for co-directing with Eugene Klotz the project that developed The Geometer's Sketchpad. Biography Schattschneider was born in Staten Island; her mother, Charlotte Lucile Ingalls Wood, taught Latin and was herself the daughter of a Staten Island school principal, and her father, Robert W. Wood, Jr., was an electrical engineer who worked for the New York City Bureau of Bridge Design.. Her family moved to Lake Placid, New York during World War II, while her father served as an engineer for the U. S. Army; she began her schooling in Lake Placid, but returned to Staten Island after the war. She did her undergraduate studies in mathematics at the University of ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Mathematica
Wolfram Mathematica is a software system with built-in libraries for several areas of technical computing that allow machine learning, statistics, symbolic computation, data manipulation, network analysis, time series analysis, NLP, optimization, plotting functions and various types of data, implementation of algorithms, creation of user interfaces, and interfacing with programs written in other programming languages. It was conceived by Stephen Wolfram, and is developed by Wolfram Research of Champaign, Illinois. The Wolfram Language is the programming language used in ''Mathematica''. Mathematica 1.0 was released on June 23, 1988 in Champaign, Illinois and Santa Clara, California. __TOC__ Notebook interface Wolfram Mathematica (called ''Mathematica'' by some of its users) is split into two parts: the kernel and the front end. The kernel interprets expressions (Wolfram Language code) and returns result expressions, which can then be displayed by the front end. The origin ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Kaleidocycle With Scalene Triangles
A kaleidocycle or flextangle is a flexible polyhedron connecting six tetrahedra (or disphenoids) on opposite edges into a cycle. If the faces of the disphenoids are equilateral triangles, it can be constructed from a stretched triangular tiling net with four triangles in one direction and an even number in the other direction. The kaleidocycle has degenerate pairs of coinciding edges in transition, which function as hinges. The kaleidocycle has an additional property that it can be continuously twisted around a ring axis, showing 4 sets of 6 triangular faces. The kaleidocycle is invariant under twists about its ring axis by k\pi/2, where k is an integer, and can therefore be continuously twisted. Kaleidocycles can be constructed from a single piece of paper (with dimensions l \times 2l) without tearing or using adhesive. Because of this and their continuous twisting property, they are often given as examples of simple origami toys. The kaleidocycle is sometimes called a flexahedro ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
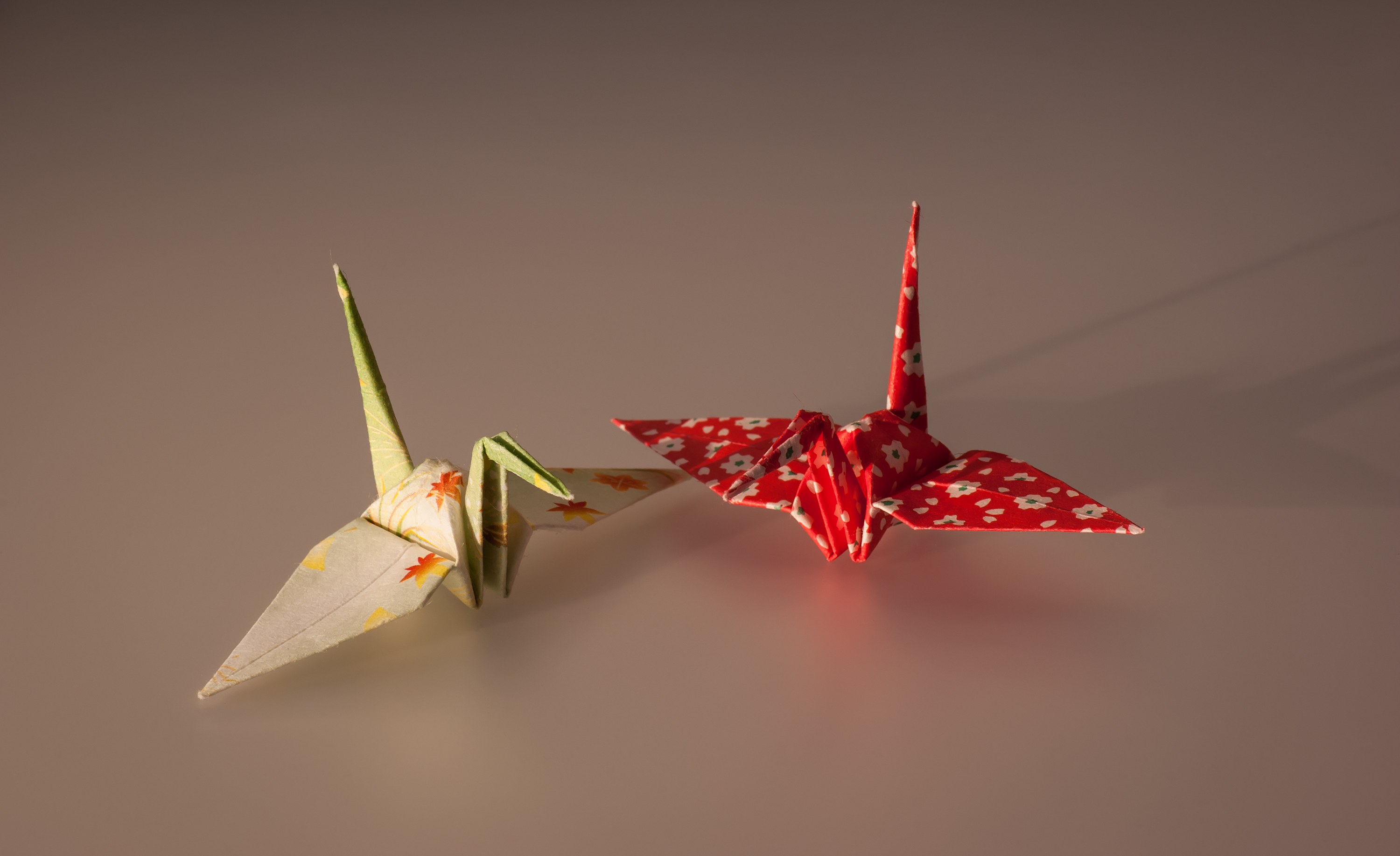
Origami
) is the Japanese paper art, art of paper folding. In modern usage, the word "origami" is often used as an inclusive term for all folding practices, regardless of their culture of origin. The goal is to transform a flat square sheet of paper into a finished sculpture through folding and sculpting techniques. Modern origami practitioners generally discourage the use of cuts, glue, or markings on the paper. Origami folders often use the Japanese word ' to refer to designs which use cuts. On the other hand, in the detailed Japanese classification, origami is divided into stylized ceremonial origami (儀礼折り紙, ''girei origami'') and recreational origami (遊戯折り紙, ''yūgi origami''), and only recreational origami is generally recognized as origami. In Japan, ceremonial origami is generally called "origata" (:ja:折形) to distinguish it from recreational origami. The term "origata" is one of the old terms for origami. The small number of basic Origami techniques, ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Isosceles Triangle
In geometry, an isosceles triangle () is a triangle that has two sides of equal length. Sometimes it is specified as having ''exactly'' two sides of equal length, and sometimes as having ''at least'' two sides of equal length, the latter version thus including the equilateral triangle as a special case. Examples of isosceles triangles include the isosceles right triangle, the golden triangle, and the faces of bipyramids and certain Catalan solids. The mathematical study of isosceles triangles dates back to ancient Egyptian mathematics and Babylonian mathematics. Isosceles triangles have been used as decoration from even earlier times, and appear frequently in architecture and design, for instance in the pediments and gables of buildings. The two equal sides are called the legs and the third side is called the base of the triangle. The other dimensions of the triangle, such as its height, area, and perimeter, can be calculated by simple formulas from the lengths of the legs an ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Triangular Tiling
In geometry, the triangular tiling or triangular tessellation is one of the three regular tilings of the Euclidean plane, and is the only such tiling where the constituent shapes are not parallelogons. Because the internal angle of the equilateral triangle is 60 degrees, six triangles at a point occupy a full 360 degrees. The triangular tiling has Schläfli symbol of English mathematician John Conway called it a deltille, named from the triangular shape of the Greek letter delta (Δ). The triangular tiling can also be called a kishextille by a kis operation that adds a center point and triangles to replace the faces of a hextille. It is one of three regular tilings of the plane. The other two are the square tiling and the hexagonal tiling. Uniform colorings There are 9 distinct uniform colorings of a triangular tiling. (Naming the colors by indices on the 6 triangles around a vertex: 111111, 111112, 111212, 111213, 111222, 112122, 121212, 121213, 121314) Three of them can ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |