|
James P. Pierpont
James P. Pierpont (June 16, 1866 – December 9, 1938) was a Connecticut-born American mathematician. His father Cornelius Pierpont was a wealthy New Haven businessman. He did undergraduate studies at Worcester Polytechnic Institute, initially in mechanical engineering, but turned to mathematics. He went to Europe after graduating in 1886. He studied in Berlin, and later in Vienna. He prepared his PhD at the University of Vienna under Leopold Gegenbauer Leopold Bernhard Gegenbauer (2 February 1849, Asperhofen – 3 June 1903, Gießhübl) was an Austrian mathematician remembered best as an algebraist. Gegenbauer polynomials are named after him. Leopold Gegenbauer was the son of a doctor. He ... and Gustav Ritter von Escherich. His thesis, defended in 1894, is entitled ''Zur Geschichte der Gleichung fünften Grades bis zum Jahre 1858''. After his defense, he returned to New Haven and was appointed as a lecturer at Yale University, where he spent most of his car ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Connecticut
Connecticut () is the southernmost state in the New England region of the Northeastern United States. It is bordered by Rhode Island to the east, Massachusetts to the north, New York to the west, and Long Island Sound to the south. Its capital is Hartford and its most populous city is Bridgeport. Historically the state is part of New England as well as the tri-state area with New York and New Jersey. The state is named for the Connecticut River which approximately bisects the state. The word "Connecticut" is derived from various anglicized spellings of "Quinnetuket”, a Mohegan-Pequot word for "long tidal river". Connecticut's first European settlers were Dutchmen who established a small, short-lived settlement called House of Hope in Hartford at the confluence of the Park and Connecticut Rivers. Half of Connecticut was initially claimed by the Dutch colony New Netherland, which included much of the land between the Connecticut and Delaware Rivers, although the firs ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Yale University
Yale University is a private research university in New Haven, Connecticut. Established in 1701 as the Collegiate School, it is the third-oldest institution of higher education in the United States and among the most prestigious in the world. It is a member of the Ivy League. Chartered by the Connecticut Colony, the Collegiate School was established in 1701 by clergy to educate Congregational ministers before moving to New Haven in 1716. Originally restricted to theology and sacred languages, the curriculum began to incorporate humanities and sciences by the time of the American Revolution. In the 19th century, the college expanded into graduate and professional instruction, awarding the first PhD in the United States in 1861 and organizing as a university in 1887. Yale's faculty and student populations grew after 1890 with rapid expansion of the physical campus and scientific research. Yale is organized into fourteen constituent schools: the original undergraduate col ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
19th-century American Mathematicians
The 19th (nineteenth) century began on 1 January 1801 ( MDCCCI), and ended on 31 December 1900 ( MCM). The 19th century was the ninth century of the 2nd millennium. The 19th century was characterized by vast social upheaval. Slavery was abolished in much of Europe and the Americas. The First Industrial Revolution, though it began in the late 18th century, expanding beyond its British homeland for the first time during this century, particularly remaking the economies and societies of the Low Countries, the Rhineland, Northern Italy, and the Northeastern United States. A few decades later, the Second Industrial Revolution led to ever more massive urbanization and much higher levels of productivity, profit, and prosperity, a pattern that continued into the 20th century. The Islamic gunpowder empires fell into decline and European imperialism brought much of South Asia, Southeast Asia, and almost all of Africa under colonial rule. It was also marked by the collapse of the large S ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
1938 Deaths
Events January * January 1 ** The new constitution of Estonia enters into force, which many consider to be the ending of the Era of Silence and the authoritarian regime. ** State-owned railway networks are created by merger, in France ( SNCF) and the Netherlands (Nederlandse Spoorwegen – NS). * January 20 – King Farouk of Egypt marries Safinaz Zulficar, who becomes Queen Farida, in Cairo. * January 27 – The Honeymoon Bridge at Niagara Falls, New York, collapses as a result of an ice jam. February * February 4 ** Adolf Hitler abolishes the War Ministry and creates the Oberkommando der Wehrmacht (High Command of the Armed Forces), giving him direct control of the German military. In addition, he dismisses political and military leaders considered unsympathetic to his philosophy or policies. General Werner von Fritsch is forced to resign as Commander of Chief of the German Army following accusations of homosexuality, and replaced by General Walther ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
1866 Births
Events January–March * January 1 ** Fisk University, a historically black university, is established in Nashville, Tennessee. ** The last issue of the abolitionist magazine '' The Liberator'' is published. * January 6 – Ottoman troops clash with supporters of Maronite leader Youssef Bey Karam, at St. Doumit in Lebanon; the Ottomans are defeated. * January 12 ** The ''Royal Aeronautical Society'' is formed as ''The Aeronautical Society of Great Britain'' in London, the world's oldest such society. ** British auxiliary steamer sinks in a storm in the Bay of Biscay, on passage from the Thames to Australia, with the loss of 244 people, and only 19 survivors. * January 18 – Wesley College, Melbourne, is established. * January 26 – Volcanic eruption in the Santorini caldera begins. * February 7 – Battle of Abtao: A Spanish naval squadron fights a combined Peruvian-Chilean fleet, at the island of Abtao, in the Chiloé Archipelago of southern Chile. * February 13 � ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Bulletin Of The American Mathematical Society
The ''Bulletin of the American Mathematical Society'' is a quarterly mathematical journal published by the American Mathematical Society. Scope It publishes surveys on contemporary research topics, written at a level accessible to non-experts. It also publishes, by invitation only, book reviews and short ''Mathematical Perspectives'' articles. History It began as the ''Bulletin of the New York Mathematical Society'' and underwent a name change when the society became national. The Bulletin's function has changed over the years; its original function was to serve as a research journal for its members. Indexing The Bulletin is indexed in Mathematical Reviews, Science Citation Index, ISI Alerting Services, CompuMath Citation Index, and Current Contents/Physical, Chemical & Earth Sciences. See also *'' Journal of the American Mathematical Society'' *''Memoirs of the American Mathematical Society'' *''Notices of the American Mathematical Society'' *'' Proceedings of the American M ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Non-Euclidean Geometry
In mathematics, non-Euclidean geometry consists of two geometries based on axioms closely related to those that specify Euclidean geometry. As Euclidean geometry lies at the intersection of metric geometry and affine geometry, non-Euclidean geometry arises by either replacing the parallel postulate with an alternative, or relaxing the metric requirement. In the former case, one obtains hyperbolic geometry and elliptic geometry, the traditional non-Euclidean geometries. When the metric requirement is relaxed, then there are affine planes associated with the planar algebras, which give rise to kinematic geometries that have also been called non-Euclidean geometry. The essential difference between the metric geometries is the nature of parallel lines. Euclid's fifth postulate, the parallel postulate, is equivalent to Playfair's postulate, which states that, within a two-dimensional plane, for any given line and a point ''A'', which is not on , there is exactly one line through ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Maurice Fréchet
Maurice may refer to: People *Saint Maurice (died 287), Roman legionary and Christian martyr *Maurice (emperor) or Flavius Mauricius Tiberius Augustus (539–602), Byzantine emperor *Maurice (bishop of London) (died 1107), Lord Chancellor and Lord Keeper of England *Maurice of Carnoet (1117–1191), Breton abbot and saint * Maurice, Count of Oldenburg (fl. 1169–1211) *Maurice of Inchaffray (14th century), Scottish cleric who became a bishop *Maurice, Elector of Saxony (1521–1553), German Saxon nobleman *Maurice, Duke of Saxe-Lauenburg (1551–1612) *Maurice of Nassau, Prince of Orange (1567–1625), stadtholder of the Netherlands *Maurice, Landgrave of Hesse-Kassel or Maurice the Learned (1572–1632) *Maurice of Savoy (1593–1657), prince of Savoy and a cardinal *Maurice, Duke of Saxe-Zeitz (1619–1681) *Maurice of the Palatinate (1620–1652), Count Palatine of the Rhine *Maurice of the Netherlands (1843–1850), prince of Orange-Nassau * Maurice Chevalier (1888–1972), F ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Lebesgue Integration
In mathematics, the integral of a non-negative function of a single variable can be regarded, in the simplest case, as the area between the graph of that function and the -axis. The Lebesgue integral, named after French mathematician Henri Lebesgue, extends the integral to a larger class of functions. It also extends the domains on which these functions can be defined. Long before the 20th century, mathematicians already understood that for non-negative functions with a smooth enough graph—such as continuous functions on closed bounded intervals—the ''area under the curve'' could be defined as the integral, and computed using approximation techniques on the region by polygons. However, as the need to consider more irregular functions arose—e.g., as a result of the limiting processes of mathematical analysis and the mathematical theory of probability—it became clear that more careful approximation techniques were needed to define a suitable integral. Also, one might ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Real Analysis
In mathematics, the branch of real analysis studies the behavior of real numbers, sequences and series of real numbers, and real functions. Some particular properties of real-valued sequences and functions that real analysis studies include convergence, limits, continuity, smoothness, differentiability and integrability. Real analysis is distinguished from complex analysis, which deals with the study of complex numbers and their functions. Scope Construction of the real numbers The theorems of real analysis rely on the properties of the real number system, which must be established. The real number system consists of an uncountable set (\mathbb), together with two binary operations denoted and , and an order denoted . The operations make the real numbers a field, and, along with the order, an ordered field. The real number system is the unique ''complete ordered field'', in the sense that any other complete ordered field is isomorphic to it. Intuitively, completeness means ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
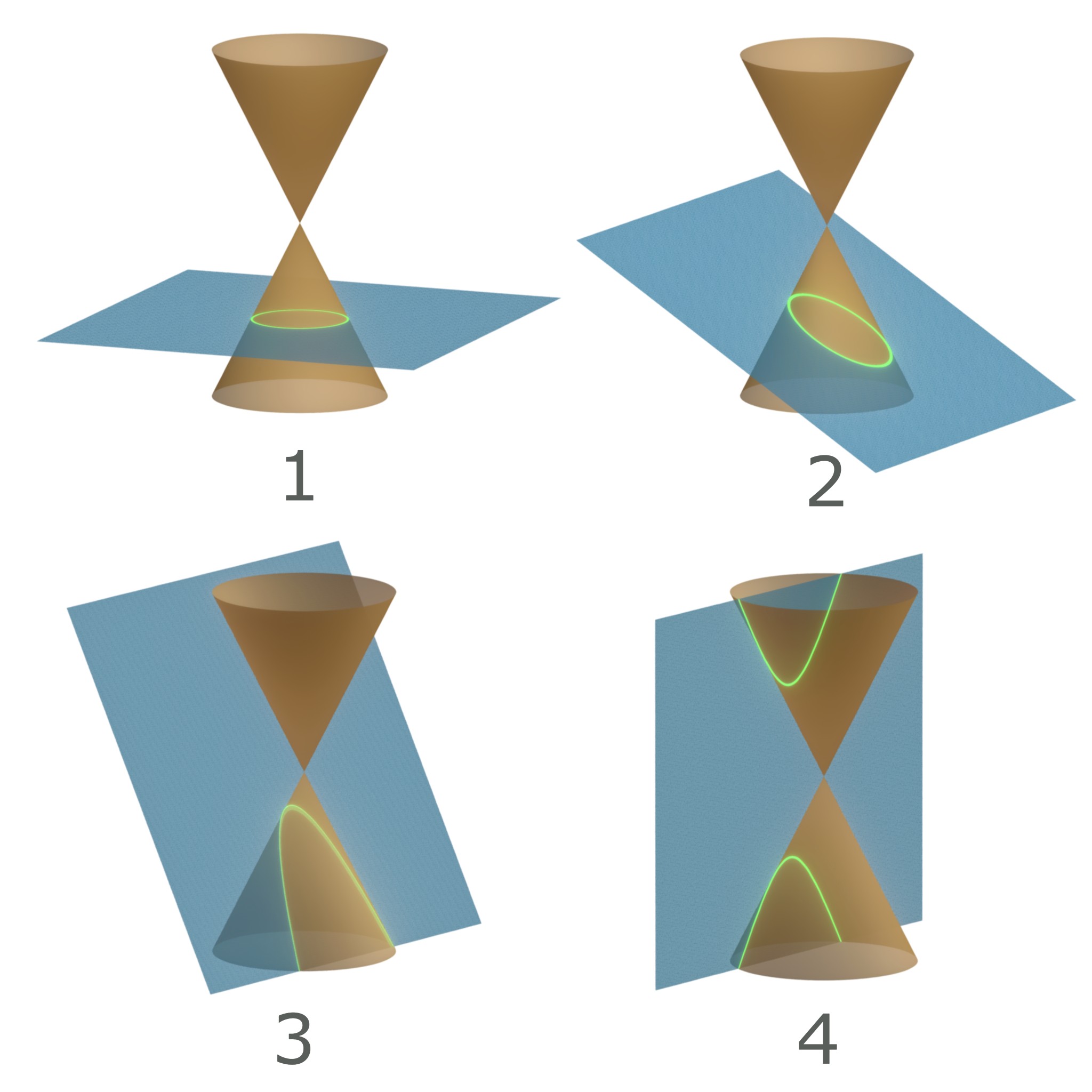
Conic Section
In mathematics, a conic section, quadratic curve or conic is a curve obtained as the intersection of the surface of a cone with a plane. The three types of conic section are the hyperbola, the parabola, and the ellipse; the circle is a special case of the ellipse, though historically it was sometimes called a fourth type. The ancient Greek mathematicians studied conic sections, culminating around 200 BC with Apollonius of Perga's systematic work on their properties. The conic sections in the Euclidean plane have various distinguishing properties, many of which can be used as alternative definitions. One such property defines a non-circular conic to be the set of those points whose distances to some particular point, called a ''focus'', and some particular line, called a ''directrix'', are in a fixed ratio, called the ''eccentricity''. The type of conic is determined by the value of the eccentricity. In analytic geometry, a conic may be defined as a plane algebraic curve of ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |