|
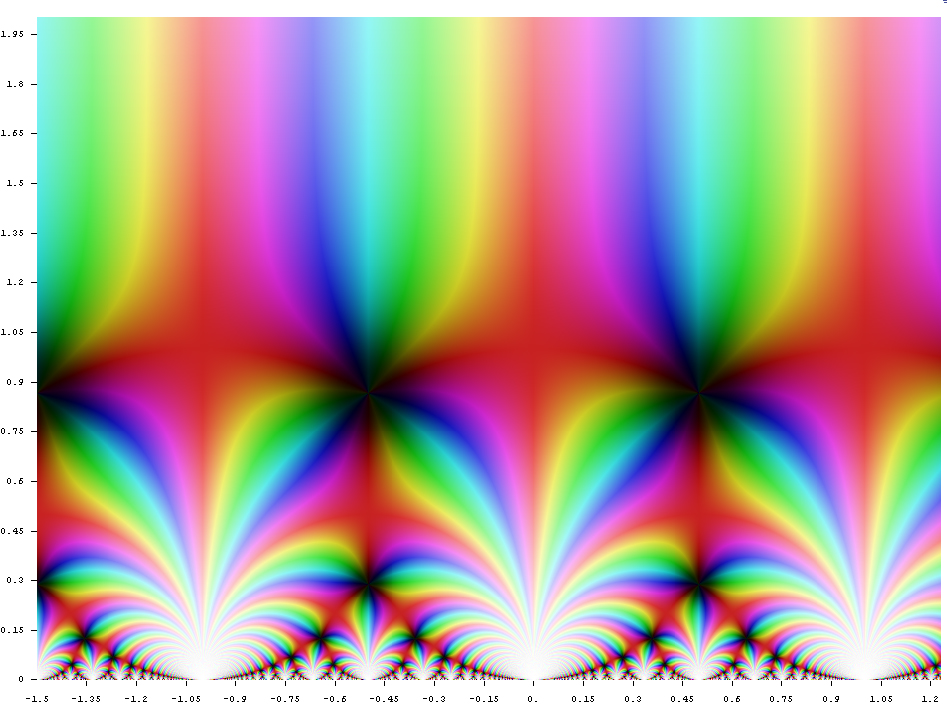
J-Line Pump
In the study of the arithmetic of elliptic curves, the ''j''-line over a ring (mathematics), ring ''R'' is the coarse moduli scheme attached to the moduli problem sending a ring R to the set of isomorphism classes of elliptic curves over R. Since elliptic curves over the complex numbers are isomorphic (over an algebraic closure) if and only if their j-invariants agree, the affine space \mathbb^1_j parameterizing j-invariant, j-invariants of elliptic curves yields a coarse moduli space. However, this fails to be a fine moduli space due to the presence of elliptic curves with automorphisms, necessitating the construction of the Moduli stack of elliptic curves. This is related to the congruence subgroup \Gamma(1) in the following way: : M([\Gamma(1)]) = \mathrm(R[j]) Here the ''j''-invariant is normalized such that j=0 has complex multiplication by \mathbb[\zeta_3], and j=1728 has complex multiplication by \mathbb[i]. The ''j''-line can be seen as giving a coordinatization of the ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Elliptic Curves
In mathematics, an elliptic curve is a smooth, projective, algebraic curve of genus one, on which there is a specified point . An elliptic curve is defined over a field and describes points in , the Cartesian product of with itself. If the field's characteristic is different from 2 and 3, then the curve can be described as a plane algebraic curve which consists of solutions for: :y^2 = x^3 + ax + b for some coefficients and in . The curve is required to be non-singular, which means that the curve has no cusps or self-intersections. (This is equivalent to the condition , that is, being square-free in .) It is always understood that the curve is really sitting in the projective plane, with the point being the unique point at infinity. Many sources define an elliptic curve to be simply a curve given by an equation of this form. (When the coefficient field has characteristic 2 or 3, the above equation is not quite general enough to include all non-singular cubic curve ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Ring (mathematics)
In mathematics, rings are algebraic structures that generalize fields: multiplication need not be commutative and multiplicative inverses need not exist. In other words, a ''ring'' is a set equipped with two binary operations satisfying properties analogous to those of addition and multiplication of integers. Ring elements may be numbers such as integers or complex numbers, but they may also be non-numerical objects such as polynomials, square matrices, functions, and power series. Formally, a ''ring'' is an abelian group whose operation is called ''addition'', with a second binary operation called ''multiplication'' that is associative, is distributive over the addition operation, and has a multiplicative identity element. (Some authors use the term " " with a missing i to refer to the more general structure that omits this last requirement; see .) Whether a ring is commutative (that is, whether the order in which two elements are multiplied might change the result) has ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Moduli Scheme
In mathematics, a moduli scheme is a moduli space that exists in the category of schemes developed by Alexander Grothendieck. Some important moduli problems of algebraic geometry can be satisfactorily solved by means of scheme theory alone, while others require some extension of the 'geometric object' concept (algebraic spaces, algebraic stacks of Michael Artin). History Work of Grothendieck and David Mumford (see geometric invariant theory) opened up this area in the early 1960s. The more algebraic and abstract approach to moduli problems is to set them up as a representable functor question, then apply a criterion that singles out the representable functors for schemes. When this programmatic approach works, the result is a ''fine moduli scheme''. Under the influence of more geometric ideas, it suffices to find a scheme that gives the correct geometric points. This is more like the classical idea that the moduli problem is to express the algebraic structure naturally coming with a ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
J-invariant
In mathematics, Felix Klein's -invariant or function, regarded as a function of a Complex analysis, complex variable , is a modular function of weight zero for defined on the upper half-plane of complex numbers. It is the unique such function which is Holomorphic function, holomorphic away from a simple pole at the Cusp (singularity), cusp such that :j\left(e^\right) = 0, \quad j(i) = 1728 = 12^3. Rational functions of are modular, and in fact give all modular functions. Classically, the -invariant was studied as a parameterization of elliptic curves over , but it also has surprising connections to the symmetries of the Monster group (this connection is referred to as monstrous moonshine). Definition The -invariant can be defined as a function on the upper half-plane :j(\tau) = 1728 \frac = 1728 \frac = 1728 \frac with the third definition implying j(\tau) can be expressed as a Cube (algebra), cube, also since 1728 (number), 1728 = 12^3. The given functions are ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Coarse Moduli Space
In mathematics, a moduli scheme is a moduli space that exists in the category of schemes developed by Alexander Grothendieck. Some important moduli problems of algebraic geometry can be satisfactorily solved by means of scheme theory alone, while others require some extension of the 'geometric object' concept (algebraic spaces, algebraic stacks of Michael Artin). History Work of Grothendieck and David Mumford (see geometric invariant theory) opened up this area in the early 1960s. The more algebraic and abstract approach to moduli problems is to set them up as a representable functor question, then apply a criterion that singles out the representable functors for schemes. When this programmatic approach works, the result is a ''fine moduli scheme''. Under the influence of more geometric ideas, it suffices to find a scheme that gives the correct geometric points. This is more like the classical idea that the moduli problem is to express the algebraic structure naturally coming w ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Fine Moduli Space
In mathematics, in particular algebraic geometry, a moduli space is a geometric space (usually a scheme or an algebraic stack) whose points represent algebro-geometric objects of some fixed kind, or isomorphism classes of such objects. Such spaces frequently arise as solutions to classification problems: If one can show that a collection of interesting objects (e.g., the smooth algebraic curves of a fixed genus) can be given the structure of a geometric space, then one can parametrize such objects by introducing coordinates on the resulting space. In this context, the term "modulus" is used synonymously with "parameter"; moduli spaces were first understood as spaces of parameters rather than as spaces of objects. A variant of moduli spaces is formal moduli. Motivation Moduli spaces are spaces of solutions of geometric classification problems. That is, the points of a moduli space correspond to solutions of geometric problems. Here different solutions are identified if they ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Moduli Stack Of Elliptic Curves
In mathematics, the moduli stack of elliptic curves, denoted as \mathcal_ or \mathcal_, is an algebraic stack over \text(\mathbb) classifying elliptic curves. Note that it is a special case of the moduli stack of algebraic curves \mathcal_. In particular its points with values in some field correspond to elliptic curves over the field, and more generally morphisms from a scheme S to it correspond to elliptic curves over S. The construction of this space spans over a century because of the various generalizations of elliptic curves as the field has developed. All of these generalizations are contained in \mathcal_. Properties Smooth Deligne-Mumford stack The moduli stack of elliptic curves is a smooth separated Deligne–Mumford stack of finite type over \text(\mathbb), but is not a scheme as elliptic curves have non-trivial automorphisms. j-invariant There is a proper morphism of \mathcal_ to the affine line, the coarse moduli space of elliptic curves, given by the ''j''-inv ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Congruence Subgroup
In mathematics, a congruence subgroup of a matrix group with integer entries is a subgroup defined by congruence conditions on the entries. A very simple example would be invertible matrix, invertible 2 × 2 integer matrices of determinant 1, in which the off-diagonal entries are ''even''. More generally, the notion of congruence subgroup can be defined for arithmetic subgroups of algebraic groups; that is, those for which we have a notion of 'integral structure' and can define reduction maps modulo an integer. The existence of congruence subgroups in an arithmetic group provides it with a wealth of subgroups, in particular it shows that the group is residually finite. An important question regarding the algebraic structure of arithmetic groups is the congruence subgroup problem, which asks whether all subgroups of finite Index of a subgroup, index are essentially congruence subgroups. Congruence subgroups of 2×2 matrices are fundamental objects in the classical the ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Complex Multiplication
In mathematics, complex multiplication (CM) is the theory of elliptic curves ''E'' that have an endomorphism ring larger than the integers. Put another way, it contains the theory of elliptic functions with extra symmetries, such as are visible when the period lattice is the Gaussian integer lattice or Eisenstein integer lattice. It has an aspect belonging to the theory of special functions, because such elliptic functions, or abelian functions of several complex variables, are then 'very special' functions satisfying extra identities and taking explicitly calculable special values at particular points. It has also turned out to be a central theme in algebraic number theory, allowing some features of the theory of cyclotomic fields to be carried over to wider areas of application. David Hilbert is said to have remarked that the theory of complex multiplication of elliptic curves was not only the most beautiful part of mathematics but of all science. There is also the higher-dime ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Classical Modular Curve
In number theory, the classical modular curve is an irreducible plane algebraic curve given by an equation :, such that is a point on the curve. Here denotes the -invariant. The curve is sometimes called , though often that notation is used for the abstract algebraic curve for which there exist various models. A related object is the classical modular polynomial, a polynomial in one variable defined as . It is important to note that the classical modular curves are part of the larger theory of modular curves. In particular it has another expression as a compactified quotient of the complex upper half-plane . Geometry of the modular curve The classical modular curve, which we will call , is of degree greater than or equal to when , with equality if and only if is a prime. The polynomial has integer coefficients, and hence is defined over every field. However, the coefficients are sufficiently large that computational work with the curve can be difficult. As a polynomial ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Complex Projective Space
In mathematics, complex projective space is the projective space with respect to the field of complex numbers. By analogy, whereas the points of a real projective space label the lines through the origin of a real Euclidean space, the points of a complex projective space label the ''complex'' lines through the origin of a complex Euclidean space (see below for an intuitive account). Formally, a complex projective space is the space of complex lines through the origin of an (''n''+1)-dimensional complex vector space. The space is denoted variously as P(C''n''+1), P''n''(C) or CP''n''. When , the complex projective space CP1 is the Riemann sphere, and when , CP2 is the complex projective plane (see there for a more elementary discussion). Complex projective space was first introduced by as an instance of what was then known as the "geometry of position", a notion originally due to Lazare Carnot, a kind of synthetic geometry that included other projective geometries as well. Sub ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Projective Line
In mathematics, a projective line is, roughly speaking, the extension of a usual line by a point called a ''point at infinity''. The statement and the proof of many theorems of geometry are simplified by the resultant elimination of special cases; for example, two distinct projective lines in a projective plane meet in exactly one point (there is no "parallel" case). There are many equivalent ways to formally define a projective line; one of the most common is to define a projective line over a field ''K'', commonly denoted P1(''K''), as the set of one-dimensional subspaces of a two-dimensional ''K''-vector space. This definition is a special instance of the general definition of a projective space. The projective line over the reals is a manifold; see real projective line for details. Homogeneous coordinates An arbitrary point in the projective line P1(''K'') may be represented by an equivalence class of ''homogeneous coordinates'', which take the form of a pair : _1 : x_2/mat ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |