|
Ibn Mu'adh Al-Jayyani
Abū ʿAbd Allāh Muḥammad ibn Muʿādh al-Jayyānī ( ar, أبو عبد الله محمد بن معاذ الجياني; 989, Cordova, Al-Andalus – 1079, Jaén, Al-Andalus) was an Arab, mathematician, Islamic scholar, and Qadi from Al-Andalus (in present-day Spain). Al-Jayyānī wrote important commentaries on Euclid's '' Elements'' and he wrote the first known treatise on spherical trigonometry. Life Little is known about his life. Confusion exists over the identity of ''al-Jayyānī'' of the same name mentioned by ibn Bashkuwal (died 1183), Qur'anic scholar, Arabic Philologist, and expert in inheritance laws (farāʾiḍī). It is unknown whether they are the same person. ''The book of unknown arcs of a sphere'' Al-Jayyānī wrote ''The book of unknown arcs of a sphere'', which is considered "the first treatise on spherical trigonometry", although spherical trigonometry in its ancient Hellenistic form was dealt with by earlier mathematicians such as Menelaus of Alexa ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Córdoba, Spain
Córdoba (; ),, Arabic: قُرطبة DIN 31635, DIN: . or Cordova () in English, is a city in Andalusia, Spain, and the capital of the Province of Córdoba (Spain), province of Córdoba. It is the third most populated Municipalities in Spain, municipality in Andalusia and the 11th overall in the country. The city primarily lies on the right bank of the Guadalquivir, in the south of the Iberian Peninsula. Once a Roman settlement, it was taken over by the Visigothic Kingdom, Visigoths, followed by the Umayyad conquest of Hispania, Muslim conquests in the eighth century and later becoming the capital of the Umayyad Caliphate of Córdoba. During these Islamic Golden Age, Muslim periods, Córdoba was transformed into a world leading center of education and learning, producing figures such as Maimonides, Averroes, Ibn Hazm, and Al-Zahrawi, and by the 10th century it had grown to be the second-largest city in Europe. Following the Siege of Córdoba (1236), Christian conquest in 1236, it ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Islamic Inheritance
Islamic Inheritance jurisprudence is a field of Islamic jurisprudence ( ar, فقه) that deals with inheritance, a topic that is prominently dealt with in the Qur'an. It is often called ''Mīrāth'', and its branch of Islamic law is technically known as ''ʿilm al-farāʾiḍ'' ( ar, علم الفرائض, "the science of the ordained quotas"). Inheritance and the Qur'an The Qur'an introduced a number of different rights and restrictions on matters of inheritance, including what were at that time general improvements to the treatment of women and family life. The Qur'an also presented efforts to fix the laws of inheritance, and thus forming a complete legal system. This development was in contrast to pre-Islamic societies where rules of inheritance varied considerably. They do, however, also differ from ongoing secular egalitarian improvements since that time, up to, though principally in, the modern era. Furthermore, the Qur'an introduced additional heirs that were not ent ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Dictionary Of Scientific Biography
The ''Dictionary of Scientific Biography'' is a scholarly reference work that was published from 1970 through 1980 by publisher Charles Scribner's Sons, with main editor the science historian Charles Gillispie, from Princeton University. It consisted of sixteen volumes. It is supplemented by the ''New Dictionary of Scientific Biography''. Both these publications are included in a later electronic book, called the ''Complete Dictionary of Scientific Biography''. ''Dictionary of Scientific Biography'' The ''Dictionary of Scientific Biography'' is a scholarly English-language reference work consisting of biographies of scientists from antiquity to modern times, but excluding scientists who were alive when the ''Dictionary'' was first published. It includes scientists who worked in the areas of mathematics, physics, chemistry, biology, and earth sciences. The work is notable for being one of the most substantial reference works in the field of history of science, containing extens ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
List Of Arab Scientists And Scholars
This is a list of Arab scientists and scholars from the Muslim World, including Al-Andalus (Spain), who lived from antiquity up until the beginning of the modern age, consisting primarily of scholars during the Middle Ages. For a list of contemporary Arab scientists and engineers see List of modern Arab scientists and engineers Both the Arabic and Latin names are given. The following Arabic naming articles are not used for indexing: :*''Al'' - the :* ''Ibn'', ''bin'', ''banu'' - son of :* ''abu, abi'' - father of, the one with A *Ali (601, Mecca – 661, Kufa ), Arabic grammarian, rhetoric, theologian, exegesis and mystic * Aisha (613, Mecca – 678, Medina), Islamic scholar, hadith narrator, her intellect and knowledge in various subjects, including poetry and medicine. *Abbas Ibn Firnas, astronomer, mathematician, physicist, inventor * Aisha al-Bauniyya (1402–1475), an Arab woman Sufi master and poet *Avempace (1085, Zaragoza – 1138, Fez), philosopher, astronomer ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Regiomontanus
Johannes Müller von Königsberg (6 June 1436 – 6 July 1476), better known as Regiomontanus (), was a mathematician, astrologer and astronomer of the German Renaissance, active in Vienna, Buda and Nuremberg. His contributions were instrumental in the development of Copernican heliocentrism in the decades following his death. Regiomontanus wrote under the Latinized name of ''Ioannes de Monteregio'' (or ''Monte Regio''; ''Regio Monte''); the toponym ''Regiomontanus'' was first used by Philipp Melanchthon in 1534. He is named after Königsberg, Bavaria, Königsberg in Lower Franconia, not the larger Königsberg (modern Kaliningrad) in Prussia. Life Although little is known of Regiomontanus' early life, it is believed that at eleven years of age, he became a student at the University of Leipzig, Electorate of Saxony, Saxony. In 1451 he continued his studies at University of Vienna, Alma Mater Rudolfina, the university in Vienna, Duchy of Austria, Austria. There he became a pupil ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Ratio
In mathematics, a ratio shows how many times one number contains another. For example, if there are eight oranges and six lemons in a bowl of fruit, then the ratio of oranges to lemons is eight to six (that is, 8:6, which is equivalent to the ratio 4:3). Similarly, the ratio of lemons to oranges is 6:8 (or 3:4) and the ratio of oranges to the total amount of fruit is 8:14 (or 4:7). The numbers in a ratio may be quantities of any kind, such as counts of people or objects, or such as measurements of lengths, weights, time, etc. In most contexts, both numbers are restricted to be Positive integer, positive. A ratio may be specified either by giving both constituting numbers, written as "''a'' to ''b''" or "''a'':''b''", or by giving just the value of their quotient Equal quotients correspond to equal ratios. Consequently, a ratio may be considered as an ordered pair of numbers, a Fraction (mathematics), fraction with the first number in the numerator and the second in the denom ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Triangle
A triangle is a polygon with three Edge (geometry), edges and three Vertex (geometry), vertices. It is one of the basic shapes in geometry. A triangle with vertices ''A'', ''B'', and ''C'' is denoted \triangle ABC. In Euclidean geometry, any three points, when non-Collinearity, collinear, determine a unique triangle and simultaneously, a unique Plane (mathematics), plane (i.e. a two-dimensional Euclidean space). In other words, there is only one plane that contains that triangle, and every triangle is contained in some plane. If the entire geometry is only the Euclidean plane, there is only one plane and all triangles are contained in it; however, in higher-dimensional Euclidean spaces, this is no longer true. This article is about triangles in Euclidean geometry, and in particular, the Euclidean plane, except where otherwise noted. Types of triangle The terminology for categorizing triangles is more than two thousand years old, having been defined on the very first page of ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
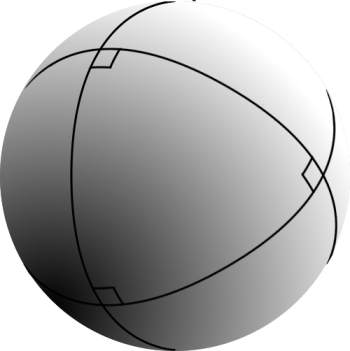
Spherical Triangle
Spherical trigonometry is the branch of spherical geometry that deals with the metrical relationships between the sides and angles of spherical triangles, traditionally expressed using trigonometric functions. On the sphere, geodesics are great circles. Spherical trigonometry is of great importance for calculations in astronomy, geodesy, and navigation. The origins of spherical trigonometry in Greek mathematics and the major developments in Islamic mathematics are discussed fully in History of trigonometry and Mathematics in medieval Islam. The subject came to fruition in Early Modern times with important developments by John Napier, Delambre and others, and attained an essentially complete form by the end of the nineteenth century with the publication of Todhunter's textbook ''Spherical trigonometry for the use of colleges and Schools''. Since then, significant developments have been the application of vector methods, quaternion methods, and the use of numerical methods. Pr ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Law Of Sines
In trigonometry, the law of sines, sine law, sine formula, or sine rule is an equation relating the lengths of the sides of any triangle to the sines of its angles. According to the law, \frac \,=\, \frac \,=\, \frac \,=\, 2R, where , and are the lengths of the sides of a triangle, and , and are the opposite angles (see figure 2), while is the radius of the triangle's circumcircle. When the last part of the equation is not used, the law is sometimes stated using the reciprocals; \frac \,=\, \frac \,=\, \frac. The law of sines can be used to compute the remaining sides of a triangle when two angles and a side are known—a technique known as triangulation. It can also be used when two sides and one of the non-enclosed angles are known. In some such cases, the triangle is not uniquely determined by this data (called the ''ambiguous case'') and the technique gives two possible values for the enclosed angle. The law of sines is one of two trigonometric equations commonly a ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Special Right Triangles
A special right triangle is a right triangle with some regular feature that makes calculations on the triangle easier, or for which simple formulas exist. For example, a right triangle may have angles that form simple relationships, such as 45°–45°–90°. This is called an "angle-based" right triangle. A "side-based" right triangle is one in which the lengths of the sides form ratios of whole numbers, such as 3 : 4 : 5, or of other special numbers such as the golden ratio. Knowing the relationships of the angles or ratios of sides of these special right triangles allows one to quickly calculate various lengths in geometric problems without resorting to more advanced methods. Angle-based "Angle-based" special right triangles are specified by the relationships of the angles of which the triangle is composed. The angles of these triangles are such that the larger (right) angle, which is 90 degrees or radians, is equal to the sum of the other two angles ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Routledge
Routledge () is a British multinational publisher. It was founded in 1836 by George Routledge, and specialises in providing academic books, journals and online resources in the fields of the humanities, behavioural science, education, law, and social science. The company publishes approximately 1,800 journals and 5,000 new books each year and their backlist encompasses over 70,000 titles. Routledge is claimed to be the largest global academic publisher within humanities and social sciences. In 1998, Routledge became a subdivision and imprint of its former rival, Taylor & Francis Group (T&F), as a result of a £90-million acquisition deal from Cinven, a venture capital group which had purchased it two years previously for £25 million. Following the merger of Informa and T&F in 2004, Routledge became a publishing unit and major imprint within the Informa "academic publishing" division. Routledge is headquartered in the main T&F office in Milton Park, Abingdon, Oxfordshire and ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Seyyed Hossein Nasr
Seyyed Hossein Nasr (; fa, سید حسین نصر, born April 7, 1933) is an Iranian philosopher and University Professor of Islamic studies at George Washington University. Born in Tehran, Nasr completed his education in Iran and the United States, earning a bachelor's degree in physics from Massachusetts Institute of Technology, a master's in geology and geophysics, and a doctorate in the history of science from Harvard University. He returned to his homeland in 1958, turning down teaching positions at MIT and Harvard, and was appointed a professor of philosophy and Islamic sciences at Tehran University. He held various academic positions in Iran, including vice-chancellor at Tehran University and President of Sharif University of Technology, Aryamehr University, and established the Iranian Research Institute of Philosophy, Imperial Iranian Academy of Philosophy at the request of Empress Farah Pahlavi, which soon became one of the most prominent centers of philosophical activit ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |