|
Interval Class Vector
In musical set theory, an interval vector is an array of natural numbers which summarize the intervals present in a set of pitch classes. (That is, a set of pitches where octaves are disregarded.) Other names include: ic vector (or interval-class vector), PIC vector (or pitch-class interval vector) and APIC vector (or absolute pitch-class interval vector, which Michiel Schuijer states is more proper.) While primarily an analytic tool, interval vectors can also be useful for composers, as they quickly show the sound qualities that are created by different collections of pitch class. That is, sets with high concentrations of conventionally dissonant intervals (i.e., seconds and sevenths) sound more dissonant, while sets with higher numbers of conventionally consonant intervals (i.e., thirds and sixths) sound more consonant. While the actual perception of consonance and dissonance involves many contextual factors, such as register, an interval vector can nevertheless be a help ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
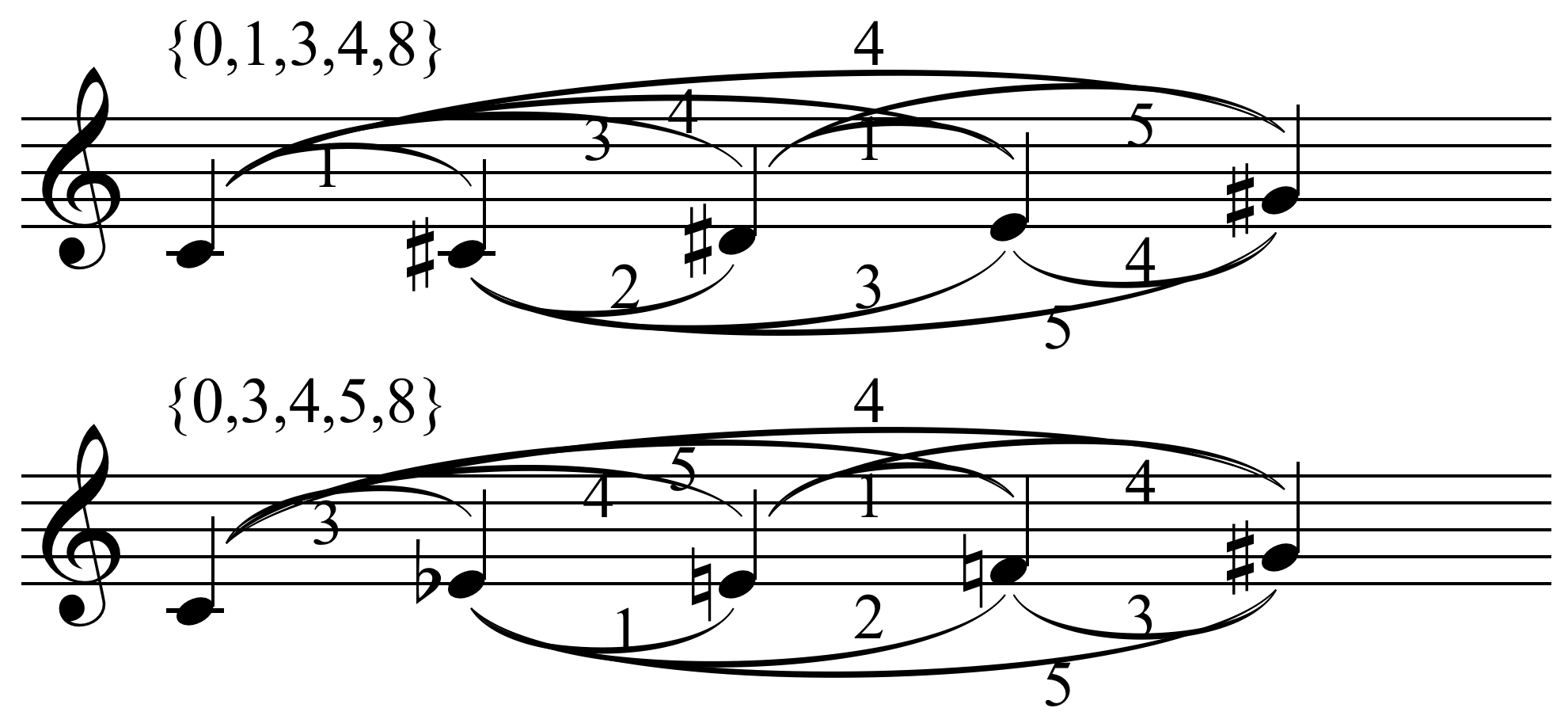
Z-relation Z17 Example
In Set theory (music), musical set theory, an interval vector is an array of natural numbers which summarize the Interval (music), intervals present in a Set (music), set of pitch classes. (That is, a set of Pitch (music), pitches where octaves are disregarded.) Other names include: ic vector (or interval-class vector), PIC vector (or pitch-class interval vector) and APIC vector (or absolute pitch-class interval vector, which Michiel Schuijer states is more proper.) While primarily an analytic tool, interval vectors can also be useful for composers, as they quickly show the sound qualities that are created by different collections of pitch class. That is, sets with high concentrations of conventionally dissonant intervals (i.e., seconds and sevenths) sound more dissonant, while sets with higher numbers of conventionally consonant intervals (i.e., thirds and sixths) sound more consonance and dissonance, consonant. While the actual perception of consonance and dissonance involv ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Inversional Equivalency
In music theory, an inversion is a type of change to Interval (music), intervals, Chord (music), chords, Part (music), voices (in counterpoint), and Melody, melodies. In each of these cases, "inversion" has a distinct but related meaning. The concept of inversion also plays an important role in set theory (music), musical set theory. Intervals An interval (music), interval is inverted by raising or lowering either of the notes by one or more octaves so that the positions of the notes reverse (i.e. the higher note becomes the lower note and vice versa). For example, the inversion of an interval consisting of a C with an E above it (the third measure below) is an E with a C above it – to work this out, the C may be moved up, the E may be lowered, or both may be moved. : The tables to the right show the changes in interval quality and interval number under inversion. Thus, perfect intervals remain perfect, major intervals become minor and vice versa, and augmented inter ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
David Lewin
David Benjamin Lewin (July 2, 1933 – May 5, 2003) was an American music theorist, music critic and composer. Called "the most original and far-ranging theorist of his generation", he did his most influential theoretical work on the development of transformational theory, which involves the application of mathematical group theory to music. Biography Lewin was born in New York City and studied piano from a young age and was for a time a pupil of Eduard Steuermann. He graduated from Harvard in 1954 with a degree in mathematics. Lewin then studied theory and composition with Roger Sessions, Milton Babbitt, Edward T. Cone, and Earl Kim at Princeton University, earning an M.F.A. in 1958. He returned to Harvard as a Junior Fellow in the Harvard Society of Fellows from 1958 to 1961. After holding teaching positions at the University of California, Berkeley (1961–67), the State University of New York at Stony Brook (1967–79), and Yale University (1979–85), he returned to Harvard as ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Transformation Theory (music)
Transformational theory is a branch of music theory developed by David Lewin in the 1980s, and formally introduced in his 1987 work, ''Generalized Musical Intervals and Transformations''. The theory—which models Transformation (music), musical transformations as elements of a Group theory, mathematical group—can be used to analyze both tonality, tonal and atonal music. The goal of transformational theory is to change the focus from musical objects—such as the "C major chord" or "G major chord"—to relations between musical objects (related by transformation). Thus, instead of saying that a C major chord is followed by G major, a transformational theorist might say that the first chord has been "transformed" into the second by the "dominant (music), Dominant operation." (Symbolically, one might write "Dominant(C major) = G major.") While traditional set theory (music), musical set theory focuses on the makeup of musical objects, transformational theory focuses on the interva ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Triangular Number
A triangular number or triangle number counts objects arranged in an equilateral triangle. Triangular numbers are a type of figurate number, other examples being square numbers and cube numbers. The th triangular number is the number of dots in the triangular arrangement with dots on each side, and is equal to the sum of the natural numbers from 1 to . The sequence of triangular numbers, starting with the 0th triangular number, is (This sequence is included in the On-Line Encyclopedia of Integer Sequences .) Formula The triangular numbers are given by the following explicit formulas: T_n= \sum_^n k = 1+2+3+ \dotsb +n = \frac = , where \textstyle is a binomial coefficient. It represents the number of distinct pairs that can be selected from objects, and it is read aloud as " plus one choose two". The first equation can be illustrated using a visual proof. For every triangular number T_n, imagine a "half-square" arrangement of objects corresponding to the triangular numb ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Z-relation
In musical set theory, an interval vector is an array of natural numbers which summarize the intervals present in a set of pitch classes. (That is, a set of pitches where octaves are disregarded.) Other names include: ic vector (or interval-class vector), PIC vector (or pitch-class interval vector) and APIC vector (or absolute pitch-class interval vector, which Michiel Schuijer states is more proper.) While primarily an analytic tool, interval vectors can also be useful for composers, as they quickly show the sound qualities that are created by different collections of pitch class. That is, sets with high concentrations of conventionally dissonant intervals (i.e., seconds and sevenths) sound more dissonant, while sets with higher numbers of conventionally consonant intervals (i.e., thirds and sixths) sound more consonant. While the actual perception of consonance and dissonance involves many contextual factors, such as register, an interval vector can nevertheless be a help ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Set Class
A set (pitch set, pitch-class set, set class, set form, set genus, pitch collection) in music theory, as in mathematics and general parlance, is a collection of objects. In musical contexts the term is traditionally applied most often to collections of pitches or pitch-classes, but theorists have extended its use to other types of musical entities, so that one may speak of sets of durations or timbres, for example.Wittlich, Gary (1975). "Sets and Ordering Procedures in Twentieth-Century Music", ''Aspects of Twentieth-Century Music'', p.475. Wittlich, Gary (ed.). Englewood Cliffs, New Jersey: Prentice-Hall. . A set by itself does not necessarily possess any additional structure, such as an ordering or permutation. Nevertheless, it is often musically important to consider sets that are equipped with an order relation (called ''segments''); in such contexts, bare sets are often referred to as "unordered", for the sake of emphasis. Two-element sets are called dyads, three-eleme ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
List Of Pitch-class Sets
This is a list of set classes by Forte number. For a list of ordered collections, see: list of tone rows and series. Sets are listed next to their complements. Inversions are marked "B" (sets not marked "A" or "B" are symmetrical). "T" and "E" are conventionally used in sets to notate 10 and 11, respectively, as single characters. There are two slightly different methods of obtaining a normal form. This results in two different normal form sets for the same Forte number in a few cases. The alternative notation for those chords are listed in the footnotes. Elliott Carter had earlier (1960–67) produced a numbered listing of pitch class sets, or "chords", as Carter referred to them, for his own use. Donald Martino had produced tables of hexachords, tetrachords, trichords, and pentachords for combinatoriality in his article, "The Source Set and its Aggregate Formations" (1961).Schuijer, Michael (2008). ''Analyzing Atonal Music: Pitch-Class Set Theory and Its Contexts'', p.97 ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Major Triad
In music theory, a major chord is a chord that has a root, a major third, and a perfect fifth. When a chord comprises only these three notes, it is called a major triad. For example, the major triad built on C, called a C major triad, has pitches C–E–G: In harmonic analysis and on lead sheets, a C major chord can be notated as C, CM, CΔ, or Cmaj. A major triad is represented by the integer notation . A major triad can also be described by its intervals: the interval between the bottom and middle notes is a major third, and the interval between the middle and top notes is a minor third. By contrast, a minor triad has a minor third interval on the bottom and major third interval on top. They both contain fifths, because a major third (four semitones) plus a minor third (three semitones) equals a perfect fifth (seven semitones). Chords that are constructed of consecutive (or "stacked") thirds are called ''tertian.'' In Western classical music from 1600 to 1820 and in W ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Deep Scale Property
In music, a common tone is a pitch class that is a member of, or common to (shared by) two or more scales or sets. Common tone theorem A common tone is a pitch class that is a member of, or common to, a musical scale and a transposition of that scale, as in modulation. Six of seven possible common tones are shared by closely related keys, though keys may also be thought of as more or less closely related according to their number of common tones. "Obviously, tonal distance is in some sense a function of the extent of intersection between diatonic PC collections of tonal systems". In diatonic set theory the common tone theorem explains that scales possessing the deep scale property share a different number of common tones, not counting enharmonic equivalents (for example, C and C have no common tones with C major), for every different transposition of the scale. However many times an interval class occurs in a diatonic scale is the number of tones common both to the origin ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Equal Division Of The Octave
An equal temperament is a musical temperament or tuning system, which approximates just intervals by dividing an octave (or other interval) into equal steps. This means the ratio of the frequencies of any adjacent pair of notes is the same, which gives an equal perceived step size as pitch is perceived roughly as the logarithm of frequency. In classical music and Western music in general, the most common tuning system since the 18th century has been twelve-tone equal temperament (also known as 12 equal temperament, 12-TET or 12-ET; informally abbreviated to twelve equal), which divides the octave into 12 parts, all of which are equal on a logarithmic scale, with a ratio equal to the 12th root of 2 ( ≈ 1.05946). That resulting smallest interval, the width of an octave, is called a semitone or half step. In Western countries the term ''equal temperament'', without qualification, generally means 12-TET. In modern times, 12-TET is usually tuned relative to a standard pitch of ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
.png "Click for more on -> Major Triad")
