|
Inertia Ellipsoid
In classical mechanics, Poinsot's construction (after Louis Poinsot) is a geometrical method for visualizing the torque-free motion of a rotating rigid body, that is, the motion of a rigid body on which no external forces are acting. This motion has four constants: the kinetic energy of the body and the three components of the angular momentum, expressed with respect to an inertial laboratory frame. The angular velocity vector \boldsymbol\omega of the rigid rotor is ''not constant'', but satisfies Euler's equations. Without explicitly solving these equations, Louis Poinsot was able to visualize the motion of the endpoint of the angular velocity vector. To this end he used the conservation of kinetic energy and angular momentum as constraints on the motion of the angular velocity vector \boldsymbol\omega. If the rigid rotor is symmetric (has two equal moments of inertia), the vector \boldsymbol\omega describes a cone (and its endpoint a circle). This is the torque-free precession ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Classical Mechanics
Classical mechanics is a physical theory describing the motion of macroscopic objects, from projectiles to parts of machinery, and astronomical objects, such as spacecraft, planets, stars, and galaxies. For objects governed by classical mechanics, if the present state is known, it is possible to predict how it will move in the future (determinism), and how it has moved in the past (reversibility). The earliest development of classical mechanics is often referred to as Newtonian mechanics. It consists of the physical concepts based on foundational works of Sir Isaac Newton, and the mathematical methods invented by Gottfried Wilhelm Leibniz, Joseph-Louis Lagrange, Leonhard Euler, and other contemporaries, in the 17th century to describe the motion of bodies under the influence of a system of forces. Later, more abstract methods were developed, leading to the reformulations of classical mechanics known as Lagrangian mechanics and Hamiltonian mechanics. These advances, ma ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Herpolhode
A herpolhode is the curve traced out by the endpoint of the angular velocity vector ω of a rigid rotor, a rotating rigid body. The endpoint of the angular velocity moves in a plane in absolute space, called the invariable plane, that is orthogonal to the angular momentum In physics, angular momentum (rarely, moment of momentum or rotational momentum) is the rotational analog of linear momentum. It is an important physical quantity because it is a conserved quantity—the total angular momentum of a closed syst ... vector L. The fact that the herpolhode is a curve in the invariable plane appears as part of Poinsot's construction. The trajectory of the angular velocity around the angular momentum in the invariable plane is a circle in the case of a symmetric top, but in the general case wiggles inside an annulus, while still being concave towards the angular momentum. See also * Poinsot's construction * Polhode References H. Goldstein, ''Classical Mechanics'', Addison-W ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
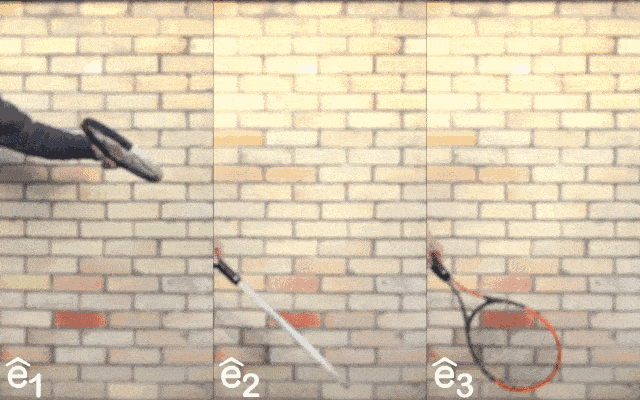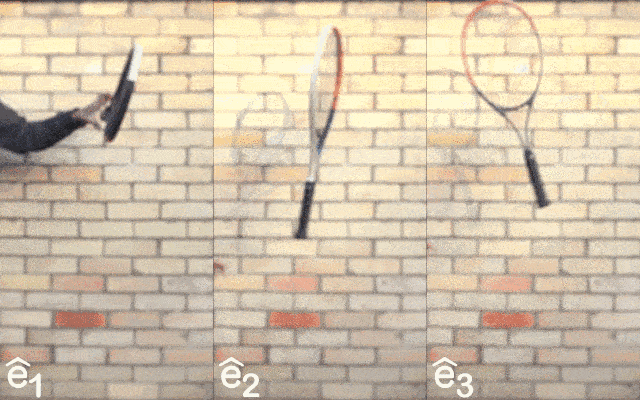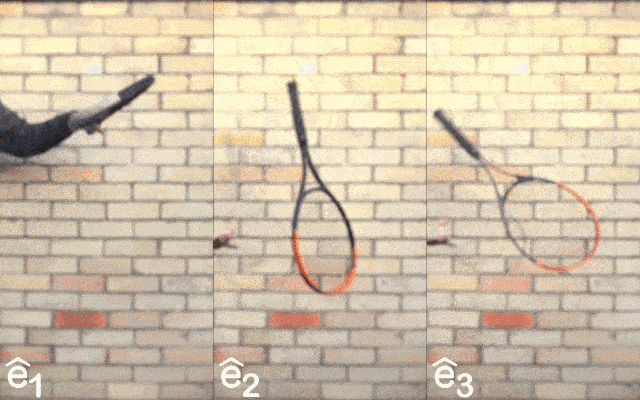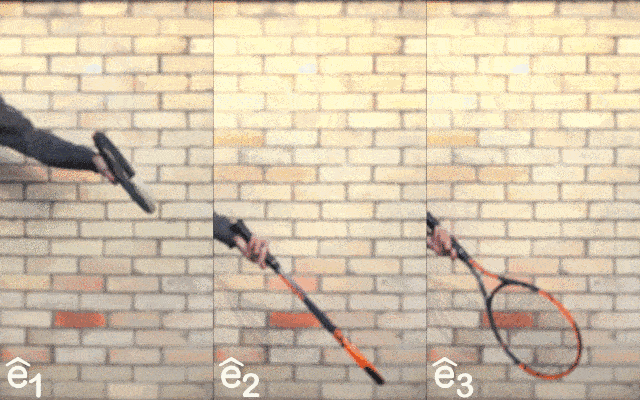
Tennis Racket Theorem
The tennis racket theorem or intermediate axis theorem is a result in classical mechanics describing the movement of a rigid body with three distinct principal moments of inertia. It is also dubbed the Dzhanibekov effect, after Soviet cosmonaut Vladimir Dzhanibekov who noticed one of the theorem's logical consequences while in space in 1985, although the effect was already known for at least 150 years before that and was included in a book by Louis Poinsot in 1834. The theorem describes the following effect: rotation of an object around its first and third principal axes is stable, while rotation around its second principal axis (or intermediate axis) is not. This can be demonstrated with the following experiment: hold a tennis racket at its handle, with its face being horizontal, and try to throw it in the air so that it will perform a full rotation around the horizontal axis perpendicular to the handle, and try to catch the handle. In almost all cases, during that rotation ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Jacques Philippe Marie Binet
Jacques Philippe Marie Binet (; 2 February 1786 – 12 May 1856) was a French mathematician, physicist and astronomer born in Rennes; he died in Paris, France, in 1856. He made significant contributions to number theory, and the mathematical foundations of matrix algebra which would later lead to important contributions by Cayley and others. In his memoir on the theory of the conjugate axis and of the moment of inertia of bodies he enumerated the principle now known as ''Binet's theorem''. He is also recognized as the first to describe the rule for multiplying matrices in 1812, and ''Binet's formula'' expressing Fibonacci numbers in closed form is named in his honour, although the same result was known to Abraham de Moivre a century earlier. Career Binet graduated from l'École Polytechnique in 1806, and returned as a teacher in 1807. He advanced in position until 1816 when he became an inspector of studies at l'École. He held this post until 13 November 1830, when he was dismi ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Tennis Racket Theorem
The tennis racket theorem or intermediate axis theorem is a result in classical mechanics describing the movement of a rigid body with three distinct principal moments of inertia. It is also dubbed the Dzhanibekov effect, after Soviet cosmonaut Vladimir Dzhanibekov who noticed one of the theorem's logical consequences while in space in 1985, although the effect was already known for at least 150 years before that and was included in a book by Louis Poinsot in 1834. The theorem describes the following effect: rotation of an object around its first and third principal axes is stable, while rotation around its second principal axis (or intermediate axis) is not. This can be demonstrated with the following experiment: hold a tennis racket at its handle, with its face being horizontal, and try to throw it in the air so that it will perform a full rotation around the horizontal axis perpendicular to the handle, and try to catch the handle. In almost all cases, during that rotation ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Dzhanibekov Effect
The tennis racket theorem or intermediate axis theorem is a result in classical mechanics describing the movement of a rigid body with three distinct principal moments of inertia. It is also dubbed the Dzhanibekov effect, after Soviet cosmonaut Vladimir Dzhanibekov who noticed one of the theorem's logical consequences while in space in 1985, although the effect was already known for at least 150 years before that and was included in a book by Louis Poinsot in 1834. The theorem describes the following effect: rotation of an object around its first and third principal axes is stable, while rotation around its second principal axis (or intermediate axis) is not. This can be demonstrated with the following experiment: hold a tennis racket at its handle, with its face being horizontal, and try to throw it in the air so that it will perform a full rotation around the horizontal axis perpendicular to the handle, and try to catch the handle. In almost all cases, during that rotation ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
List Of Tumblers (small Solar System Bodies)
This is a list of tumblers, minor planets, comets and natural satellites whose angular momentum vector is far from the principal axis of inertia, so that they do not rotate in a fairly constant manner with a constant period. Instead of rotating around a constant axis or around a wobbling axis, they appear to tumble (see Poinsot's ellipsoid for an explanation). For true tumbling, the three moments of inertia must be different. If two are equal, then the axis of rotation will simply precess in a circle. As of 2018, there are 3 natural satellites and 198 confirmed or likely tumblers out of a total of nearly 800,000 discovered small Solar System bodies. The data is sourced from the "Lightcurve Data Base" (LCDB). The tumbling of a body can be caused by the torque from asymmetrically emitted radiation known as the YORP effect. Note that the rotation periods given below are approximate. The rotation period is not constant for a tumbler. Natural satellites This is a list of tumbling na ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Moons Of Pluto
The dwarf planet Pluto has five natural satellites. In order of distance from Pluto, they are Charon (moon), Charon, Styx (moon), Styx, Nix (moon), Nix, Kerberos (moon), Kerberos, and Hydra (moon), Hydra. Charon, the largest, is mutually tidally locked with Pluto, and is massive enough that Pluto–Charon is sometimes considered a Double planet, double dwarf planet. History The innermost and largest moon, Charon (moon), Charon, was discovered by James Christy on 22 June 1978, nearly half a century after Pluto was discovered. This led to a substantial revision in estimates of Pluto's size, which had previously assumed that the observed mass and reflected light of the system were all attributable to Pluto alone. Two additional moons were imaged by astronomers of the Pluto Companion Search Team preparing for the ''New Horizons'' mission and working with the Hubble Space Telescope on 15 May 2005, which received the Provisional designation in astronomy, provisional designations S/2005 ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Hyperion (moon)
Hyperion , also known as Saturn VII, is a moon of Saturn discovered by William Cranch Bond, his son George Phillips Bond and William Lassell in 1848. It is distinguished by its irregular shape, its chaotic rotation, and its unexplained sponge-like appearance. It was the first non-round moon to be discovered. Name The moon is named after Hyperion, the Titan god of watchfulness and observation – the elder brother of Cronus, the Greek equivalent of the Roman god Saturn. It is also designated ''Saturn VII''. The adjectival form of the name is ''Hyperionian''. Hyperion's discovery came shortly after John Herschel had suggested names for the seven previously known satellites of Saturn in his 1847 publication ''Results of Astronomical Observations made at the Cape of Good Hope''. William Lassell, who saw Hyperion two days after William Bond, had already endorsed Herschel's naming scheme and suggested the name Hyperion in accordance with it. He also beat Bond to pu ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Annulus (mathematics)
In mathematics, an annulus (plural annuli or annuluses) is the region between two concentric circles. Informally, it is shaped like a ring or a hardware washer. The word "annulus" is borrowed from the Latin word ''anulus'' or ''annulus'' meaning 'little ring'. The adjectival form is annular (as in annular eclipse). The open annulus is topologically equivalent to both the open cylinder and the punctured plane. Area The area of an annulus is the difference in the areas of the larger circle of radius and the smaller one of radius : :A = \pi R^2 - \pi r^2 = \pi\left(R^2 - r^2\right). The area of an annulus is determined by the length of the longest line segment within the annulus, which is the chord tangent to the inner circle, in the accompanying diagram. That can be shown using the Pythagorean theorem since this line is tangent to the smaller circle and perpendicular to its radius at that point, so and are sides of a right-angled triangle with hypotenuse , and the ar ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Almost Periodic
In mathematics, an almost periodic function is, loosely speaking, a function of a real number that is periodic function, periodic to within any desired level of accuracy, given suitably long, well-distributed "almost-periods". The concept was first studied by Harald Bohr and later generalized by Vyacheslav Stepanov, Hermann Weyl and Abram Samoilovitch Besicovitch, amongst others. There is also a notion of almost periodic functions on locally compact abelian groups, first studied by John von Neumann. Almost periodicity is a property of dynamical systems that appear to retrace their paths through phase space, but not exactly. An example would be a planetary system, with planets in orbits moving with Orbital period, periods that are not commensurability (mathematics), commensurable (i.e., with a period vector that is not Proportionality (mathematics), proportional to a vector space, vector of integers). A Kronecker's theorem on diophantine approximation, theorem of Kronecker from diop ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
2-manifold
In the part of mathematics referred to as topology, a surface is a two-dimensional manifold. Some surfaces arise as the boundaries of three-dimensional solids; for example, the sphere is the boundary of the solid ball. Other surfaces arise as graphs of functions of two variables; see the figure at right. However, surfaces can also be defined abstractly, without reference to any ambient space. For example, the Klein bottle is a surface that cannot be embedded in three-dimensional Euclidean space. Topological surfaces are sometimes equipped with additional information, such as a Riemannian metric or a complex structure, that connects them to other disciplines within mathematics, such as differential geometry and complex analysis. The various mathematical notions of surface can be used to model surfaces in the physical world. In general In mathematics, a surface is a geometrical shape that resembles a deformed plane. The most familiar examples arise as boundaries of sol ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
