|
Idealized Greenhouse Model
The temperatures of a planet's surface and atmosphere are governed by a delicate balancing of their energy flows. The idealized greenhouse model is based on the fact that certain gases in the Earth's atmosphere, including carbon dioxide and water vapour, are transparent to the high-frequency solar radiation, but are much more opaque to the lower frequency infrared radiation leaving Earth's surface. Thus heat is easily let ''in'', but is partially trapped by these gases as it tries to ''leave''. Rather than get hotter and hotter, Kirchhoff's law of thermal radiation says that the gases of the atmosphere also have to re-emit the infrared energy that they absorb, and they do so, also at long infrared wavelengths, both upwards into space as well as downwards back towards the Earth's surface. In the long-term, the planet's thermal inertia is surmounted and a new thermal equilibrium is reached when all energy arriving on the planet is leaving again at the same rate. In this steady-state ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Zero-dimensional Space
In mathematics, a zero-dimensional topological space (or nildimensional space) is a topological space that has dimension zero with respect to one of several inequivalent notions of assigning a dimension to a given topological space. A graphical illustration of a nildimensional space is a point. Definition Specifically: * A topological space is zero-dimensional with respect to the Lebesgue covering dimension if every open cover of the space has a refinement which is a cover by disjoint open sets. * A topological space is zero-dimensional with respect to the finite-to-finite covering dimension if every finite open cover of the space has a refinement that is a finite open cover such that any point in the space is contained in exactly one open set of this refinement. * A topological space is zero-dimensional with respect to the small inductive dimension if it has a base consisting of clopen sets. The three notions above agree for separable, metrisable spaces. Properties of ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Absorptance
Absorptance of the surface of a material is its effectiveness in absorbing radiant energy. It is the ratio of the absorbed to the incident radiant power. This should not be confused with absorbance and absorption coefficient. Mathematical definitions Hemispherical absorptance Hemispherical absorptance of a surface, denoted ''A'', is defined as :A = \frac, where *Φea is the radiant flux ''absorbed'' by that surface; *Φei is the radiant flux received by that surface. Spectral hemispherical absorptance Spectral hemispherical absorptance in frequency and spectral hemispherical absorptance in wavelength of a surface, denoted ''A''ν and ''A''λ respectively, are defined as :A_\nu = \frac, :A_\lambda = \frac, where *Φe,νa is the spectral radiant flux in frequency ''absorbed'' by that surface; *Φe,νi is the spectral radiant flux in frequency received by that surface; *Φe,λa is the spectral radiant flux in wavelength ''absorbed'' by that surface; *Φe,λi is the spectral radiant f ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Stefan–Boltzmann Constant
The Stefan–Boltzmann constant (also Stefan's constant), a physical constant denoted by the Greek letter ''σ'' (sigma), is the constant of proportionality in the Stefan–Boltzmann law: "the total intensity radiated over all wavelengths increases as the temperature increases", of a black body which is proportional to the fourth power of the thermodynamic temperature. The theory of thermal radiation lays down the theory of quantum mechanics, by using physics to relate to molecular, atomic and sub-atomic levels. Slovenian physicist Josef Stefan formulated the constant in 1879; it was formally derived in 1884 by his former student and collaborator, the Austrian physicist Ludwig Boltzmann. The equation can also be derived from Planck's law, by integrating over all wavelengths at a given temperature, which will represent a small flat black body box. "The amount of thermal radiation emitted increases quickly and the principal frequency of the radiation becomes higher with incr ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Stefan–Boltzmann Law
The Stefan–Boltzmann law describes the power radiated from a black body in terms of its temperature. Specifically, the Stefan–Boltzmann law states that the total energy radiated per unit surface area of a black body across all wavelengths per unit time j^ (also known as the black-body '' radiant emittance'') is directly proportional to the fourth power of the black body's thermodynamic temperature ''T'': : j^ = \sigma T^. The constant of proportionality ''σ'', called the Stefan–Boltzmann constant, is derived from other known physical constants. Since 2019, the value of the constant is : \sigma=\frac = 5.670374419\times 10^\, \mathrm, where ''k'' is the Boltzmann constant, ''h'' is Planck's constant, and ''c'' is the speed of light in a vacuum. The radiance from a specified angle of view (watts per square metre per steradian) is given by : L = \frac\pi = \frac\sigma\pi T^. A body that does not absorb all incident radiation (sometimes known as a grey body) emits ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Black Body
A black body or blackbody is an idealized physical object, physical body that absorption (electromagnetic radiation), absorbs all incident electromagnetic radiation, regardless of frequency or angle of incidence (optics), angle of incidence. The name "black body" is given because it absorbs all colors of light. A black body also emits black-body radiation. In contrast, a white body is one with a "rough surface that reflects all incident rays completely and uniformly in all directions." A black body in thermal equilibrium (that is, at a constant temperature) emits electromagnetic black-body radiation. The radiation is emitted according to Planck's law, meaning that it has a frequency spectrum, spectrum that is determined by the temperature alone (see figure at right), not by the body's shape or composition. An ideal black body in thermal equilibrium has two main properties: #It is an ideal emitter: at every frequency, it emits as much or more thermal radiative energy as any o ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Emissivity
The emissivity of the surface of a material is its effectiveness in emitting energy as thermal radiation. Thermal radiation is electromagnetic radiation that most commonly includes both visible radiation (light) and infrared radiation, which is not visible to human eyes. A portion of the thermal radiation from very hot objects (see photograph) is easily visible to the eye. The emissivity of a surface depends on its chemical composition and geometrical structure. Quantitatively, it is the ratio of the thermal radiation from a surface to the radiation from an ideal black surface at the same temperature as given by the Stefan–Boltzmann law. The ratio varies from 0 to 1. The surface of a perfect black body (with an emissivity of 1) emits thermal radiation at the rate of approximately 448 watts per square metre at room temperature (, ). All real objects have emissivities less than 1.0, and emit radiation at correspondingly lower rates. Emissivities are important in several conte ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Solar Constant
The solar constant (''GSC'') is a flux density measuring mean solar electromagnetic radiation ( total solar irradiance) per unit area. It is measured on a surface perpendicular to the rays, one astronomical unit (au) from the Sun (roughly the distance from the Sun to the Earth). The solar constant includes radiation over the entire electromagnetic spectrum. It is measured by satellite as being 1.361 kilowatts per square meter (kW/m2) at solar minimum (the time in the 11-year solar cycle when the number of sunspots is minimal) and approximately 0.1% greater (roughly 1.362 kW/m2) at solar maximum. The solar "constant" is not a physical constant in the modern CODATA scientific sense; that is, it is not like the Planck constant or the speed of light which are absolutely constant in physics. The solar constant is an average of a varying value. In the past 400 years it has varied less than 0.2 percent.http://lasp.colorado.edu/home/sorce/data/tsi-data/ Total Solar Irradiance ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Radiative Flux
Radiative flux, also known as radiative flux density or radiation flux (or sometimes power flux density), is the amount of power radiated through a given area, in the form of photons or other elementary particles, typically measured in W/m2. It is used in astronomy to determine the magnitude and spectral class of a star and in meteorology to determine the intensity of the convection in the planetary boundary layer. Radiative flux also acts as a generalization of heat flux, which is equal to the radiative flux when restricted to the infrared spectrum. When radiative flux is incident on a surface, it is often called irradiance. Flux emitted from a surface may be called radiant exitance or radiant emittance. The ratio of irradiance reflected to the irradiance received by a surface is called albedo. Shortwave radiation flux Shortwave flux is a result of specular and diffuse reflection of incident shortwave radiation by the underlying surface. This shortwave radiation, as solar radiatio ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Bond Albedo
The Bond albedo (or ''spheric albedo'' or ''planetary albedo'' or ''bolometric albedo''), named after the American astronomer George Phillips Bond (1825–1865), who originally proposed it, is the fraction of power in the total electromagnetic radiation incident on an astronomical body that is scattered back out into space. Because the Bond albedo accounts for all of the light scattered from a body at all wavelengths and all phase angles, it is a necessary quantity for determining how much energy a body absorbs. This, in turn, is crucial for determining the equilibrium temperature of a body. Because bodies in the outer Solar System are always observed at very low phase angles from the Earth, the only reliable data for measuring their Bond albedo comes from spacecraft. Phase integral The Bond albedo (''A'') is related to the geometric albedo (''p'') by the expression :A = pq where ''q'' is termed the ''phase integral'' and is given in terms of the directional scattered flux ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
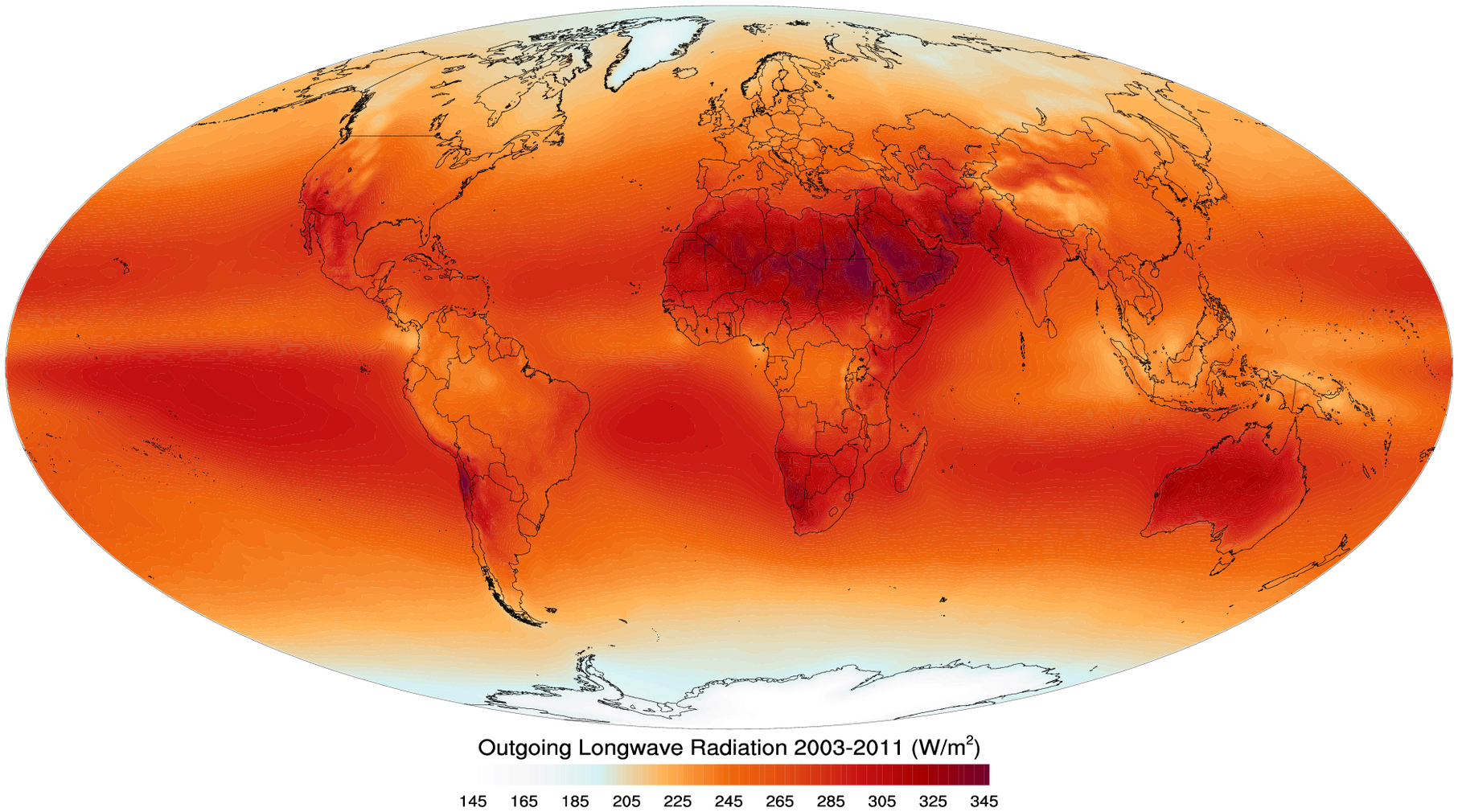
Outgoing Longwave Radiation
Outgoing Long-wave Radiation (OLR) is electromagnetic radiation of wavelengths from 3–100 μm emitted from Earth and its atmosphere out to space in the form of thermal radiation. It is also referred to as up-welling long-wave radiation and terrestrial long-wave flux, among others. The flux of energy transported by outgoing long-wave radiation is measured in W/m2. In the Earth's climate system, long-wave radiation involves processes of absorption, scattering, and emissions from atmospheric gases, aerosols, clouds and the surface. Over 99% of outgoing long-wave radiation has wavelengths between 4 μm and 100 μm, in the thermal infrared part of the electromagnetic spectrum. Contributions with wavelengths larger than 40 μm are small, therefore often only wavelengths up to 50 μm are considered . In the wavelength range between 4 μm and 10 μm the spectrum of outgoing long-wave radiation overlaps that of solar radiation, and for various applica ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |