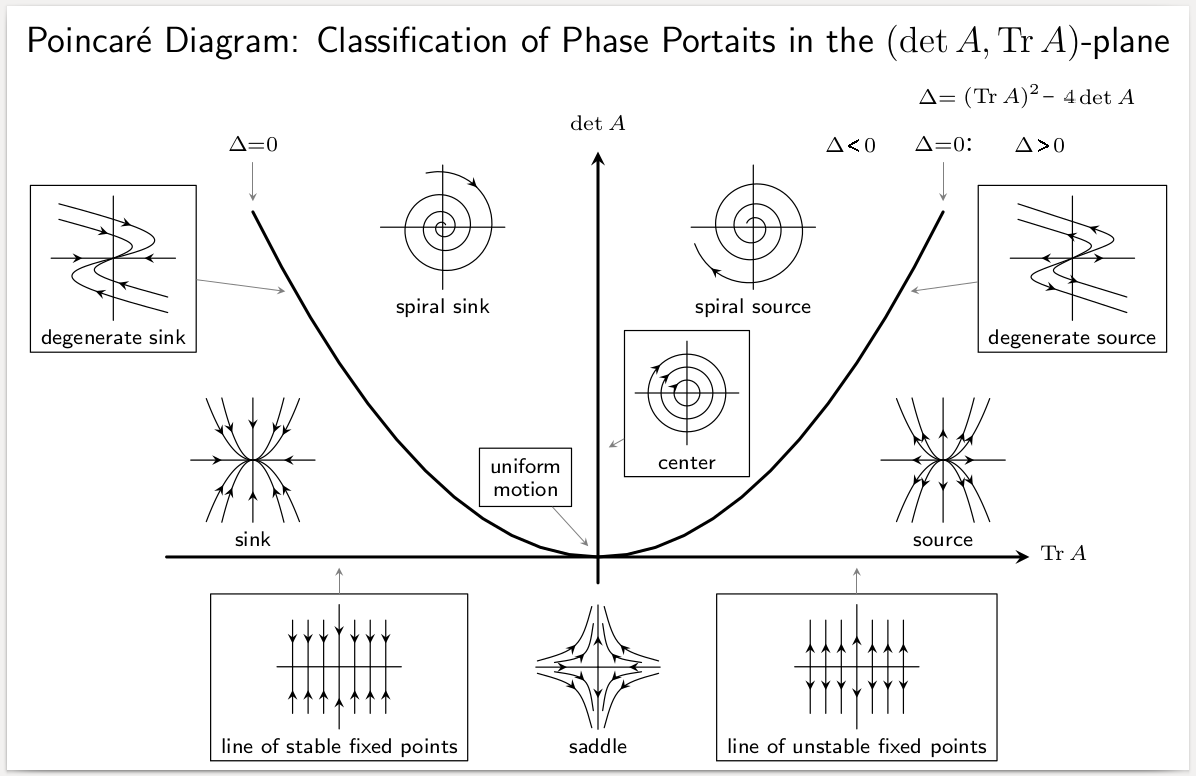
|
Hyperstability
In stability theory, hyperstability is a property of a system that requires the state vector to remain bounded if the inputs are restricted to belonging to a subset of the set of all possible inputs.Brian D. O Anderson, "A Simplified Viewpoint of Hyperstability", ''IEEE Transactions on Automatic Control, June 1968'' Definition:Zinober, Deterministic control of uncertain systems, 1990 A system is hyperstable if there are two constants k_1 \ge 0, k_2 \ge 0 such that any state trajectory of the system satisfies the inequality: :\, x(t) \, < k_1 \, x(0)\, + k_2, \, \forall t \ge 0 References See also * * |
Stability Theory
In mathematics, stability theory addresses the stability of solutions of differential equations and of trajectories of dynamical systems under small perturbations of initial conditions. The heat equation, for example, is a stable partial differential equation because small perturbations of initial data lead to small variations in temperature at a later time as a result of the maximum principle. In partial differential equations one may measure the distances between functions using Lp norms or the sup norm, while in differential geometry one may measure the distance between spaces using the Gromov–Hausdorff distance. In dynamical systems, an orbit is called ''Lyapunov stable'' if the forward orbit of any point is in a small enough neighborhood or it stays in a small (but perhaps, larger) neighborhood. Various criteria have been developed to prove stability or instability of an orbit. Under favorable circumstances, the question may be reduced to a well-studied problem involvi ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Stability Theory
In mathematics, stability theory addresses the stability of solutions of differential equations and of trajectories of dynamical systems under small perturbations of initial conditions. The heat equation, for example, is a stable partial differential equation because small perturbations of initial data lead to small variations in temperature at a later time as a result of the maximum principle. In partial differential equations one may measure the distances between functions using Lp norms or the sup norm, while in differential geometry one may measure the distance between spaces using the Gromov–Hausdorff distance. In dynamical systems, an orbit is called ''Lyapunov stable'' if the forward orbit of any point is in a small enough neighborhood or it stays in a small (but perhaps, larger) neighborhood. Various criteria have been developed to prove stability or instability of an orbit. Under favorable circumstances, the question may be reduced to a well-studied problem involvi ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
State Space Representation
In control engineering, a state-space representation is a mathematical model of a physical system as a set of input, output and state variables related by first-order differential equations or difference equations. State variables are variables whose values evolve over time in a way that depends on the values they have at any given time and on the externally imposed values of input variables. Output variables’ values depend on the values of the state variables. The "state space" is the Euclidean space in which the variables on the axes are the state variables. The state of the system can be represented as a ''state vector'' within that space. To abstract from the number of inputs, outputs and states, these variables are expressed as row and column vectors, vectors. If the dynamical system is linear, time-invariant, and finite-dimensional, then the differential and algebraic equations may be written in matrix (mathematics), matrix form. The state-space method is characterized by ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
BIBO Stability
In signal processing, specifically control theory, bounded-input, bounded-output (BIBO) stability is a form of stability for signals and systems that take inputs. If a system is BIBO stable, then the output will be bounded for every input to the system that is bounded. A signal is bounded if there is a finite value B > 0 such that the signal magnitude never exceeds B, that is :For discrete-time signals: \ , y \leq B \quad \text n \in \mathbb. :For continuous-time signals: \ , y(t), \leq B \quad \text t \in \mathbb. Time-domain condition for linear time-invariant systems Continuous-time necessary and sufficient condition For a continuous time linear time-invariant (LTI) system, the condition for BIBO stability is that the impulse response, h(t) , be absolutely integrable, i.e., its L1 norm exists. : \int_^\infty \left, h(t)\\,\mathordt = \, h \, _1 and the output \ y /math> is :\ y = h * x /math> where * denotes convolution. Then it follows by the definition of convol ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |