|
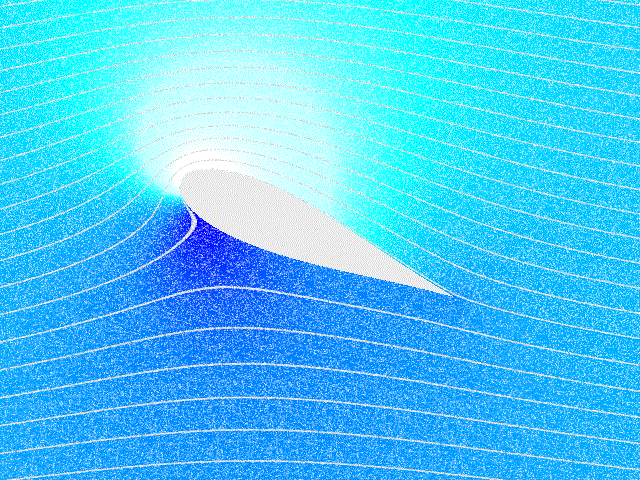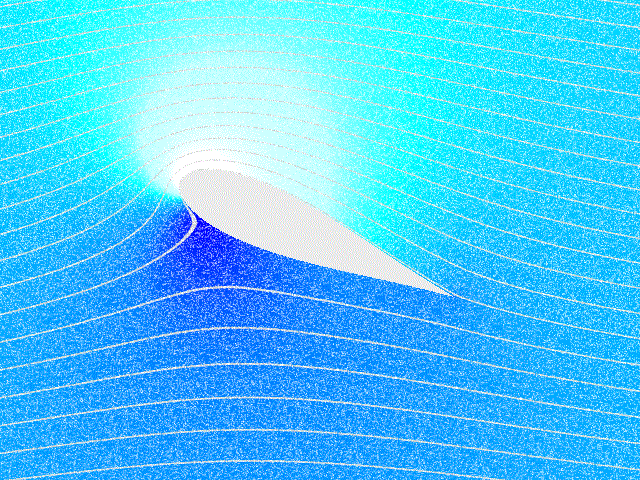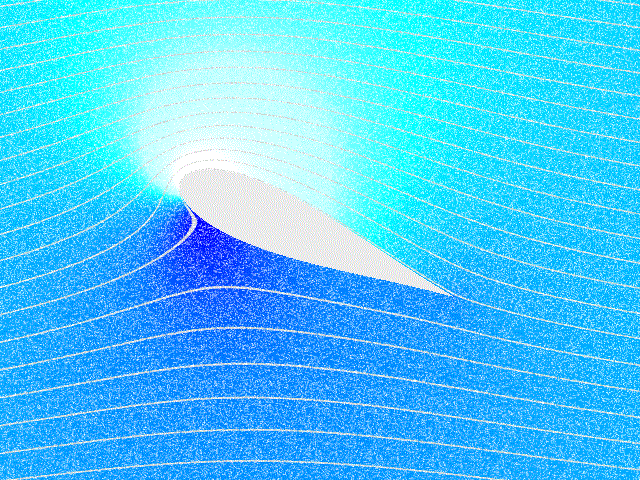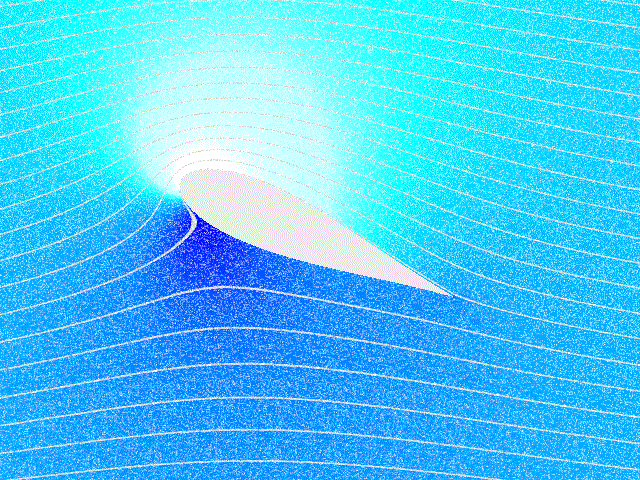
Hill's Spherical Vortex
Hill's spherical vortex is an exact solution of the Euler equations that is commonly used to model a vortex ring. The solution is also used to model the velocity distribution inside a spherical drop of one fluid moving at a constant velocity through another fluid at small Reynolds number. The vortex is named after Micaiah John Muller Hill who discovered the exact solution in 1894. The two-dimensional analogue of this vortex is the Lamb–Chaplygin dipole. The solution is described in the spherical polar coordinates system (r,\theta,\phi) with corresponding velocity components (v_r,v_\theta,0). The velocity components are identified from Stokes stream function \psi(r,\theta) as follows :v_r = \frac\frac, \quad v_\theta = - \frac\frac. The Hill's spherical vortex is described by : \psi=\begin-\frac \left(1-\frac\right) r^2\sin^2\theta \quad \text \quad r\leq a\\ \frac \left(1 - \frac\right)r^2\sin^2\theta \quad \text \quad r\geq a \end where U is a constant freestream velocity fa ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Euler Equations (fluid Dynamics)
In fluid dynamics, the Euler equations are a set of quasilinear partial differential equations governing adiabatic and inviscid flow. They are named after Leonhard Euler. In particular, they correspond to the Navier–Stokes equations with zero viscosity and zero thermal conductivity. The Euler equations can be applied to incompressible or compressible flow. The incompressible Euler equations consist of Cauchy equations for conservation of mass and balance of momentum, together with the incompressibility condition that the flow velocity is a solenoidal field. The compressible Euler equations consist of equations for conservation of mass, balance of momentum, and balance of energy, together with a suitable constitutive equation for the specific energy density of the fluid. Historically, only the equations of conservation of mass and balance of momentum were derived by Euler. However, fluid dynamics literature often refers to the full set of the compressible Euler equations – ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
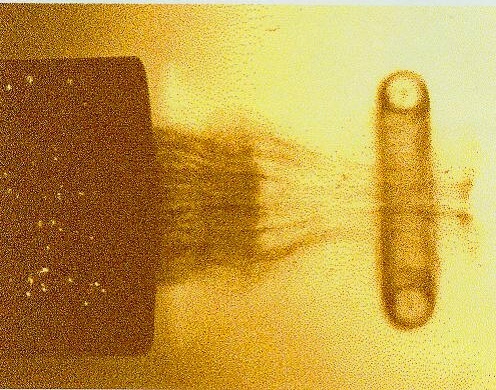
Vortex Ring
A vortex ring, also called a toroidal vortex, is a torus-shaped vortex in a fluid; that is, a region where the fluid mostly spins around an imaginary axis line that forms a closed loop. The dominant flow in a vortex ring is said to be toroidal, more precisely poloidal. Vortex rings are plentiful in turbulent flows of liquids and gases, but are rarely noticed unless the motion of the fluid is revealed by suspended particles—as in the smoke rings which are often produced intentionally or accidentally by smokers. Fiery vortex rings are also a commonly produced trick by fire eaters. Visible vortex rings can also be formed by the firing of certain artillery, in mushroom clouds, and in microbursts. A vortex ring usually tends to move in a direction that is perpendicular to the plane of the ring and such that the inner edge of the ring moves faster forward than the outer edge. Within a stationary body of fluid, a vortex ring can travel for relatively long distance, carrying the ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Micaiah John Muller Hill
Micaiah John Muller Hill FRS (1856–1929) was an English mathematician, known for Hill's spherical vortex and Hill's tetrahedra. He was born on 22 February 1856 in Bengal, India, the son of Revd. Samuel John Hill (1825–1881) and Leonora Josephina Muller (1833–1917). Hill received a bachelor's degree in 1873 and an M.A. in 1876 from University College, London. In 1880–1884 he was a professor of mathematics at Mason College (which later became Birmingham University). In 1891 he earned his Sc.D. from Cambridge University. From 1884 to 1907 he was Professor of Pure Mathematics at University College, London and from 1907 to 1923 Astor Professor of Mathematics, University of London. In 1894 Hill was elected FRS. In 1926 and 1927 he served as president of the Mathematical Association. Hill was one of the people to whom C. L. T. Griffith sent, in 1912, some of Ramanujan's work. He married Minnie Grace Tarbotton, daughter of Marriott Ogle Tarbotton at St Saviour's Church, Pad ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Lamb–Chaplygin Dipole
The Lamb–Chaplygin dipole model is a mathematical description for a particular inviscid and steady dipolar vortex flow. It is a non-trivial solution to the two-dimensional Euler equations. The model is named after Horace Lamb and Sergey Alexeyevich Chaplygin, who independently discovered this flow structure. This dipole is the two-dimensional analogue of Hill's spherical vortex. __TOC__ The model A two-dimensional (2D), solenoidal vector field \mathbf may be described by a scalar stream function \psi, via \mathbf = -\mathbf \times \mathbf \psi, where \mathbf is the right-handed unit vector perpendicular to the 2D plane. By definition, the stream function is related to the vorticity \omega via a Poisson equation: -\nabla^2\psi = \omega. The Lamb–Chaplygin model follows from demanding the following characteristics: * The dipole has a circular atmosphere/separatrix with radius R: \psi\left(r = R\right) = 0. * The dipole propages through an otherwise irrorational fluid (\ome ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Stokes Stream Function
In fluid dynamics, the Stokes stream function is used to describe the streamlines and flow velocity in a three-dimensional incompressible flow with axisymmetry. A surface with a constant value of the Stokes stream function encloses a streamtube, everywhere tangential to the flow velocity vectors. Further, the volume flux within this streamtube is constant, and all the streamlines of the flow are located on this surface. The velocity field associated with the Stokes stream function is solenoidal—it has zero divergence. This stream function is named in honor of George Gabriel Stokes. Cylindrical coordinates Consider a cylindrical coordinate system ( ''ρ'' , ''φ'' , ''z'' ), with the ''z''–axis the line around which the incompressible flow is axisymmetrical, ''φ'' the azimuthal angle and ''ρ'' the distance to the ''z''–axis. Then the flow velocity components ''uρ'' and ''uz'' can be expressed in terms of the Stokes stream function \Psi by: : ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Vorticity
In continuum mechanics, vorticity is a pseudovector field that describes the local spinning motion of a continuum near some point (the tendency of something to rotate), as would be seen by an observer located at that point and traveling along with the flow. It is an important quantity in the dynamical theory of fluids and provides a convenient framework for understanding a variety of complex flow phenomena, such as the formation and motion of vortex rings. Mathematically, the vorticity \vec is the curl of the flow velocity \vec: :\vec \equiv \nabla \times \vec\,, where \nabla is the nabla operator. Conceptually, \vec could be determined by marking parts of a continuum in a small neighborhood of the point in question, and watching their ''relative'' displacements as they move along the flow. The vorticity \vec would be twice the mean angular velocity vector of those particles relative to their center of mass, oriented according to the right-hand rule. In a two-dimensional fl ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Potential Flow
In fluid dynamics, potential flow (or ideal flow) describes the velocity field as the gradient of a scalar function: the velocity potential. As a result, a potential flow is characterized by an irrotational velocity field, which is a valid approximation for several applications. The irrotationality of a potential flow is due to the curl of the gradient of a scalar always being equal to zero. In the case of an incompressible flow the velocity potential satisfies Laplace's equation, and potential theory is applicable. However, potential flows also have been used to describe compressible flows. The potential flow approach occurs in the modeling of both stationary as well as nonstationary flows. Applications of potential flow are for instance: the outer flow field for aerofoils, water waves, electroosmotic flow, and groundwater flow. For flows (or parts thereof) with strong vorticity effects, the potential flow approximation is not applicable. Characteristics and applications ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Keith Moffatt
Henry Keith Moffatt, FRS FRSE (born 12 April 1935) is a Scottish mathematician with research interests in the field of fluid dynamics, particularly magnetohydrodynamics and the theory of turbulence. He was Professor of Mathematical Physics at the University of Cambridge from 1980 to 2002. Early life and education Moffatt was born on 12 April 1935 to Emmeline Marchant and Frederick Henry Moffatt''.'' He was schooled at George Watson's College, Edinburgh, going on to study Mathematical Sciences at the University of Edinburgh, graduating in 1957. He then went to Trinity College, Cambridge, where he studied mathematics and, 1959, he was a Wrangler. In 1960, he was awarded a Smith's Prize while preparing his PhD. He received his PhD in 1962, the title of his dissertation was ''Magnetohydrodynamic Turbulence.'' Career After completing his PhD, Moffatt joined the staff of the Mathematics Faculty in Cambridge as an Assistant Lecturer and became a Fellow of Trinity College. He was ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
William Mitchinson Hicks
William Mitchinson Hicks, FRS (23 September 1850, in Launceston, Cornwall – 17 August 1934, in Crowhurst, Sussex) was a British mathematician and physicist. He studied at St John's College, Cambridge, graduating in 1873, and became a Fellow at the College. Hicks spent most of his career at Sheffield, contributing to the development of the university there. He was Principal of Firth College from 1892 to 1897. In 1897, Firth College merged with two other colleges to form the University College of Sheffield, and Hicks was its first Principal until 1905, when the College received its own Royal Charter and became the University of Sheffield. Hicks was the first Vice Chancellor of the University, serving from 1905. From 1883 to 1892, he was Professor of Physics and Mathematics at Sheffield, and was Professor of Physics there from 1892 to 1917. He was elected a Fellow of the Royal Society in 1885. He was awarded the Royal Society's Royal Medal in 1912: ''"On the ground of his r ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Hicks Equation
In fluid dynamics, Hicks equation, sometimes also referred as Bragg–Hawthorne equation or Squire–Long equation, is a partial differential equation that describes the distribution of stream function for axisymmetric inviscid fluid, named after William Mitchinson Hicks, who derived it first in 1898. The equation was also re-derived by Stephen Bragg and William Hawthorne in 1950 and by Robert R. Long in 1953 and by Herbert Squire in 1956. The Hicks equation without swirl was first introduced by George Gabriel Stokes in 1842. The Grad–Shafranov equation appearing in plasma physics also takes the same form as the Hicks equation. Representing (r,\theta,z) as coordinates in the sense of cylindrical coordinate system with corresponding flow velocity components denoted by (v_r,v_\theta,v_z), the stream function \psi that defines the meridional motion can be defined as :rv_r = - \frac, \quad rv_z = \frac that satisfies the continuity equation for axisymmetric flows automatically. Th ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Grad–Shafranov Equation
The Grad–Shafranov equation ( H. Grad and H. Rubin (1958); Vitalii Dmitrievich Shafranov (1966)) is the equilibrium equation in ideal magnetohydrodynamics (MHD) for a two dimensional plasma, for example the axisymmetric toroidal plasma in a tokamak. This equation takes the same form as the Hicks equation from fluid dynamics.Smith, S. G. L., & Hattori, Y. (2012). Axisymmetric magnetic vortices with swirl. Communications in Nonlinear Science and Numerical Simulation, 17(5), 2101-2107. This equation is a two-dimensional, nonlinear, elliptic partial differential equation obtained from the reduction of the ideal MHD equations to two dimensions, often for the case of toroidal axisymmetry (the case relevant in a tokamak). Taking (r,\theta,z) as the cylindrical coordinates, the flux function \psi is governed by the equation, \frac - \frac \frac + \frac = - \mu_0 r^\frac - \frac \frac, where \mu_0 is the magnetic permeability, p(\psi) is the pressure, F(\psi)=rB_ and the magnetic field and ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Bessel Functions
Bessel functions, first defined by the mathematician Daniel Bernoulli and then generalized by Friedrich Bessel, are canonical solutions of Bessel's differential equation x^2 \frac + x \frac + \left(x^2 - \alpha^2 \right)y = 0 for an arbitrary complex number \alpha, the ''order'' of the Bessel function. Although \alpha and -\alpha produce the same differential equation, it is conventional to define different Bessel functions for these two values in such a way that the Bessel functions are mostly smooth functions of \alpha. The most important cases are when \alpha is an integer or half-integer. Bessel functions for integer \alpha are also known as cylinder functions or the cylindrical harmonics because they appear in the solution to Laplace's equation in cylindrical coordinates. Spherical Bessel functions with half-integer \alpha are obtained when the Helmholtz equation is solved in spherical coordinates. Applications of Bessel functions The Bessel function is a generalization ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |