|
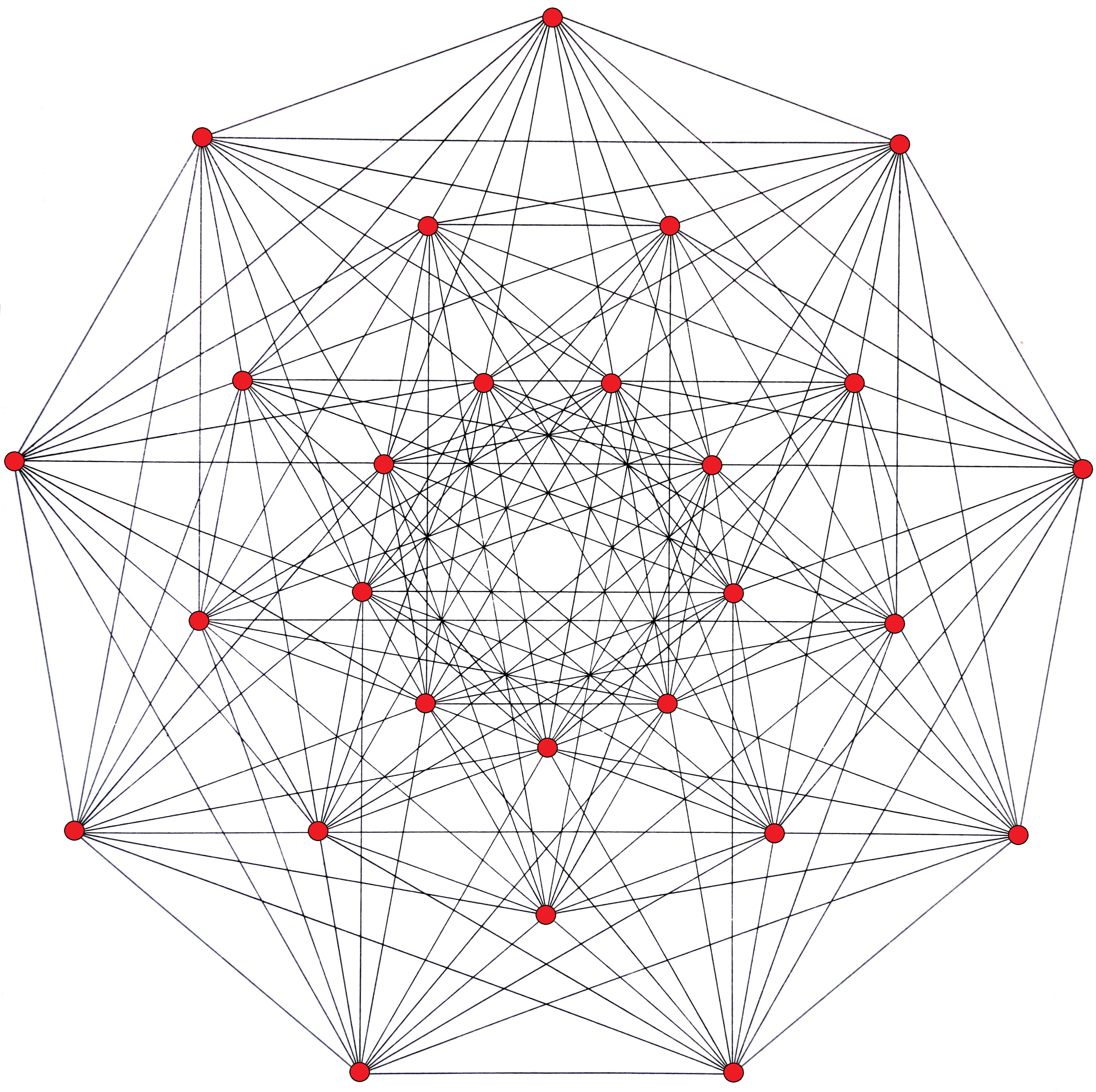
Hessian Polyhedron
In geometry, the Hessian polyhedron is a regular complex polyhedron 333, , in \mathbb^3. It has 27 vertices, 72 3 edges, and 27 33 faces. It is self-dual. Coxeter named it after Ludwig Otto Hesse for sharing the ''Hessian configuration'' \left begin 9&4\\3&12 \end\right /math> or (94123), 9 points lying by threes on twelve lines, with four lines through each point. Its complex reflection group is 3 sub>3 sub>3 or , order 648, also called a Hessian group. It has 27 copies of , order 24, at each vertex. It has 24 order-3 reflections. Its Coxeter number is 12, with degrees of the fundamental invariants 3, 6, and 12, which can be seen in projective symmetry of the polytopes. The Witting polytope, 3333, contains the Hessian polyhedron as cells and vertex figures. It has a real representation as the ''221'' polytope, , in 6-dimensional space, sharing the same 27 vertices. The 216 edges in ''221'' can be seen as the 72 3 edges represented as 3 simple edges. Coordinates Its 27 ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Complex Polyhedron 3-3-3-3-3
Complex commonly refers to: * Complexity, the behaviour of a system whose components interact in multiple ways so possible interactions are difficult to describe ** Complex system, a system composed of many components which may interact with each other * Complex (psychology), a core pattern of emotions etc. in the personal unconscious organized around a common theme such as power or status Complex may also refer to: Arts, entertainment and media * Complex (English band), formed in 1968, and their 1971 album ''Complex'' * Complex (band), a Japanese rock band * ''Complex'' (album), by Montaigne, 2019, and its title track * ''Complex'' (EP), by Rifle Sport, 1985 * "Complex" (song), by Gary Numan, 1979 * Complex Networks, publisher of magazine ''Complex'', now online Biology * Protein–ligand complex, a complex of a protein bound with a ligand * Exosome complex, a multi-protein intracellular complex * Protein complex, a group of two or more associated polypeptide chains * Specie ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Hessian Configuration
In geometry, the Hesse configuration, introduced by Colin Maclaurin and studied by , is a configuration of 9 points and 12 lines with three points per line and four lines through each point. It can be realized in the complex projective plane as the set of inflection points of an elliptic curve, but it has no realization in the Euclidean plane. Description The Hesse configuration has the same incidence relations as the lines and points of the affine plane over the field of 3 elements. That is, the points of the Hesse configuration may be identified with ordered pairs of numbers modulo 3, and the lines of the configuration may correspondingly be identified with the triples of points satisfying a linear equation . Alternatively, the points of the configuration may be identified by the squares of a tic-tac-toe board, and the lines may be identified with the lines and broken diagonals of the board. Each point belongs to four lines: in the tic tac toe interpretation of the configura ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
K-face
In solid geometry, a face is a flat surface (a planar region) that forms part of the boundary of a solid object; a three-dimensional solid bounded exclusively by faces is a ''polyhedron''. In more technical treatments of the geometry of polyhedra and higher-dimensional polytopes, the term is also used to mean an element of any dimension of a more general polytope (in any number of dimensions).. Polygonal face In elementary geometry, a face is a polygon on the boundary of a polyhedron. Other names for a polygonal face include polyhedron side and Euclidean plane ''tile''. For example, any of the six squares that bound a cube is a face of the cube. Sometimes "face" is also used to refer to the 2-dimensional features of a 4-polytope. With this meaning, the 4-dimensional tesseract has 24 square faces, each sharing two of 8 cubic cells. Number of polygonal faces of a polyhedron Any convex polyhedron's surface has Euler characteristic :V - E + F = 2, where ''V'' is the number of ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
F-vector
Polyhedral combinatorics is a branch of mathematics, within combinatorics and discrete geometry, that studies the problems of counting and describing the faces of convex polyhedra and higher-dimensional convex polytopes. Research in polyhedral combinatorics falls into two distinct areas. Mathematicians in this area study the combinatorics of polytopes; for instance, they seek inequalities that describe the relations between the numbers of vertices, edges, and faces of higher dimensions in arbitrary polytopes or in certain important subclasses of polytopes, and study other combinatorial properties of polytopes such as their connectivity and diameter (number of steps needed to reach any vertex from any other vertex). Additionally, many computer scientists use the phrase “polyhedral combinatorics” to describe research into precise descriptions of the faces of certain specific polytopes (especially 0-1 polytopes, whose vertices are subsets of a hypercube) arising from integer progr ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Configuration (polytope)
In geometry, H. S. M. Coxeter called a regular polytope a special kind of configuration. Other configurations in geometry are something different. These ''polytope configurations'' may be more accurately called incidence matrices, where like elements are collected together in rows and columns. Regular polytopes will have one row and column per ''k''-face element, while other polytopes will have one row and column for each k-face type by their symmetry classes. A polytope with no symmetry will have one row and column for every element, and the matrix will be filled with 0 if the elements are not connected, and 1 if they are connected. Elements of the same ''k'' will not be connected and will have a "*" table entry. Every polytope, and abstract polytope has a Hasse diagram expressing these connectivities, which can be systematically described with an incidence matrix. Configuration matrix for regular polytopes A configuration for a regular polytope is represented by a matrix whe ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
3-3 Duoprism
In the geometry of 4 dimensions, the 3-3 duoprism or triangular duoprism is a 4-polytope, four-dimensional convex polytope. It can be constructed as the Cartesian product of two triangles and is the simplest of an infinite family of four-dimensional polytopes constructed as Cartesian products of two polygons, the duoprisms. It has 9 vertices, 18 edges, 15 faces (9 squares, and 6 triangles), in 6 triangular prism cells. It has Coxeter diagram , and symmetry , order 72. Its vertices and edges form a 3\times 3 rook's graph. Hypervolume The hypervolume of a Uniform_4-polytope#Duoprisms:_.5Bp.5D_.C3.97_.5Bq.5D, uniform 3-3 duoprism, with edge length ''a'', is V_4 = a^4. This is the square of the Equilateral_triangle#Principal_properties, area of an equilateral triangle, A = a^2. Graph The graph of vertices and edges of the 3-3 duoprism has 9 vertices and 18 edges. Like the Berlekamp–van Lint–Seidel graph and the unknown solution to Conway's 99-graph problem, every edge is part of ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Complex Polyhedron 3-3-3-3-3-one-blue-van Oss Polygon
Complex commonly refers to: * Complexity, the behaviour of a system whose components interact in multiple ways so possible interactions are difficult to describe ** Complex system, a system composed of many components which may interact with each other * Complex (psychology), a core pattern of emotions etc. in the personal unconscious organized around a common theme such as power or status Complex may also refer to: Arts, entertainment and media * Complex (English band), formed in 1968, and their 1971 album ''Complex'' * Complex (band), a Japanese rock band * ''Complex'' (album), by Montaigne, 2019, and its title track * ''Complex'' (EP), by Rifle Sport, 1985 * "Complex" (song), by Gary Numan, 1979 * Complex Networks, publisher of magazine ''Complex'', now online Biology * Protein–ligand complex, a complex of a protein bound with a ligand * Exosome complex, a multi-protein intracellular complex * Protein complex, a group of two or more associated polypeptide chains * Specie ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Complex Polyhedron 3-3-3-3-3-one-blue-face
Complex commonly refers to: * Complexity, the behaviour of a system whose components interact in multiple ways so possible interactions are difficult to describe ** Complex system, a system composed of many components which may interact with each other * Complex (psychology), a core pattern of emotions etc. in the personal unconscious organized around a common theme such as power or status Complex may also refer to: Arts, entertainment and media * Complex (English band), formed in 1968, and their 1971 album ''Complex'' * Complex (band), a Japanese rock band * ''Complex'' (album), by Montaigne, 2019, and its title track * ''Complex'' (EP), by Rifle Sport, 1985 * "Complex" (song), by Gary Numan, 1979 * Complex Networks, publisher of magazine ''Complex'', now online Biology * Protein–ligand complex, a complex of a protein bound with a ligand * Exosome complex, a multi-protein intracellular complex * Protein complex, a group of two or more associated polypeptide chains * Specie ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
2 21 Polytope
In 6-dimensional geometry, the 221 polytope is a uniform 6-polytope, constructed within the symmetry of the E6 group. It was discovered by Thorold Gosset, published in his 1900 paper. He called it an 6-ic semi-regular figure. It is also called the Schläfli polytope. Its Coxeter symbol is 221, describing its bifurcating Coxeter-Dynkin diagram, with a single ring on the end of one of the 2-node sequences. He also studied its connection with the 27 lines on the cubic surface, which are naturally in correspondence with the vertices of 221. The rectified 221 is constructed by points at the mid-edges of the 221. The birectified 221 is constructed by points at the triangle face centers of the 221, and is the same as the rectified 122. These polytopes are a part of family of 39 convex uniform polytopes in 6-dimensions, made of uniform 5-polytope facets and vertex figures, defined by all permutations of rings in this Coxeter-Dynkin diagram: . 2_21 polytope The 221 has 27 verti ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Vertex Figure
In geometry, a vertex figure, broadly speaking, is the figure exposed when a corner of a polyhedron or polytope is sliced off. Definitions Take some corner or Vertex (geometry), vertex of a polyhedron. Mark a point somewhere along each connected edge. Draw lines across the connected faces, joining adjacent points around the face. When done, these lines form a complete circuit, i.e. a polygon, around the vertex. This polygon is the vertex figure. More precise formal definitions can vary quite widely, according to circumstance. For example Coxeter (e.g. 1948, 1954) varies his definition as convenient for the current area of discussion. Most of the following definitions of a vertex figure apply equally well to infinite tessellation, tilings or, by extension, to Honeycomb (geometry), space-filling tessellation with polytope Cell (geometry), cells and other higher-dimensional polytopes. As a flat slice Make a slice through the corner of the polyhedron, cutting through all the edges ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Cell (geometry)
In solid geometry, a face is a flat surface (a planar region) that forms part of the boundary of a solid object; a three-dimensional solid bounded exclusively by faces is a ''polyhedron''. In more technical treatments of the geometry of polyhedra and higher-dimensional polytopes, the term is also used to mean an element of any dimension of a more general polytope (in any number of dimensions).. Polygonal face In elementary geometry, a face is a polygon on the boundary of a polyhedron. Other names for a polygonal face include polyhedron side and Euclidean plane ''tile''. For example, any of the six squares that bound a cube is a face of the cube. Sometimes "face" is also used to refer to the 2-dimensional features of a 4-polytope. With this meaning, the 4-dimensional tesseract has 24 square faces, each sharing two of 8 cubic cells. Number of polygonal faces of a polyhedron Any convex polyhedron's surface has Euler characteristic :V - E + F = 2, where ''V'' is the number of ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Witting Polytope
In 4-dimensional complex geometry, the Witting polytope is a regular complex polytope, named as: 3333, and Coxeter diagram . It has 240 vertices, 2160 3 edges, 2160 Möbius–Kantor polygon, 33 faces, and 240 Hessian polyhedron, 333 cells. It is self-dual. Each vertex belongs to 27 edges, 72 faces, and 27 cells, corresponding to the Hessian polyhedron vertex figure. Symmetry Its symmetry by 3[3]3[3]3[3]3 or , order 155,520. It has 240 copies of , order 648 at each cell. Structure The Configuration (polytope), configuration matrix is: \left [\begin240&27&72&27\\3&2160&8&8\\8&8&2160&3\\27&72&27&240\end\right ] The number of vertices, edges, faces, and cells are seen in the diagonal of the matrix. These are computed by the order of the group divided by the order of the subgroup, by removing certain complex reflections, shown with X below. The number of elements of the k-faces are seen in rows below the diagonal. The number of elements in the vertex figure, etc., are given in rows ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |