|
Hessian Centre For Artificial Intelligence
A Hessian is an inhabitant of the German state of Hesse. Hessian may also refer to: Named from the toponym *Hessian (soldier), eighteenth-century German regiments in service with the British Empire **Hessian (boot), a style of boot **Hessian fabric, coarse woven material **Hessian fly or barley midge, a species of fly (thought to be introduced by Hessian soldiers) *Hessian dialects, West Central German group of dialects *Hessian crucible, a type of ceramic crucible *Hessian Cup, a regional cup competition in German football Named for Otto Hesse *Hessian matrix, in mathematics, is a matrix of second partial derivatives ** Hessian affine region detector, a feature detector used in the fields of computer vision and image analysis **Hessian automatic differentiation ** Hessian equations, partial differential equations (PDEs) based on the Hessian matrix *Hessian pair or Hessian duad in mathematics *Hessian form of an elliptic curve *Hessian group * Hessian polyhedron * Glossary of ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Hesse
Hesse (, , ) or Hessia (, ; german: Hessen ), officially the State of Hessen (german: links=no, Land Hessen), is a States of Germany, state in Germany. Its capital city is Wiesbaden, and the largest urban area is Frankfurt. Two other major historic cities are Darmstadt and Kassel. With an area of 21,114.73 square kilometers and a population of just over six million, it ranks seventh and fifth, respectively, among the sixteen German states. Frankfurt Rhine-Main, Germany's second-largest metropolitan area (after Rhine-Ruhr), is mainly located in Hesse. As a cultural region, Hesse also includes the area known as Rhenish Hesse (Rheinhessen) in the neighbouring state of Rhineland-Palatinate. Name The German name '':wikt:Hessen#German, Hessen'', like the names of other German regions (''Schwaben'' "Swabia", ''Franken'' "Franconia", ''Bayern'' "Bavaria", ''Sachsen'' "Saxony"), derives from the dative plural form of the name of the inhabitants or German tribes, eponymous tribe, the Hes ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Hessian Equation
In mathematics, ''k''-Hessian equations (or Hessian equations for short) are partial differential equations (PDEs) based on the Hessian matrix. More specifically, a Hessian equation is the ''k''-trace, or the ''k''th elementary symmetric polynomial of eigenvalues of the Hessian matrix. When ''k'' ≥ 2, the ''k''-Hessian equation is a fully nonlinear partial differential equation. It can be written as _k f, where 1\leqslant k \leqslant n, _k \sigma_k(\lambda(^2u)), and \lambda(^2u)=(\lambda_1,\cdots,\lambda_n), are the eigenvalues of the ''Hessian matrix'' ^2u= partial_i \partial_ju and \sigma_k(\lambda)=\sum_\lambda_\cdots\lambda_, is a k th elementary symmetric polynomial. Much like differential equations often study the actions of differential operators (e.g. elliptic operators and elliptic equations), Hessian equations can be understood as simply eigenvalue equations acted upon by the Hessian differential operator. Special cases include the Monge–Ampère equation. and Po ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Stephen Hessian
Stephen Stanley Hessian (October 2, 1891 – November 5, 1962) was a lawyer and political figure on Prince Edward Island. He represented 5th Kings from 1920 to 1923 and 3rd Kings from 1935 to 1939 and from 1955 to 1962 in the Legislative Assembly of Prince Edward Island as a Prince Edward Island Liberal Party, Liberal. He was born in Georgetown, Prince Edward Island, the son of Thomas G. Hessian and Hannah Cummings, and was educated at Saint Dunstan's University, Saint Dunstan's College.Normandin, Al ''Canadian Parliamentary Guide, 1938'' In 1928, he married Blanche Wickham. Hessian was speaker for the provincial assembly from 1935 to 1939. Hessian died in Lagos, Nigeria at the age of 71 while representing the province at a Commonwealth Parliamentary Association conference. References Speakers of the Legislative Assembly of Prince Edward Island Prince Edward Island Liberal Party MLAs 1891 births 1962 deaths {{PrinceEdwardIsland-politician-stub ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Patrick J
Patrick may refer to: *Patrick (given name), list of people and fictional characters with this name *Patrick (surname), list of people with this name People *Saint Patrick (c. 385–c. 461), Christian saint *Gilla Pátraic (died 1084), Patrick or Patricius, Bishop of Dublin * Patrick, 1st Earl of Salisbury (c. 1122–1168), Anglo-Norman nobleman * Patrick (footballer, born 1983), Brazilian right-back *Patrick (footballer, born 1985), Brazilian striker *Patrick (footballer, born 1992), Brazilian midfielder *Patrick (footballer, born 1994), Brazilian right-back *Patrick (footballer, born May 1998), Brazilian forward *Patrick (footballer, born November 1998), Brazilian attacking midfielder * Patrick (footballer, born 1999), Brazilian defender * Patrick (footballer, born 2000), Brazilian defender *John Byrne (Scottish playwright) (born 1940), also a painter under the pseudonym Patrick *Don Harris (wrestler) (born 1960), American professional wrestler who uses the ring name Patrick Fil ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
The Hessian
''The Hessian'' is a 1972 novel by Howard Fast set in the time of the American Revolution. Plot The book begins with an incident in 1781 when a small detachment of Hessian (German auxiliaries in the British service) soldiers encounter a mentally retarded man, Saul Clamberham, on a Connecticut hill-side. Clamberham follows the Jägers out of confused curiosity, making meaningless markings on a slate. Misunderstanding the situation, an exasperated Hessian officer, with little English, becomes convinced that Clamberham is a spy and has the autistic villager hanged from a tree. Outraged, the local population ambush the Hessians and kill all but a drummer boy who escapes. The narrator of the story: the town physician and a Continental Army The Continental Army was the army of the United Colonies (the Thirteen Colonies) in the Revolutionary-era United States. It was formed by the Second Continental Congress after the outbreak of the American Revolutionary War, and was establis ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Hessian (Web Service Protocol)
Hessian is a binary Web service protocol that makes Web services usable without requiring a large framework, and without learning a new set of protocols . Because it is a binary protocol, it is well-suited to sending binary data without any need to extend the protocol with attachments. Hessian was developed by Caucho Technology, Inc. The company has released Java, Python and ActionScript for Adobe Flash implementations of Hessian under an open source license (the Apache license). Third-party implementations in several other languages (C++, C#, JavaScript, Perl, PHP, Ruby, Objective-C, D, and Erlang) are also available as open-source. Adaptations Although Hessian is primarily intended for Web services, it can be adapted for TCP traffic by using the ''HessianInput'' and ''HessianOutput'' classes in Caucho's Java implementation. Implementations Cotton( Erlang) HessDroid( Android) Hessian (on Rubyforge)(Ruby) Hessian.js(JavaScript) Hessian4J(Java) HessianC#( C#) HessianCPP( ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Glossary Of Classical Algebraic Geometry
The terminology of algebraic geometry changed drastically during the twentieth century, with the introduction of the general methods, initiated by David Hilbert and the Italian school of algebraic geometry in the beginning of the century, and later formalized by André Weil, Jean-Pierre Serre and Alexander Grothendieck. Much of the classical terminology, mainly based on case study, was simply abandoned, with the result that books and papers written before this time can be hard to read. This article lists some of this classical terminology, and describes some of the changes in conventions. translates many of the classical terms in algebraic geometry into scheme-theoretic terminology. Other books defining some of the classical terminology include , , , , , . Conventions The change in terminology from around 1948 to 1960 is not the only difficulty in understanding classical algebraic geometry. There was also a lot of background knowledge and assumptions, much of which has now ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
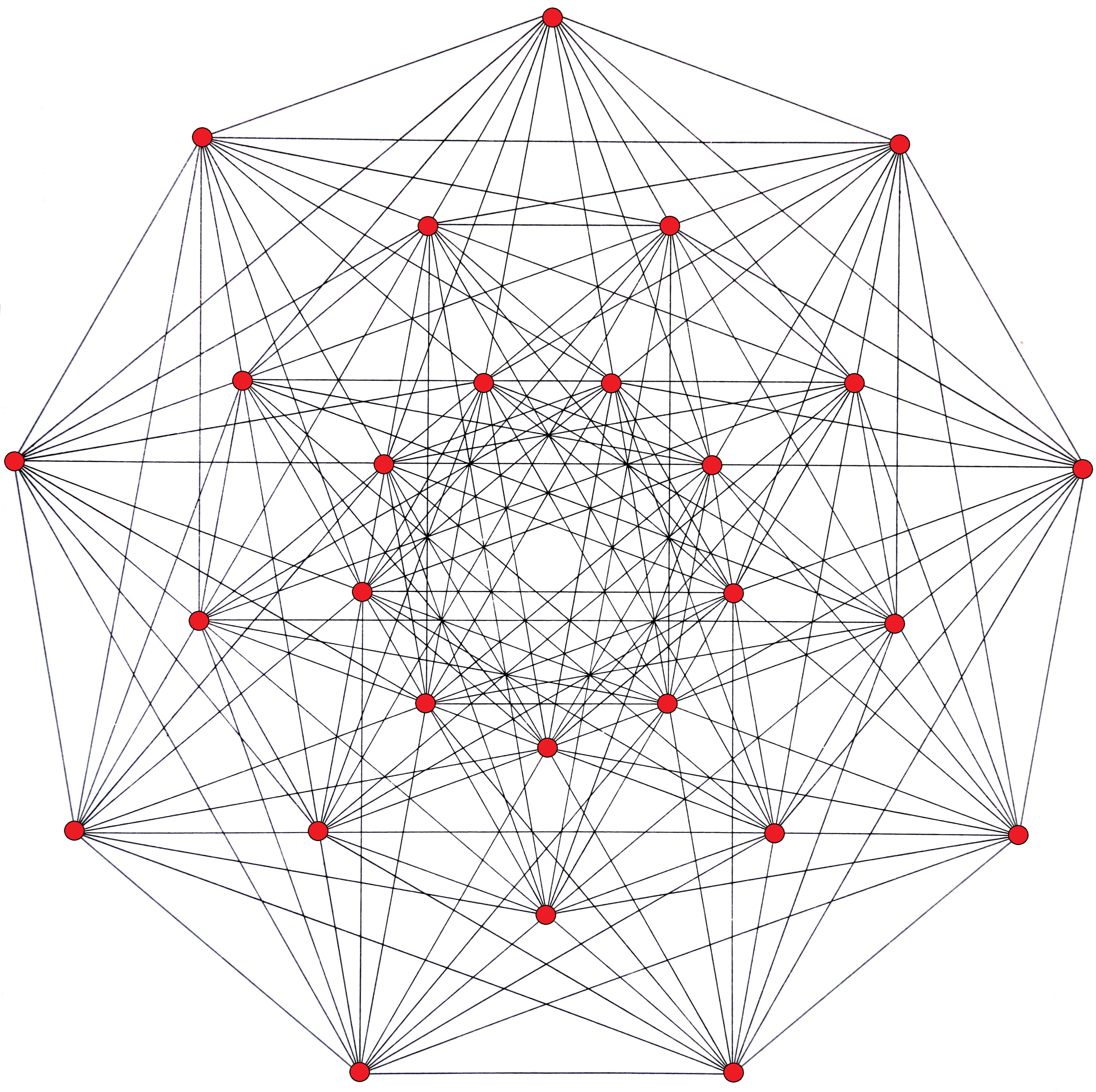
Hessian Polyhedron
In geometry, the Hessian polyhedron is a regular complex polyhedron 333, , in \mathbb^3. It has 27 vertices, 72 3 edges, and 27 33 faces. It is self-dual. Coxeter named it after Ludwig Otto Hesse for sharing the ''Hessian configuration'' \left begin 9&4\\3&12 \end\right /math> or (94123), 9 points lying by threes on twelve lines, with four lines through each point. Its complex reflection group is 3 sub>3 sub>3 or , order 648, also called a Hessian group. It has 27 copies of , order 24, at each vertex. It has 24 order-3 reflections. Its Coxeter number is 12, with degrees of the fundamental invariants 3, 6, and 12, which can be seen in projective symmetry of the polytopes. The Witting polytope, 3333, contains the Hessian polyhedron as cells and vertex figures. It has a real representation as the ''221'' polytope, , in 6-dimensional space, sharing the same 27 vertices. The 216 edges in ''221'' can be seen as the 72 3 edges represented as 3 simple edges. Coordinates Its 27 ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Hessian Group
In mathematics, the Hessian group is a finite group of order 216, introduced by who named it for Otto Hesse. It may be represented as the group of affine transformations with determinant 1 of the affine plane over the field of 3 elements.Hessian group oGroupNames/ref> It has a normal subgroup that is an elementary abelian group of order 32, and the quotient by this subgroup is isomorphic to the group SL2(3) of order 24. It also acts on the Hesse pencil of elliptic curves, and forms the automorphism group of the Hesse configuration of the 9 inflection points of these curves and the 12 lines through triples of these points. The triple cover of this group is a complex reflection group In mathematics, a complex reflection group is a finite group acting on a finite-dimensional complex vector space that is generated by complex reflections: non-trivial elements that fix a complex hyperplane pointwise. Complex reflection groups arise ..., 3 sub>3 sub>3 or of order 648, and the product of ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Hessian Form Of An Elliptic Curve
In geometry, the Hessian curve is a plane curve similar to folium of Descartes. It is named after the German mathematician Otto Hesse. This curve was suggested for application in elliptic curve cryptography, because arithmetic in this curve representation is faster and needs less memory than arithmetic in standard Weierstrass form.Cauchy-Desbove's Formulae: ''Hessian-elliptic Curves and Side-Channel Attacks'', Marc Joye and Jean-Jacques Quisquarter Definition Let K be a field and consider an elliptic curve E in the following special case of Weierstrass form over K : Y^2+a_1 XY+a_3 Y=X^3 where the curve has discriminant \Delta = \left(a_3^3 \left(a_1^3 - 27a_3\right)\right) = a_3^3 \delta. Then the point P=(0,0) has order 3. To prove that P=(0,0) has order 3, note that the tangent to E at P is the line Y=0 which intersects E with multiplicity 3 at P. Conversely, given a point P of order 3 on an elliptic curve E both defined over a field K one can put the curve into Weier ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Hessian Pair
In mathematics, a Hessian pair or Hessian duad, named for Otto Hesse, is a pair of points of the projective line canonically associated with a set of 3 points of the projective line. More generally, one can define the Hessian pair of any triple of elements from a set that can be identified with a projective line, such as a rational curve, a pencil of divisors, a pencil of lines, and so on. Definition If is a set of 3 distinct points of the projective line, then the Hessian pair is a set of two points that can be defined by any of the following properties: *''P'' and ''Q'' are the roots of the Hessian of the binary cubic form with roots ''A'', ''B'', ''C''. *''P'' and ''Q'' are the two points fixed by the unique projective transformation taking ''A'' to ''B'', ''B'' to ''C'', and ''C'' to ''A''. *''P'' and ''Q'' are the two points that when added to ''A'', ''B'', ''C'' form an equianharmonic set (a set of 4 points with cross-ratio a cube root of 1). *''P'' and ''Q'' are the ima ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Hessian Automatic Differentiation
In applied mathematics, Hessian automatic differentiation are techniques based on automatic differentiation (AD) that calculate the second derivative of an n-dimensional function, known as the Hessian matrix In mathematics, the Hessian matrix or Hessian is a square matrix of second-order partial derivatives of a scalar-valued function, or scalar field. It describes the local curvature of a function of many variables. The Hessian matrix was developed .... When examining a function in a neighborhood of a point, one can discard many complicated global aspects of the function and accurately approximate it with simpler functions. The quadratic approximation is the best-fitting quadratic in the neighborhood of a point, and is frequently used in engineering and science. To calculate the quadratic approximation, one must first calculate its gradient and Hessian matrix. Let f: \mathbb^n \rightarrow \mathbb , for each x \in \mathbb^n the Hessian matrix H(x) \in \mathbb^ is the seco ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |