|
Heaviside
Oliver Heaviside FRS (; 18 May 1850 – 3 February 1925) was an English self-taught mathematician and physicist who invented a new technique for solving differential equations (equivalent to the Laplace transform), independently developed vector calculus, and rewrote Maxwell's equations in the form commonly used today. He significantly shaped the way Maxwell's equations are understood and applied in the decades following Maxwell's death. His formulation of the telegrapher's equations became commercially important during his own lifetime, after their significance went unremarked for a long while, as few others were versed at the time in his novel methodology. Although at odds with the scientific establishment for most of his life, Heaviside changed the face of telecommunications, mathematics, and science. Biography Early life Heaviside was born in Camden Town, London, at 55 Kings Street (now Plender Street), the youngest of three children of Thomas, a draughtsman and wood engr ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Heaviside Step Function
The Heaviside step function, or the unit step function, usually denoted by or (but sometimes , or ), is a step function, named after Oliver Heaviside (1850–1925), the value of which is zero for negative arguments and one for positive arguments. It is an example of the general class of step functions, all of which can be represented as linear combinations of translations of this one. The function was originally developed in operational calculus for the solution of differential equations, where it represents a signal that switches on at a specified time and stays switched on indefinitely. Oliver Heaviside, who developed the operational calculus as a tool in the analysis of telegraphic communications, represented the function as . The Heaviside function may be defined as: * a piecewise function: H(x) := \begin 1, & x > 0 \\ 0, & x \le 0 \end * using the Iverson bracket notation: H(x) := 0.html" ;"title=">0">>0/math> * an indicator function: H(x) := \mathbf_=\mathbf 1_(x) * ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Operational Calculus
Operational calculus, also known as operational analysis, is a technique by which problems in analysis, in particular differential equations, are transformed into algebraic problems, usually the problem of solving a polynomial equation. History The idea of representing the processes of calculus, differentiation and integration, as operators has a long history that goes back to Gottfried Wilhelm Leibniz. The mathematician Louis François Antoine Arbogast was one of the first to manipulate these symbols independently of the function to which they were applied. This approach was further developed by Francois-Joseph Servois who developed convenient notations. Servois was followed by a school of British and Irish mathematicians including Charles James Hargreave, George Boole, Bownin, Carmichael, Doukin, Graves, Murphy, William Spottiswoode and Sylvester. Treatises describing the application of operator methods to ordinary and partial differential equations were written by Robert B ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Kennelly–Heaviside Layer
The Heaviside layer, sometimes called the Kennelly–Heaviside layer, named after Arthur E. Kennelly and Oliver Heaviside, is a layer of ionised gas occurring roughly between 90km and 150 km (56 and 93 mi) above the ground — one of several layers in the Earth's ionosphere. It is also known as the E region. It reflects medium-frequency radio waves. Because of this reflective layer, radio waves radiated into the sky can return to Earth beyond the horizon. This "skywave" or "skip" propagation technique has been used since the 1920s for radio communication at long distances, up to transcontinental distances. Propagation is affected by the time of day. During the daytime the solar wind presses this layer closer to the Earth, thereby limiting how far it can reflect radio waves. Conversely, on the night ( lee) side of the Earth, the solar wind drags the ionosphere further away, thereby greatly increasing the range which radio waves can travel by reflection. The exten ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Heaviside Condition
The Heaviside condition, named for Oliver Heaviside (1850–1925), is the condition an electrical transmission line must meet in order for there to be no distortion of a transmitted signal. Also known as the distortionless condition, it can be used to improve the performance of a transmission line by adding loading to the cable. The condition A transmission line can be represented as a distributed-element model of its primary line constants as shown in the figure. The primary constants are the electrical properties of the cable per unit length and are: capacitance ''C'' (in farads per meter), inductance ''L'' (in henries per meter), series resistance ''R'' (in ohms per meter), and shunt conductance ''G'' (in siemens per meter). The series resistance and shunt conductivity cause losses in the line; for an ideal transmission line, \scriptstyle R=G=0. The Heaviside condition is satisfied when :\frac = \frac. This condition is for no distortion, but not for no loss. Backgro ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Laplace Transform
In mathematics, the Laplace transform, named after its discoverer Pierre-Simon Laplace (), is an integral transform In mathematics, an integral transform maps a function from its original function space into another function space via integration, where some of the properties of the original function might be more easily characterized and manipulated than in ... that converts a Function (mathematics), function of a Real number, real Variable (mathematics), variable (usually t, in the ''time domain'') to a function of a Complex number, complex variable s (in the complex frequency domain, also known as ''s''-domain, or s-plane). The transform has many applications in science and engineering because it is a tool for solving differential equations. In particular, it transforms ordinary differential equations into algebraic equations and convolution into multiplication. For suitable functions ''f'', the Laplace transform is the integral \mathcal\(s) = \int_0^\infty f(t)e^ \, dt. H ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
History Of Special Relativity
The history of special relativity consists of many theoretical results and empirical findings obtained by Albert A. Michelson, Hendrik Lorentz, Henri Poincaré and others. It culminated in the theory of special relativity proposed by Albert Einstein and subsequent work of Max Planck, Hermann Minkowski and others. Introduction Although Isaac Newton based his physics on absolute time and space, he also adhered to the principle of relativity of Galileo Galilei restating it precisely for mechanical systems. This can be stated as: as far as the laws of mechanics are concerned, all observers in inertial motion are equally privileged, and no preferred state of motion can be attributed to any particular inertial observer. However, as to electromagnetic theory and electrodynamics, during the 19th century the wave theory of light as a disturbance of a "light medium" or Luminiferous ether was widely accepted, the theory reaching its most developed form in the work of James Clerk Maxwell. Ac ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
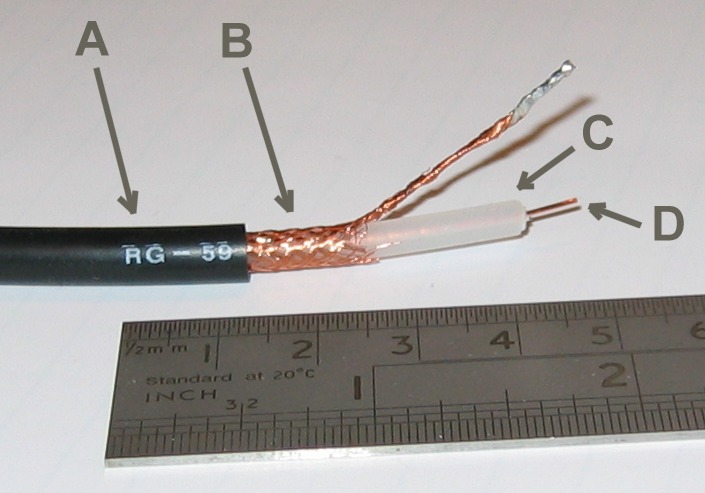Coaxial Cable
Coaxial cable, or coax (pronounced ) is a type of electrical cable consisting of an inner conductor surrounded by a concentric conducting shield, with the two separated by a dielectric ( insulating material); many coaxial cables also have a protective outer sheath or jacket. The term ''coaxial'' refers to the inner conductor and the outer shield sharing a geometric axis. Coaxial cable is a type of transmission line, used to carry high-frequency electrical signals with low losses. It is used in such applications as telephone trunk lines, broadband internet networking cables, high-speed computer data busses, cable television signals, and connecting radio transmitters and receivers to their antennas. It differs from other shielded cables because the dimensions of the cable and connectors are controlled to give a precise, constant conductor spacing, which is needed for it to function efficiently as a transmission line. Coaxial cable was used in the first (1858) and followin ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Maxwell's Equations
Maxwell's equations, or Maxwell–Heaviside equations, are a set of coupled partial differential equations that, together with the Lorentz force law, form the foundation of classical electromagnetism, classical optics, and electric circuits. The equations provide a mathematical model for electric, optical, and radio technologies, such as power generation, electric motors, wireless communication, lenses, radar etc. They describe how electric and magnetic fields are generated by charges, currents, and changes of the fields.''Electric'' and ''magnetic'' fields, according to the theory of relativity, are the components of a single electromagnetic field. The equations are named after the physicist and mathematician James Clerk Maxwell, who, in 1861 and 1862, published an early form of the equations that included the Lorentz force law. Maxwell first used the equations to propose that light is an electromagnetic phenomenon. The modern form of the equations in their most common formul ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Energy Current
Energy current is a flow of energy defined by the Poynting vector (), as opposed to normal current (flow of charge). It was originally postulated by Oliver Heaviside. It is also an informal name for Energy flux. Explanation "Energy current" is a somewhat informal term that is used, on occasion, to describe the process of energy transfer in situations where the transfer can usefully be viewed in terms of a flow. It is particularly used when the transfer of energy is more significant to the discussion than the process by which the energy is transferred. For instance, the flow of fuel oil in a pipeline could be considered as an energy current, although this would not be a convenient way of visualising the fullness of the storage tanks. The units of energy current are those of power ( W). This is closely related to energy flux, which is the energy transferred per unit area per unit time (measured in W/m). Energy current in electromagnetism A specific use of the concept of energ ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Jefimenko's Equations
In electromagnetism, Jefimenko's equations (named after Oleg D. Jefimenko) give the electric field and magnetic field due to a distribution of electric charges and electric current in space, that takes into account the propagation delay (retarded time) of the fields due to the finite speed of light and relativistic effects. Therefore they can be used for ''moving'' charges and currents. They are the particular solutions to Maxwell's equations for any arbitrary distribution of charges and currents. Equations Electric and magnetic fields Jefimenko's equations give the electric field E and magnetic field B produced by an arbitrary charge or current distribution, of charge density ''ρ'' and current density J:Introduction to Electrodynamics (3rd Edition), D. J. Griffiths, Pearson Education, Dorling Kindersley, 2007, . \mathbf(\mathbf, t) = \frac \int \left frac\rho(\mathbf', t_r) + \frac\frac\frac - \frac\frac\frac \rightdV', \mathbf(\mathbf, t) = -\frac \int \left frac \times \ ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Gravitoelectromagnetism
Gravitoelectromagnetism, abbreviated GEM, refers to a set of formal analogies between the equations for electromagnetism and relativistic gravitation; specifically: between Maxwell's field equations and an approximation, valid under certain conditions, to the Einstein field equations for general relativity. Gravitomagnetism is a widely used term referring specifically to the kinetic effects of gravity, in analogy to the magnetic effects of moving electric charge. The most common version of GEM is valid only far from isolated sources, and for slowly moving test particles. The analogy and equations differing only by some small factors were first published in 1893, before general relativity, by Oliver Heaviside as a separate theory expanding Newton's law. Background This approximate reformulation of gravitation as described by general relativity in the weak field limit makes an apparent field appear in a frame of reference different from that of a freely moving inertial body. ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Heaviside Cover-up Method
The Heaviside cover-up method, named after Oliver Heaviside, is one possible approach in determining the coefficients when performing the partial-fraction expansion of a rational function.Calculus and Analytic Geometry 7th Edition, Thomas/Finney, 1988, pp. 482-489 Method Separation of a fractional algebraic expression into partial fractions is the reverse of the process of combining fractions by converting each fraction to the lowest common denominator (LCD) and adding the numerators. This separation can be accomplished by the Heaviside cover-up method, another method for determining the coefficients of a partial fraction. Case one has fractional expressions where factors in the denominator are unique. Case two has fractional expressions where some factors may repeat as powers of a binomial. In integral calculus we would want to write a fractional algebraic expression as the sum of its partial fractions in order to take the integral of each simple fraction separately. Once th ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
