|
Glissette
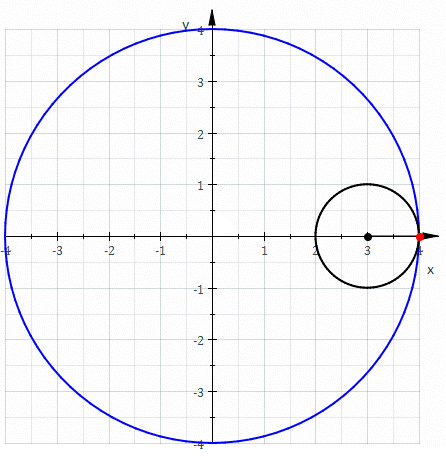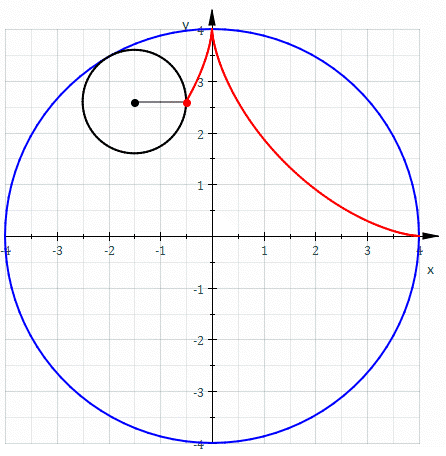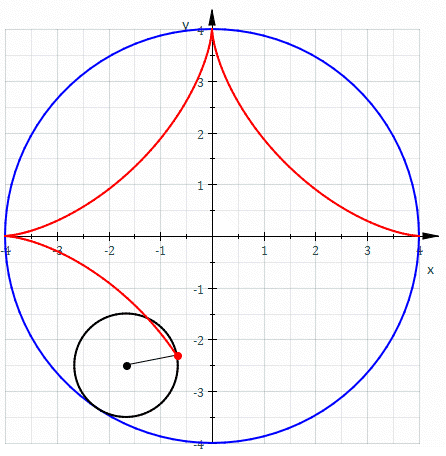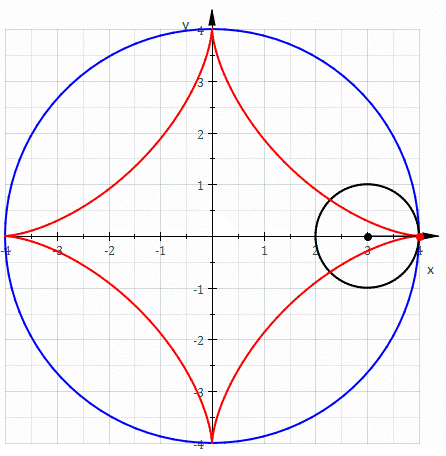
In geometry, a glissette is a curve determined by either the locus of any point, or the envelope of any line or curve, that is attached to a curve that slides against or along two other fixed curves. Examples Ellipse A basic example is that of a line segment of which the endpoints slide along two perpendicular lines. The glissette of any point on the line forms an ellipse. Astroid Similarly, the envelope glissette of the line segment in the example above is an astroid In mathematics, an astroid is a particular type of roulette curve: a hypocycloid with four cusps. Specifically, it is the locus of a point on a circle as it rolls inside a fixed circle with four times the radius. By double generation, it .... Conchoid Any conchoid may be regarded as a glissette, with a line and one of its points sliding along a given line and fixed point. References {{Reflist External links Glissette at Wolfram Mathworld Curves ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Glissette Ellipse
In geometry, a glissette is a curve determined by either the locus of any point, or the envelope of any line or curve, that is attached to a curve that slides against or along two other fixed curves. Examples Ellipse A basic example is that of a line segment of which the endpoints slide along two perpendicular lines. The glissette of any point on the line forms an ellipse In mathematics, an ellipse is a plane curve surrounding two focus (geometry), focal points, such that for all points on the curve, the sum of the two distances to the focal points is a constant. It generalizes a circle, which is the special ty .... Astroid Similarly, the envelope glissette of the line segment in the example above is an astroid. Conchoid Any conchoid may be regarded as a glissette, with a line and one of its points sliding along a given line and fixed point. References {{Reflist External links Glissette at Wolfram Mathworld Curves ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Geometry
Geometry (; ) is, with arithmetic, one of the oldest branches of mathematics. It is concerned with properties of space such as the distance, shape, size, and relative position of figures. A mathematician who works in the field of geometry is called a '' geometer''. Until the 19th century, geometry was almost exclusively devoted to Euclidean geometry, which includes the notions of point, line, plane, distance, angle, surface, and curve, as fundamental concepts. During the 19th century several discoveries enlarged dramatically the scope of geometry. One of the oldest such discoveries is Carl Friedrich Gauss' ("remarkable theorem") that asserts roughly that the Gaussian curvature of a surface is independent from any specific embedding in a Euclidean space. This implies that surfaces can be studied ''intrinsically'', that is, as stand-alone spaces, and has been expanded into the theory of manifolds and Riemannian geometry. Later in the 19th century, it appeared that geom ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Curve
In mathematics, a curve (also called a curved line in older texts) is an object similar to a line, but that does not have to be straight. Intuitively, a curve may be thought of as the trace left by a moving point. This is the definition that appeared more than 2000 years ago in Euclid's ''Elements'': "The urvedline is ��the first species of quantity, which has only one dimension, namely length, without any width nor depth, and is nothing else than the flow or run of the point which ��will leave from its imaginary moving some vestige in length, exempt of any width." This definition of a curve has been formalized in modern mathematics as: ''A curve is the image of an interval to a topological space by a continuous function''. In some contexts, the function that defines the curve is called a ''parametrization'', and the curve is a parametric curve. In this article, these curves are sometimes called ''topological curves'' to distinguish them from more constrained curves su ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Locus (mathematics)
In geometry, a locus (plural: ''loci'') (Latin word for "place", "location") is a set of all points (commonly, a line, a line segment, a curve or a surface), whose location satisfies or is determined by one or more specified conditions.. In other words, the set of the points that satisfy some property is often called the ''locus of a point'' satisfying this property. The use of the singular in this formulation is a witness that, until the end of the 19th century, mathematicians did not consider infinite sets. Instead of viewing lines and curves as sets of points, they viewed them as places where a point may be ''located'' or may move. History and philosophy Until the beginning of the 20th century, a geometrical shape (for example a curve) was not considered as an infinite set of points; rather, it was considered as an entity on which a point may be located or on which it moves. Thus a circle in the Euclidean plane was defined as the ''locus'' of a point that is at a given dist ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Envelope (mathematics)
In geometry, an envelope of a planar family of curves is a curve that is tangent to each member of the family at some point, and these points of tangency together form the whole envelope. Classically, a point on the envelope can be thought of as the intersection of two " infinitesimally adjacent" curves, meaning the limit of intersections of nearby curves. This idea can be generalized to an envelope of surfaces in space, and so on to higher dimensions. To have an envelope, it is necessary that the individual members of the family of curves are differentiable curves as the concept of tangency does not apply otherwise, and there has to be a smooth transition proceeding through the members. But these conditions are not sufficient – a given family may fail to have an envelope. A simple example of this is given by a family of concentric circles of expanding radius. Envelope of a family of curves Let each curve ''C''''t'' in the family be given as the solution of an equation '' ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Ellipse
In mathematics, an ellipse is a plane curve surrounding two focal points, such that for all points on the curve, the sum of the two distances to the focal points is a constant. It generalizes a circle, which is the special type of ellipse in which the two focal points are the same. The elongation of an ellipse is measured by its eccentricity e, a number ranging from e = 0 (the limiting case of a circle) to e = 1 (the limiting case of infinite elongation, no longer an ellipse but a parabola). An ellipse has a simple algebraic solution for its area, but only approximations for its perimeter (also known as circumference), for which integration is required to obtain an exact solution. Analytically, the equation of a standard ellipse centered at the origin with width 2a and height 2b is: : \frac+\frac = 1 . Assuming a \ge b, the foci are (\pm c, 0) for c = \sqrt. The standard parametric equation is: : (x,y) = (a\cos(t),b\sin(t)) \quad \text \quad 0\leq t\leq 2\pi. Ellip ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Astroid
In mathematics, an astroid is a particular type of roulette curve: a hypocycloid with four cusps. Specifically, it is the locus of a point on a circle as it rolls inside a fixed circle with four times the radius. By double generation, it is also the locus of a point on a circle as it rolls inside a fixed circle with 4/3 times the radius. It can also be defined as the envelope of a line segment of fixed length that moves while keeping an end point on each of the axes. It is therefore the envelope of the moving bar in the Trammel of Archimedes. Its modern name comes from the Greek word for "star". It was proposed, originally in the form of "Astrois", by Joseph Johann von Littrow in 1838. The curve had a variety of names, including tetracuspid (still used), cubocycloid, and paracycle. It is nearly identical in form to the evolute of an ellipse. Equations If the radius of the fixed circle is ''a'' then the equation is given by :x^ + y^ = a^. \, This implies that an astroid ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Astroid Glissette
In mathematics, an astroid is a particular type of roulette curve: a hypocycloid with four cusps. Specifically, it is the locus of a point on a circle as it rolls inside a fixed circle with four times the radius. By double generation, it is also the locus of a point on a circle as it rolls inside a fixed circle with 4/3 times the radius. It can also be defined as the envelope of a line segment of fixed length that moves while keeping an end point on each of the axes. It is therefore the envelope of the moving bar in the Trammel of Archimedes. Its modern name comes from the Greek word for "star". It was proposed, originally in the form of "Astrois", by Joseph Johann von Littrow in 1838. The curve had a variety of names, including tetracuspid (still used), cubocycloid, and paracycle. It is nearly identical in form to the evolute of an ellipse. Equations If the radius of the fixed circle is ''a'' then the equation is given by :x^ + y^ = a^. \, This implies that an astroid is ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Conchoid (mathematics)
In geometry, a conchoid is a curve derived from a fixed point , another curve, and a length . It was invented by the ancient Greek mathematician Nicomedes. Description For every line through that intersects the given curve at the two points on the line which are from are on the conchoid. The conchoid is, therefore, the cissoid of the given curve and a circle of radius and center . They are called ''conchoids'' because the shape of their outer branches resembles conch shells. The simplest expression uses polar coordinates with at the origin. If :r=\alpha(\theta) expresses the given curve, then :r=\alpha(\theta)\pm d expresses the conchoid. If the curve is a line, then the conchoid is the ''conchoid of Nicomedes''. For instance, if the curve is the line , then the line's polar form is and therefore the conchoid can be expressed parametrically as :x=a \pm d \cos \theta,\, y=a \tan \theta \pm d \sin \theta. A limaçon is a conchoid with a circle as the given curv ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Nicomedes , 94–74 BC
{{hndis ...
Nicomedes may refer to: *Nicomedes (mathematician), ancient Greek mathematician who discovered the conchoid *Nicomedes of Sparta, regent during the youth of King Pleistoanax, commanded the Spartan army at the Battle of Tanagra (457 BC) *Saint Nicomedes, Martyr of unknown era, whose feast is observed 15 September Four kings of Bithynia in Anatolia, 3rd–1st century BC: *Nicomedes I of Bithynia, ruled 278–255 BC *Nicomedes II of Bithynia, 149–127 BC *Nicomedes III of Bithynia, 127–94 BC *Nicomedes IV of Bithynia Nicomedes IV Philopator ( grc-gre, Νικομήδης Φιλοπάτωρ) was the king of Bithynia from c. 94 BC to 74 BC. (''numbered as III. not IV.'') He was the first son and successor of Nicomedes III of Bithynia. Life Memnon of Heraclea wro ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |