|
Fractal Dimension On Networks
Fractal analysis is useful in the study of complex networks, present in both natural and artificial systems such as computer systems, brain and social networks, allowing further development of the field in network science. Self-similarity of complex networks Many real networks have two fundamental properties, scale-free property and small-world property. If the degree distribution of the network follows a power-law, the network is scale-free; if any two arbitrary nodes in a network can be connected in a very small number of steps, the network is said to be small-world. The small-world properties can be mathematically expressed by the slow increase of the average diameter of the network, with the total number of nodes N, \left\langle l\right\rangle\sim\ln where l is the shortest distance between two nodes. Equivalently, we obtain: N\sim e^ where l_0 is a characteristic length. For a self-similar structure, a power-law relation is expected rather than the exponential r ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Fractal Analysis
Fractal analysis is assessing fractal characteristics of data. It consists of several methods to assign a fractal dimension and other fractal characteristics to a dataset which may be a theoretical dataset, or a pattern or signal extracted from phenomena including topography, natural geometric objects, ecology and aquatic sciences, sound, market fluctuations, heart rates, frequency domain in electroencephalography signals, digital images, molecular motion, and data science. Fractal analysis is now widely used in all areas of science. An important limitation of fractal analysis is that arriving at an empirically determined fractal dimension does not necessarily prove that a pattern is fractal; rather, other essential characteristics have to be considered. Fractal analysis is valuable in expanding our knowledge of the structure and function of various systems, and as a potential tool to mathematically assess novel areas of study. Fractal calculus was formulated which is a generaliz ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Box Counting
Box counting is a method of gathering data for analyzing complex patterns by breaking a dataset, object, image, etc. into smaller and smaller pieces, typically "box"-shaped, and analyzing the pieces at each smaller scale. The essence of the process has been compared to zooming in or out using optical or computer based methods to examine how observations of detail change with scale. In box counting, however, rather than changing the magnification or resolution of a lens, the investigator changes the size of the element used to inspect the object or pattern (see Figure 1). Computer based box counting algorithms have been applied to patterns in 1-, 2-, and 3-dimensional spaces. The technique is usually implemented in software for use on patterns extracted from digital media, although the fundamental method can be used to investigate some patterns physically. The technique arose out of and is used in fractal analysis. It also has application in related fields such as lacunarity an ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Modern Physics Letters B
''Modern Physics Letters B'' is a peer-reviewed scientific journal on physics, especially the areas of condensed matter, statistical and applied physics, and high-Tc superconductivity. It was established in 1987 and is published by World Scientific. Related journals * ''Modern Physics Letters A'' Abstracting and indexing The journal is abstracted and indexed in: * Science Citation Index * Materials Science Citation Index * Current Contents/Physical, Chemical & Earth Sciences * Astrophysics Data System * Mathematical Reviews * Inspec * CSA Meteorological & Geoastrophysical Abstracts * Scopus According to the ''Journal Citation Reports'', the journal has a 2020 impact factor The impact factor (IF) or journal impact factor (JIF) of an academic journal is a scientometric index calculated by Clarivate that reflects the yearly mean number of citations of articles published in the last two years in a given journal, as i ... of 1.668. References External links * {{Official ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Metric Dimension (graph Theory)
In graph theory, the metric dimension of a graph ''G'' is the minimum cardinality of a subset ''S'' of vertices such that all other vertices are uniquely determined by their distances to the vertices in ''S''. Finding the metric dimension of a graph is an NP-hard problem; the decision version, determining whether the metric dimension is less than a given value, is NP-complete. Detailed definition For an ordered subset W = \ of vertices and a vertex ''v'' in a connected graph ''G'', the representation of ''v'' with respect to ''W'' is the ordered ''k''-tuple r(v, W) = (d(v,w_1), d(v,w_2),\dots,d(v,w_k)), where ''d''(''x'',''y'') represents the distance between the vertices ''x'' and ''y''. The set ''W'' is a resolving set (or locating set) for ''G'' if every two vertices of ''G'' have distinct representations. The metric dimension of ''G'' is the minimum cardinality of a resolving set for ''G''. A resolving set containing a minimum number of vertices is called a basis (or reference se ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Graph Theory
In mathematics, graph theory is the study of '' graphs'', which are mathematical structures used to model pairwise relations between objects. A graph in this context is made up of '' vertices'' (also called ''nodes'' or ''points'') which are connected by ''edges'' (also called ''links'' or ''lines''). A distinction is made between undirected graphs, where edges link two vertices symmetrically, and directed graphs, where edges link two vertices asymmetrically. Graphs are one of the principal objects of study in discrete mathematics. Definitions Definitions in graph theory vary. The following are some of the more basic ways of defining graphs and related mathematical structures. Graph In one restricted but very common sense of the term, a graph is an ordered pair G=(V,E) comprising: * V, a set of vertices (also called nodes or points); * E \subseteq \, a set of edges (also called links or lines), which are unordered pairs of vertices (that is, an edge is associated with t ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Fractal Scaling Analysis Of WWW Network
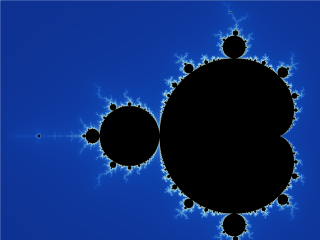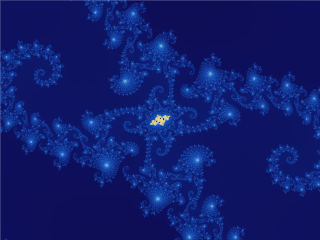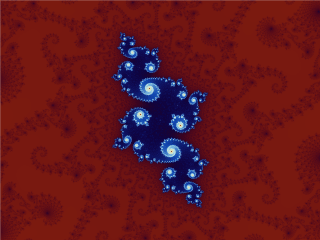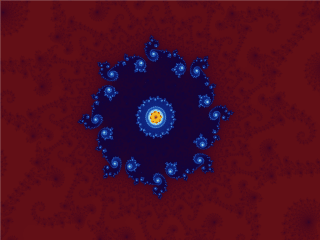
In mathematics, a fractal is a geometric shape containing detailed structure at arbitrarily small scales, usually having a fractal dimension strictly exceeding the topological dimension. Many fractals appear similar at various scales, as illustrated in successive magnifications of the Mandelbrot set. This exhibition of similar patterns at increasingly smaller scales is called self-similarity, also known as expanding symmetry or unfolding symmetry; if this replication is exactly the same at every scale, as in the Menger sponge, the shape is called affine self-similar. Fractal geometry lies within the mathematical branch of measure theory. One way that fractals are different from finite geometric figures is how they scale. Doubling the edge lengths of a filled polygon multiplies its area by four, which is two (the ratio of the new to the old side length) raised to the power of two (the conventional dimension of the filled polygon). Likewise, if the radius of a filled sphere is ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Centrality
In graph theory and network analysis, indicators of centrality assign numbers or rankings to nodes within a graph corresponding to their network position. Applications include identifying the most influential person(s) in a social network, key infrastructure nodes in the Internet or urban networks, super-spreaders of disease, and brain networks. Centrality concepts were first developed in social network analysis, and many of the terms used to measure centrality reflect their sociological origin.Newman, M.E.J. 2010. ''Networks: An Introduction.'' Oxford, UK: Oxford University Press. Definition and characterization of centrality indices Centrality indices are answers to the question "What characterizes an important vertex?" The answer is given in terms of a real-valued function on the vertices of a graph, where the values produced are expected to provide a ranking which identifies the most important nodes. The word "importance" has a wide number of meanings, leading to man ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Fractal
In mathematics, a fractal is a geometric shape containing detailed structure at arbitrarily small scales, usually having a fractal dimension strictly exceeding the topological dimension. Many fractals appear similar at various scales, as illustrated in successive magnifications of the Mandelbrot set. This exhibition of similar patterns at increasingly smaller scales is called self-similarity, also known as expanding symmetry or unfolding symmetry; if this replication is exactly the same at every scale, as in the Menger sponge, the shape is called affine self-similar. Fractal geometry lies within the mathematical branch of measure theory. One way that fractals are different from finite geometric figures is how they scale. Doubling the edge lengths of a filled polygon multiplies its area by four, which is two (the ratio of the new to the old side length) raised to the power of two (the conventional dimension of the filled polygon). Likewise, if the radius of a filled s ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Skeleton Of A Network
A skeleton is the structural frame that supports the body of an animal. There are several types of skeletons, including the exoskeleton, which is the stable outer shell of an organism, the endoskeleton, which forms the support structure inside the body, and the hydroskeleton, a flexible internal skeleton supported by fluid pressure. Vertebrates are animals with a vertebral column, and their skeletons are typically composed of bone and cartilage. Invertebrates are animals that lack a vertebral column. The skeletons of invertebrates vary, including hard exoskeleton shells, plated endoskeletons, or spicules. Cartilage is a rigid connective tissue that is found in the skeletal systems of vertebrates and invertebrates. Etymology The term ''skeleton'' comes . ''Sceleton'' is an archaic form of the word. Classification Skeletons can be defined by several attributes. Solid skeletons consist of hard substances, such as bone, cartilage, or cuticle. These can be further divided by locat ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Box Counting
Box counting is a method of gathering data for analyzing complex patterns by breaking a dataset, object, image, etc. into smaller and smaller pieces, typically "box"-shaped, and analyzing the pieces at each smaller scale. The essence of the process has been compared to zooming in or out using optical or computer based methods to examine how observations of detail change with scale. In box counting, however, rather than changing the magnification or resolution of a lens, the investigator changes the size of the element used to inspect the object or pattern (see Figure 1). Computer based box counting algorithms have been applied to patterns in 1-, 2-, and 3-dimensional spaces. The technique is usually implemented in software for use on patterns extracted from digital media, although the fundamental method can be used to investigate some patterns physically. The technique arose out of and is used in fractal analysis. It also has application in related fields such as lacunarity an ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Self-similarity
__NOTOC__ In mathematics, a self-similar object is exactly or approximately similar to a part of itself (i.e., the whole has the same shape as one or more of the parts). Many objects in the real world, such as coastlines, are statistically self-similar: parts of them show the same statistical properties at many scales. Self-similarity is a typical property of fractals. Scale invariance is an exact form of self-similarity where at any magnification there is a smaller piece of the object that is similar to the whole. For instance, a side of the Koch snowflake is both symmetrical and scale-invariant; it can be continually magnified 3x without changing shape. The non-trivial similarity evident in fractals is distinguished by their fine structure, or detail on arbitrarily small scales. As a counterexample, whereas any portion of a straight line may resemble the whole, further detail is not revealed. A time developing phenomenon is said to exhibit self-similarity if the numerica ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |