|
Facility Location (competitive Game)
The competitive facility location game is a kind of competitive game in which service-providers select locations to place their facilities in order to maximize their profits.Eva Tardos and Tom Wexler, "Network Formation Games". Chapter 19 in The game has the following components: * There are several consumers who need a certain service, e.g, electricity connection. * There are several producers that can supply this service, e.g, electricity companies. * Each producer can build its facility (e.g, a power station) in one of several locations. * For every pair of consumer (C) and location (L), there is a fixed cost of serving C from L (e.g, depending on the distance between the power station and the consumer's house). This cost is denoted Cost ,L The game is a sequential game with three steps: # Each producer selects a location for placing its facility. # Each producer set a price for each user (price discrimination is allowed, since there is a different cost for serving different con ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Competitive Game
Competition is a rivalry where two or more parties strive for a common goal which cannot be shared: where one's gain is the other's loss (an example of which is a zero-sum game). Competition can arise between entities such as organisms, individuals, economic and social groups, etc. The rivalry can be over attainment of any exclusive goal, including recognition: Competition occurs in nature, between living organisms which co-exist in the same environment. Animals compete over water supplies, food, mates, and other biological resources. Humans usually compete for food and mates, though when these needs are met deep rivalries often arise over the pursuit of wealth, power, prestige, and fame when in a static, repetitive, or unchanging environment. Competition is a major tenet of market economies and business, often associated with business competition as companies are in competition with at least one other firm over the same group of customers. Competition inside a company is usu ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Sequential Game
In game theory, a sequential game is a game where one player chooses their action before the others choose theirs. The other players must have information on the first player's choice so that the difference in time has no strategic effect. Sequential games are governed by the time axis and represented in the form of decision trees. Sequential games with perfect information can be analysed mathematically using combinatorial game theory. Decision trees are the extensive form of dynamic games that provide information on the possible ways that a given game can be played. They show the sequence in which players act and the number of times that they can each make a decision. Decision trees also provide information on what each player knows or does not know at the point in time they decide on an action to take. Payoffs for each player are given at the decision nodes of the tree. Extensive form representations were introduced by Neumann and further developed by Kuhn in the earliest yea ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Price Discrimination
Price discrimination is a microeconomic pricing strategy where identical or largely similar goods or services are sold at different prices by the same provider in different markets. Price discrimination is distinguished from product differentiation by the more substantial difference in production cost for the differently priced products involved in the latter strategy. Price differentiation essentially relies on the variation in the customers' willingness to payApollo, M. (2014). Dual Pricing–Two Points of View (Citizen and Non-citizen) Case of Entrance Fees in Tourist Facilities in Nepal. Procedia - Social and Behavioral Sciences, 120, 414-422. https://doi.org/10.1016/j.sbspro.2014.02.119 and in the elasticity of their demand. For price discrimination to succeed, a firm must have market power, such as a dominant market share, product uniqueness, sole pricing power, etc. All prices under price discrimination are higher than the equilibrium price in a perfectly-competitive m ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Backward Induction
Backward induction is the process of reasoning backwards in time, from the end of a problem or situation, to determine a sequence of optimal actions. It proceeds by examining the last point at which a decision is to be made and then identifying what action would be most optimal at that moment. Using this information, one can then determine what to do at the second-to-last time of decision. This process continues backwards until one has determined the best action for every possible situation (i.e. for every possible information set) at every point in time. Backward induction was first used in 1875 by Arthur Cayley, who uncovered the method while trying to solve the infamous Secretary problem. In the mathematical optimization method of dynamic programming, backward induction is one of the main methods for solving the Bellman equation. In game theory, backward induction is a method used to compute subgame perfect equilibria in sequential games. The only difference is that optimi ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Potential Game
In game theory, a game is said to be a potential game if the incentive of all players to change their strategy can be expressed using a single global function called the potential function. The concept originated in a 1996 paper by Dov Monderer and Lloyd Shapley. The properties of several types of potential games have since been studied. Games can be either ''ordinal'' or ''cardinal'' potential games. In cardinal games, the difference in individual payoffs for each player from individually changing one's strategy, other things equal, has to have the same value as the difference in values for the potential function. In ordinal games, only the signs of the differences have to be the same. The potential function is a useful tool to analyze equilibrium properties of games, since the incentives of all players are mapped into one function, and the set of pure Nash equilibria can be found by locating the local optima of the potential function. Convergence and finite-time convergence o ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Nash Equilibrium
In game theory, the Nash equilibrium, named after the mathematician John Nash, is the most common way to define the solution of a non-cooperative game involving two or more players. In a Nash equilibrium, each player is assumed to know the equilibrium strategies of the other players, and no one has anything to gain by changing only one's own strategy. The principle of Nash equilibrium dates back to the time of Cournot, who in 1838 applied it to competing firms choosing outputs. If each player has chosen a strategy an action plan based on what has happened so far in the game and no one can increase one's own expected payoff by changing one's strategy while the other players keep their's unchanged, then the current set of strategy choices constitutes a Nash equilibrium. If two players Alice and Bob choose strategies A and B, (A, B) is a Nash equilibrium if Alice has no other strategy available that does better than A at maximizing her payoff in response to Bob choosing B, and ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Subgame Perfect Equilibrium
In game theory, a subgame perfect equilibrium (or subgame perfect Nash equilibrium) is a refinement of a Nash equilibrium used in dynamic games. A strategy profile is a subgame perfect equilibrium if it represents a Nash equilibrium of every subgame of the original game. Informally, this means that at any point in the game, the players' behavior from that point onward should represent a Nash equilibrium of the continuation game (i.e. of the subgame), no matter what happened before. Every finite extensive game with perfect recall has a subgame perfect equilibrium. Perfect recall is a term introduced by Harold W. Kuhn in 1953 and ''"equivalent to the assertion that each player is allowed by the rules of the game to remember everything he knew at previous moves and all of his choices at those moves"''. A common method for determining subgame perfect equilibria in the case of a finite game is backward induction. Here one first considers the last actions of the game and determ ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
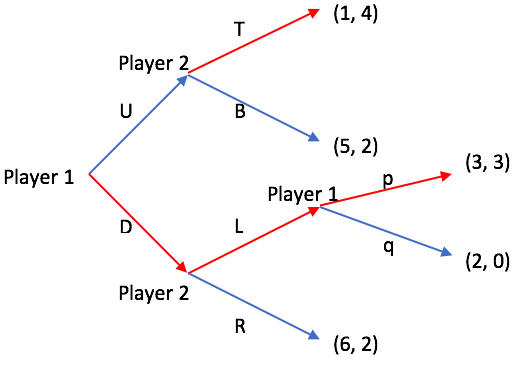
Price Of Stability
In game theory, the price of stability (PoS) of a game is the ratio between the best objective function value of one of its equilibria and that of an optimal outcome. The PoS is relevant for games in which there is some objective authority that can influence the players a bit, and maybe help them converge to a good Nash equilibrium. When measuring how efficient a Nash equilibrium is in a specific game we often also talk about the price of anarchy (PoA), which is the ratio between the ''worst'' objective function value of one of its equilibria and that of an optimal outcome. Examples Another way of expressing PoS is: : \text = \frac ,\ \text \geq 0. In particular, if the optimal solution is a Nash equilibrium, then the PoS is 1. In the following prisoner’s dilemma game, since there is a single equilibrium (B,R) we have PoS = PoA = 1/2. On this example which is a version of the battle of sexes game, there are two equilibrium points, (T,L) and (B,R), wi ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Price Of Anarchy
The Price of Anarchy (PoA) is a concept in economics and game theory that measures how the efficiency of a system degrades due to selfish behavior of its agents. It is a general notion that can be extended to diverse systems and notions of efficiency. For example, consider the system of transportation of a city and many agents trying to go from some initial location to a destination. Let efficiency in this case mean the average time for an agent to reach the destination. In the 'centralized' solution, a central authority can tell each agent which path to take in order to minimize the average travel time. In the 'decentralized' version, each agent chooses its own path. The Price of Anarchy measures the ratio between average travel time in the two cases. Usually the system is modeled as a game and the efficiency is some function of the outcomes (e.g. maximum delay in a network, congestion in a transportation system, social welfare in an auction, etc.). Different concepts of equilibr ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Utility Game
As a topic of economics, utility is used to model worth or value. Its usage has evolved significantly over time. The term was introduced initially as a measure of pleasure or happiness as part of the theory of utilitarianism by moral philosophers such as Jeremy Bentham and John Stuart Mill. The term has been adapted and reapplied within neoclassical economics, which dominates modern economic theory, as a utility function that represents a single consumer's preference ordering over a choice set but is not comparable across consumers. This concept of utility is personal and based on choice rather than on pleasure received, and so is specified more rigorously than the original concept but makes it less useful (and controversial) for ethical decisions. Utility function Consider a set of alternatives among which a person can make a preference ordering. The utility obtained from these alternatives is an unknown function of the utilities obtained from each alternative, not the sum o ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Facility Location (optimization Problem)
The study of facility location problems (FLP), also known as location analysis, is a branch of operations research and computational geometry concerned with the optimal placement of facilities to minimize transportation costs while considering factors like avoiding placing hazardous materials near housing, and competitors' facilities. The techniques also apply to cluster analysis. Minimum facility location A simple facility location problem is the Weber problem, in which a single facility is to be placed, with the only optimization criterion being the minimization of the weighted sum of distances from a given set of point sites. More complex problems considered in this discipline include the placement of multiple facilities, constraints on the locations of facilities, and more complex optimization criteria. In a basic formulation, the facility location problem consists of a set of potential facility sites ''L'' where a facility can be opened, and a set of demand points ''D'' that ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Facility Location (cooperative Game)
The cooperative facility location game is a cooperative game of cost sharing. The goal is to share the cost of opening new facilities between the clients enjoying these facilities.Kamal Jain and Mohammad Mahdian, "Cost Sharing". Chapter 15 in The game has the following components: * There are several consumers who need a certain service, e.g, electricity connection. * There are several locations where facilities (e.g. power-stations) can be built. * For every pair of consumer (C) and location (L), there is a fixed cost of serving C from L (e.g, depending on the distance between the power station and the consumer's house). This cost is denoted Cost ,L * The cost of serving a group of consumers is lower than the sum of the cost of serving each consumer alone. EXAMPLE: * There are two facilities, F1 which costs 2 and F2 which costs 2. * There are three consumers, Alice Bob and Carl. * Alice can be served only from F1, with cost 2. So the cost of serving her alone is 2+2=4. * Bob can b ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |