|
Dorian M. Goldfeld
Dorian Morris Goldfeld (born January 21, 1947) is an American mathematician working in analytic number theory and automorphic forms at Columbia University. Professional career Goldfeld received his B.S. degree in 1967 from Columbia University. His doctoral dissertation, entitled "Some Methods of Averaging in the Analytical Theory of Numbers", was completed under the supervision of Patrick X. Gallagher in 1969, also at Columbia. He has held positions at the University of California at Berkeley ( Miller Fellow, 1969–1971), Hebrew University (1971–1972), Tel Aviv University (1972–1973), Institute for Advanced Study (1973–1974), in Italy (1974–1976), at MIT (1976–1982), University of Texas at Austin (1983–1985) and Harvard (1982–1985). Since 1985, he has been a professor at Columbia University. He is a member of the editorial board of '' Acta Arithmetica'' and of ''The Ramanujan Journal''. On January 1, 2018 he became the Editor-in-Chief of the Journal of Num ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Oberwolfach
Oberwolfach ( gsw, label= Low Alemannic, Obberwolfä) is a town in the district of Ortenau in Baden-Württemberg, Germany. It is the site of the Oberwolfach Research Institute for Mathematics, or Mathematisches Forschungsinstitut Oberwolfach. Geography Geographical situation The town of Oberwolfach lies between 270 and 948 meters above sea level in the central Schwarzwald (Black Forest) on the river Wolf, a tributary of the Kinzig. Neighbouring localities The district is neighboured by Bad Peterstal-Griesbach to the north, Bad Rippoldsau-Schapbach in Landkreis Freudenstadt to the east, by the towns of Wolfach and Hausach to the south, and by Oberharmersbach Oberharmersbach ( gsw, label= Low Alemannic, Haamerschbach) is a town in the district of Ortenau in Baden-Württemberg in Germany Germany,, officially the Federal Republic of Germany, is a country in Central Europe. It is the second ... to the west. References External links Gemeinde Oberwolfach: ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
University Of Texas At Austin
The University of Texas at Austin (UT Austin, UT, or Texas) is a public research university in Austin, Texas. It was founded in 1883 and is the oldest institution in the University of Texas System. With 40,916 undergraduate students, 11,075 graduate students and 3,133 teaching faculty as of Fall 2021, it is also the largest institution in the system. It is ranked among the top universities in the world by major college and university rankings, and admission to its programs is considered highly selective. UT Austin is considered one of the United States's Public Ivies. The university is a major center for academic research, with research expenditures totaling $679.8 million for fiscal year 2018. It joined the Association of American Universities in 1929. The university houses seven museums and seventeen libraries, including the LBJ Presidential Library and the Blanton Museum of Art, and operates various auxiliary research facilities, such as the J. J. Pickle Research Ca ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Benedict Gross
Benedict Hyman Gross is an American mathematician who is a professor at the University of California San Diego, the George Vasmer Leverett Professor of Mathematics Emeritus at Harvard University, and former Dean of Harvard College.Curriculum vitae from Gross' web site at Harvard, retrieved 2010-04-21. He is known for his work in , particularly the on s of |
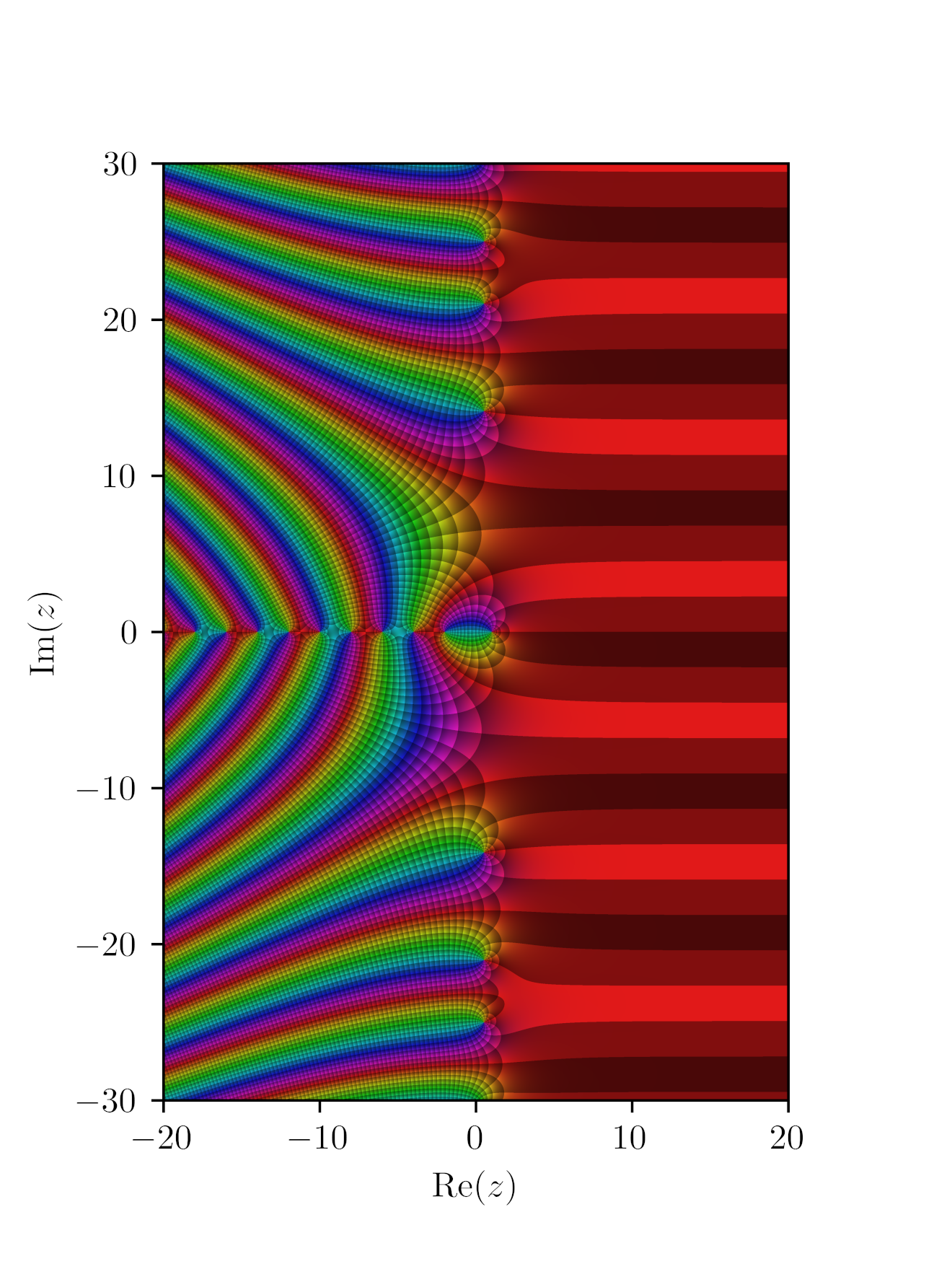
L-function
In mathematics, an ''L''-function is a meromorphic function on the complex plane, associated to one out of several categories of mathematical objects. An ''L''-series is a Dirichlet series, usually convergent on a half-plane, that may give rise to an ''L''-function via analytic continuation. The Riemann zeta function is an example of an ''L''-function, and one important conjecture involving ''L''-functions is the Riemann hypothesis and its generalization. The theory of ''L''-functions has become a very substantial, and still largely conjectural, part of contemporary analytic number theory. In it, broad generalisations of the Riemann zeta function and the ''L''-series for a Dirichlet character are constructed, and their general properties, in most cases still out of reach of proof, are set out in a systematic way. Because of the Euler product formula there is a deep connection between ''L''-functions and the theory of prime numbers. The mathematical field that studies L-func ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Elliptic Curve
In mathematics, an elliptic curve is a smooth, projective, algebraic curve of genus one, on which there is a specified point . An elliptic curve is defined over a field and describes points in , the Cartesian product of with itself. If the field's characteristic is different from 2 and 3, then the curve can be described as a plane algebraic curve which consists of solutions for: :y^2 = x^3 + ax + b for some coefficients and in . The curve is required to be non-singular, which means that the curve has no cusps or self-intersections. (This is equivalent to the condition , that is, being square-free in .) It is always understood that the curve is really sitting in the projective plane, with the point being the unique point at infinity. Many sources define an elliptic curve to be simply a curve given by an equation of this form. (When the coefficient field has characteristic 2 or 3, the above equation is not quite general enough to include all non-singular cubic cu ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Class Number Problem For Imaginary Quadratic Fields
In mathematics, the Gauss class number problem (for imaginary quadratic fields), as usually understood, is to provide for each ''n'' ≥ 1 a complete list of imaginary quadratic fields \mathbb(\sqrt) (for negative integers ''d'') having class number ''n''. It is named after Carl Friedrich Gauss. It can also be stated in terms of discriminants. There are related questions for real quadratic fields and for the behavior as d \to -\infty. The difficulty is in effective computation of bounds: for a given discriminant, it is easy to compute the class number, and there are several ineffective lower bounds on class number (meaning that they involve a constant that is not computed), but effective bounds (and explicit proofs of completeness of lists) are harder. Gauss's original conjectures The problems are posed in Gauss's Disquisitiones Arithmeticae of 1801 (Section V, Articles 303 and 304). are a set of more precise conjectures about the structure of class groups of quadratic ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Carl Friedrich Gauss
Johann Carl Friedrich Gauss (; german: Gauß ; la, Carolus Fridericus Gauss; 30 April 177723 February 1855) was a German mathematician and physicist who made significant contributions to many fields in mathematics and science. Sometimes referred to as the ''Princeps mathematicorum'' () and "the greatest mathematician since antiquity", Gauss had an exceptional influence in many fields of mathematics and science, and he is ranked among history's most influential mathematicians. Also available at Retrieved 23 February 2014. Comprehensive biographical article. Biography Early years Johann Carl Friedrich Gauss was born on 30 April 1777 in Brunswick (Braunschweig), in the Duchy of Brunswick-Wolfenbüttel (now part of Lower Saxony, Germany), to poor, working-class parents. His mother was illiterate and never recorded the date of his birth, remembering only that he had been born on a Wednesday, eight days before the Feast of the Ascension (which occurs 39 days after Easter). Ga ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
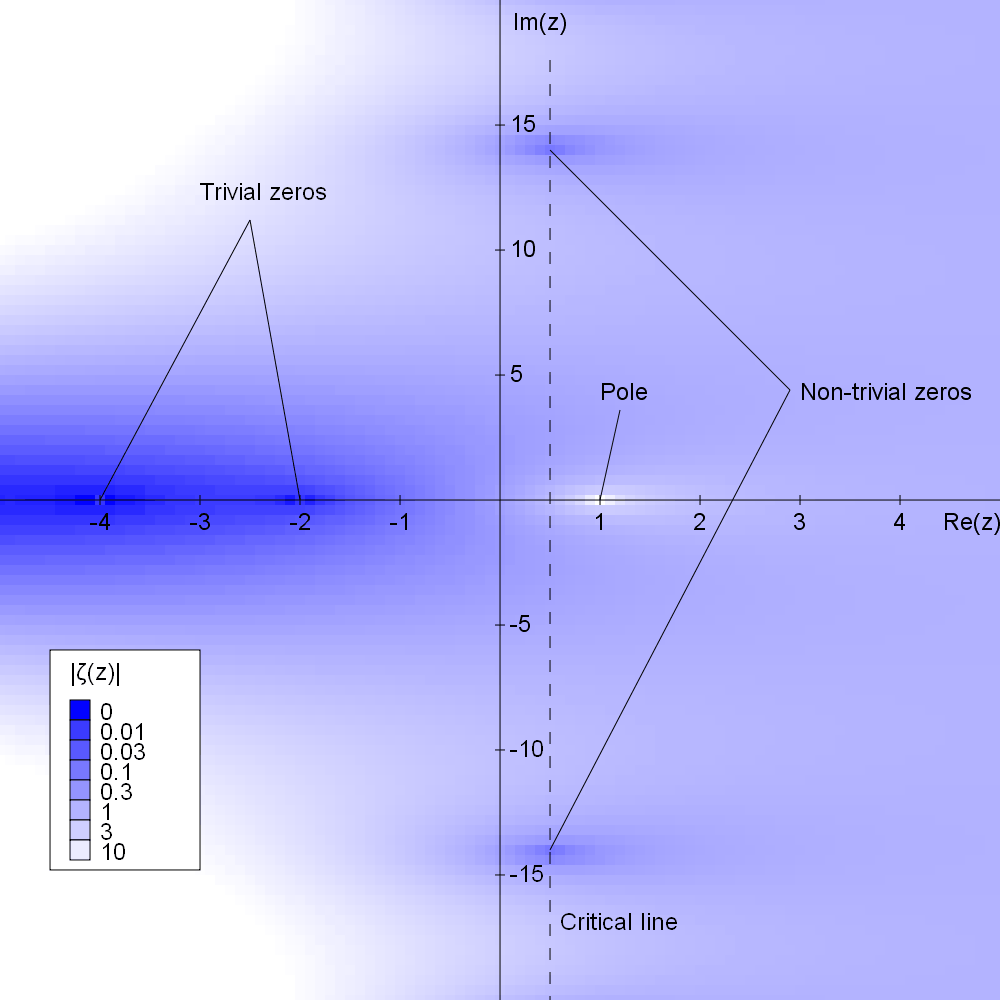
Riemann Hypothesis
In mathematics, the Riemann hypothesis is the conjecture that the Riemann zeta function has its zeros only at the negative even integers and complex numbers with real part . Many consider it to be the most important unsolved problem in pure mathematics. It is of great interest in number theory because it implies results about the distribution of prime numbers. It was proposed by , after whom it is named. The Riemann hypothesis and some of its generalizations, along with Goldbach's conjecture and the twin prime conjecture, make up Hilbert's eighth problem in David Hilbert's list of twenty-three unsolved problems; it is also one of the Clay Mathematics Institute's Millennium Prize Problems, which offers a million dollars to anyone who solves any of them. The name is also used for some closely related analogues, such as the Riemann hypothesis for curves over finite fields. The Riemann zeta function ζ(''s'') is a function whose argument ''s'' may be any complex number ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Artin's Conjecture On Primitive Roots
In number theory, Artin's conjecture on primitive roots states that a given integer ''a'' that is neither a square number nor −1 is a primitive root modulo infinitely many primes ''p''. The conjecture also ascribes an asymptotic density to these primes. This conjectural density equals Artin's constant or a rational multiple thereof. The conjecture was made by Emil Artin to Helmut Hasse on September 27, 1927, according to the latter's diary. The conjecture is still unresolved as of 2022. In fact, there is no single value of ''a'' for which Artin's conjecture is proved. Formulation Let ''a'' be an integer that is not a square number and not −1. Write ''a'' = ''a''0''b''2 with ''a''0 square-free. Denote by ''S''(''a'') the set of prime numbers ''p'' such that ''a'' is a primitive root modulo ''p''. Then the conjecture states # ''S''(''a'') has a positive asymptotic density inside the set of primes. In particular, ''S''(''a'') is infinite. # Under the conditions th ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Mathematika
''Mathematika'' is a peer-reviewed mathematics journal that publishes both pure and applied mathematical articles. The journal was founded by Harold Davenport in the 1950s. The journal is published by the London Mathematical Society, on behalf of the journal's owner University College London. Indexing and abstracting According to the ''Journal Citation Reports'', the journal has a 2020 impact factor of 0.844. The journal in indexing in the following bibliographic databases: * MathSciNet * Science Citation Index Expanded * Web of Science * Zentralblatt MATH zbMATH Open, formerly Zentralblatt MATH, is a major reviewing service providing reviews and abstracts for articles in pure and applied mathematics, produced by the Berlin office of FIZ Karlsruhe – Leibniz Institute for Information Infrastructur ... References {{reflist London Mathematical Society Mathematics education in the United Kingdom Mathematics journals Publications established in 1954 Quarterly journals W ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Number Theory
Number theory (or arithmetic or higher arithmetic in older usage) is a branch of pure mathematics devoted primarily to the study of the integers and arithmetic function, integer-valued functions. German mathematician Carl Friedrich Gauss (1777–1855) said, "Mathematics is the queen of the sciences—and number theory is the queen of mathematics."German original: "Die Mathematik ist die Königin der Wissenschaften, und die Arithmetik ist die Königin der Mathematik." Number theorists study prime numbers as well as the properties of mathematical objects made out of integers (for example, rational numbers) or defined as generalizations of the integers (for example, algebraic integers). Integers can be considered either in themselves or as solutions to equations (Diophantine geometry). Questions in number theory are often best understood through the study of Complex analysis, analytical objects (for example, the Riemann zeta function) that encode properties of the integers, primes ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Journal Of Number Theory
The ''Journal of Number Theory'' (''JNT'') is a bimonthly peer-reviewed scientific journal covering all aspects of number theory. The journal was established in 1969 by R.P. Bambah, P. Roquette, A. Ross, A. Woods, and H. Zassenhaus (Ohio State University). It is currently published monthly by Elsevier and the editor-in-chief is Dorian Goldfeld (Columbia University). According to the ''Journal Citation Reports'', the journal has a 2020 impact factor The impact factor (IF) or journal impact factor (JIF) of an academic journal is a scientometric index calculated by Clarivate that reflects the yearly mean number of citations of articles published in the last two years in a given journal, as i ... of 0.72. References External links * Number theory Mathematics journals Publications established in 1969 Elsevier academic journals Monthly journals English-language journals {{math-journal-stub ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |