|
Degrees Of Freedom (statistics)
In statistics, the number of degrees of freedom is the number of values in the final calculation of a statistic that are free to vary. Estimates of statistical parameters can be based upon different amounts of information or data. The number of independent pieces of information that go into the estimate of a parameter is called the degrees of freedom. In general, the degrees of freedom of an estimate of a parameter are equal to the number of independent scores that go into the estimate minus the number of parameters used as intermediate steps in the estimation of the parameter itself. For example, if the variance is to be estimated from a random sample of N independent scores, then the degrees of freedom is equal to the number of independent scores (''N'') minus the number of parameters estimated as intermediate steps (one, namely, the sample mean) and is therefore equal to N-1. Mathematically, degrees of freedom is the number of dimensions of the domain of a random vector, or e ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] [Amazon] |
Statistics
Statistics (from German language, German: ', "description of a State (polity), state, a country") is the discipline that concerns the collection, organization, analysis, interpretation, and presentation of data. In applying statistics to a scientific, industrial, or social problem, it is conventional to begin with a statistical population or a statistical model to be studied. Populations can be diverse groups of people or objects such as "all people living in a country" or "every atom composing a crystal". Statistics deals with every aspect of data, including the planning of data collection in terms of the design of statistical survey, surveys and experimental design, experiments. When census data (comprising every member of the target population) cannot be collected, statisticians collect data by developing specific experiment designs and survey sample (statistics), samples. Representative sampling assures that inferences and conclusions can reasonably extend from the sample ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] [Amazon] |
Nu (letter)
Nu, or ny (; uppercase Ν, lowercase ν; ''ni'' ), is the thirteenth letter of the Greek alphabet, representing the voiced alveolar nasal . In the system of Greek numerals it has a value of 50. It is derived from the Phoenician nun . Its Latin equivalent is N, though the lowercase (\nu) resembles the Roman lowercase v. The name of the letter is written in Ancient Greek and traditional Modern Greek polytonic orthography, while in Modern Greek it is written . Letters that arose from nu include Roman N and Cyrillic script En. Symbology The lower-case letter is used as a symbol in many academic fields. Uppercase nu is not used, because it appears identical to Latin N. * Mathematics: ** Degrees of freedom in statistics. ** The greatest fixed point of a function, as commonly used in the μ-calculus. ** Free names of a process, as used in the π-calculus. ** One of the Greeks in mathematical finance, known as "vega". ** The reciprocal of 1 plus the interest rate in fi ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] [Amazon] |
Expected Value
In probability theory, the expected value (also called expectation, expectancy, expectation operator, mathematical expectation, mean, expectation value, or first Moment (mathematics), moment) is a generalization of the weighted average. Informally, the expected value is the arithmetic mean, mean of the possible values a random variable can take, weighted by the probability of those outcomes. Since it is obtained through arithmetic, the expected value sometimes may not even be included in the sample data set; it is not the value you would expect to get in reality. The expected value of a random variable with a finite number of outcomes is a weighted average of all possible outcomes. In the case of a continuum of possible outcomes, the expectation is defined by Integral, integration. In the axiomatic foundation for probability provided by measure theory, the expectation is given by Lebesgue integration. The expected value of a random variable is often denoted by , , or , with a ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] [Amazon] |
Random Variable
A random variable (also called random quantity, aleatory variable, or stochastic variable) is a Mathematics, mathematical formalization of a quantity or object which depends on randomness, random events. The term 'random variable' in its mathematical definition refers to neither randomness nor variability but instead is a mathematical function (mathematics), function in which * the Domain of a function, domain is the set of possible Outcome (probability), outcomes in a sample space (e.g. the set \ which are the possible upper sides of a flipped coin heads H or tails T as the result from tossing a coin); and * the Range of a function, range is a measurable space (e.g. corresponding to the domain above, the range might be the set \ if say heads H mapped to -1 and T mapped to 1). Typically, the range of a random variable is a subset of the Real number, real numbers. Informally, randomness typically represents some fundamental element of chance, such as in the roll of a dice, d ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] [Amazon] |
Student's T
In probability theory and statistics, Student's distribution (or simply the distribution) t_\nu is a continuous probability distribution that generalizes the standard normal distribution. Like the latter, it is symmetric around zero and bell-shaped. However, t_\nu has heavier tails, and the amount of probability mass in the tails is controlled by the parameter \nu. For \nu = 1 the Student's distribution t_\nu becomes the standard Cauchy distribution, which has very "fat" tails; whereas for \nu \to \infty it becomes the standard normal distribution \mathcal(0, 1), which has very "thin" tails. The name "Student" is a pseudonym used by William Sealy Gosset in his scientific paper publications during his work at the Guinness Brewery in Dublin, Ireland. The Student's distribution plays a role in a number of widely used statistical analyses, including Student's -test for assessing the statistical significance of the difference between two sample means, the cons ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] [Amazon] |
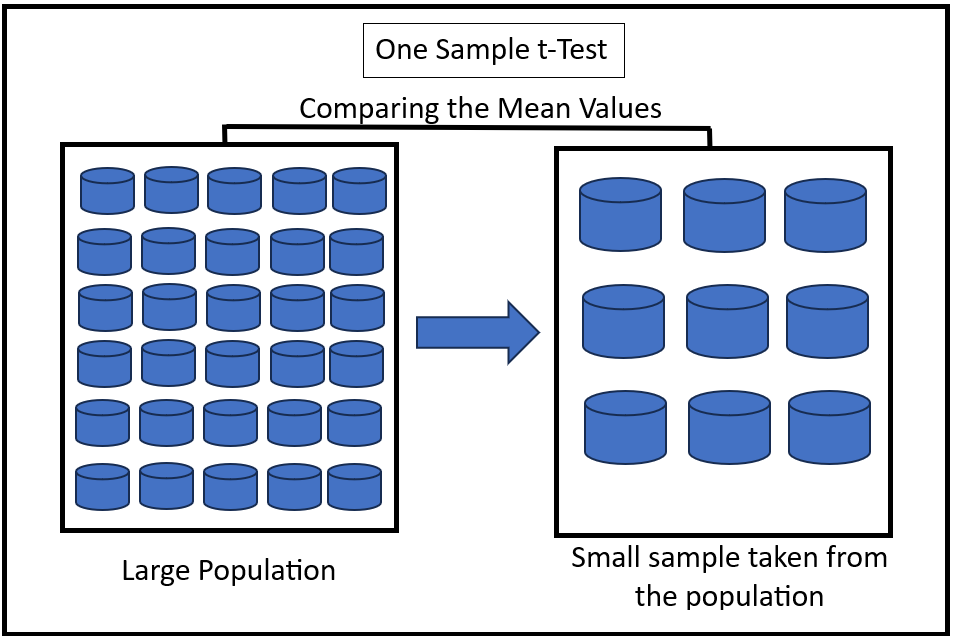
T-test
Student's ''t''-test is a statistical test used to test whether the difference between the response of two groups is Statistical significance, statistically significant or not. It is any statistical hypothesis testing, statistical hypothesis test in which the test statistic follows a Student's t-distribution, Student's ''t''-distribution under the null hypothesis. It is most commonly applied when the test statistic would follow a normal distribution if the value of a Scale parameter, scaling term in the test statistic were known (typically, the scaling term is unknown and is therefore a nuisance parameter). When the scaling term is estimated based on the data, the test statistic—under certain conditions—follows a Student's ''t'' distribution. The ''t''-test's most common application is to test whether the means of two populations are significantly different. In many cases, a Z-test, ''Z''-test will yield very similar results to a ''t''-test because the latter converges to the fo ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] [Amazon] |
Chi-squared Distribution
In probability theory and statistics, the \chi^2-distribution with k Degrees of freedom (statistics), degrees of freedom is the distribution of a sum of the squares of k Independence (probability theory), independent standard normal random variables. The chi-squared distribution \chi^2_k is a special case of the gamma distribution and the univariate Wishart distribution. Specifically if X \sim \chi^2_k then X \sim \text(\alpha=\frac, \theta=2) (where \alpha is the shape parameter and \theta the scale parameter of the gamma distribution) and X \sim \text_1(1,k) . The scaled chi-squared distribution s^2 \chi^2_k is a reparametrization of the gamma distribution and the univariate Wishart distribution. Specifically if X \sim s^2 \chi^2_k then X \sim \text(\alpha=\frac, \theta=2 s^2) and X \sim \text_1(s^2,k) . The chi-squared distribution is one of the most widely used probability distributions in inferential statistics, notably in hypothesis testing and in constru ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] [Amazon] |
Residual Sum-of-squares
In statistics, the residual sum of squares (RSS), also known as the sum of squared residuals (SSR) or the sum of squared estimate of errors (SSE), is the sum of the squares of residuals (deviations predicted from actual empirical values of data). It is a measure of the discrepancy between the data and an estimation model, such as a linear regression. A small RSS indicates a tight fit of the model to the data. It is used as an optimality criterion in parameter selection and model selection. In general, total sum of squares = explained sum of squares + residual sum of squares. For a proof of this in the multivariate ordinary least squares (OLS) case, see partitioning in the general OLS model. One explanatory variable In a model with a single explanatory variable, RSS is given by: :\operatorname = \sum_^n (y_i - f(x_i))^2 where ''y''''i'' is the ''i''th value of the variable to be predicted, ''x''''i'' is the ''i''th value of the explanatory variable, and f(x_i) is the ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] [Amazon] |
Orthogonal Complement
In the mathematical fields of linear algebra and functional analysis, the orthogonal complement of a subspace W of a vector space V equipped with a bilinear form B is the set W^\perp of all vectors in V that are orthogonal to every vector in W. Informally, it is called the perp, short for perpendicular complement. It is a subspace of V. Example Let V = (\R^5, \langle \cdot, \cdot \rangle) be the vector space equipped with the usual dot product \langle \cdot, \cdot \rangle (thus making it an inner product space), and let W = \, with \mathbf = \begin 1 & 0\\ 0 & 1\\ 2 & 6\\ 3 & 9\\ 5 & 3\\ \end. then its orthogonal complement W^\perp = \ can also be defined as W^\perp = \, being \mathbf = \begin -2 & -3 & -5 \\ -6 & -9 & -3 \\ 1 & 0 & 0 \\ 0 & 1 & 0 \\ 0 & 0 & 1 \end. The fact that every column vector in \mathbf is orthogonal to every column vector in \mathbf can be checked by direct computation. The fact that the spans of these vectors are orthogonal then follows by bilinearity ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] [Amazon] |
Linear Span
In mathematics, the linear span (also called the linear hull or just span) of a set S of elements of a vector space V is the smallest linear subspace of V that contains S. It is the set of all finite linear combinations of the elements of , and the intersection of all linear subspaces that contain S. It is often denoted pp. 29-30, §§ 2.5, 2.8 or \langle S \rangle. For example, in geometry, two linearly independent vectors span a plane. To express that a vector space is a linear span of a subset , one commonly uses one of the following phrases: spans ; is a spanning set of ; is spanned or generated by ; is a generator set or a generating set of . Spans can be generalized to many mathematical structures, in which case, the smallest substructure containing S is generally called the substructure ''generated'' by S. Definition Given a vector space over a field , the span of a set of vectors (not necessarily finite) is defined to be the intersection of all subsp ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] [Amazon] |
Euclidean Subspace
In geometry, a flat is an affine subspace, i.e. a subset of an affine space that is itself an affine space. Particularly, in the case the parent space is Euclidean, a flat is a Euclidean subspace which inherits the notion of distance from its parent space. In an -dimensional space, there are -flats of every dimension from 0 to ; flats one dimension lower than the parent space, -flats, are called ''hyperplanes''. The flats in a plane (two-dimensional space) are points, lines, and the plane itself; the flats in three-dimensional space are points, lines, planes, and the space itself. The definition of flat excludes non-straight curves and non-planar surfaces, which are subspaces having different notions of distance: arc length and geodesic length, respectively. Flats occur in linear algebra, as geometric realizations of solution sets of systems of linear equations. A flat is a manifold and an algebraic variety, and is sometimes called a ''linear manifold'' or ''linear va ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] [Amazon] |
Oblique Projection
Oblique projection is a simple type of technical drawing of graphical projection used for producing two-dimensional (2D) images of three-dimensional (3D) objects. The objects are not in perspective and so do not correspond to any view of an object that can be obtained in practice, but the technique yields somewhat convincing and useful results. Oblique projection is commonly used in technical drawing. The cavalier projection was used by French military artists in the 18th century to depict fortifications. Oblique projection was used almost universally by Chinese artists from the 1st or 2nd centuries to the 18th century, especially to depict rectilinear objects such as houses. Various graphical projection techniques can be used in computer graphics, including in Computer Aided Design (CAD), computer games, computer generated animations, and special effects used in movies. Overview Oblique projection is a type of parallel projection: * it projects an image by inters ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] [Amazon] |