|
Tensors In General Relativity
In mathematics, a tensor is an algebraic object that describes a multilinear relationship between sets of algebraic objects related to a vector space. Tensors may map between different objects such as vectors, scalars, and even other tensors. There are many types of tensors, including scalars and vectors (which are the simplest tensors), dual vectors, multilinear maps between vector spaces, and even some operations such as the dot product. Tensors are defined independent of any basis, although they are often referred to by their components in a basis related to a particular coordinate system. Tensors have become important in physics because they provide a concise mathematical framework for formulating and solving physics problems in areas such as mechanics ( stress, elasticity, fluid mechanics, moment of inertia, ...), electrodynamics ( electromagnetic tensor, Maxwell tensor, permittivity, magnetic susceptibility, ...), general relativity (stress–energy tenso ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Vector Space
In mathematics and physics, a vector space (also called a linear space) is a set whose elements, often called '' vectors'', may be added together and multiplied ("scaled") by numbers called '' scalars''. Scalars are often real numbers, but can be complex numbers or, more generally, elements of any field. The operations of vector addition and scalar multiplication must satisfy certain requirements, called ''vector axioms''. The terms real vector space and complex vector space are often used to specify the nature of the scalars: real coordinate space or complex coordinate space. Vector spaces generalize Euclidean vectors, which allow modeling of physical quantities, such as forces and velocity, that have not only a magnitude, but also a direction. The concept of vector spaces is fundamental for linear algebra, together with the concept of matrix, which allows computing in vector spaces. This provides a concise and synthetic way for manipulating and studying systems of li ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Moment Of Inertia
The moment of inertia, otherwise known as the mass moment of inertia, angular mass, second moment of mass, or most accurately, rotational inertia, of a rigid body is a quantity that determines the torque needed for a desired angular acceleration about a rotational axis, akin to how mass determines the force needed for a desired acceleration. It depends on the body's mass distribution and the axis chosen, with larger moments requiring more torque to change the body's rate of rotation. It is an extensive (additive) property: for a point mass the moment of inertia is simply the mass times the square of the perpendicular distance to the axis of rotation. The moment of inertia of a rigid composite system is the sum of the moments of inertia of its component subsystems (all taken about the same axis). Its simplest definition is the second moment of mass with respect to distance from an axis. For bodies constrained to rotate in a plane, only their moment of inertia about an a ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Elwin Bruno Christoffel
Elwin Bruno Christoffel (; 10 November 1829 – 15 March 1900) was a German mathematician and physicist. He introduced fundamental concepts of differential geometry, opening the way for the development of tensor calculus, which would later provide the mathematical basis for general relativity. Life Christoffel was born on 10 November 1829 in Montjoie (now Monschau) in Prussia in a family of cloth merchants. He was initially educated at home in languages and mathematics, then attended the Jesuit Gymnasium and the Friedrich-Wilhelms Gymnasium in Cologne. In 1850 he went to the University of Berlin, where he studied mathematics with Gustav Dirichlet (which had a strong influence over him) among others, as well as attending courses in physics and chemistry. He received his doctorate in Berlin in 1856 for a thesis on the motion of electricity in homogeneous bodies written under the supervision of Martin Ohm, Ernst Kummer and Heinrich Gustav Magnus. After receiving his doctorate ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Bernhard Riemann
Georg Friedrich Bernhard Riemann (; 17 September 1826 – 20 July 1866) was a German mathematician who made contributions to analysis, number theory, and differential geometry. In the field of real analysis, he is mostly known for the first rigorous formulation of the integral, the Riemann integral, and his work on Fourier series. His contributions to complex analysis include most notably the introduction of Riemann surfaces, breaking new ground in a natural, geometric treatment of complex analysis. His 1859 paper on the prime-counting function, containing the original statement of the Riemann hypothesis, is regarded as a foundational paper of analytic number theory. Through his pioneering contributions to differential geometry, Riemann laid the foundations of the mathematics of general relativity. He is considered by many to be one of the greatest mathematicians of all time. Biography Early years Riemann was born on 17 September 1826 in Breselenz, a village near D ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Gregorio Ricci-Curbastro
Gregorio Ricci-Curbastro (; 12January 1925) was an Italian mathematician. He is most famous as the discoverer of tensor calculus. With his former student Tullio Levi-Civita, he wrote his most famous single publication, a pioneering work on the calculus of tensors, signing it as Gregorio Ricci. This appears to be the only time that Ricci-Curbastro used the shortened form of his name in a publication, and continues to cause confusion. Ricci-Curbastro also published important works in other fields, including a book on higher algebra and infinitesimal analysis, and papers on the theory of real numbers, an area in which he extended the research begun by Richard Dedekind. Early life and education Completing privately his high school studies at only 16 years of age, he enrolled on the course of philosophy-mathematics at Rome University (1869). The following year the Papal State fell and so Gregorio was called by his father to the city of his birth, Lugo di Romagna. Subsequently he ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Tullio Levi-Civita
Tullio Levi-Civita, (, ; 29 March 1873 – 29 December 1941) was an Italian mathematician, most famous for his work on absolute differential calculus ( tensor calculus) and its applications to the theory of relativity, but who also made significant contributions in other areas. He was a pupil of Gregorio Ricci-Curbastro, the inventor of tensor calculus. His work included foundational papers in both pure and applied mathematics, celestial mechanics (notably on the three-body problem), analytic mechanics (the Levi-Civita separability conditions in the Hamilton–Jacobi equation) and hydrodynamics. Biography Born into an Italian Jewish family in Padua, Levi-Civita was the son of Giacomo Levi-Civita, a lawyer and former senator. He graduated in 1892 from the University of Padua Faculty of Mathematics. In 1894 he earned a teaching diploma after which he was appointed to the Faculty of Science teacher's college in Pavia. In 1898 he was appointed to the Padua Chair of Rational Mec ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Tensor Field
In mathematics and physics, a tensor field assigns a tensor to each point of a mathematical space (typically a Euclidean space or manifold). Tensor fields are used in differential geometry, algebraic geometry, general relativity, in the analysis of stress and strain in materials, and in numerous applications in the physical sciences. As a tensor is a generalization of a scalar (a pure number representing a value, for example speed) and a vector (a pure number plus a direction, like velocity), a tensor field is a generalization of a scalar field or vector field that assigns, respectively, a scalar or vector to each point of space. If a tensor is defined on a vector fields set over a module , we call a tensor field on . Many mathematical structures called "tensors" are also tensor fields. For example, the Riemann curvature tensor is a tensor ''field'' as it is defined on a manifold: it is named after Bernhard Riemann, and associates a tensor to each point of a Riemann ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
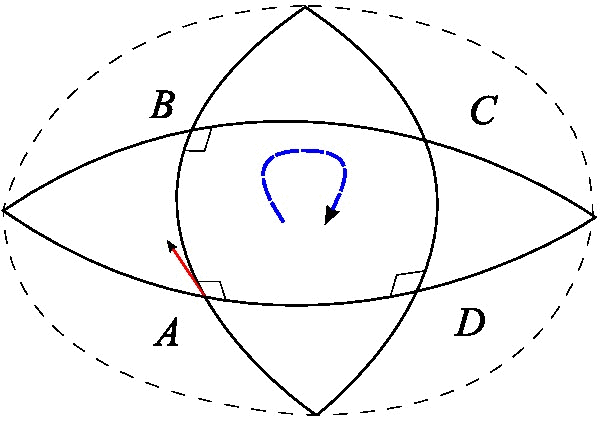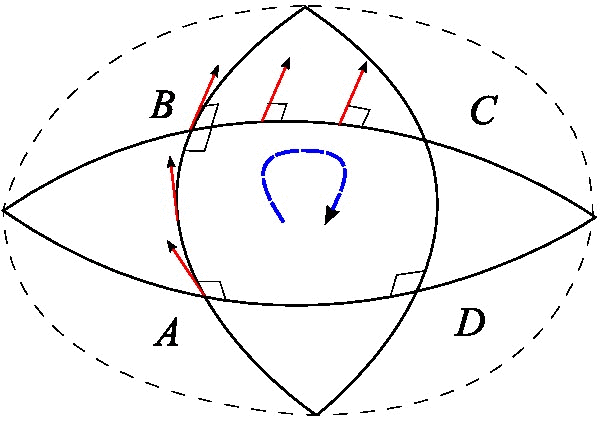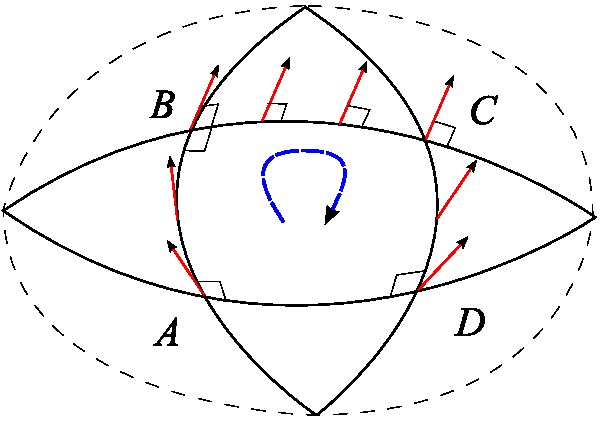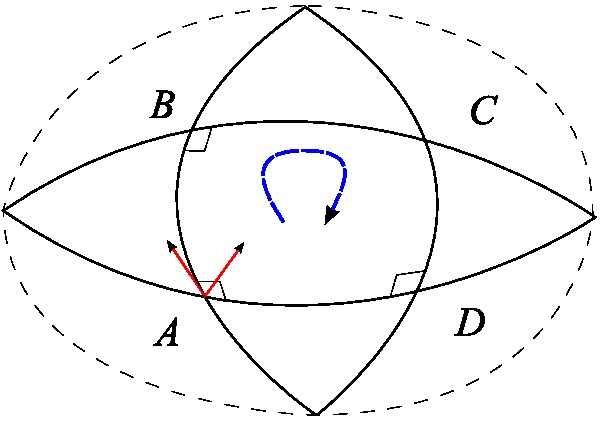
Riemann Curvature Tensor
In the mathematical field of differential geometry, the Riemann curvature tensor or Riemann–Christoffel tensor (after Bernhard Riemann and Elwin Bruno Christoffel) is the most common way used to express the curvature of Riemannian manifolds. It assigns a tensor to each point of a Riemannian manifold (i.e., it is a tensor field). It is a local invariant of Riemannian metrics which measures the failure of the second covariant derivatives to commute. A Riemannian manifold has zero curvature if and only if it is ''flat'', i.e. locally isometric to the Euclidean space. The curvature tensor can also be defined for any pseudo-Riemannian manifold, or indeed any manifold equipped with an affine connection. It is a central mathematical tool in the theory of general relativity, the modern theory of gravity, and the curvature of spacetime is in principle observable via the geodesic deviation equation. The curvature tensor represents the tidal force experienced by a rigid body movin ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Stress–energy Tensor
The stress–energy tensor, sometimes called the stress–energy–momentum tensor or the energy–momentum tensor, is a tensor physical quantity that describes the density and flux of energy and momentum in spacetime, generalizing the stress tensor of Newtonian physics. It is an attribute of matter, radiation, and non-gravitational force fields. This density and flux of energy and momentum are the sources of the gravitational field in the Einstein field equations of general relativity, just as mass density is the source of such a field in Newtonian gravity. Definition The stress–energy tensor involves the use of superscripted variables (''not'' exponents; see tensor index notation and Einstein summation notation). If Cartesian coordinates in SI units are used, then the components of the position four-vector are given by: , , , and , where ''t'' is time in seconds, and ''x'', ''y'', and ''z'' are distances in meters. The stress–energy tensor is defined as the tensor ''T ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
General Relativity
General relativity, also known as the general theory of relativity and Einstein's theory of gravity, is the geometric theory of gravitation published by Albert Einstein in 1915 and is the current description of gravitation in modern physics. General relativity generalizes special relativity and refines Newton's law of universal gravitation, providing a unified description of gravity as a geometric property of space and time or four-dimensional spacetime. In particular, the ' is directly related to the energy and momentum of whatever matter and radiation are present. The relation is specified by the Einstein field equations, a system of second order partial differential equations. Newton's law of universal gravitation, which describes classical gravity, can be seen as a prediction of general relativity for the almost flat spacetime geometry around stationary mass distributions. Some predictions of general relativity, however, are beyond Newton's law of universal gr ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Magnetic Susceptibility
In electromagnetism, the magnetic susceptibility (Latin: , "receptive"; denoted ) is a measure of how much a material will become magnetized in an applied magnetic field. It is the ratio of magnetization (magnetic moment per unit volume) to the applied magnetizing field intensity . This allows a simple classification, into two categories, of most materials' responses to an applied magnetic field: an alignment with the magnetic field, , called paramagnetism, or an alignment against the field, , called diamagnetism. Magnetic susceptibility indicates whether a material is attracted into or repelled out of a magnetic field. Paramagnetic materials align with the applied field and are attracted to regions of greater magnetic field. Diamagnetic materials are anti-aligned and are pushed away, toward regions of lower magnetic fields. On top of the applied field, the magnetization of the material adds its own magnetic field, causing the field lines to concentrate in paramagnetism, or be exc ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Permittivity
In electromagnetism, the absolute permittivity, often simply called permittivity and denoted by the Greek letter ''ε'' ( epsilon), is a measure of the electric polarizability of a dielectric. A material with high permittivity polarizes more in response to an applied electric field than a material with low permittivity, thereby storing more energy in the material. In electrostatics, the permittivity plays an important role in determining the capacitance of a capacitor. In the simplest case, the electric displacement field D resulting from an applied electric field E is :\mathbf = \varepsilon \mathbf. More generally, the permittivity is a thermodynamic function of state. It can depend on the frequency, magnitude, and direction of the applied field. The SI unit for permittivity is farad per meter (F/m). The permittivity is often represented by the relative permittivity ''ε''r which is the ratio of the absolute permittivity ''ε'' and the vacuum permittivity ''ε''0 ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |