|
Cunningham Correction Factor
In fluid dynamics, the Cunningham correction factor or Cunningham slip correction factor is used to account for noncontinuum effects when calculating the drag on small particles. The derivation of Stokes' law, which is used to calculate the drag force on small particles, assumes a no-slip condition which is no longer correct at high Knudsen number. The Cunningham slip correction factor allows predicting the drag force on a particle moving a fluid with Knudsen number between the continuum regime and free molecular flow. The drag coefficient calculated with standard correlations is divided by the Cunningham correction factor, C given below. Ebenezer CunninghamCunningham, E., "On the velocity of steady fall of spherical particles through fluid medium," ''Proc. Roy. Soc. A'' 83(1910)357. derived the correction factor in 1910 and with Robert Andrews Millikan, verified the correction in the same year. :C = 1+ \frac\cdot (A_1+A_2\cdot e^) where :''C'' is the correction factor :λ is ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
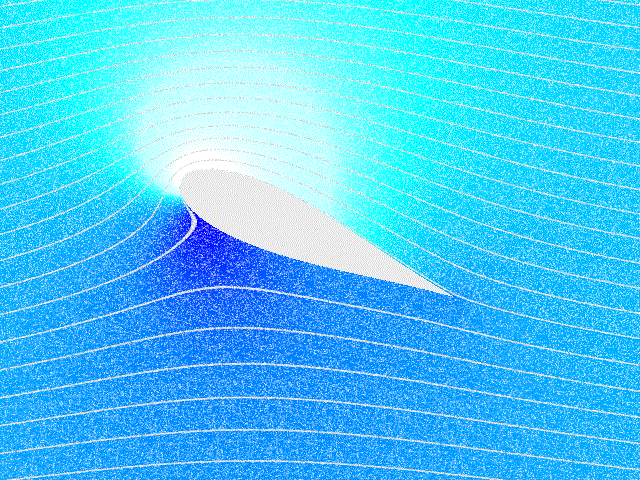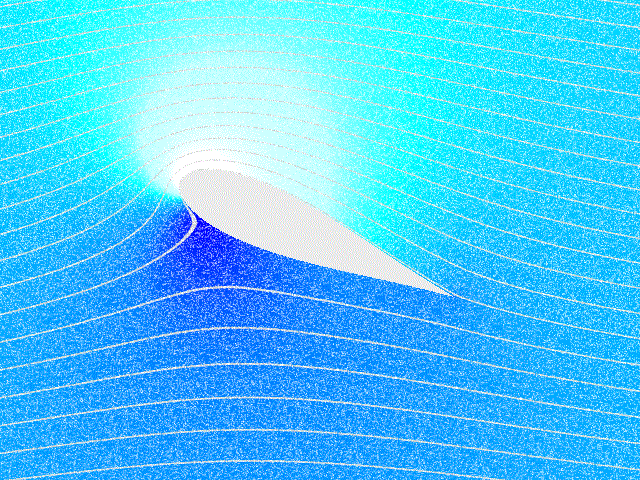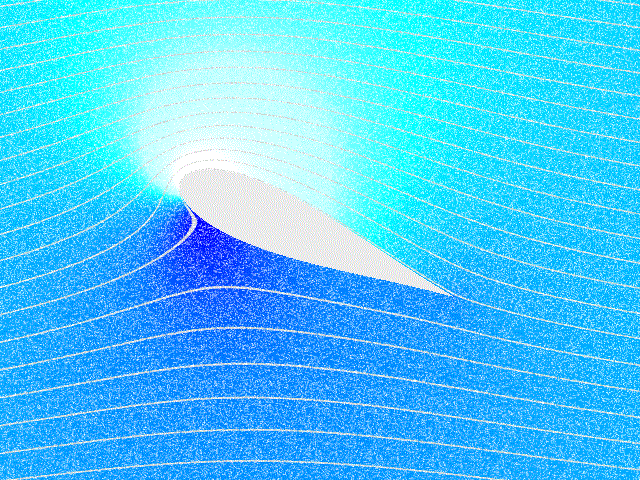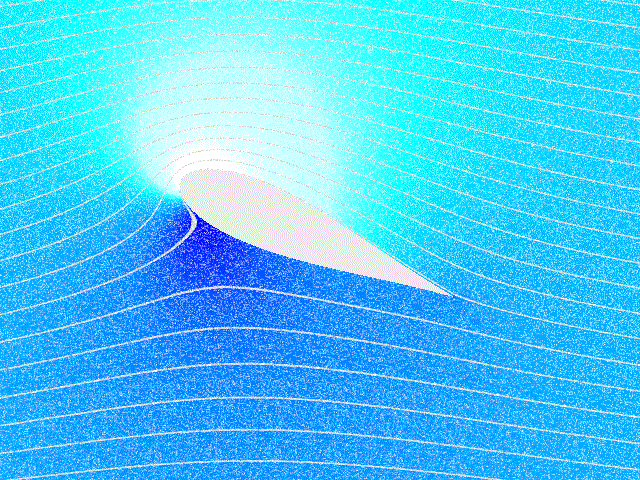
Fluid Dynamics
In physics and engineering, fluid dynamics is a subdiscipline of fluid mechanics that describes the flow of fluids—liquids and gases. It has several subdisciplines, including '' aerodynamics'' (the study of air and other gases in motion) and hydrodynamics (the study of liquids in motion). Fluid dynamics has a wide range of applications, including calculating forces and moments on aircraft, determining the mass flow rate of petroleum through pipelines, predicting weather patterns, understanding nebulae in interstellar space and modelling fission weapon detonation. Fluid dynamics offers a systematic structure—which underlies these practical disciplines—that embraces empirical and semi-empirical laws derived from flow measurement and used to solve practical problems. The solution to a fluid dynamics problem typically involves the calculation of various properties of the fluid, such as flow velocity, pressure, density, and temperature, as functions of space a ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Stokes' Law
In 1851, George Gabriel Stokes derived an expression, now known as Stokes' law, for the frictional force – also called drag force – exerted on spherical objects with very small Reynolds numbers in a viscous fluid. Stokes' law is derived by solving the Stokes flow limit for small Reynolds numbers of the Navier–Stokes equations.Batchelor (1967), p. 233. Statement of the law The force of viscosity on a small sphere moving through a viscous fluid is given by: :F_ = 6 \pi \mu R v where: * ''F''d is the frictional force – known as Stokes' drag – acting on the interface between the fluid and the particle * ''μ'' is the dynamic viscosity (some authors use the symbol ''η'') * ''R'' is the radius of the spherical object * ''v'' is the flow velocity relative to the object. In SI units, ''F''d is given in newtons (= kg m s−2), ''μ'' in Pa·s (= kg m−1 s−1), ''R'' in meters, and ''v'' in m/s. Stokes' law makes the following assumptions for the behavior of a particle ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
No-slip Condition
In fluid dynamics, the no-slip condition for viscous fluids assumes that at a solid boundary, the fluid will have zero velocity relative to the boundary. The fluid velocity at all fluid–solid boundaries is equal to that of the solid boundary. Conceptually, one can think of the outermost molecules of fluid as stuck to the surfaces past which it flows. Because the solution is prescribed at given locations, this is an example of a Dirichlet boundary condition. Physical justification Particles close to a surface do not move along with a flow when adhesion is stronger than cohesion. At the fluid-solid interface, the force of attraction between the fluid particles and solid particles (Adhesive forces) is greater than that between the fluid particles (Cohesive forces). This force imbalance brings down the fluid velocity to zero. The no slip condition is only defined for viscous flows and where continuum concept is valid. Exceptions As with most of the engineering approximations, the ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Knudsen Number
The Knudsen number (Kn) is a dimensionless number defined as the ratio of the molecular mean free path length to a representative physical length scale. This length scale could be, for example, the radius of a body in a fluid. The number is named after Danish physicist Martin Knudsen (1871–1949). The Knudsen number helps determine whether statistical mechanics or the continuum mechanics formulation of fluid dynamics should be used to model a situation. If the Knudsen number is near or greater than one, the mean free path of a molecule is comparable to a length scale of the problem, and the continuum assumption of fluid mechanics is no longer a good approximation. In such cases, statistical methods should be used. Definition The Knudsen number is a dimensionless number defined as :\mathrm\ = \frac , where : \lambda = mean free path 1 : L = representative physical length scale 1 The representative length scale considered, L, may correspond to various physical traits ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Drag Force
In fluid dynamics, drag (sometimes called air resistance, a type of friction, or fluid resistance, another type of friction or fluid friction) is a force acting opposite to the relative motion of any object moving with respect to a surrounding fluid. This can exist between two fluid layers (or surfaces) or between a fluid and a solid surface. Unlike other resistive forces, such as dry friction, which are nearly independent of velocity, the drag force depends on velocity. Drag force is proportional to the velocity for low-speed flow and the squared velocity for high speed flow, where the distinction between low and high speed is measured by the Reynolds number. Even though the ultimate cause of drag is viscous friction, turbulent drag is independent of viscosity. Drag forces always tend to decrease fluid velocity relative to the solid object in the fluid's path. Examples Examples of drag include the component of the net aerodynamic or hydrodynamic force acting opposite to the di ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Free Molecular Flow
Free molecular flow describes the fluid dynamics of gas where the mean free path of the molecules is larger than the size of the chamber or of the object under test. For tubes/objects of the size of several cm, this means pressures well below 10−3 mbar. This is also called the regime of high vacuum, or even ultra-high vacuum. This is opposed to viscous flow encountered at higher pressures. The presence of free molecular flow can be calculated, at least in estimation, with the Knudsen number (Kn). If Kn > 10, the system is in free molecular flow, also known as Knudsen flow. In free molecular flow, the pressure of the remaining gas can be considered as effectively zero. Thus, boiling points do not depend on the residual pressure. The flow can be considered to be individual particles moving in straight lines. Practically, the "vapor" cannot move around bends or into other spaces behind obstacles, as they simply hit the tube wall. This implies conventional pumps cannot be used, ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Drag Coefficient
In fluid dynamics, the drag coefficient (commonly denoted as: c_\mathrm, c_x or c_) is a dimensionless quantity that is used to quantify the drag or resistance of an object in a fluid environment, such as air or water. It is used in the drag equation in which a lower drag coefficient indicates the object will have less aerodynamic or hydrodynamic drag. The drag coefficient is always associated with a particular surface area. The drag coefficient of any object comprises the effects of the two basic contributors to fluid dynamic drag: skin friction and form drag. The drag coefficient of a lifting airfoil or hydrofoil also includes the effects of lift-induced drag. The drag coefficient of a complete structure such as an aircraft also includes the effects of interference drag. Definition The drag coefficient c_\mathrm d is defined as c_\mathrm d = \dfrac where: * F_\mathrm d is the drag force, which is by definition the force component in the direction of the flow vel ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Ebenezer Cunningham
Ebenezer Cunningham (7 May 1881 in Hackney, London – 12 February 1977) was a British mathematician who is remembered for his research and exposition at the dawn of special relativity. Biography Cunningham went up to St John's College, Cambridge in 1899 and graduated Senior Wrangler in 1902, winning the Smith's Prize in 1904. In 1904, as a lecturer at the University of Liverpool, he began work on a new theorem in relativity with fellow lecturer Harry Bateman. They brought the methods of inversive geometry into electromagnetic theory with their transformations ( spherical wave transformation): :Each four-dimensional solution o Maxwell's equationscould then be inverted in a four-dimensional ''hypersphere of pseudo-radius K'' in order to produce a new solution. Central to Cunningham's paper was the demonstration that Maxwell's equations retained their form under these transformations. He worked with Karl Pearson in 1907 at University College London. Cunningham married Ada Co ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Robert Andrews Millikan
Robert Andrews Millikan (March 22, 1868 – December 19, 1953) was an American experimental physicist honored with the Nobel Prize for Physics in 1923 for the measurement of the elementary electric charge and for his work on the photoelectric effect. Millikan graduated from Oberlin College in 1891 and obtained his doctorate at Columbia University in 1895. In 1896 he became an assistant at the University of Chicago, where he became a full professor in 1910. In 1909 Millikan began a series of experiments to determine the electric charge carried by a single electron. He began by measuring the course of charged water droplets in an electric field. The results suggested that the charge on the droplets is a multiple of the elementary electric charge, but the experiment was not accurate enough to be convincing. He obtained more precise results in 1910 with his famous oil-drop experiment in which he replaced water (which tended to evaporate too quickly) with oil. In 1914 Millik ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Mean Free Path
In physics, mean free path is the average distance over which a moving particle (such as an atom, a molecule, or a photon) travels before substantially changing its direction or energy (or, in a specific context, other properties), typically as a result of one or more successive collisions with other particles. Scattering theory Imagine a beam of particles being shot through a target, and consider an infinitesimally thin slab of the target (see the figure). The atoms (or particles) that might stop a beam particle are shown in red. The magnitude of the mean free path depends on the characteristics of the system. Assuming that all the target particles are at rest but only the beam particle is moving, that gives an expression for the mean free path: :\ell = (\sigma n)^, where is the mean free path, is the number of target particles per unit volume, and is the effective cross-sectional area for collision. The area of the slab is , and its volume is . The typical number of ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Brownian Motion
Brownian motion, or pedesis (from grc, πήδησις "leaping"), is the random motion of particles suspended in a medium (a liquid or a gas). This pattern of motion typically consists of random fluctuations in a particle's position inside a fluid sub-domain, followed by a relocation to another sub-domain. Each relocation is followed by more fluctuations within the new closed volume. This pattern describes a fluid at thermal equilibrium, defined by a given temperature. Within such a fluid, there exists no preferential direction of flow (as in transport phenomena). More specifically, the fluid's overall linear and angular momenta remain null over time. The kinetic energies of the molecular Brownian motions, together with those of molecular rotations and vibrations, sum up to the caloric component of a fluid's internal energy (the equipartition theorem). This motion is named after the botanist Robert Brown, who first described the phenomenon in 1827, while looking th ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Fluid Dynamics
In physics and engineering, fluid dynamics is a subdiscipline of fluid mechanics that describes the flow of fluids—liquids and gases. It has several subdisciplines, including '' aerodynamics'' (the study of air and other gases in motion) and hydrodynamics (the study of liquids in motion). Fluid dynamics has a wide range of applications, including calculating forces and moments on aircraft, determining the mass flow rate of petroleum through pipelines, predicting weather patterns, understanding nebulae in interstellar space and modelling fission weapon detonation. Fluid dynamics offers a systematic structure—which underlies these practical disciplines—that embraces empirical and semi-empirical laws derived from flow measurement and used to solve practical problems. The solution to a fluid dynamics problem typically involves the calculation of various properties of the fluid, such as flow velocity, pressure, density, and temperature, as functions of space a ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |