|
Commute Time
Commute, commutation or commutative may refer to: * Commuting, the process of travelling between a place of residence and a place of work Mathematics * Commutative property, a property of a mathematical operation whose result is insensitive to the order of its arguments **Equivariant map, a function whose composition with another function has the commutative property **Commutative diagram, a graphical description of commuting compositions of arrows in a mathematical category **Commutative semigroup, commutative monoid, abelian group, and commutative ring, algebraic structures with the commutative property **Commuting matrices, sets of matrices whose products do not depend on the order of multiplication **Commutator, a measure of the failure of two elements to be commutative in a group or ring Science and technology * Commutator (electric), a rotary switch on the shaft of an electric motor or generator * Commutation (neurophysiology), how certain neural circuits in the brain exhibi ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Commuting
Commuting is periodically recurring travel between one's place of residence and place of work or study, where the traveler, referred to as a commuter, leaves the boundary of their home community. By extension, it can sometimes be any regular or often repeated travel between locations, even when not work-related. The modes of travel, time taken and distance traveled in commuting varies widely across the globe. Most people in least-developed countries continue to walk to work. The cheapest method of commuting after walking is usually by bicycle, so this is common in low-income countries, but is also increasingly practised by people in wealthier countries for environmental and health reasons. In middle-income countries, motorcycle commuting is very common. The next technology adopted as countries develop is more dependent on location: in more populous, older cities, especially in Eurasia mass transit (rail, bus, etc.) predominates, while in smaller, younger cities, and larg ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Commutator
In mathematics, the commutator gives an indication of the extent to which a certain binary operation fails to be commutative. There are different definitions used in group theory and ring theory. Group theory The commutator of two elements, and , of a group , is the element : . This element is equal to the group's identity if and only if and commute (from the definition , being equal to the identity if and only if ). The set of all commutators of a group is not in general closed under the group operation, but the subgroup of ''G'' generated by all commutators is closed and is called the ''derived group'' or the ''commutator subgroup'' of ''G''. Commutators are used to define nilpotent and solvable groups and the largest abelian quotient group. The definition of the commutator above is used throughout this article, but many other group theorists define the commutator as :. Identities (group theory) Commutator identities are an important tool in group theory. The expr ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Commute
Commute, commutation or commutative may refer to: * Commuting, the process of travelling between a place of residence and a place of work Mathematics * Commutative property, a property of a mathematical operation whose result is insensitive to the order of its arguments **Equivariant map, a function whose composition with another function has the commutative property **Commutative diagram, a graphical description of commuting compositions of arrows in a mathematical category **Commutative semigroup, commutative monoid, abelian group, and commutative ring, algebraic structures with the commutative property **Commuting matrices, sets of matrices whose products do not depend on the order of multiplication **Commutator, a measure of the failure of two elements to be commutative in a group or ring Science and technology * Commutator (electric), a rotary switch on the shaft of an electric motor or generator * Commutation (neurophysiology), how certain neural circuits in the brain exhibi ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Commutation (law)
In law, a commutation is the substitution of a lesser penalty for that given after a conviction for a crime. The penalty can be lessened in severity, in duration, or both. Unlike most pardons by government and overturning by the court (a full overturning is equal to an acquittal In common law jurisdictions, an acquittal certifies that the accused is free from the charge of an offense, as far as criminal law is concerned. The finality of an acquittal is dependent on the jurisdiction. In some countries, such as the ...), a commutation does not affect the status of a defendant's underlying criminal conviction. Although the concept of commutation may be used to broadly describe the substitution of a lesser criminal penalty for the original sentence, some jurisdictions have historically used the term only for the substitution of a sentence of a different character than was originally imposed by the court. For example, the substitution of a sentence of parole for the original ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Packet Switching
In telecommunications, packet switching is a method of grouping Data (computing), data into ''network packet, packets'' that are transmitted over a digital Telecommunications network, network. Packets are made of a header (computing), header and a payload (computing), payload. Data in the header is used by networking hardware to direct the packet to its destination, where the payload is extracted and used by an operating system, application software, or protocol suite, higher layer protocols. Packet switching is the primary basis for data communications in computer networks worldwide. In the early 1960s, American computer scientist Paul Baran developed the concept that he called "distributed adaptive message block switching", with the goal of providing a fault-tolerant, efficient routing method for telecommunication messages as part of a research program at the RAND Corporation, funded by the United States Department of Defense. His ideas contradicted then-established principles ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Commutation (telemetry)
In telemetry, commutation is a process whereby multiple data streams ("measurands"), possibly with differing data rates, are combined into a single Frame (networking), frame-based stream for transmission (telecommunications), transmission, before being separated again (decommutated) upon reception; it is a form of time-division multiplexing. Frame synchronization must be achieved before a data stream can be decommutated. Etymology Commutation is named by analogy with Commutator (electric), electric commutators, which engage multiple electrical contacts in sequence as they rotate; similarly, telemetry commutation involves sampling a sequence of data points in turn, before returning to the first data point. Hardware or software which performs commutation is referred to as a commutator; its opposite at the receiving end is a decommutator. Dedicated hardware generally supports faster commutation and decommutation than software on a general purpose architecture. Mechanism A set of Wor ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Commutation (neurophysiology)
In neurophysiology, commutation is the process by which the brain's neural circuits exhibit non-commutativity. Physiologist Douglas B. Tweed and coworkers have considered whether certain neural circuits in the brain exhibit noncommutativity and state: In noncommutative algebra, order makes a difference to multiplication, so that a\times b\neq b\times a. This feature is necessary for computing rotary motion, because order makes a difference to the combined effect of two rotations. It has therefore been proposed that there are non-commutative operators in the brain circuits that deal with rotations, including motor system circuits that steer the eyes, head and limbs, and sensory system circuits that handle spatial information. This idea is controversial: studies of eye and head control have revealed behaviours that are consistent with non-commutativity in the brain, but none that clearly rules out all commutative models. Tweed goes on to demonstrate non-commutative computation ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
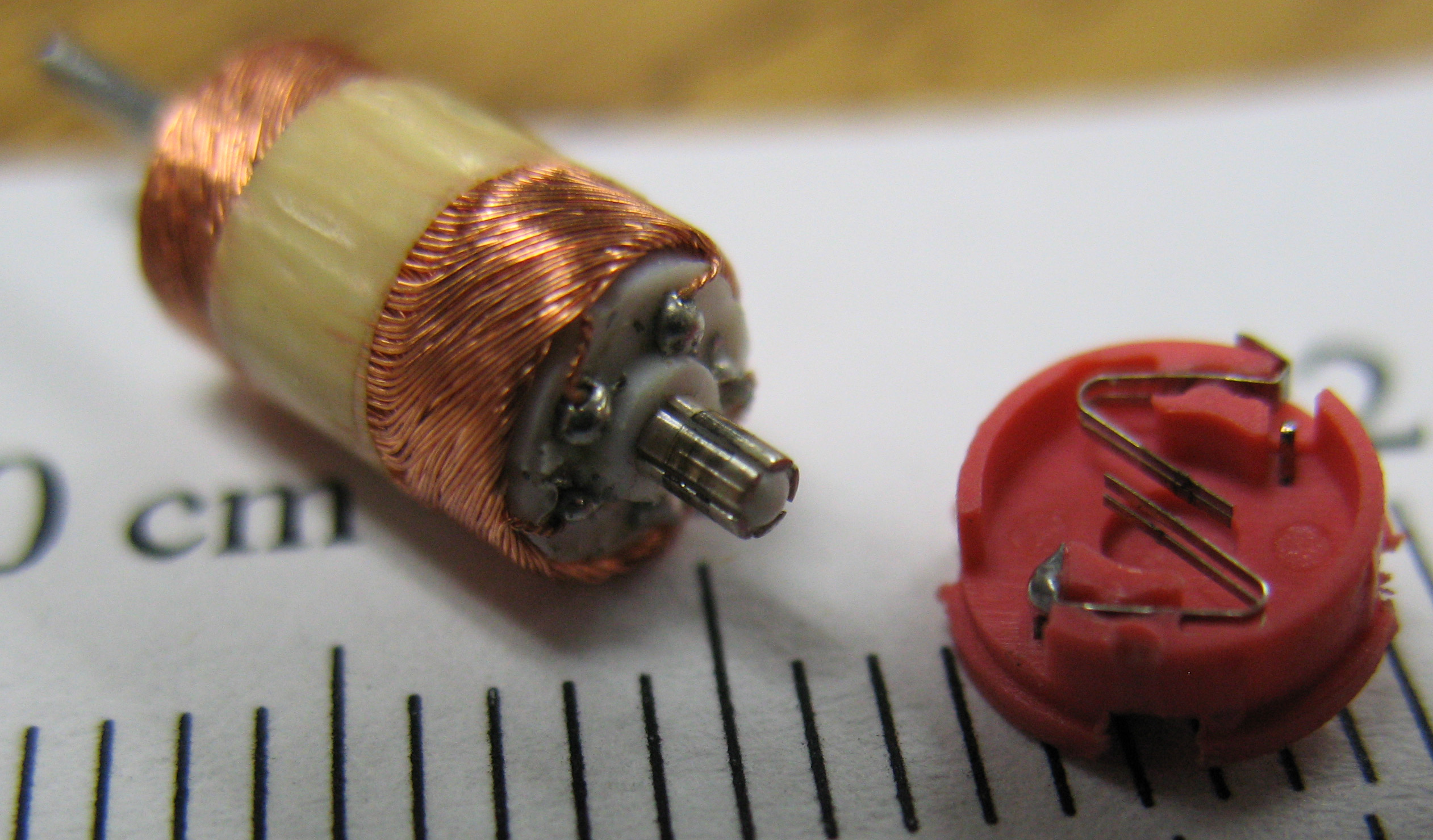
Commutator (electric)
A commutator is a rotary electrical switch in certain types of electric motors and electrical generators that periodically reverses the current direction between the rotor and the external circuit. It consists of a cylinder composed of multiple metal contact segments on the rotating armature of the machine. Two or more electrical contacts called " brushes" made of a soft conductive material like carbon press against the commutator, making sliding contact with successive segments of the commutator as it rotates. The windings (coils of wire) on the armature are connected to the commutator segments. Commutators are used in direct current (DC) machines: dynamos (DC generators) and many DC motors as well as universal motors. In a motor the commutator applies electric current to the windings. By reversing the current direction in the rotating windings each half turn, a steady rotating force (torque) is produced. In a generator the commutator picks off the current generated in ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Commuting Matrices
In linear algebra, two matrices A and B are said to commute if AB=BA, or equivalently if their commutator ,B AB-BA is zero. A set of matrices A_1, \ldots, A_k is said to commute if they commute pairwise, meaning that every pair of matrices in the set commute with each other. Characterizations and properties * Commuting matrices preserve each other's eigenspaces. As a consequence, commuting matrices over an algebraically closed field are simultaneously triangularizable; that is, there are bases over which they are both upper triangular. In other words, if A_1,\ldots,A_k commute, there exists a similarity matrix P such that P^ A_i P is upper triangular for all i \in \. The converse is not necessarily true, as the following counterexample shows: *:\begin 1 & 2 \\ 0 & 3 \end\begin 1 & 1 \\ 0 & 1 \end = \begin 1 & 3 \\ 0 & 3 \end \ne \begin 1 & 5 \\ 0 & 3 \end=\begin 1 & 1 \\ 0 & 1 \end\begin 1 & 2 \\ 0 & 3 \end. : However, if the square of the commutator of two matrices is zero, that ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Commutative Property
In mathematics, a binary operation is commutative if changing the order of the operands does not change the result. It is a fundamental property of many binary operations, and many mathematical proofs depend on it. Most familiar as the name of the property that says something like or , the property can also be used in more advanced settings. The name is needed because there are operations, such as division and subtraction, that do not have it (for example, ); such operations are ''not'' commutative, and so are referred to as ''noncommutative operations''. The idea that simple operations, such as the multiplication and addition of numbers, are commutative was for many years implicitly assumed. Thus, this property was not named until the 19th century, when mathematics started to become formalized. A similar property exists for binary relations; a binary relation is said to be symmetric if the relation applies regardless of the order of its operands; for example, equality is symme ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Commutative Ring
In mathematics, a commutative ring is a ring in which the multiplication operation is commutative. The study of commutative rings is called commutative algebra. Complementarily, noncommutative algebra is the study of ring properties that are not specific to commutative rings. This distinction results from the high number of fundamental properties of commutative rings that do not extend to noncommutative rings. Definition and first examples Definition A ''ring'' is a set R equipped with two binary operations, i.e. operations combining any two elements of the ring to a third. They are called ''addition'' and ''multiplication'' and commonly denoted by "+" and "\cdot"; e.g. a+b and a \cdot b. To form a ring these two operations have to satisfy a number of properties: the ring has to be an abelian group under addition as well as a monoid under multiplication, where multiplication distributes over addition; i.e., a \cdot \left(b + c\right) = \left(a \cdot b\right) + \left(a \cdot ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Abelian Group
In mathematics, an abelian group, also called a commutative group, is a group in which the result of applying the group operation to two group elements does not depend on the order in which they are written. That is, the group operation is commutative. With addition as an operation, the integers and the real numbers form abelian groups, and the concept of an abelian group may be viewed as a generalization of these examples. Abelian groups are named after early 19th century mathematician Niels Henrik Abel. The concept of an abelian group underlies many fundamental algebraic structures, such as fields, rings, vector spaces, and algebras. The theory of abelian groups is generally simpler than that of their non-abelian counterparts, and finite abelian groups are very well understood and fully classified. Definition An abelian group is a set A, together with an operation \cdot that combines any two elements a and b of A to form another element of A, denoted a \cdot b. The symbo ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |