|
Claude Lemaréchal
Claude Lemaréchal is a French applied mathematician, and former senior researcher (''directeur de recherche'') at INRIA near Grenoble, France. In mathematical optimization, Claude Lemaréchal is known for his work in numerical methods for nonlinear optimization, especially for problems with nondifferentiable kinks. Lemaréchal and Philip Wolfe pioneered bundle methods of descent for convex minimization.Citation of Claude Lemaréchal for the George Dantzig Prize in 1994 in ''Optima'', Issue 44 (1994) pages 4-5. Awards In 1994, Claude Lemaréchal and Roger J-B Wets were each ...[...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Mathematical Optimization
Mathematical optimization (alternatively spelled ''optimisation'') or mathematical programming is the selection of a best element, with regard to some criteria, from some set of available alternatives. It is generally divided into two subfields: discrete optimization and continuous optimization. Optimization problems arise in all quantitative disciplines from computer science and engineering to operations research and economics, and the development of solution methods has been of interest in mathematics for centuries. In the more general approach, an optimization problem consists of maxima and minima, maximizing or minimizing a Function of a real variable, real function by systematically choosing Argument of a function, input values from within an allowed set and computing the Value (mathematics), value of the function. The generalization of optimization theory and techniques to other formulations constitutes a large area of applied mathematics. Optimization problems Opti ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Philip Wolfe (mathematician)
Philip Starr "Phil" Wolfe (August 11, 1927 – December 29, 2016) was an American mathematician and one of the founders of convex optimization theory and mathematical programming. Life Wolfe received his bachelor's degree, masters, and Ph.D. degrees from the University of California, Berkeley. He and his wife, Hallie, lived in Ossining, New York. Career In 1954, he was offered an instructorship at Princeton, where he worked on generalizations of linear programming, such as quadratic programming and general non-linear programming, leading to the Frank–Wolfe algorithm in joint work with Marguerite Frank, then a visitor at Princeton. When Maurice Sion was on sabbatical at the Institute for Advanced Study, Sion and Wolfe published in 1957 an example of a zero-sum game without a minimax value. Wolfe joined RAND corporation in 1957, where he worked with George Dantzig, resulting in the now well known Dantzig–Wolfe decomposition method. In 1965, he moved to IBM Int ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Nonlinear Programming
In mathematics, nonlinear programming (NLP) is the process of solving an optimization problem where some of the constraints are not linear equalities or the objective function is not a linear function. An optimization problem is one of calculation of the extrema (maxima, minima or stationary points) of an objective function over a set of unknown real variables and conditional to the satisfaction of a system of equalities and inequalities, collectively termed constraints. It is the sub-field of mathematical optimization that deals with problems that are not linear. Definition and discussion Let ''n'', ''m'', and ''p'' be positive integers. Let ''X'' be a subset of ''Rn'' (usually a box-constrained one), let ''f'', ''gi'', and ''hj'' be real-valued functions on ''X'' for each ''i'' in and each ''j'' in , with at least one of ''f'', ''gi'', and ''hj'' being nonlinear. A nonlinear programming problem is an optimization problem of the form : \begin \text & f(x) \\ \text ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Subgradient Method
Subgradient methods are convex optimization methods which use subderivatives. Originally developed by Naum Z. Shor and others in the 1960s and 1970s, subgradient methods are convergent when applied even to a non-differentiable objective function. When the objective function is differentiable, sub-gradient methods for unconstrained problems use the same search direction as the method of gradient descent. Subgradient methods are slower than Newton's method when applied to minimize twice continuously differentiable convex functions. However, Newton's method fails to converge on problems that have non-differentiable kinks. In recent years, some interior-point methods have been suggested for convex minimization problems, but subgradient projection methods and related bundle methods of descent remain competitive. For convex minimization problems with very large number of dimensions, subgradient-projection methods are suitable, because they require little storage. Subgradient project ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Le Chesnay
Le Chesnay () is a former commune in the Yvelines department in the Île-de-France region in north-central France. On 1 January 2019, it was merged into the new commune Le Chesnay-Rocquencourt. It is located in the western suburbs of Paris, from the center of Paris. History On 1 July 1815, Napoleon's Grande Armée fought its last battle in Rocquencourt and Le Chesnay. After the defeat of Waterloo on 18 June 1815, Grouchy's army withdrew to Paris via Namur and Dinant, reaching Paris on 29 June, a few days before the Prussians, who camped at Versailles. While negotiating the final armistice, Exelmans was ordered to attack the Prussians at Versailles on 1 July 1815. Under attack the Prussians retreated from Versailles and headed east, but were blocked by the French at Vélizy. They failed to re-enter Versailles and headed for Saint-Germain-en-Laye. Their first squadron came under fire at the entrance of Rocquencourt and attempted to escape through the fields. They were ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Rocquencourt, Yvelines
Rocquencourt () is a former commune in the Yvelines department in the Île-de-France in north-central France. On 1 January 2019, it was merged into the new commune Le Chesnay-Rocquencourt. 29 November 2018 It is about north-west of and west of center . The commune is mainly known as the location of a research unit of |
Lagrangian Multiplier
In mathematical optimization, the method of Lagrange multipliers is a strategy for finding the local maxima and minima of a function subject to equation constraints (i.e., subject to the condition that one or more equations have to be satisfied exactly by the chosen values of the variables). It is named after the mathematician Joseph-Louis Lagrange. Summary and rationale The basic idea is to convert a constrained problem into a form such that the derivative test of an unconstrained problem can still be applied. The relationship between the gradient of the function and gradients of the constraints rather naturally leads to a reformulation of the original problem, known as the Lagrangian function or Lagrangian. In the general case, the Lagrangian is defined as \mathcal(x, \lambda) \equiv f(x) + \langle \lambda, g(x)\rangle for functions f, g; the notation \langle \cdot, \cdot \rangle denotes an inner product. The value \lambda is called the Lagrange multiplier. In simple case ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
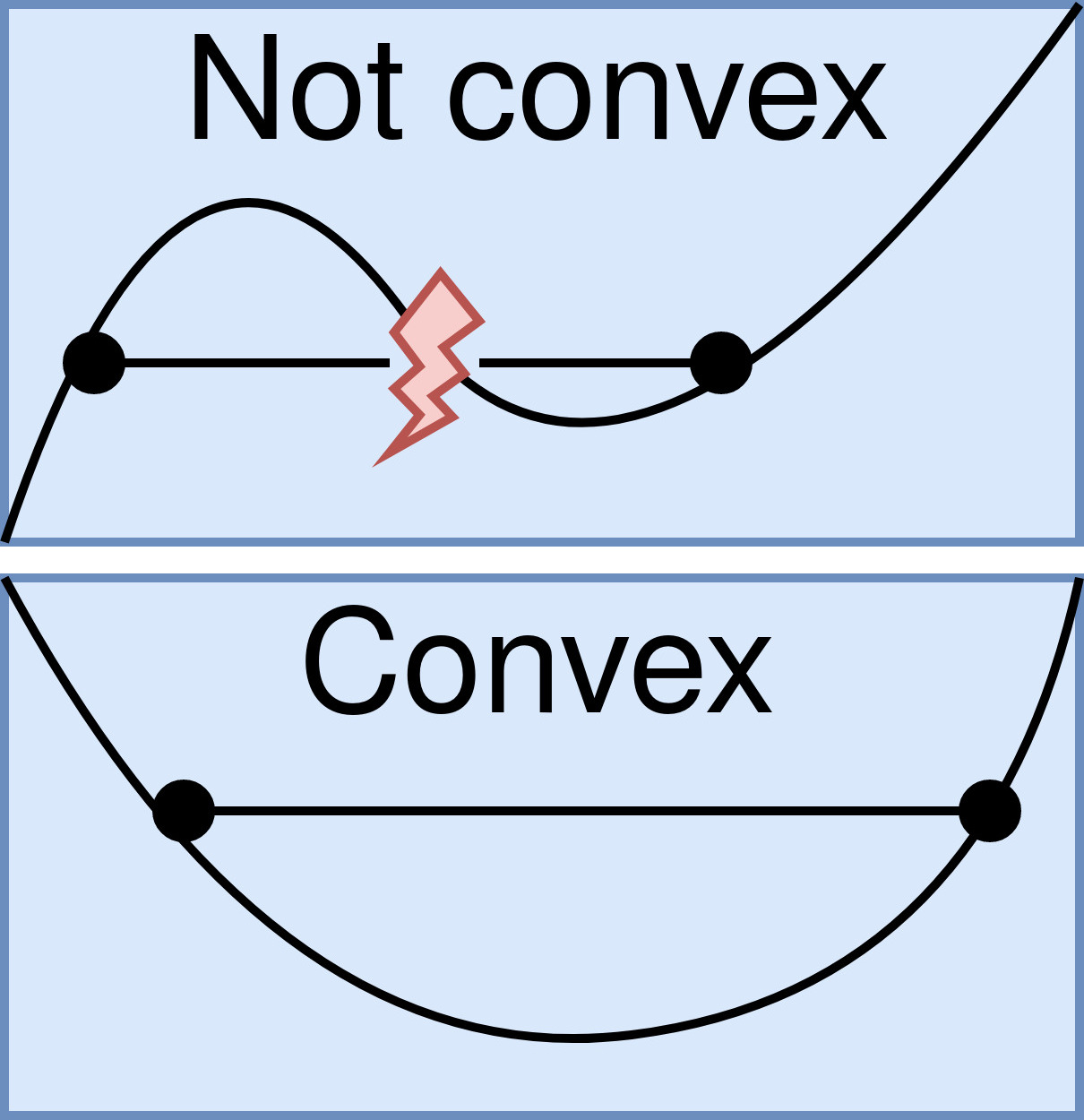
Convex Function
In mathematics, a real-valued function is called convex if the line segment between any two distinct points on the graph of a function, graph of the function lies above or on the graph between the two points. Equivalently, a function is convex if its epigraph (mathematics), ''epigraph'' (the set of points on or above the graph of the function) is a convex set. In simple terms, a convex function graph is shaped like a cup \cup (or a straight line like a linear function), while a concave function's graph is shaped like a cap \cap. A twice-differentiable function, differentiable function of a single variable is convex if and only if its second derivative is nonnegative on its entire domain of a function, domain. Well-known examples of convex functions of a single variable include a linear function f(x) = cx (where c is a real number), a quadratic function cx^2 (c as a nonnegative real number) and an exponential function ce^x (c as a nonnegative real number). Convex functions pl ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Optimization
Mathematical optimization (alternatively spelled ''optimisation'') or mathematical programming is the selection of a best element, with regard to some criteria, from some set of available alternatives. It is generally divided into two subfields: discrete optimization and continuous optimization. Optimization problems arise in all quantitative disciplines from computer science and engineering to operations research and economics, and the development of solution methods has been of interest in mathematics for centuries. In the more general approach, an optimization problem consists of maxima and minima, maximizing or minimizing a Function of a real variable, real function by systematically choosing Argument of a function, input values from within an allowed set and computing the Value (mathematics), value of the function. The generalization of optimization theory and techniques to other formulations constitutes a large area of applied mathematics. Optimization problems Opti ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Scheduling (production Processes)
Scheduling is the process of arranging, controlling and optimizing work and workloads in a Production (economics), production process or manufacturing process. Scheduling is used to allocate plant and machinery resources, plan human resources, plan production processes and purchase materials. It is an important tool for manufacturing and engineering, where it can have a major impact on the productivity of a process. In manufacturing, the purpose of scheduling is to keep due dates of customers and then minimize the production time and costs, by telling a production facility when to make, with which staff, and on which equipment. Production scheduling aims to maximize the efficiency of the operation, utilize maximum resources available and reduce costs. In some situations, scheduling can involve random attributes, such as random processing times, random due dates, random weights, and stochastic machine breakdowns. In this case, the scheduling problems are referred to as "stochastic s ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
George B
George may refer to: Names * George (given name) * George (surname) People * George (singer), American-Canadian singer George Nozuka, known by the mononym George * George Papagheorghe, also known as Jorge / GEØRGE * George, stage name of Giorgio Moroder * George, son of Andrew I of Hungary Places South Africa * George, South Africa, a city ** George Airport United States * George, Iowa, a city * George, Missouri, a ghost town * George, Washington, a city * George County, Mississippi * George Air Force Base, a former U.S. Air Force base located in California Computing * George (algebraic compiler) also known as 'Laning and Zierler system', an algebraic compiler by Laning and Zierler in 1952 * GEORGE (computer), early computer built by Argonne National Laboratory in 1957 * GEORGE (operating system), a range of operating systems (George 1–4) for the ICT 1900 range of computers in the 1960s * GEORGE (programming language), an autocode system invented by Charles Le ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |