|
Classical Central-force Problem
In classical mechanics, the central-force problem is to determine the motion of a particle in a single central potential field. A central force is a force (possibly negative) that points from the particle directly towards a fixed point in space, the center, and whose magnitude only depends on the distance of the object to the center. In a few important cases, the problem can be solved analytically, i.e., in terms of well-studied functions such as trigonometric functions. The solution of this problem is important to classical mechanics, since many naturally occurring forces are central. Examples include gravity and electromagnetism as described by Newton's law of universal gravitation and Coulomb's law, respectively. The problem is also important because some more complicated problems in classical physics (such as the two-body problem with forces along the line connecting the two bodies) can be reduced to a central-force problem. Finally, the solution to the central-force proble ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Central Force
In classical mechanics, a central force on an object is a force that is directed towards or away from a point called center of force. : \vec = \mathbf(\mathbf) = \left\vert F( \mathbf ) \right\vert \hat where \vec F is the force, F is a vector valued force function, ''F'' is a scalar valued force function, r is the position vector, , , r, , is its length, and \hat = \mathbf r / \, \mathbf r\, is the corresponding unit vector. Not all central force fields are conservative or spherically symmetric. However, a central force is conservative if and only if it is spherically symmetric or rotationally invariant. Properties Central forces that are conservative can always be expressed as the negative gradient of a potential energy:- : \mathbf(\mathbf) = - \mathbf V(\mathbf)\textV(\mathbf) = \int_^ F(r)\,\mathrmr (the upper bound of integration is arbitrary, as the potential is defined up to an additive constant). In a conservative field, the total mechanical energy (kinetic and p ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Kinetic Energy
In physics, the kinetic energy of an object is the energy that it possesses due to its motion. It is defined as the work needed to accelerate a body of a given mass from rest to its stated velocity. Having gained this energy during its acceleration, the body maintains this kinetic energy unless its speed changes. The same amount of work is done by the body when decelerating from its current speed to a state of rest. Formally, a kinetic energy is any term in a system's Lagrangian which includes a derivative with respect to time. In classical mechanics, the kinetic energy of a non-rotating object of mass ''m'' traveling at a speed ''v'' is \fracmv^2. In relativistic mechanics, this is a good approximation only when ''v'' is much less than the speed of light. The standard unit of kinetic energy is the joule, while the English unit of kinetic energy is the foot-pound. History and etymology The adjective ''kinetic'' has its roots in the Greek word κίνησις ''kinesis'', m ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Angular Momentum
In physics, angular momentum (rarely, moment of momentum or rotational momentum) is the rotational analog of linear momentum. It is an important physical quantity because it is a conserved quantity—the total angular momentum of a closed system remains constant. Angular momentum has both a direction and a magnitude, and both are conserved. Bicycles and motorcycles, frisbees, rifled bullets, and gyroscopes owe their useful properties to conservation of angular momentum. Conservation of angular momentum is also why hurricanes form spirals and neutron stars have high rotational rates. In general, conservation limits the possible motion of a system, but it does not uniquely determine it. The three-dimensional angular momentum for a point particle is classically represented as a pseudovector , the cross product of the particle's position vector (relative to some origin) and its momentum vector; the latter is in Newtonian mechanics. Unlike linear momentum, angular m ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Particle In A Spherically Symmetric Potential
In the quantum mechanics description of a particle in spherical coordinates, a spherically symmetric potential, is a potential that depends only on the distance between the particle and a defined centre point. One example of a spherical potential is the electron within a hydrogen atom. The electron's potential depends only on its distance from the proton in the atom's nucleus. This spherical potential can be derived from Coulomb's law. In the general case, the dynamics of a particle in a spherically symmetric potential are governed by a Hamiltonian of the following form: \hat = \frac + V(r) Where m_0 is the mass of the particle, \hat is the momentum operator, and the potential V(r) depends only on r, the modulus of the radius vector. The possible quantum states of the particle are found by using the above Hamiltonian to solve the Schrödinger equation for its eigenvalues, which are wave functions. To describe these spherically symmetric systems, it is natural to use spheri ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Quantum Mechanics
Quantum mechanics is a fundamental theory in physics that provides a description of the physical properties of nature at the scale of atoms and subatomic particles. It is the foundation of all quantum physics including quantum chemistry, quantum field theory, quantum technology, and quantum information science. Classical physics, the collection of theories that existed before the advent of quantum mechanics, describes many aspects of nature at an ordinary (macroscopic) scale, but is not sufficient for describing them at small (atomic and subatomic) scales. Most theories in classical physics can be derived from quantum mechanics as an approximation valid at large (macroscopic) scale. Quantum mechanics differs from classical physics in that energy, momentum, angular momentum, and other quantities of a bound system are restricted to discrete values ( quantization); objects have characteristics of both particles and waves (wave–particle duality); and there are limits to ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
General Relativity
General relativity, also known as the general theory of relativity and Einstein's theory of gravity, is the geometric theory of gravitation published by Albert Einstein in 1915 and is the current description of gravitation in modern physics. General relativity generalizes special relativity and refines Newton's law of universal gravitation, providing a unified description of gravity as a geometric property of space and time or four-dimensional spacetime. In particular, the ' is directly related to the energy and momentum of whatever matter and radiation are present. The relation is specified by the Einstein field equations, a system of second order partial differential equations. Newton's law of universal gravitation, which describes classical gravity, can be seen as a prediction of general relativity for the almost flat spacetime geometry around stationary mass distributions. Some predictions of general relativity, however, are beyond Newton's law of universal gravitat ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
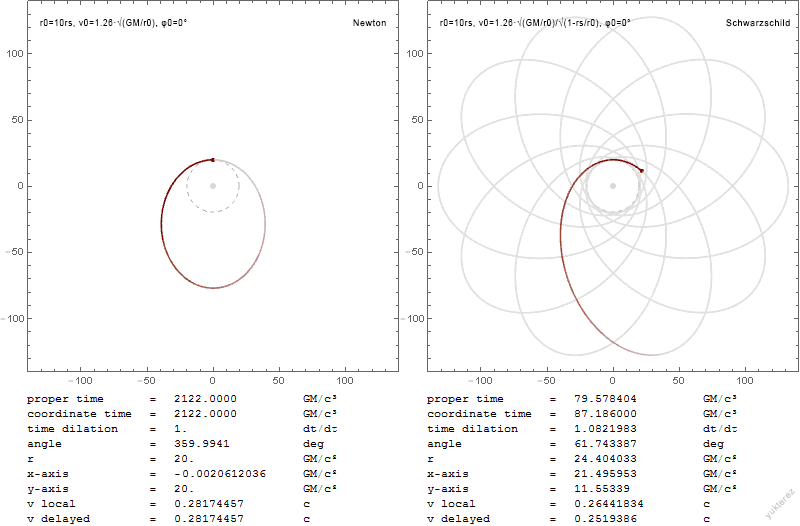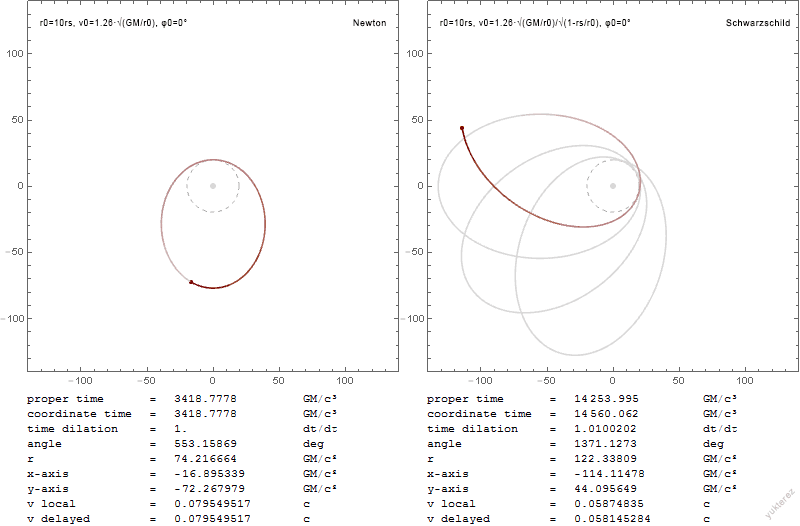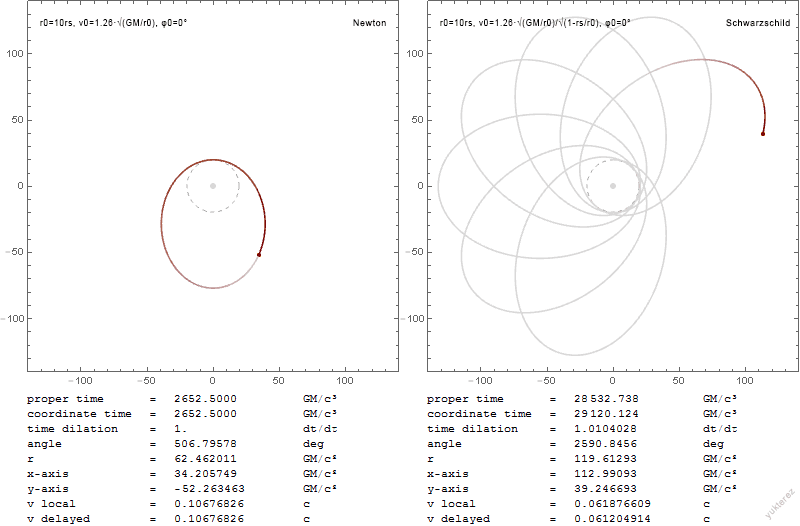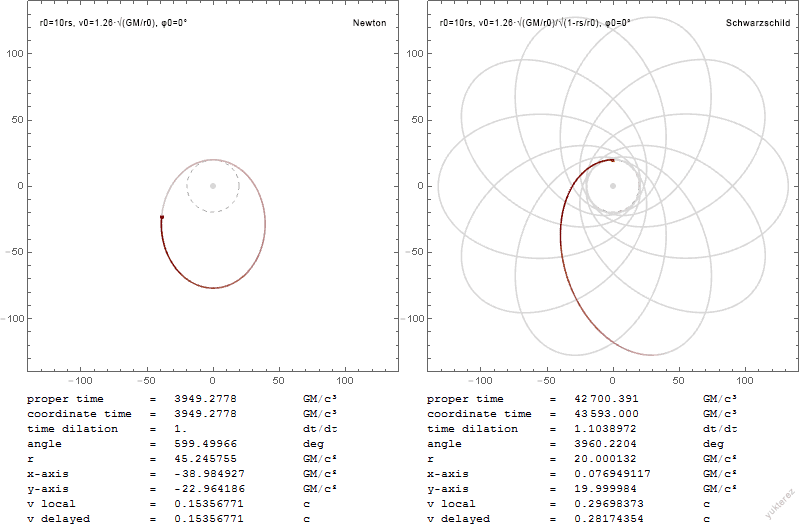
Schwarzschild Geodesics
In general relativity, Schwarzschild geodesics describe the motion of test particles in the gravitational field of a central fixed mass M, that is, motion in the Schwarzschild metric. Schwarzschild geodesics have been pivotal in the validation of Einstein's theory of general relativity. For example, they provide accurate predictions of the anomalous precession of the planets in the Solar System and of the deflection of light by gravity. Schwarzschild geodesics pertain only to the motion of particles of masses so small they contribute little to the gravitational field. However, they are highly accurate in many astrophysical scenarios provided that m is many-fold smaller than the central mass M, e.g., for planets orbiting their sun. Schwarzschild geodesics are also a good approximation to the relative motion of two bodies of arbitrary mass, provided that the Schwarzschild mass M is set equal to the sum of the two individual masses m_1 and m_2. This is important in predicting the mo ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Spherical Symmetry
In geometry, circular symmetry is a type of continuous symmetry for a planar object that can be rotated by any arbitrary angle and map onto itself. Rotational circular symmetry is isomorphic with the circle group in the complex plane, or the special orthogonal group SO(2), and unitary group U(1). Reflective circular symmetry is isomorphic with the orthogonal group O(2). Two dimensions A 2-dimensional object with circular symmetry would consist of concentric circles and annular domains. Rotational circular symmetry has all cyclic symmetry, Z''n'' as subgroup symmetries. Reflective circular symmetry has all dihedral symmetry, Dih''n'' as subgroup symmetries. Three dimensions In 3-dimensions, a surface or solid of revolution has circular symmetry around an axis, also called cylindrical symmetry or axial symmetry. An example is a right circular cone. Circular symmetry in 3 dimensions has all pyramidal symmetry, C''n''v as subgroups. A double-cone, bicone, cylinder, toro ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Scalar Potential
In mathematical physics, scalar potential, simply stated, describes the situation where the difference in the potential energies of an object in two different positions depends only on the positions, not upon the path taken by the object in traveling from one position to the other. It is a scalar field in three-space: a directionless value (scalar) that depends only on its location. A familiar example is potential energy due to gravity. A ''scalar potential'' is a fundamental concept in vector analysis and physics (the adjective ''scalar'' is frequently omitted if there is no danger of confusion with ''vector potential''). The scalar potential is an example of a scalar field. Given a vector field , the scalar potential is defined such that: : \mathbf = -\nabla P = - \left( \frac, \frac, \frac \right), where is the gradient of and the second part of the equation is minus the gradient for a function of the Cartesian coordinates . In some cases, mathematicians may use ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Spherical Coordinate System
In mathematics, a spherical coordinate system is a coordinate system for three-dimensional space where the position of a point is specified by three numbers: the ''radial distance'' of that point from a fixed origin, its ''polar angle'' measured from a fixed zenith direction, and the ''azimuthal angle'' of its orthogonal projection on a reference plane that passes through the origin and is orthogonal to the zenith, measured from a fixed reference direction on that plane. It can be seen as the three-dimensional version of the polar coordinate system. The radial distance is also called the ''radius'' or ''radial coordinate''. The polar angle may be called '' colatitude'', ''zenith angle'', '' normal angle'', or ''inclination angle''. When radius is fixed, the two angular coordinates make a coordinate system on the sphere sometimes called spherical polar coordinates. The use of symbols and the order of the coordinates differs among sources and disciplines. This article will us ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Partial Derivative
In mathematics, a partial derivative of a function of several variables is its derivative with respect to one of those variables, with the others held constant (as opposed to the total derivative, in which all variables are allowed to vary). Partial derivatives are used in vector calculus and differential geometry. The partial derivative of a function f(x, y, \dots) with respect to the variable x is variously denoted by It can be thought of as the rate of change of the function in the x-direction. Sometimes, for z=f(x, y, \ldots), the partial derivative of z with respect to x is denoted as \tfrac. Since a partial derivative generally has the same arguments as the original function, its functional dependence is sometimes explicitly signified by the notation, such as in: :f'_x(x, y, \ldots), \frac (x, y, \ldots). The symbol used to denote partial derivatives is ∂. One of the first known uses of this symbol in mathematics is by Marquis de Condorcet from 1770, who used it for ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Del In Cylindrical And Spherical Coordinates
This is a list of some vector calculus formulae for working with common curvilinear coordinates, curvilinear coordinate systems. Notes * This article uses the standard notation ISO 80000-2, which supersedes ISO 31-11#Coordinate systems, ISO 31-11, for spherical coordinate system, spherical coordinates (other sources may reverse the definitions of ''θ'' and ''φ''): ** The polar angle is denoted by \theta \in [0, \pi]: it is the angle between the ''z''-axis and the radial vector connecting the origin to the point in question. ** The azimuthal angle is denoted by \varphi \in [0, 2\pi]: it is the angle between the ''x''-axis and the projection of the radial vector onto the ''xy''-plane. * The function can be used instead of the mathematical function owing to its Domain of a function, domain and Image (mathematics), image. The classical arctan function has an image of , whereas atan2 is defined to have an image of . Coordinate conversions CAUTION: the operation \arctan\lef ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
