|
Call Provisions
In finance, a call option, often simply labeled a "call", is a contract between the buyer and the seller of the call option to exchange a security at a set price. The buyer of the call option has the right, but not the obligation, to buy an agreed quantity of a particular commodity or financial instrument (the underlying) from the seller of the option at a certain time (the expiration date) for a certain price (the strike price). This effectively gives the owner a ''long'' position in the given asset. The seller (or "writer") is obliged to sell the commodity or financial instrument to the buyer if the buyer so decides. This effectively gives the seller a ''short'' position in the given asset. The buyer pays a fee (called a premium) for this right. The term "call" comes from the fact that the owner has the right to "call the stock away" from the seller. Price of options Option values vary with the value of the underlying instrument over time. The price of the call contrac ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Long Call Option
Long may refer to: Measurement * Long, characteristic of something of great duration * Long, characteristic of something of great length * Longitude (abbreviation: long.), a geographic coordinate * Longa (music), note value in early music mensural notation Places Asia * Long District, Laos * Long District, Phrae, Thailand * Longjiang (other) or River Long (lit. "dragon river"), one of several rivers in China * Yangtze River or Changjiang (lit. "Long River"), China Elsewhere * Long, Somme, France * Long, Washington, United States People * Long (surname) * Long (surname 龍) (Chinese surname) Fictional characters * Long (''Bloody Roar''), in the video game series Sports * Long, a fielding term in cricket * Long, in tennis and similar games, beyond the service line during a serve and beyond the baseline during play Other uses * , a U.S. Navy ship name * Long (finance), a position in finance, especially stock markets * Lòng, name for a laneway in Shanghai * Long in ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
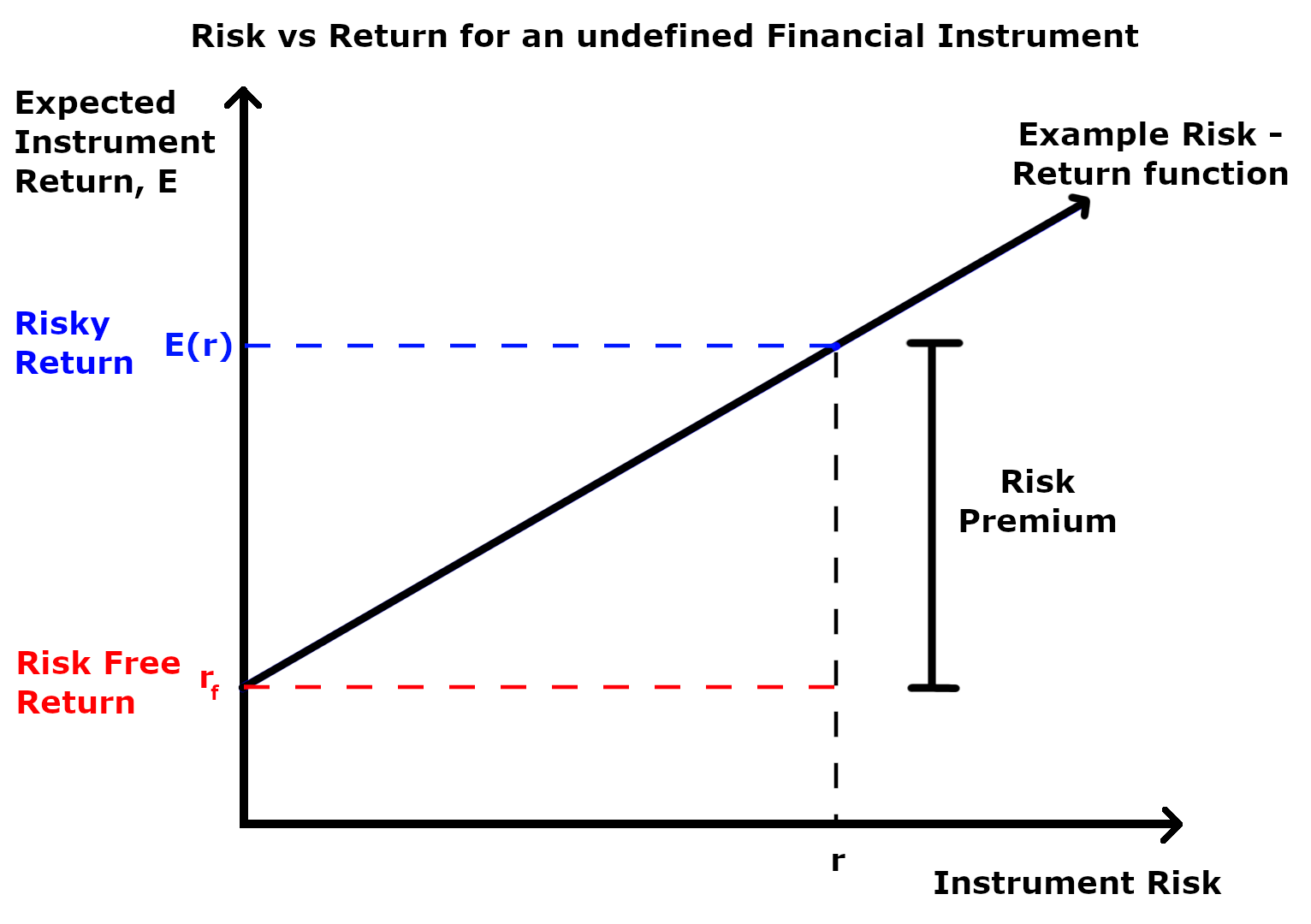
Risk Premium
A risk premium is a measure of excess return that is required by an individual to compensate being subjected to an increased level of risk. It is used widely in finance and economics, the general definition being the expected risky return less the risk-free return, as demonstrated by the formula below. Risk \ premium = E(r) - r_f Where E(r) is the risky expected rate of return and r_f is the risk-free return. The inputs for each of these variables and the ultimate interpretation of the risk premium value differs depending on the application as explained in the following sections. Regardless of the application, the market premium can be volatile as both comprising variables can be impacted independent of each other by both cyclical and abrupt changes. This means that the market premium is dynamic in nature and ever-changing. Additionally, a general observation regardless of application is that the risk premium is larger during economic downturns and during periods of increased u ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Put–call Parity
In financial mathematics, put–call parity defines a relationship between the price of a European call option and European put option, both with the identical strike price and expiry, namely that a portfolio of a long call option and a short put option is equivalent to (and hence has the same value as) a single forward contract at this strike price and expiry. This is because if the price at expiry is above the strike price, the call will be exercised, while if it is below, the put will be exercised, and thus in either case one unit of the asset will be purchased for the strike price, exactly as in a forward contract. The validity of this relationship requires that certain assumptions be satisfied; these are specified and the relationship is derived below. In practice transaction costs and financing costs (leverage) mean this relationship will not exactly hold, but in liquid markets the relationship is close to exact. Assumptions Put–call parity is a static replication, and t ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Put Option
In finance, a put or put option is a derivative instrument in financial markets that gives the holder (i.e. the purchaser of the put option) the right to sell an asset (the ''underlying''), at a specified price (the ''strike''), by (or at) a specified date (the ''expiry'' or ''maturity'') to the ''writer'' (i.e. seller) of the put. The purchase of a put option is interpreted as a negative sentiment about the future value of the underlying stock. page 15 , 4.2.3 Positive and negative sentiment The term "put" comes from the fact that the owner has the right to "put up for sale" the stock or index. Puts may also be combined with other derivatives as part of more complex investment strategies, and in particular, may be useful for hedging. Holding a European put option is equivalent to holding the corresponding call option and selling an appropriate forward contract. This equivalence is called " put-call parity". Put options are most commonly used in the stock market to protect ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Pre-emption Right
A pre-emption right, right of pre-emption, or first option to buy is a contractual right to acquire certain property newly coming into existence before it can be offered to any other person or entity. It comes from the Latin verb ''emo, emere, emi, emptum'', to buy or purchase, plus the inseparable preposition ''pre'', before. A right to acquire existing property in preference to any other person is usually referred to as a ''right of first refusal''. Company shares In practice, the most common form of pre-emption right is the right of existing shareholders to acquire new shares issued by a company in a rights issue, usually a public offering. In this context, the pre-emptive right is also called subscription right or subscription privilege. It is the right but not the obligation of existing shareholders to buy the new shares before they are offered to the public. In that way, existing shareholders can maintain their proportional ownership of the company and thus prevent stock dilut ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Option Time Value
In finance, the time value (TV) (''extrinsic'' or ''instrumental'' value) of an option is the premium a rational investor would pay over its ''current'' exercise value ( intrinsic value), based on the probability it will increase in value before expiry. For an American option this value is always greater than zero in a fair market, thus an option is ''always'' worth more than its current exercise value. As an option can be thought of as 'price insurance' (e.g., an airline insuring against unexpected soaring fuel costs caused by a hurricane), TV can be thought of as the ''risk premium'' the option seller charges the buyer—the higher the expected risk (volatility \cdot time), the higher the premium. Conversely, TV can be thought of as the price an investor is willing to pay for potential upside. Time value decays to zero at expiration, with a general rule that it will lose of its value during the first half of its life and in the second half. As an option moves closer to expiry, m ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Naked Put
A naked option or uncovered option is an options contract where the option writer (i.e., the seller) does not hold the underlying security position to cover the contract in case of assignment (like in a covered option). Nor does the seller hold any option of the same class on the same underlying security that could protect against potential losses (like in an options spread). A naked option involving a "call" is called a "naked call" or "uncovered call", while one involving a " put" is a "naked put" or "uncovered put". The naked option is one of riskiest options strategies, and therefore most brokers restrict them to only those traders that have the highest options level approval and have a margin account. Naked option are attractive because the seller receives the premium cost of the option without buying a corresponding position to hedge against potential losses. In the case of a naked put, the seller hopes that the underlying equity or stock price stays the same or rises. In t ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Naked Call
A naked option or uncovered option is an options contract where the option writer (i.e., the seller) does not hold the underlying security position to cover the contract in case of assignment (like in a covered option). Nor does the seller hold any option of the same class on the same underlying security that could protect against potential losses (like in an options spread). A naked option involving a "call" is called a "naked call" or "uncovered call", while one involving a " put" is a "naked put" or "uncovered put". The naked option is one of riskiest options strategies, and therefore most brokers restrict them to only those traders that have the highest options level approval and have a margin account. Naked option are attractive because the seller receives the premium cost of the option without buying a corresponding position to hedge against potential losses. In the case of a naked put, the seller hopes that the underlying equity or stock price stays the same or rises. In t ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Moneyness
In finance Finance is the study and discipline of money, currency and capital assets. It is related to, but not synonymous with economics, the study of production, distribution, and consumption of money, assets, goods and services (the discipline of fina ..., moneyness is the relative position of the current price (or future price) of an underlying Financial asset, asset (e.g., a stock) with respect to the strike price of a derivative (finance), derivative, most commonly a call option or a put option. Moneyness is firstly a three-fold classification: * If the derivative would have positive intrinsic value (finance), intrinsic value if it were to Expiration (options), expire today, it is said to be in the money; * If the derivative would be worthless if expiring with the underlying at its current price, it is said to be out of the money; * And if the current underlying price and strike price are equal, the derivative is said to be at the money. There are two slightly differe ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Covered Call
A covered option is a financial transaction in which the holder of securities sells (or "writes") a type of financial options contract known as a "call" or a " put" against stock that they own or are shorting. The seller of a covered option receives compensation, or "premium", for this transaction, which can limit losses; however, the act of selling a covered option also limits their profit potential to the upside. One covered option is sold for every hundred shares the seller wishes to cover. A covered option constructed with a call is called a "covered call", while one constructed with a put is a "covered put". This strategy is generally considered conservative because the seller of a covered option reduces both their risk and their return. Characteristics Covered calls are bullish by nature, while covered puts are bearish. The payoff from selling a covered call is identical to selling a short naked put. Both variants are a short implied volatility strategy. Covered calls c ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Financial Mathematics
Mathematical finance, also known as quantitative finance and financial mathematics, is a field of applied mathematics, concerned with mathematical modeling of financial markets. In general, there exist two separate branches of finance that require advanced quantitative techniques: derivatives pricing on the one hand, and risk and portfolio management on the other. Mathematical finance overlaps heavily with the fields of computational finance and financial engineering. The latter focuses on applications and modeling, often by help of stochastic asset models, while the former focuses, in addition to analysis, on building tools of implementation for the models. Also related is quantitative investing, which relies on statistical and numerical models (and lately machine learning) as opposed to traditional fundamental analysis when managing portfolios. French mathematician Louis Bachelier's doctoral thesis, defended in 1900, is considered the first scholarly work on mathematical finan ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
