|
Cahill–Keyes Projection
The Cahill–Keyes projection is a polyhedral compromise map projection first proposed by Gene Keyes in 1975. The projection is a refinement of an earlier 1909 projection by Bernard Cahill. The projection was designed to achieve a number of desirable characteristics, namely symmetry of component maps (octants), scalability allowing the map to continue to work well even at high resolution, uniformity of geocells, metric-based joining edges, minimized distortion compared to a globe, and an easily understood orientation to enhance general usability and teachability. Construction The Cahill–Keyes projection was designed with four fundamental considerations in mind: visual fidelity to a globe, proportional geocells, 10,000 km lengths for each of its octants' three main joined edges, and an M-shape Master-Map profile. The resulting map comprises 8 octants. Each octant is an equilateral triangle with three segments per side. One side runs along the equator, and the oth ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Meridian (geography)
In geography and geodesy, a meridian is the locus connecting points of equal longitude, which is the angle (in degrees or other units) east or west of a given prime meridian (currently, the IERS Reference Meridian). In other words, it is a line of longitude. The position of a point along the meridian is given by that longitude and its latitude, measured in angular degrees north or south of the Equator. On a Mercator projection or on a Gall-Peters projection, each meridian is perpendicular to all circles of latitude. A meridian is half of a great circle on Earth's surface. The length of a meridian on a modern ellipsoid model of Earth (WGS 84) has been estimated as . Pre-Greenwich The first prime meridian was set by Eratosthenes in 200 BCE. This prime meridian was used to provide measurement of the earth, but had many problems because of the lack of latitude measurement. Many years later around the 19th century there were still concerns of the prime meridian. Multiple loc ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Dymaxion Map
The Dymaxion map or Fuller map is a projection of a world map onto the surface of an icosahedron, which can be unfolded and flattened to two dimensions. The flat map is heavily interrupted in order to preserve shapes and sizes. The projection was invented by Buckminster Fuller. The March 1, 1943, edition of ''Life'' magazine included a photographic essay titled "Life Presents R. Buckminster Fuller's Dymaxion World". The article included several examples of its use together with a pull-out section that could be assembled as a "three-dimensional approximation of a globe or laid out as a flat map, with which the world may be fitted together and rearranged to illuminate special aspects of its geography." Fuller applied for a patent in the United States in February 1944, showing a projection onto a cuboctahedron, which he called "dymaxion". The patent was issued in January 1946. In 1954, Fuller and cartographer Shoji Sadao produced the Airocean World Map, a version of the Dymaxion ma ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
List Of Map Projections
This is a summary of map projections that have articles of their own on Wikipedia or that are otherwise notable Notability is the property of being worthy of notice, having fame, or being considered to be of a high degree of interest, significance, or distinction. It also refers to the capacity to be such. Persons who are notable due to public responsibi .... Because there is no limit to the number of possible map projections, there can be no comprehensive list. Table of projections *The first known popularizer/user and not necessarily the creator. Key Type of projection ; Cylindrical: In standard presentation, these map regularly-spaced meridians to equally spaced vertical lines, and parallels to horizontal lines. ; Pseudocylindrical: In standard presentation, these map the central meridian and parallels as straight lines. Other meridians are curves (or possibly straight from pole to equator), regularly spaced along parallels. ; Conic: In standard presentation, conic ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Map Projection
In cartography, map projection is the term used to describe a broad set of transformations employed to represent the two-dimensional curved surface of a globe on a plane. In a map projection, coordinates, often expressed as latitude and longitude, of locations from the surface of the globe are transformed to coordinates on a plane. Projection is a necessary step in creating a two-dimensional map and is one of the essential elements of cartography. All projections of a sphere on a plane necessarily distort the surface in some way and to some extent. Depending on the purpose of the map, some distortions are acceptable and others are not; therefore, different map projections exist in order to preserve some properties of the sphere-like body at the expense of other properties. The study of map projections is primarily about the characterization of their distortions. There is no limit to the number of possible map projections. More generally, projections are considered in several fi ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
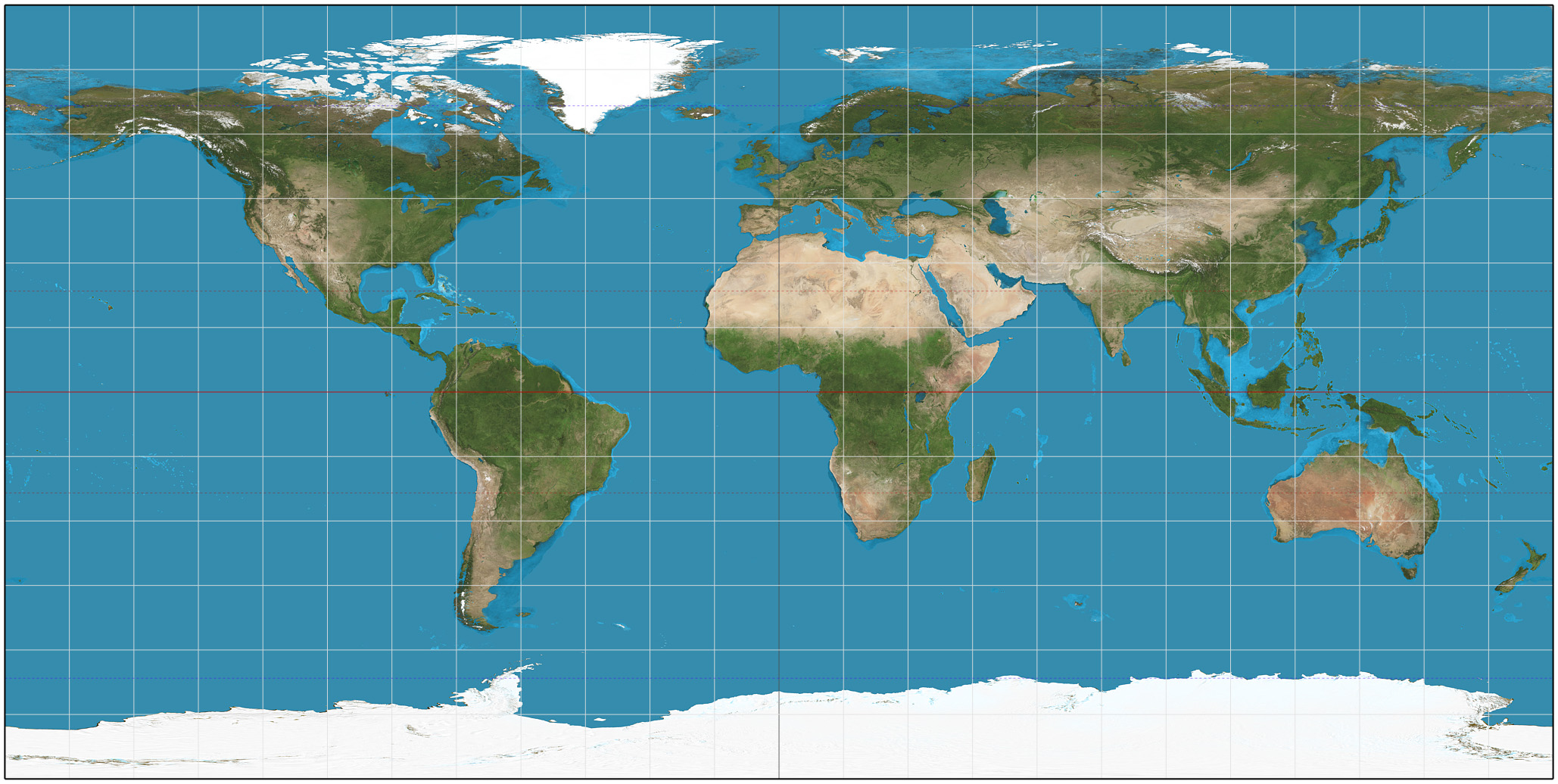
World Map
A world map is a map of most or all of the surface of Earth. World maps, because of their scale, must deal with the problem of map projection, projection. Maps rendered in two dimensions by necessity distort the display of the three-dimensional surface of the earth. While this is true of any map, these distortions reach extremes in a world map. Many techniques have been developed to present world maps that address diverse technical and aesthetic goals. Charting a world map requires global knowledge of the earth, its oceans, and its continents. From prehistory through the Middle ages, creating an accurate world map would have been impossible because less than half of Earth's coastlines and only a small fraction of its continental interiors were known to any culture. With exploration that began during the European Renaissance, knowledge of the Earth's surface accumulated rapidly, such that most of the world's coastlines had been mapped, at least roughly, by the mid-1700s and the ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Cartography
Cartography (; from grc, χάρτης , "papyrus, sheet of paper, map"; and , "write") is the study and practice of making and using maps. Combining science, aesthetics and technique, cartography builds on the premise that reality (or an imagined reality) can be modeled in ways that communicate spatial information effectively. The fundamental objectives of traditional cartography are to: * Set the map's agenda and select traits of the object to be mapped. This is the concern of map editing. Traits may be physical, such as roads or land masses, or may be abstract, such as toponyms or political boundaries. * Represent the terrain of the mapped object on flat media. This is the concern of map projections. * Eliminate characteristics of the mapped object that are not relevant to the map's purpose. This is the concern of generalization. * Reduce the complexity of the characteristics that will be mapped. This is also the concern of generalization. * Orchestrate the elements of the ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Geographic Coordinate System
The geographic coordinate system (GCS) is a spherical or ellipsoidal coordinate system for measuring and communicating positions directly on the Earth as latitude and longitude. It is the simplest, oldest and most widely used of the various spatial reference systems that are in use, and forms the basis for most others. Although latitude and longitude form a coordinate tuple like a cartesian coordinate system, the geographic coordinate system is not cartesian because the measurements are angles and are not on a planar surface. A full GCS specification, such as those listed in the EPSG and ISO 19111 standards, also includes a choice of geodetic datum (including an Earth ellipsoid), as different datums will yield different latitude and longitude values for the same location. History The invention of a geographic coordinate system is generally credited to Eratosthenes of Cyrene, who composed his now-lost ''Geography'' at the Library of Alexandria in the 3rd century ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Geographical Pole
A geographical pole or geographic pole is either of the two points on Earth where its axis of rotation intersects its surface. The North Pole lies in the Arctic Ocean while the South Pole is in Antarctica. North and South poles are also defined for other planets or satellites in the Solar System, with a North pole being on the same side of the invariable plane as Earth's North pole. Relative to Earth's surface, the geographic poles move by a few metres over periods of a few years. This is a combination of Chandler wobble, a free oscillation with a period of about 433 days; an annual motion responding to seasonal movements of air and water masses; and an irregular drift towards the 80th west meridian. As cartography requires exact and unchanging coordinates, the averaged locations of geographical poles are taken as fixed ''cartographic poles'' and become the points where the body's great circles of longitude intersect. See also * Earth's rotation * Polar motion * Poles of as ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Equator
The equator is a circle of latitude, about in circumference, that divides Earth into the Northern and Southern hemispheres. It is an imaginary line located at 0 degrees latitude, halfway between the North and South poles. The term can also be used for any other celestial body that is roughly spherical. In spatial (3D) geometry, as applied in astronomy, the equator of a rotating spheroid (such as a planet) is the parallel (circle of latitude) at which latitude is defined to be 0°. It is an imaginary line on the spheroid, equidistant from its poles, dividing it into northern and southern hemispheres. In other words, it is the intersection of the spheroid with the plane perpendicular to its axis of rotation and midway between its geographical poles. On and near the equator (on Earth), noontime sunlight appears almost directly overhead (no more than about 23° from the zenith) every day, year-round. Consequently, the equator has a rather stable daytime temperature throug ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Equilateral Triangle
In geometry, an equilateral triangle is a triangle in which all three sides have the same length. In the familiar Euclidean geometry, an equilateral triangle is also equiangular; that is, all three internal angles are also congruent to each other and are each 60°. It is also a regular polygon, so it is also referred to as a regular triangle. Principal properties Denoting the common length of the sides of the equilateral triangle as a, we can determine using the Pythagorean theorem that: *The area is A=\frac a^2, *The perimeter is p=3a\,\! *The radius of the circumscribed circle is R = \frac *The radius of the inscribed circle is r=\frac a or r=\frac *The geometric center of the triangle is the center of the circumscribed and inscribed circles *The altitude (height) from any side is h=\frac a Denoting the radius of the circumscribed circle as ''R'', we can determine using trigonometry that: *The area of the triangle is \mathrm=\fracR^2 Many of these quantities have simple r ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |