|
Branching Process
In probability theory, a branching process is a type of mathematical object known as a stochastic process, which consists of collections of random variables indexed by some set, usually natural or non-negative real numbers. The original purpose of branching processes was to serve as a mathematical model of a population in which each individual in generation n produces some random number of individuals in generation n+1, according, in the simplest case, to a fixed probability distribution that does not vary from individual to individual. Branching processes are used to model reproduction; for example, the individuals might correspond to bacteria, each of which generates 0, 1, or 2 offspring with some probability in a single time unit. Branching processes can also be used to model other systems with similar dynamics, e.g., the spread of surnames in genealogy or the propagation of neutrons in a nuclear reactor. A central question in the theory of branchi ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Probability Theory
Probability theory or probability calculus is the branch of mathematics concerned with probability. Although there are several different probability interpretations, probability theory treats the concept in a rigorous mathematical manner by expressing it through a set of axioms of probability, axioms. Typically these axioms formalise probability in terms of a probability space, which assigns a measure (mathematics), measure taking values between 0 and 1, termed the probability measure, to a set of outcomes called the sample space. Any specified subset of the sample space is called an event (probability theory), event. Central subjects in probability theory include discrete and continuous random variables, probability distributions, and stochastic processes (which provide mathematical abstractions of determinism, non-deterministic or uncertain processes or measured Quantity, quantities that may either be single occurrences or evolve over time in a random fashion). Although it is no ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Independent And Identically Distributed Random Variables
Independent or Independents may refer to: Arts, entertainment, and media Artist groups * Independents (artist group), a group of modernist painters based in Pennsylvania, United States * Independentes (English: Independents), a Portuguese artist group Music Groups, labels, and genres * Independent music, a number of genres associated with independent labels * Independent record label, a record label not associated with a major label * Independent Albums, American albums chart Albums * ''Independent'' (Ai album), 2012 * ''Independent'' (Faze album), 2006 * ''Independent'' (Sacred Reich album), 1993 Songs * "Independent" (song), a 2007 song by Webbie * "Independent", a 2002 song by Ayumi Hamasaki from '' H'' News media organizations * Independent Media Center (also known as Indymedia or IMC), an open publishing network of journalist collectives that report on political and social issues, e.g., in ''The Indypendent'' newspaper of NYC * ITV (TV network) (Independent Televi ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Bruss–Duerinckx Theorem
The theorem of the envelopment of societies for resource-dependent populations, also called the Bruss–Duerinckx theorem, is a mathematical result on the behavior of populations that choose their society form according to only two hypotheses that they see as most "natural": * Hypothesis 1 (H1): Individuals want to survive and see a future for their descendants. * Hypothesis 2 (H2): The average individual prefers a higher standard of living to a lower one. H1 is supposed to precede H2 in the case of incompatibility with H2. Here populations with a society structure are modeled by so-called resource-dependent branching processes (RDBPs). The objective of RDBPs is to model different society structures and to compare the advantages and disadvantages of different societies, with the focus being on human societies. A RDBP is a discrete time branching process (BP) in which individuals are supposed to have to work to be able to live and to reproduce. The population decides on a current s ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Branching Random Walk
Branching may refer to: * Branching (linguistics), the general tendency towards a given order of words within sentences and smaller grammatical units within sentences * Branching (polymer chemistry), the attachment of side chains to a polymer's backbone chain * Branching (revision control), a way of duplicating an object under revision control See also * Branching process, a kind of stochastic process * Branch (other) {{disambig ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Random Tree
In common usage, randomness is the apparent or actual lack of definite pattern or predictability in information. A random sequence of events, symbols or steps often has no :wikt:order, order and does not follow an intelligible pattern or combination. Individual random events are, by definition, unpredictable, but if there is a known probability distribution, the frequency of different outcomes over repeated events (or "trials") is predictable.Strictly speaking, the frequency of an outcome will converge almost surely to a predictable value as the number of trials becomes arbitrarily large. Non-convergence or convergence to a different value is possible, but has probability zero. Consistent non-convergence is thus evidence of the lack of a fixed probability distribution, as in many evolutionary processes. For example, when throwing two dice, the outcome of any particular roll is unpredictable, but a sum of 7 will tend to occur twice as often as 4. In this view, randomness is no ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Superprocess
An (\xi,d,\beta)-superprocess, X(t,dx), within mathematics probability theory is a stochastic process on \mathbb \times \mathbb^d that is usually constructed as a special limit of near-critical branching diffusions. Informally, it can be seen as a branching process where each particle splits and dies at infinite rates, and evolves according to a diffusion equation, and we follow the rescaled population of particles, seen as a measure on \mathbb. Scaling limit of a discrete branching process Simplest setting For any integer N\geq 1, consider a branching Brownian process Y^N(t,dx) defined as follows: * Start at t=0 with N independent particles distributed according to a probability distribution \mu. * Each particle independently move according to a Brownian motion. * Each particle independently dies with rate N. * When a particle dies, with probability 1/2 it gives birth to two offspring in the same location. The notation Y^N(t,dx) means should be interpreted as: at each t ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Ordinary Differential Equation
In mathematics, an ordinary differential equation (ODE) is a differential equation (DE) dependent on only a single independent variable (mathematics), variable. As with any other DE, its unknown(s) consists of one (or more) Function (mathematics), function(s) and involves the derivatives of those functions. The term "ordinary" is used in contrast with partial differential equation, ''partial'' differential equations (PDEs) which may be with respect to one independent variable, and, less commonly, in contrast with stochastic differential equations, ''stochastic'' differential equations (SDEs) where the progression is random. Differential equations A linear differential equation is a differential equation that is defined by a linear polynomial in the unknown function and its derivatives, that is an equation of the form :a_0(x)y +a_1(x)y' + a_2(x)y'' +\cdots +a_n(x)y^+b(x)=0, where a_0(x),\ldots,a_n(x) and b(x) are arbitrary differentiable functions that do not need to be linea ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
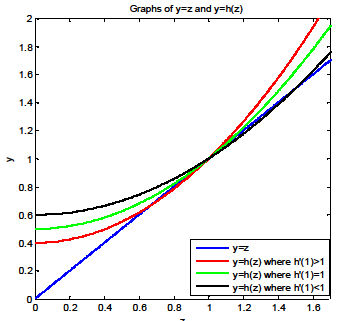
Probability Generating Function
In probability theory, the probability generating function of a discrete random variable is a power series representation (the generating function) of the probability mass function of the random variable. Probability generating functions are often employed for their succinct description of the sequence of probabilities Pr(''X'' = ''i'') in the probability mass function for a random variable ''X'', and to make available the well-developed theory of power series with non-negative coefficients. Definition Univariate case If ''X'' is a discrete random variable taking values ''x'' in the non-negative integers , then the ''probability generating function'' of ''X'' is defined as G(z) = \operatorname (z^X) = \sum_^ p(x) z^x, where p is the probability mass function of X. Note that the subscripted notations G_X and p_X are often used to emphasize that these pertain to a particular random variable X, and to its distribution. The power series converges absolutely at least for all ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Extinction Probability
In population genetics, extinction probability is the chance of an inherited trait becoming extinct as a function of time Time is the continuous progression of existence that occurs in an apparently irreversible process, irreversible succession from the past, through the present, and into the future. It is a component quantity of various measurements used to sequ ... ''t''. If ''t'' = ∞ this may be the complement of the chance of becoming a universal trait. This opposing process is also known as proceeding to fixation. References Statistical genetics Stochastic processes Population models {{Probability-stub ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |