|
Bragg-Hawthorne Equation
In fluid dynamics, Hicks equation, sometimes also referred as Bragg–Hawthorne equation or Squire–Long equation, is a partial differential equation that describes the distribution of stream function for axisymmetric inviscid fluid, named after William Mitchinson Hicks, who derived it first in 1898. The equation was also re-derived by Stephen Bragg and William Hawthorne in 1950 and by Robert R. Long in 1953 and by Herbert Squire in 1956. The Hicks equation without swirl was first introduced by George Gabriel Stokes in 1842. The Grad–Shafranov equation appearing in plasma physics also takes the same form as the Hicks equation. Representing (r,\theta,z) as coordinates in the sense of cylindrical coordinate system with corresponding flow velocity components denoted by (v_r,v_\theta,v_z), the stream function \psi that defines the meridional motion can be defined as :rv_r = - \frac, \quad rv_z = \frac that satisfies the continuity equation for axisymmetric flows automatically ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Fluid Dynamics
In physics and engineering, fluid dynamics is a subdiscipline of fluid mechanics that describes the flow of fluids— liquids and gases. It has several subdisciplines, including ''aerodynamics'' (the study of air and other gases in motion) and hydrodynamics (the study of liquids in motion). Fluid dynamics has a wide range of applications, including calculating forces and moments on aircraft, determining the mass flow rate of petroleum through pipelines, predicting weather patterns, understanding nebulae in interstellar space and modelling fission weapon detonation. Fluid dynamics offers a systematic structure—which underlies these practical disciplines—that embraces empirical and semi-empirical laws derived from flow measurement and used to solve practical problems. The solution to a fluid dynamics problem typically involves the calculation of various properties of the fluid, such as flow velocity, pressure, density, and temperature, as functions of space and time. ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Stream Function
The stream function is defined for incompressible flow, incompressible (divergence-free) fluid flow, flows in two dimensions – as well as in three dimensions with axisymmetry. The flow velocity components can be expressed as the derivatives of the scalar field, scalar stream function. The stream function can be used to plot Streamlines, streaklines, and pathlines, streamlines, which represent the trajectories of particles in a steady flow. The two-dimensional Lagrange stream function was introduced by Joseph Louis Lagrange in 1781. The Stokes stream function is for axisymmetrical three-dimensional flow, and is named after George Gabriel Stokes. Considering the particular case of fluid dynamics, the difference between the stream function values at any two points gives the volumetric flow rate (or volumetric flux) through a line connecting the two points. Since streamlines are tangent to the flow velocity vector of the flow, the value of the stream function must be constant along ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
William Mitchinson Hicks
William Mitchinson Hicks, FRS (23 September 1850, in Launceston, Cornwall – 17 August 1934, in Crowhurst, Sussex) was a British mathematician and physicist. He studied at St John's College, Cambridge, graduating in 1873, and became a Fellow at the College. Hicks spent most of his career at Sheffield, contributing to the development of the university there. He was Principal of Firth College from 1892 to 1897. In 1897, Firth College merged with two other colleges to form the University College of Sheffield, and Hicks was its first Principal until 1905, when the College received its own Royal Charter and became the University of Sheffield. Hicks was the first Vice Chancellor of the University, serving from 1905. From 1883 to 1892, he was Professor of Physics and Mathematics at Sheffield, and was Professor of Physics there from 1892 to 1917. He was elected a Fellow of the Royal Society in 1885. He was awarded the Royal Society's Royal Medal in 1912: ''"On the ground of ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Stephen Bragg
Stephen Lawrence Bragg (17 November 1923 – 14 November 2014) was a British engineer who was Vice Chancellor of Brunel University from 1971 to 1981. He was the son of Lawrence Bragg and grandson of William Henry Bragg. Early life, education and career He was born on 17 November 1923 to Lawrence Bragg, physicist, X-ray crystallographer and Nobel Prize winner for physics (1915) and his wife Alice Grace Jenny née Hopkinson. He studied engineering at the University of Cambridge graduating with an BA in 1945 and an MA in 1949. He went on to study at the Massachusetts Institute of Technology receiving an SM in 1949. He worked for Rolls-Royce between 1951 and 1971, helping develop the Blue Streak missile, and rose to the position of chief scientist, responsible for liaison with universities. Bragg encouraged interactions between academia and industry, and spent five years on the University Grants Committee. In 1971 he left Rolls-Royce, three days before it was declared insolven ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
William Hawthorne
Sir William Rede Hawthorne CBE, FRS, FREng, FIMECHE, FRAES, (22 May 1913 – 16 September 2011) was a British professor of engineering who worked on the development of the jet engine. Bragg-Hawthorne equation is named after him. Life Hawthorne was born in Newcastle-upon-Tyne, England, the son of a civil engineer from Belfast. He had two younger brothers, John and Edward. He was educated at Westminster School, London, then read mathematics and engineering at Trinity College, Cambridge, graduating in 1934 with a double first. He spent two years as a graduate apprentice with Babcock & Wilcox Ltd, then went to the Massachusetts Institute of Technology (MIT) in Cambridge, MA, where his research on laminar and turbulent flames earned him a ScD two years later. In 1939 he married Barbara Runkle (d. 1992, granddaughter of MIT's second President John Daniel Runkle), and they had one son and two daughters. After MIT, he returned to Babcock & Wilcox. In 1940, he joined the Royal ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Herbert Squire
Herbert Brian Squire FRS (13 July 1909 – 22 November 1961), was a British aerospace engineer and Zaharoff Professor of Aviation at Imperial College London. Biography Born on 13 July 1909, Squire was educated at Bedford School and at Balliol College, Oxford, where he read mathematics. After research at the University of Oxford, and at the University of Göttingen between 1932 and 1933, he became a scientific officer at the Royal Aircraft Establishment. In 1946 he was appointed as chairman of the Helicopter Committee of the Aeronautics Research Council and, in 1947, he was appointed as principal scientific officer at the Royal Aircraft Establishment, working on jet propulsion. Between 1952 and 1961 he was Zaharoff Professor of Aviation at Imperial College London. He was elected as a Fellow of the Royal Society The Royal Society, formally The Royal Society of London for Improving Natural Knowledge, is a learned society and the United Kingdom's national academy of scien ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
George Gabriel Stokes
Sir George Gabriel Stokes, 1st Baronet, (; 13 August 1819 – 1 February 1903) was an Irish migration to Great Britain, Irish English physicist and mathematician. Born in County Sligo, Ireland, Stokes spent all of his career at the University of Cambridge, where he was the Lucasian Professor of Mathematics from 1849 until his death in 1903. As a physicist, Stokes made seminal contributions to fluid mechanics, including the Navier–Stokes equations; and to physical optics, with notable works on Polarization (waves), polarization and fluorescence. As a mathematician, he popularised "Stokes' theorem" in vector calculus and contributed to the theory of asymptotic expansions. Stokes, along with Felix Hoppe-Seyler, first demonstrated the oxygen transport function of hemoglobin and showed color changes produced by aeration of hemoglobin solutions. Stokes was made a baronet by the British monarch in 1889. In 1893 he received the Royal Society's Copley Medal, then the most prestigious ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Grad–Shafranov Equation
The Grad–Shafranov equation ( H. Grad and H. Rubin (1958); Vitalii Dmitrievich Shafranov (1966)) is the equilibrium equation in ideal magnetohydrodynamics (MHD) for a two dimensional plasma, for example the axisymmetric toroidal plasma in a tokamak. This equation takes the same form as the Hicks equation from fluid dynamics.Smith, S. G. L., & Hattori, Y. (2012). Axisymmetric magnetic vortices with swirl. Communications in Nonlinear Science and Numerical Simulation, 17(5), 2101-2107. This equation is a two-dimensional, nonlinear, elliptic partial differential equation obtained from the reduction of the ideal MHD equations to two dimensions, often for the case of toroidal axisymmetry (the case relevant in a tokamak). Taking (r,\theta,z) as the cylindrical coordinates, the flux function \psi is governed by the equation, \frac - \frac \frac + \frac = - \mu_0 r^\frac - \frac \frac, where \mu_0 is the magnetic permeability, p(\psi) is the pressure, F(\psi)=rB_ and the magnetic field and ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Plasma Physics
Plasma ()πλάσμα , Henry George Liddell, Robert Scott, ''A Greek English Lexicon'', on Perseus is one of the . It contains a significant portion of charged particles – s and/or s. The presence of these charged particles is what primarily sets plasma apart from the other fundamental states of matter. It is the most abundant form of |
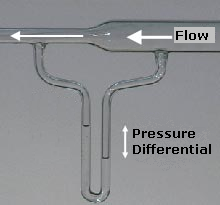
Bernoulli's Principle
In fluid dynamics, Bernoulli's principle states that an increase in the speed of a fluid occurs simultaneously with a decrease in static pressure or a decrease in the fluid's potential energy. The principle is named after the Swiss mathematician and physicist Daniel Bernoulli, who published it in his book ''Hydrodynamica'' in 1738. Although Bernoulli deduced that pressure decreases when the flow speed increases, it was Leonhard Euler in 1752 who derived Bernoulli's equation in its usual form. The principle is only applicable for isentropic flows: when the effects of irreversible processes (like turbulence) and non-adiabatic processes (e.g. thermal radiation) are small and can be neglected. Bernoulli's principle can be applied to various types of fluid flow, resulting in various forms of Bernoulli's equation. The simple form of Bernoulli's equation is valid for incompressible flows (e.g. most liquid flows and gases moving at low Mach number). More advanced forms may be applied ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Circulation (fluid Dynamics)
In physics, circulation is the line integral of a vector field around a closed curve. In fluid dynamics, the field is the fluid velocity field. In electrodynamics, it can be the electric or the magnetic field. Circulation was first used independently by Frederick Lanchester, Martin Kutta and Nikolay Zhukovsky. It is usually denoted Γ (Greek uppercase gamma). Definition and properties If V is a vector field and dl is a vector representing the differential length of a small element of a defined curve, the contribution of that differential length to circulation is dΓ: :\mathrm\Gamma=\mathbf\cdot \mathrm\mathbf=, \mathbf, , \mathrm\mathbf, \cos \theta. Here, ''θ'' is the angle between the vectors V and dl. The circulation Γ of a vector field V around a closed curve ''C'' is the line integral: :\Gamma=\oint_\mathbf\cdot \mathrm d \mathbf. In a conservative vector field this integral evaluates to zero for every closed curve. That means that a line integral between any two ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Prandtl–Batchelor Theorem
In fluid dynamics, Prandtl–Batchelor theorem states that ''if in a two-dimensional laminar flow at high Reynolds number closed streamlines occur, then the vorticity in the closed streamline region must be a constant''. A similar statement holds true for axisymmetric flows. The theorem is named after Ludwig Prandtl and George Batchelor. Prandtl in his celebrated 1904 paper stated this theorem in arguments, George Batchelor unaware of this work proved the theorem in 1956. The problem was also studied in the same year by Richard Feynman and Paco Lagerstrom and by W.W. Wood in 1957. Mathematical proof At high Reynolds numbers, Euler equations reduce to solving a problem for stream function The stream function is defined for incompressible flow, incompressible (divergence-free) fluid flow, flows in two dimensions – as well as in three dimensions with axisymmetry. The flow velocity components can be expressed as the derivatives of t ..., :\nabla^2\psi = - \omega(\psi), \quad \psi=\p ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |