|
Brachistochrone Bernoulli Direct Method
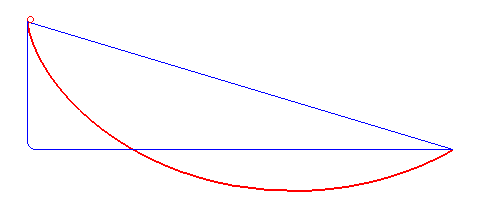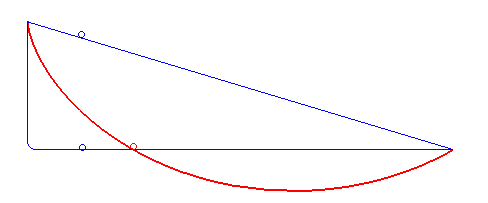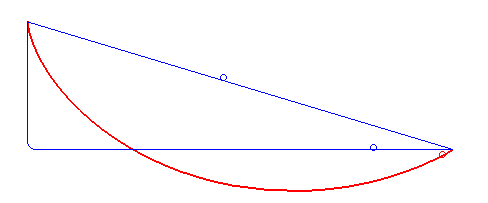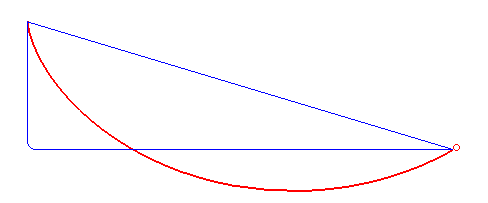
In physics and mathematics, a brachistochrone curve (), or curve of fastest descent, is the one lying on the plane between a point ''A'' and a lower point ''B'', where ''B'' is not directly below ''A'', on which a bead slides frictionlessly under the influence of a uniform gravitational field to a given end point in the shortest time. The problem was posed by Johann Bernoulli in 1696. The brachistochrone curve is the same shape as the tautochrone curve; both are cycloids. However, the portion of the cycloid used for each of the two varies. More specifically, the brachistochrone can use up to a complete rotation of the cycloid (at the limit when A and B are at the same level), but always starts at a cusp. In contrast, the tautochrone problem can use only up to the first half rotation, and always ends at the horizontal.Stewart, James. "Section 10.1 - Curves Defined by Parametric Equations." ''Calculus: Early Transcendentals''. 7th ed. Belmont, CA: Thomson Brooks/Cole, 2012. 640. Pr ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Brachistochrone
In physics and mathematics, a brachistochrone curve (), or curve of fastest descent, is the one lying on the plane between a point ''A'' and a lower point ''B'', where ''B'' is not directly below ''A'', on which a bead slides frictionlessly under the influence of a uniform gravitational field to a given end point in the shortest time. The problem was posed by Johann Bernoulli in 1696. The brachistochrone curve is the same shape as the tautochrone curve; both are cycloids. However, the portion of the cycloid used for each of the two varies. More specifically, the brachistochrone can use up to a complete rotation of the cycloid (at the limit when A and B are at the same level), but always starts at a Cusp (singularity), cusp. In contrast, the tautochrone problem can use only up to the first half rotation, and always ends at the horizontal.Stewart, James. "Section 10.1 - Curves Defined by Parametric Equations." ''Calculus: Early Transcendentals''. 7th ed. Belmont, CA: Thomson Brooks/ ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Gottfried Leibniz
Gottfried Wilhelm (von) Leibniz . ( – 14 November 1716) was a German polymath active as a mathematician, philosopher, scientist and diplomat. He is one of the most prominent figures in both the history of philosophy and the history of mathematics. He wrote works on philosophy, theology, ethics, politics, law, history and philology. Leibniz also made major contributions to physics and technology, and anticipated notions that surfaced much later in probability theory, biology, medicine, geology, psychology, linguistics and computer science. In addition, he contributed to the field of library science: while serving as overseer of the Wolfenbüttel library in Germany, he devised a cataloging system that would have served as a guide for many of Europe's largest libraries. Leibniz's contributions to this vast array of subjects were scattered in various learned journals, in tens of thousands of letters and in unpublished manuscripts. He wrote in several languages, primarily in Latin, ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Tangent
In geometry, the tangent line (or simply tangent) to a plane curve at a given point is the straight line that "just touches" the curve at that point. Leibniz defined it as the line through a pair of infinitely close points on the curve. More precisely, a straight line is said to be a tangent of a curve at a point if the line passes through the point on the curve and has slope , where ''f'' is the derivative of ''f''. A similar definition applies to space curves and curves in ''n''-dimensional Euclidean space. As it passes through the point where the tangent line and the curve meet, called the point of tangency, the tangent line is "going in the same direction" as the curve, and is thus the best straight-line approximation to the curve at that point. The tangent line to a point on a differentiable curve can also be thought of as a '' tangent line approximation'', the graph of the affine function that best approximates the original function at the given point. Similarly, t ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Snell's Law
Snell's law (also known as Snell–Descartes law and ibn-Sahl law and the law of refraction) is a formula used to describe the relationship between the angles of incidence and refraction, when referring to light or other waves passing through a boundary between two different isotropic media, such as water, glass, or air. This law was named after the Dutch astronomer and mathematician Willebrord Snellius (also called Snell). In optics, the law is used in ray tracing to compute the angles of incidence or refraction, and in experimental optics to find the refractive index of a material. The law is also satisfied in meta-materials, which allow light to be bent "backward" at a negative angle of refraction with a negative refractive index. Snell's law states that, for a given pair of media, the ratio of the sines of angle of incidence (\theta_1 ) and angle of refraction (\theta_2 ) is equal to the refractive index of the second medium w.r.t the first (n21) which is equal to the ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Conservation Of Energy
In physics and chemistry, the law of conservation of energy states that the total energy of an isolated system remains constant; it is said to be ''conserved'' over time. This law, first proposed and tested by Émilie du Châtelet, means that energy can neither be created nor destroyed; rather, it can only be transformed or transferred from one form to another. For instance, chemical energy is converted to kinetic energy when a stick of dynamite explodes. If one adds up all forms of energy that were released in the explosion, such as the kinetic energy and potential energy of the pieces, as well as heat and sound, one will get the exact decrease of chemical energy in the combustion of the dynamite. Classically, conservation of energy was distinct from conservation of mass. However, special relativity shows that mass is related to energy and vice versa by ''E = mc2'', and science now takes the view that mass-energy as a whole is conserved. Theoretically, this implies that ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Fermat’s Principle
Fermat's principle, also known as the principle of least time, is the link between geometrical optics, ray optics and physical optics, wave optics. In its original "strong" form, Fermat's principle states that the path taken by Line (geometry)#Ray, a ray between two given points is the path that can be traveled in the least time. In order to be true in all cases, this statement must be weakened by replacing the "least" time with a time that is "stationary point, stationary" with respect to variations of the path — so that a deviation in the path causes, at most, a ''second-order'' change in the traversal time. To put it loosely, a ray path is surrounded by close paths that can be traversed in ''very'' close times. It #A ray as a signal path (line of sight), can be shown that this technical definition corresponds to more intuitive notions of a ray, such as a Line-of-sight propagation, line of sight or the path of a narrow beam. First proposed by the French mathema ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Brachistochrone Bernoulli Direct Method
In physics and mathematics, a brachistochrone curve (), or curve of fastest descent, is the one lying on the plane between a point ''A'' and a lower point ''B'', where ''B'' is not directly below ''A'', on which a bead slides frictionlessly under the influence of a uniform gravitational field to a given end point in the shortest time. The problem was posed by Johann Bernoulli in 1696. The brachistochrone curve is the same shape as the tautochrone curve; both are cycloids. However, the portion of the cycloid used for each of the two varies. More specifically, the brachistochrone can use up to a complete rotation of the cycloid (at the limit when A and B are at the same level), but always starts at a cusp. In contrast, the tautochrone problem can use only up to the first half rotation, and always ends at the horizontal.Stewart, James. "Section 10.1 - Curves Defined by Parametric Equations." ''Calculus: Early Transcendentals''. 7th ed. Belmont, CA: Thomson Brooks/Cole, 2012. 640. Pr ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Constantin Carathéodory
Constantin Carathéodory ( el, Κωνσταντίνος Καραθεοδωρή, Konstantinos Karatheodori; 13 September 1873 – 2 February 1950) was a Greek mathematician who spent most of his professional career in Germany. He made significant contributions to real and complex analysis, the calculus of variations, and measure theory. He also created an axiomatic formulation of thermodynamics. Carathéodory is considered one of the greatest mathematicians of his era and the most renowned Greek mathematician since antiquity. Origins Constantin Carathéodory was born in 1873 in Berlin to Greek parents and grew up in Brussels. His father Stephanos, a lawyer, served as the Ottoman ambassador to Belgium, St. Petersburg and Berlin. His mother, Despina, née Petrokokkinos, was from the island of Chios. The Carathéodory family, originally from Bosnochori or Vyssa, was well established and respected in Constantinople, and its members held many important governmental positions. Th ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Galileo's Shortest Time Curve Conjecture
Galileo di Vincenzo Bonaiuti de' Galilei (15 February 1564 – 8 January 1642) was an Italian astronomer, physicist and engineer, sometimes described as a polymath. Commonly referred to as Galileo, his name was pronounced (, ). He was born in the city of Pisa, then part of the Duchy of Florence. Galileo has been called the "father" of observational astronomy, modern physics, the scientific method, and modern science. Galileo studied speed and velocity, gravity and free fall, the principle of relativity, inertia, projectile motion and also worked in applied science and technology, describing the properties of pendulums and "hydrostatic balances". He invented the thermoscope and various military compasses, and used the telescope for scientific observations of celestial objects. His contributions to observational astronomy include telescopic confirmation of the phases of Venus, observation of the four largest satellites of Jupiter, observation of Saturn's rings, and a ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Two New Sciences
The ''Discourses and Mathematical Demonstrations Relating to Two New Sciences'' ( it, Discorsi e dimostrazioni matematiche intorno a due nuove scienze ) published in 1638 was Galileo Galilei's final book and a scientific testament covering much of his work in physics over the preceding thirty years. It was written partly in Italian and partly in Latin. After his ''Dialogue Concerning the Two Chief World Systems'', the Roman Inquisition had banned the publication of any of Galileo's works, including any he might write in the future. After the failure of his initial attempts to publish ''Two New Sciences'' in France, Germany, and Poland, it was published by Lodewijk Elzevir who was working in Leiden, South Holland, where the writ of the Inquisition was of less consequence (see House of Elzevir). Fra Fulgenzio Micanzio, the official theologian of the Republic of Venice, had initially offered to help Galileo publish in Venice the new work, but he pointed out that publishing the ''T ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Infinitesimal Calculus
Calculus, originally called infinitesimal calculus or "the calculus of infinitesimals", is the mathematical study of continuous change, in the same way that geometry is the study of shape, and algebra is the study of generalizations of arithmetic operations. It has two major branches, differential calculus and integral calculus; the former concerns instantaneous rates of change, and the slopes of curves, while the latter concerns accumulation of quantities, and areas under or between curves. These two branches are related to each other by the fundamental theorem of calculus, and they make use of the fundamental notions of convergence of infinite sequences and infinite series to a well-defined limit. Infinitesimal calculus was developed independently in the late 17th century by Isaac Newton and Gottfried Wilhelm Leibniz. Later work, including codifying the idea of limits, put these developments on a more solid conceptual footing. Today, calculus has widespread uses in scien ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Joseph-Louis Lagrange
Joseph-Louis Lagrange (born Giuseppe Luigi LagrangiaJoseph-Louis Lagrange, comte de l’Empire ''Encyclopædia Britannica'' or Giuseppe Ludovico De la Grange Tournier; 25 January 1736 – 10 April 1813), also reported as Giuseppe Luigi Lagrange or Lagrangia, was an and , later naturalized [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
