|
Acceptance Cone
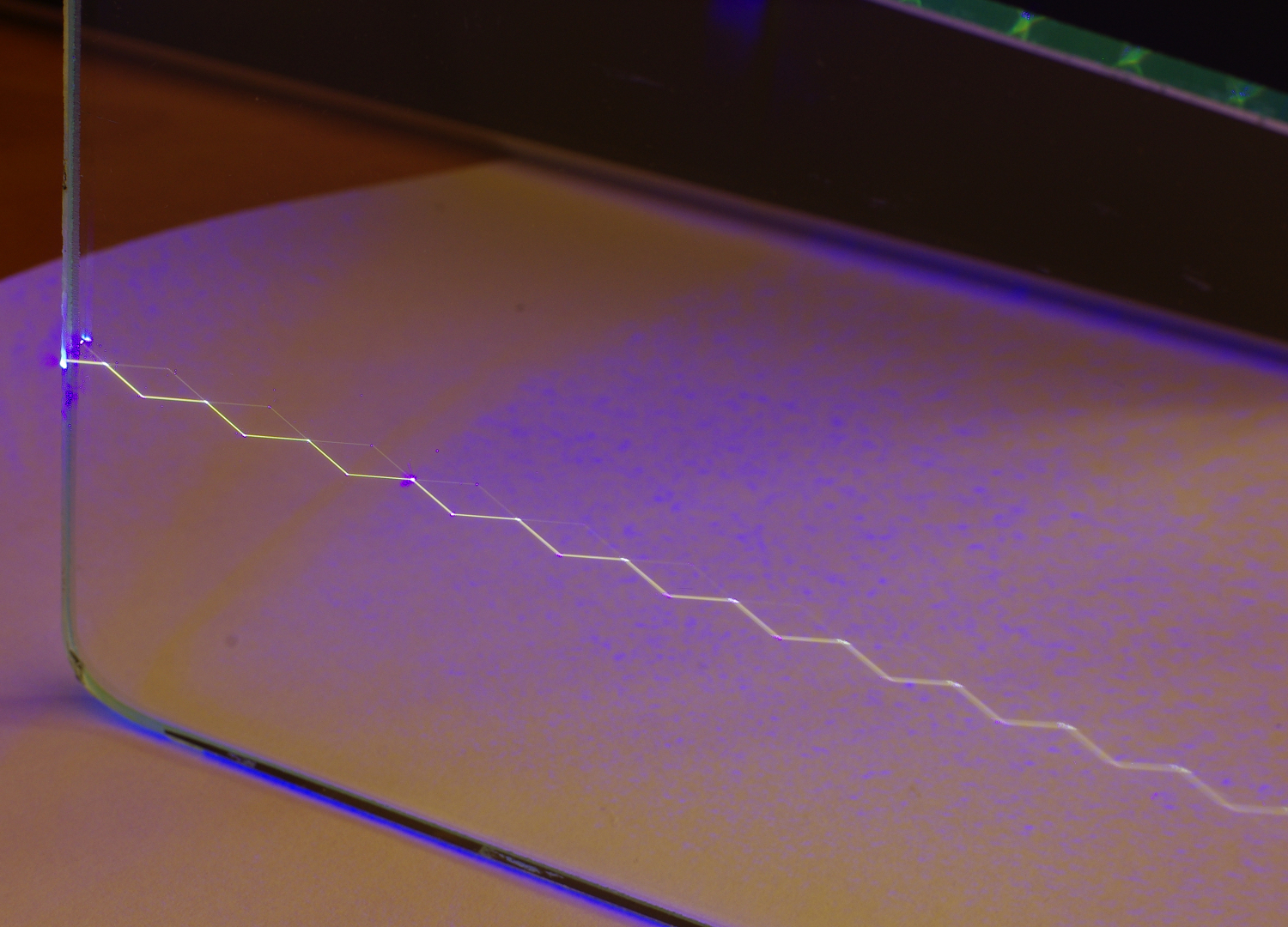
A guided ray (also bound ray or trapped ray) is a ray of light in a multi-mode optical fiber, which is confined by the core. For step index fiber, light entering the fiber will be guided if it falls within the acceptance cone of the fiber, that is if it makes an angle with the fiber axis that is less than the acceptance angle, :\theta < \arcsin \left( \sqrt \right) , where :''θ'' is the angle the ray makes with the fiber axis, ''before'' entering the fiber, :''n''0 is the along the central axis (core) of the fiber, and :''n''c is the refractive index of the cladding. The quantity is the of the fiber. The quantity |
Ray (optics)
In optics a ray is an idealized geometrical model of light, obtained by choosing a curve that is perpendicular to the ''wavefronts'' of the actual light, and that points in the direction of energy flow. Rays are used to model the propagation of light through an optical system, by dividing the real light field up into discrete rays that can be computationally propagated through the system by the techniques of '' ray tracing''. This allows even very complex optical systems to be analyzed mathematically or simulated by computer. Ray tracing uses approximate solutions to Maxwell's equations that are valid as long as the light waves propagate through and around objects whose dimensions are much greater than the light's wavelength. ''Ray optics'' or ''geometrical optics'' does not describe phenomena such as diffraction, which require wave optics theory. Some wave phenomena such as interference can be modeled in limited circumstances by adding phase to the ray model. Definition A l ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Multi-mode Optical Fiber
Multi-mode optical fiber is a type of optical fiber mostly used for communication over short distances, such as within a building or on a campus. Multi-mode links can be used for data rates up to 100 Gbit/s. Multi-mode fiber has a fairly large core diameter that enables multiple light modes to be propagated and limits the maximum length of a transmission link because of modal dispersion. The standard G.651.1 defines the most widely used forms of multi-mode optical fiber. Applications The equipment used for communications over multi-mode optical fiber is less expensive than that for single-mode optical fiber. Typical transmission speed and distance limits are 100 Mbit/s for distances up to 2 km (100BASE-FX), 1 Gbit/s up to 1000 m, and 10 Gbit/s up to 550 m. Because of its high capacity and reliability, multi-mode optical fiber generally is used for backbone applications in buildings. An increasing number of users are taking the benefits of fiber closer ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Fiber Optics
An optical fiber, or optical fibre in Commonwealth English, is a flexible, transparent fiber made by drawing glass (silica) or plastic to a diameter slightly thicker than that of a human hair. Optical fibers are used most often as a means to transmit light between the two ends of the fiber and find wide usage in fiber-optic communications, where they permit transmission over longer distances and at higher bandwidths (data transfer rates) than electrical cables. Fibers are used instead of metal wires because signals travel along them with less loss; in addition, fibers are immune to electromagnetic interference, a problem from which metal wires suffer. Fibers are also used for illumination and imaging, and are often wrapped in bundles so they may be used to carry light into, or images out of confined spaces, as in the case of a fiberscope. Specially designed fibers are also used for a variety of other applications, some of them being fiber optic sensors and fiber lasers. O ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Step-index Profile
For an optical fiber, a step-index profile is a refractive index profile characterized by a uniform refractive index within the core and a sharp decrease in refractive index at the core-cladding interface so that the cladding is of a lower refractive index. The step-index profile corresponds to a power-law index profile with the profile parameter approaching infinity. The step-index profile is used in most single-mode fibers and some multimode fibers. A step-index fiber is characterized by the core and cladding refractive indices ''n1'' and ''n2'' and the core and cladding radii a and b. Examples of standard core and cladding diameters 2a/2b are 8/125, 50/125, 62.5/125, 85/125, or 100/140 (units of µm). The fractional refractive-index change \triangle \, = \frac \ll \ 1. The value of n1 is typically between 1.44 and 1.46, and \triangle is typically between 0.001 and 0.02. Step-index optical fiber is generally made by doping high-purity fused silica glass (SiO2) with differe ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Refractive Index
In optics, the refractive index (or refraction index) of an optical medium is a dimensionless number that gives the indication of the light bending ability of that medium. The refractive index determines how much the path of light is bent, or refracted, when entering a material. This is described by Snell's law of refraction, , where ''θ''1 and ''θ''2 are the angle of incidence and angle of refraction, respectively, of a ray crossing the interface between two media with refractive indices ''n''1 and ''n''2. The refractive indices also determine the amount of light that is reflected when reaching the interface, as well as the critical angle for total internal reflection, their intensity ( Fresnel's equations) and Brewster's angle. The refractive index can be seen as the factor by which the speed and the wavelength of the radiation are reduced with respect to their vacuum values: the speed of light in a medium is , and similarly the wavelength in that medium is , where ''� ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Numerical Aperture
In optics, the numerical aperture (NA) of an optical system is a dimensionless number that characterizes the range of angles over which the system can accept or emit light. By incorporating index of refraction in its definition, NA has the property that it is constant for a beam as it goes from one material to another, provided there is no refractive power at the interface. The exact definition of the term varies slightly between different areas of optics. Numerical aperture is commonly used in microscopy to describe the acceptance cone of an objective (and hence its light-gathering ability and resolution), and in fiber optics, in which it describes the range of angles within which light that is incident on the fiber will be transmitted along it. General optics In most areas of optics, and especially in microscopy, the numerical aperture of an optical system such as an objective lens is defined by :\mathrm = n \sin \theta, where is the index of refraction of the medium i ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Snell's Law
Snell's law (also known as Snell–Descartes law and ibn-Sahl law and the law of refraction) is a formula used to describe the relationship between the angles of incidence and refraction, when referring to light or other waves passing through a boundary between two different isotropic media, such as water, glass, or air. This law was named after the Dutch astronomer and mathematician Willebrord Snellius (also called Snell). In optics, the law is used in ray tracing to compute the angles of incidence or refraction, and in experimental optics to find the refractive index of a material. The law is also satisfied in meta-materials, which allow light to be bent "backward" at a negative angle of refraction with a negative refractive index. Snell's law states that, for a given pair of media, the ratio of the sines of angle of incidence (\theta_1 ) and angle of refraction (\theta_2 ) is equal to the refractive index of the second medium w.r.t the first (n21) which is equal to the ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Critical Angle (optics)
Total internal reflection (TIR) is the optical phenomenon in which waves arriving at the interface (boundary) from one medium to another (e.g., from water to air) are not refracted into the second ("external") medium, but completely reflected back into the first ("internal") medium. It occurs when the second medium has a higher wave speed (i.e., lower refractive index) than the first, and the waves are incident at a sufficiently oblique angle on the interface. For example, the water-to-air surface in a typical fish tank, when viewed obliquely from below, reflects the underwater scene like a mirror with no loss of brightness (Fig.1). TIR occurs not only with electromagnetic waves such as light and microwaves, but also with other types of waves, including sound and water waves. If the waves are capable of forming a narrow beam (Fig.2), the reflection tends to be described in terms of "rays" rather than waves; in a medium whose properties are independent of direction, such as air, ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Total Internal Reflection
Total internal reflection (TIR) is the optical phenomenon in which waves arriving at the interface (boundary) from one medium to another (e.g., from water to air) are not refracted into the second ("external") medium, but completely reflected back into the first ("internal") medium. It occurs when the second medium has a higher wave speed (i.e., lower refractive index) than the first, and the waves are incident at a sufficiently oblique angle on the interface. For example, the water-to-air surface in a typical fish tank, when viewed obliquely from below, reflects the underwater scene like a mirror with no loss of brightness (Fig.1). TIR occurs not only with electromagnetic waves such as light and microwaves, but also with other types of waves, including sound and water waves. If the waves are capable of forming a narrow beam (Fig.2), the reflection tends to be described in terms of "rays" rather than waves; in a medium whose properties are independent of direction, such as air, ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Singlemode Fiber
In fiber-optic communication, a single-mode optical fiber (SMF), also known as fundamental- or mono-mode, is an optical fiber designed to carry only a single mode of light - the transverse mode. Modes are the possible solutions of the Helmholtz equation for waves, which is obtained by combining Maxwell's equations and the boundary conditions. These modes define the way the wave travels through space, i.e. how the wave is distributed in space. Waves can have the same mode but have different frequencies. This is the case in single-mode fibers, where we can have waves with different frequencies, but of the same mode, which means that they are distributed in space in the same way, and that gives us a single ray of light. Although the ray travels parallel to the length of the fiber, it is often called transverse mode since its electromagnetic oscillations occur perpendicular (transverse) to the length of the fiber. The 2009 Nobel Prize in Physics was awarded to Charles K. Kao for his ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Numerical Aperture
In optics, the numerical aperture (NA) of an optical system is a dimensionless number that characterizes the range of angles over which the system can accept or emit light. By incorporating index of refraction in its definition, NA has the property that it is constant for a beam as it goes from one material to another, provided there is no refractive power at the interface. The exact definition of the term varies slightly between different areas of optics. Numerical aperture is commonly used in microscopy to describe the acceptance cone of an objective (and hence its light-gathering ability and resolution), and in fiber optics, in which it describes the range of angles within which light that is incident on the fiber will be transmitted along it. General optics In most areas of optics, and especially in microscopy, the numerical aperture of an optical system such as an objective lens is defined by :\mathrm = n \sin \theta, where is the index of refraction of the medium i ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Acceptance Angle (solar Concentrator)
Acceptance angle is the maximum angle at which incoming sunlight can be captured by a solar concentrator. Its value depends on the concentration of the optic and the refractive index in which the receiver is immersed. Maximizing the acceptance angle of a concentrator is desirable in practical systems and it may be achieved by using nonimaging optics. For concentrators that concentrate light in two dimensions, the acceptance angle may be different in the two directions. Definition The "acceptance angle" figure illustrates this concept. The concentrator is a lens (optics), lens with a receiver ''R''. The left section of the figure shows a set of parallel Ray (optics), rays incident on the concentrator at an angle ''α'' ''θ'' to the optical axis. All rays now miss the receiver and all light is lost. Therefore, for incidence angles ''α'' ''θ'' all light is lost. The concentrator is then said to have a (half) acceptance angle ''θ'', ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |