|
A5 Polytope
In 5-dimensional geometry, there are 19 uniform 5-polytope, uniform polytopes with A5 symmetry. There is one self-dual regular form, the 5-simplex with 6 vertices. Each can be visualized as symmetric orthographic projections in Coxeter planes of the A5 Coxeter group, and other subgroups. Graphs Symmetric orthographic projections of these 19 polytopes can be made in the A5, A4, A3, A2 Coxeter planes. Ak graphs have ''[k+1]'' symmetry. For even k and symmetrically nodea_1ed-diagrams, symmetry doubles to ''[2(k+1)]''. These 19 polytopes are each shown in these 4 symmetry planes, with vertices and edges drawn, and vertices colored by the number of overlapping vertices in each projective position. References * Harold Scott MacDonald Coxeter, H.S.M. Coxeter: ** H.S.M. Coxeter, ''Regular Polytopes'', 3rd Edition, Dover New York, 1973 * Kaleidoscopes: Selected Writings of H.S.M. Coxeter, edited by F. Arthur Sherk, Peter McMullen, Anthony C. Thompson, Asia Ivic Weiss, Wiley-Inter ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Orthographic Projection
Orthographic projection (also orthogonal projection and analemma) is a means of representing Three-dimensional space, three-dimensional objects in Two-dimensional space, two dimensions. Orthographic projection is a form of parallel projection in which all the projection lines are orthogonal to the projection plane, resulting in every plane of the scene appearing in affine transformation on the viewing surface. The obverse of an orthographic projection is an oblique projection, which is a parallel projection in which the projection lines are ''not'' orthogonal to the projection plane. The term ''orthographic'' sometimes means a technique in multiview projection in which principal axes or the planes of the subject are also parallel with the projection plane to create the ''primary views''. If the principal planes or axes of an object in an orthographic projection are ''not'' parallel with the projection plane, the depiction is called ''axonometric'' or an ''auxiliary views''. (''A ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
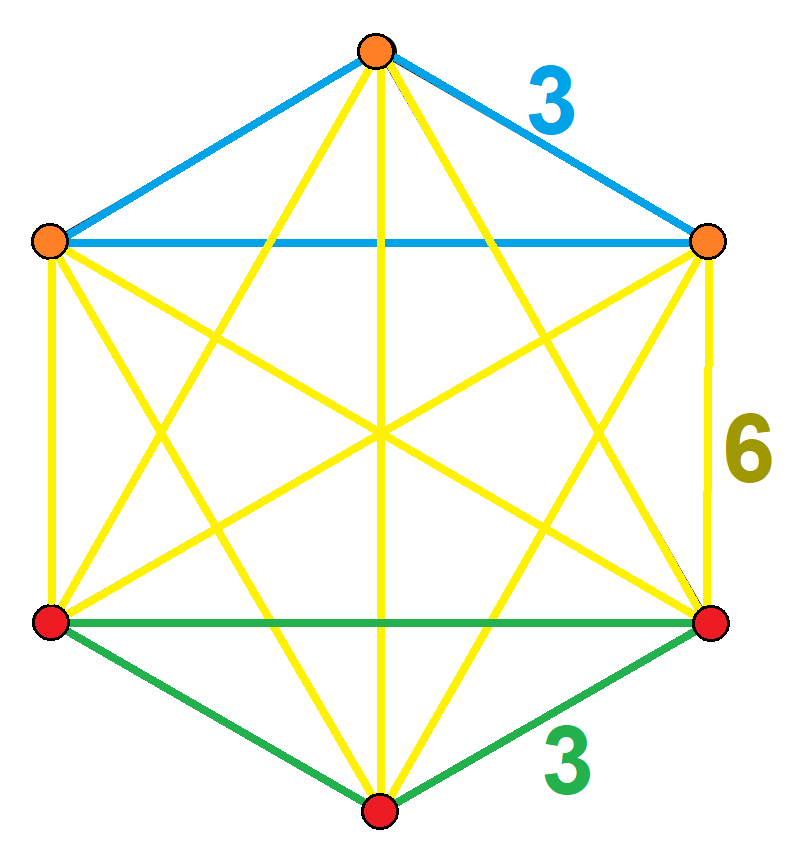
5-simplex T2
In five-dimensional geometry, a 5-simplex is a self-dual regular 5-polytope. It has six vertices, 15 edges, 20 triangle faces, 15 tetrahedral cells, and 6 5-cell facets. It has a dihedral angle of cos−1(), or approximately 78.46°. The 5-simplex is a solution to the problem: ''Make 20 equilateral triangles using 15 matchsticks, where each side of every triangle is exactly one matchstick.'' Alternate names It can also be called a hexateron, or hexa-5-tope, as a 6- facetted polytope in 5-dimensions. The name ''hexateron'' is derived from ''hexa-'' for having six facets and '' teron'' (with ''ter-'' being a corruption of ''tetra-'') for having four-dimensional facets. By Jonathan Bowers, a hexateron is given the acronym hix. As a configuration This configuration matrix represents the 5-simplex. The rows and columns correspond to vertices, edges, faces, cells and 4-faces. The diagonal numbers say how many of each element occur in the whole 5-simplex. The nondiagonal numb ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
5-simplex T12 A2
In five-dimensional geometry, a 5-simplex is a self-dual regular 5-polytope. It has six vertices, 15 edges, 20 triangle faces, 15 tetrahedral cells, and 6 5-cell facets. It has a dihedral angle of cos−1(), or approximately 78.46°. The 5-simplex is a solution to the problem: ''Make 20 equilateral triangles using 15 matchsticks, where each side of every triangle is exactly one matchstick.'' Alternate names It can also be called a hexateron, or hexa-5-tope, as a 6- facetted polytope in 5-dimensions. The name ''hexateron'' is derived from ''hexa-'' for having six facets and '' teron'' (with ''ter-'' being a corruption of ''tetra-'') for having four-dimensional facets. By Jonathan Bowers, a hexateron is given the acronym hix. As a configuration This configuration matrix represents the 5-simplex. The rows and columns correspond to vertices, edges, faces, cells and 4-faces. The diagonal numbers say how many of each element occur in the whole 5-simplex. The nondiagonal numbers ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
5-simplex T12 A3
In five-dimensional geometry, a 5-simplex is a self-dual regular 5-polytope. It has six vertices, 15 edges, 20 triangle faces, 15 tetrahedral cells, and 6 5-cell facets. It has a dihedral angle of cos−1(), or approximately 78.46°. The 5-simplex is a solution to the problem: ''Make 20 equilateral triangles using 15 matchsticks, where each side of every triangle is exactly one matchstick.'' Alternate names It can also be called a hexateron, or hexa-5-tope, as a 6- facetted polytope in 5-dimensions. The name ''hexateron'' is derived from ''hexa-'' for having six facets and '' teron'' (with ''ter-'' being a corruption of ''tetra-'') for having four-dimensional facets. By Jonathan Bowers, a hexateron is given the acronym hix. As a configuration This configuration matrix represents the 5-simplex. The rows and columns correspond to vertices, edges, faces, cells and 4-faces. The diagonal numbers say how many of each element occur in the whole 5-simplex. The nondiagonal numbers ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
5-simplex T12 A4
In five-dimensional geometry, a 5-simplex is a self-dual regular 5-polytope. It has six vertices, 15 edges, 20 triangle faces, 15 tetrahedral cells, and 6 5-cell facets. It has a dihedral angle of cos−1(), or approximately 78.46°. The 5-simplex is a solution to the problem: ''Make 20 equilateral triangles using 15 matchsticks, where each side of every triangle is exactly one matchstick.'' Alternate names It can also be called a hexateron, or hexa-5-tope, as a 6- facetted polytope in 5-dimensions. The name ''hexateron'' is derived from ''hexa-'' for having six facets and '' teron'' (with ''ter-'' being a corruption of ''tetra-'') for having four-dimensional facets. By Jonathan Bowers, a hexateron is given the acronym hix. As a configuration This configuration matrix represents the 5-simplex. The rows and columns correspond to vertices, edges, faces, cells and 4-faces. The diagonal numbers say how many of each element occur in the whole 5-simplex. The nondiagonal numbers ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
5-simplex T12
In five-dimensional geometry, a 5-simplex is a self-dual regular 5-polytope. It has six vertices, 15 edges, 20 triangle faces, 15 tetrahedral cells, and 6 5-cell facets. It has a dihedral angle of cos−1(), or approximately 78.46°. The 5-simplex is a solution to the problem: ''Make 20 equilateral triangles using 15 matchsticks, where each side of every triangle is exactly one matchstick.'' Alternate names It can also be called a hexateron, or hexa-5-tope, as a 6- facetted polytope in 5-dimensions. The name ''hexateron'' is derived from ''hexa-'' for having six facets and '' teron'' (with ''ter-'' being a corruption of ''tetra-'') for having four-dimensional facets. By Jonathan Bowers, a hexateron is given the acronym hix. As a configuration This configuration matrix represents the 5-simplex. The rows and columns correspond to vertices, edges, faces, cells and 4-faces. The diagonal numbers say how many of each element occur in the whole 5-simplex. The nondiagonal numbers ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
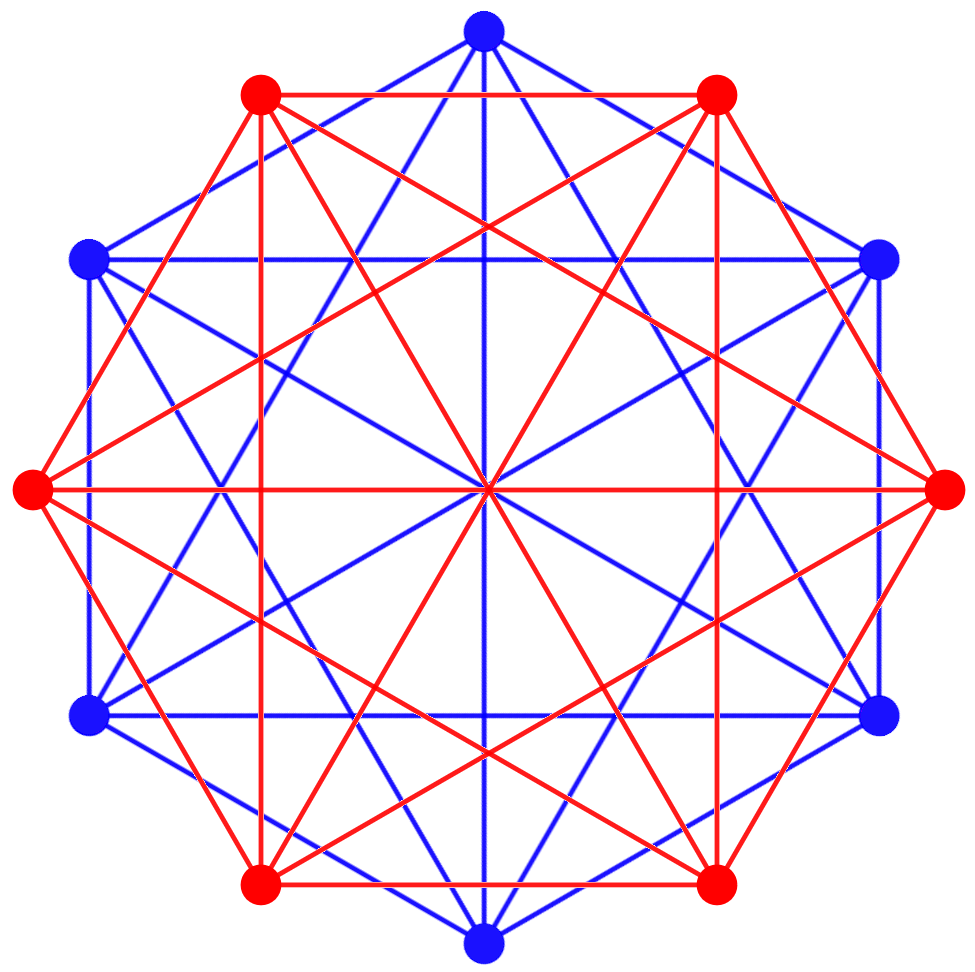
Truncated 5-simplex
In five-dimensional geometry, a truncated 5-simplex is a convex uniform 5-polytope, being a truncation of the regular 5-simplex. There are unique 2 degrees of truncation. Vertices of the truncation 5-simplex are located as pairs on the edge of the 5-simplex. Vertices of the bitruncation 5-simplex are located on the triangular faces of the 5-simplex. Truncated 5-simplex The truncated 5-simplex has 30 vertices, 75 edges, 80 triangular faces, 45 cells (15 tetrahedral, and 30 truncated tetrahedron), and 12 4-faces (6 5-cell and 6 truncated 5-cells). Alternate names * Truncated hexateron (Acronym: tix) (Jonathan Bowers) Coordinates The vertices of the ''truncated 5-simplex'' can be most simply constructed on a hyperplane in 6-space as permutations of (0,0,0,0,1,2) ''or'' of (0,1,2,2,2,2). These coordinates come from facets of the truncated 6-orthoplex and bitruncated 6-cube respectively. Images Bitruncated 5-simplex Alternate names * Bitruncated hexateron (Acronym: b ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
5-simplex T01 A2
In five-dimensional geometry, a 5-simplex is a self-dual regular 5-polytope. It has six vertices, 15 edges, 20 triangle faces, 15 tetrahedral cells, and 6 5-cell facets. It has a dihedral angle of cos−1(), or approximately 78.46°. The 5-simplex is a solution to the problem: ''Make 20 equilateral triangles using 15 matchsticks, where each side of every triangle is exactly one matchstick.'' Alternate names It can also be called a hexateron, or hexa-5-tope, as a 6- facetted polytope in 5-dimensions. The name ''hexateron'' is derived from ''hexa-'' for having six facets and '' teron'' (with ''ter-'' being a corruption of ''tetra-'') for having four-dimensional facets. By Jonathan Bowers, a hexateron is given the acronym hix. As a configuration This configuration matrix represents the 5-simplex. The rows and columns correspond to vertices, edges, faces, cells and 4-faces. The diagonal numbers say how many of each element occur in the whole 5-simplex. The nondiagonal numbers ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
5-simplex T01 A3
In five-dimensional geometry, a 5-simplex is a self-dual regular 5-polytope. It has six vertices, 15 edges, 20 triangle faces, 15 tetrahedral cells, and 6 5-cell facets. It has a dihedral angle of cos−1(), or approximately 78.46°. The 5-simplex is a solution to the problem: ''Make 20 equilateral triangles using 15 matchsticks, where each side of every triangle is exactly one matchstick.'' Alternate names It can also be called a hexateron, or hexa-5-tope, as a 6- facetted polytope in 5-dimensions. The name ''hexateron'' is derived from ''hexa-'' for having six facets and '' teron'' (with ''ter-'' being a corruption of ''tetra-'') for having four-dimensional facets. By Jonathan Bowers, a hexateron is given the acronym hix. As a configuration This configuration matrix represents the 5-simplex. The rows and columns correspond to vertices, edges, faces, cells and 4-faces. The diagonal numbers say how many of each element occur in the whole 5-simplex. The nondiagonal numbers ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
5-simplex T01 A4
In five-dimensional geometry, a 5-simplex is a self-dual regular 5-polytope. It has six vertices, 15 edges, 20 triangle faces, 15 tetrahedral cells, and 6 5-cell facets. It has a dihedral angle of cos−1(), or approximately 78.46°. The 5-simplex is a solution to the problem: ''Make 20 equilateral triangles using 15 matchsticks, where each side of every triangle is exactly one matchstick.'' Alternate names It can also be called a hexateron, or hexa-5-tope, as a 6- facetted polytope in 5-dimensions. The name ''hexateron'' is derived from ''hexa-'' for having six facets and '' teron'' (with ''ter-'' being a corruption of ''tetra-'') for having four-dimensional facets. By Jonathan Bowers, a hexateron is given the acronym hix. As a configuration This configuration matrix represents the 5-simplex. The rows and columns correspond to vertices, edges, faces, cells and 4-faces. The diagonal numbers say how many of each element occur in the whole 5-simplex. The nondiagonal numbers ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
5-simplex T01
In five-dimensional geometry, a 5-simplex is a self-dual regular 5-polytope. It has six vertices, 15 edges, 20 triangle faces, 15 tetrahedral cells, and 6 5-cell facets. It has a dihedral angle of cos−1(), or approximately 78.46°. The 5-simplex is a solution to the problem: ''Make 20 equilateral triangles using 15 matchsticks, where each side of every triangle is exactly one matchstick.'' Alternate names It can also be called a hexateron, or hexa-5-tope, as a 6- facetted polytope in 5-dimensions. The name ''hexateron'' is derived from ''hexa-'' for having six facets and '' teron'' (with ''ter-'' being a corruption of ''tetra-'') for having four-dimensional facets. By Jonathan Bowers, a hexateron is given the acronym hix. As a configuration This configuration matrix represents the 5-simplex. The rows and columns correspond to vertices, edges, faces, cells and 4-faces. The diagonal numbers say how many of each element occur in the whole 5-simplex. The nondiagonal numbers ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Birectified 5-simplex
In five-dimensional geometry, a rectified 5-simplex is a convex uniform 5-polytope, being a Rectification (geometry), rectification of the regular 5-simplex. There are three unique degrees of rectifications, including the zeroth, the 5-simplex itself. Vertices of the ''rectified 5-simplex'' are located at the edge-centers of the ''5-simplex''. Vertices of the ''birectified 5-simplex'' are located in the triangular face centers of the ''5-simplex''. Rectified 5-simplex In Five-dimensional space, five-dimensional geometry, a rectified 5-simplex is a uniform 5-polytope with 15 vertex (geometry), vertices, 60 Edge (geometry), edges, 80 Triangle, triangular Face (geometry), faces, 45 Cell (geometry), cells (30 Tetrahedron, tetrahedral, and 15 Octahedron, octahedral), and 12 4-faces (6 5-cell and 6 rectified 5-cells). It is also called 03,1 for its branching Coxeter-Dynkin diagram, shown as . Emanuel Lodewijk Elte, E. L. Elte identified it in 1912 as a semiregular polytope, labeling i ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
