geometry Geometry (; ) is, with arithmetic, one of the oldest branches of mathematics. It is concerned with properties of space such as the distance, shape, size, and relative position of figures. A mathematician who works in the field of geometry is c ...

, a uniform polytope of dimension three or higher is a

vertex-transitive In geometry, a polytope (e.g. a polygon or polyhedron) or a tiling is isogonal or vertex-transitive if all its vertices are equivalent under the symmetries of the figure. This implies that each vertex is surrounded by the same kinds of fa ...

polytope In elementary geometry, a polytope is a geometric object with flat sides ('' faces''). Polytopes are the generalization of three-dimensional polyhedra to any number of dimensions. Polytopes may exist in any general number of dimensions as an ...

bounded by uniform

facets A facet is a flat surface of a geometric shape, e.g., of a cut gemstone. Facet may also refer to: Arts, entertainment, and media * ''Facets'' (album), an album by Jim Croce * ''Facets'', a 1980 album by jazz pianist Monty Alexander and his tri ...

. The uniform polytopes in two dimensions are the

regular polygon In Euclidean geometry, a regular polygon is a polygon that is direct equiangular (all angles are equal in measure) and equilateral (all sides have the same length). Regular polygons may be either convex, star or skew. In the limit, a sequence ...

s (the definition is different in 2 dimensions to exclude vertex-transitive even-sided polygons that alternate two different lengths of edges). This is a generalization of the older category of ''semiregular'' polytopes, but also includes the

regular polytope In mathematics, a regular polytope is a polytope whose symmetry group acts transitively on its flags, thus giving it the highest degree of symmetry. All its elements or -faces (for all , where is the dimension of the polytope) — cells, ...

s. Further, star regular faces and

vertex figure In geometry, a vertex figure, broadly speaking, is the figure exposed when a corner of a polyhedron or polytope is sliced off. Definitions Take some corner or vertex of a polyhedron. Mark a point somewhere along each connected edge. Draw line ...

s (

star polygon In geometry, a star polygon is a type of non- convex polygon. Regular star polygons have been studied in depth; while star polygons in general appear not to have been formally defined, certain notable ones can arise through truncation operatio ...

s) are allowed, which greatly expand the possible solutions. A strict definition requires uniform polytopes to be finite, while a more expansive definition allows

uniform honeycomb In geometry, a uniform honeycomb or uniform tessellation or infinite uniform polytope, is a vertex-transitive honeycomb made from uniform polytope facets. All of its vertices are identical and there is the same combination and arrangement of fac ...

s (2-dimensional tilings and higher dimensional honeycombs) of Euclidean and

hyperbolic space In mathematics, hyperbolic space of dimension n is the unique simply connected, n-dimensional Riemannian manifold of constant sectional curvature equal to -1. It is homogeneous, and satisfies the stronger property of being a symmetric space. The ...

to be considered polytopes as well.

Operations

Nearly every uniform polytope can be generated by a

Wythoff construction In geometry, a Wythoff construction, named after mathematician Willem Abraham Wythoff, is a method for constructing a uniform polyhedron or plane tiling. It is often referred to as Wythoff's kaleidoscopic construction. Construction process ...

, and represented by a

Coxeter diagram Harold Scott MacDonald "Donald" Coxeter, (9 February 1907 – 31 March 2003) was a British and later also Canadian geometer. He is regarded as one of the greatest geometers of the 20th century. Biography Coxeter was born in Kensington t ...

. Notable exceptions include the

great dirhombicosidodecahedron In geometry, the great dirhombicosidodecahedron (or great snub disicosidisdodecahedron) is a nonconvex uniform polyhedron, indexed last as . It has 124 faces (40 triangles, 60 squares, and 24 pentagrams), 240 edges, and 60 vertices. This is ...

in three dimensions and the

grand antiprism In geometry, the grand antiprism or pentagonal double antiprismoid is a uniform 4-polytope (4-dimensional uniform polytope) bounded by 320 cells: 20 pentagonal antiprisms, and 300 tetrahedra. It is an anomalous, non-Wythoffian uniform 4-polytope ...

in four dimensions. The terminology for the convex uniform polytopes used in

uniform polyhedron In geometry, a uniform polyhedron has regular polygons as Face (geometry), faces and is vertex-transitive (i.e., there is an isometry mapping any vertex onto any other). It follows that all vertices are congruence (geometry), congruent. Unifor ...

uniform 4-polytope In geometry, a uniform 4-polytope (or uniform polychoron) is a 4-dimensional polytope which is vertex-transitive and whose cells are uniform polyhedra, and faces are regular polygons. There are 47 non-prismatic convex uniform 4-polytopes. Th ...

uniform 5-polytope In geometry, a uniform 5-polytope is a five-dimensional uniform polytope. By definition, a uniform 5-polytope is vertex-transitive and constructed from uniform 4-polytope Facet (geometry), facets. The complete set of convex uniform 5-polytopes ...

uniform 6-polytope In six-dimensional geometry, a uniform 6-polytope is a six-dimensional uniform polytope. A uniform polypeton is vertex-transitive, and all facets are uniform 5-polytopes. The complete set of convex uniform 6-polytopes has not been determined, bu ...

uniform tiling In geometry, a uniform tiling is a tessellation of the plane by regular polygon faces with the restriction of being vertex-transitive. Uniform tilings can exist in both the Euclidean plane and Hyperbolic space, hyperbolic plane. Uniform tilings ar ...

, and

convex uniform honeycomb In geometry, a convex uniform honeycomb is a uniform tessellation which fills three-dimensional Euclidean space with non-overlapping convex uniform polyhedral cells. Twenty-eight such honeycombs are known: * the familiar cubic honeycomb and 7 tr ...

articles were coined by Norman Johnson. Equivalently, the Wythoffian polytopes can be generated by applying basic operations to the regular polytopes in that dimension. This approach was first used by

Johannes Kepler Johannes Kepler (; ; 27 December 1571 – 15 November 1630) was a German astronomer, mathematician, astrologer, natural philosopher and writer on music. He is a key figure in the 17th-century Scientific Revolution, best known for his laws ...

, and is the basis of the Conway polyhedron notation.

Rectification operators

Regular n-polytopes have ''n'' orders of

rectification Rectification has the following technical meanings: Mathematics * Rectification (geometry), truncating a polytope by marking the midpoints of all its edges, and cutting off its vertices at those points * Rectifiable curve, in mathematics * Recti ...

. The zeroth rectification is the original form. The (''n''−1)-th rectification is the dual. A rectification reduces edges to vertices, a birectification reduces faces to vertices, a trirectification reduces cells to vertices, a quadirectification reduces 4-faces to vertices, a quintirectification reduced 5-faces to vertices, and so on. An extended

Schläfli symbol In geometry, the Schläfli symbol is a notation of the form \ that defines regular polytopes and tessellations. The Schläfli symbol is named after the 19th-century Swiss mathematician Ludwig Schläfli, who generalized Euclidean geometry to mo ...

can be used for representing rectified forms, with a single subscript: * ''k''-th rectification = t_k = ''k''r.

Truncation operators

Truncation operations that can be applied to regular ''n''-polytopes in any combination. The resulting Coxeter diagram has two ringed nodes, and the operation is named for the distance between them. Truncation cuts vertices, cantellation cuts edges, runcination cuts faces, sterication cut cells. Each higher operation also cuts lower ones too, so a cantellation also truncates vertices. # t_0,1 or t: Truncation - applied to

polygon In geometry, a polygon () is a plane figure that is described by a finite number of straight line segments connected to form a closed '' polygonal chain'' (or ''polygonal circuit''). The bounded plane region, the bounding circuit, or the two ...

s and higher. A truncation removes vertices, and inserts a new facet in place of each former vertex. Faces are truncated, doubling their edges. (The term, coined by

Kepler Johannes Kepler (; ; 27 December 1571 – 15 November 1630) was a German astronomer, mathematician, astrologer, natural philosopher and writer on music. He is a key figure in the 17th-century Scientific Revolution, best known for his laws o ...

, comes from Latin ''truncare'' 'to cut off'.) #: Cube truncation sequence

#* There are higher truncations also:

bitruncation In geometry, a bitruncation is an operation on regular polytopes. It represents a truncation beyond rectification. The original edges are lost completely and the original faces remain as smaller copies of themselves. Bitruncated regular pol ...

t_1,2 or 2t, tritruncation t_2,3 or 3t, quadritruncation t_3,4 or 4t, quintitruncation t_4,5 or 5t, etc. # t_0,2 or rr: Cantellation - applied to

polyhedra In geometry, a polyhedron (plural polyhedra or polyhedrons; ) is a three-dimensional shape with flat polygonal faces, straight edges and sharp corners or vertices. A convex polyhedron is the convex hull of finitely many points, not all on ...

and higher. It can be seen as rectifying its

. A cantellation truncates both vertices and edges and replaces them with new facets. Cells are replaced by topologically expanded copies of themselves. (The term, coined by Johnson, is derived from the verb ''cant'', like ''

bevel A bevelled edge (UK) or beveled edge (US) is an edge of a structure that is not perpendicular to the faces of the piece. The words bevel and chamfer overlap in usage; in general usage they are often interchanged, while in technical usage they ...

'', meaning to cut with a slanted face.) #: Cube cantellation sequence

#* There are higher cantellations also: bicantellation t_1,3 or r2r, tricantellation t_2,4 or r3r, quadricantellation t_3,5 or r4r, etc. #* t_0,1,2 or tr: Cantitruncation - applied to

and higher. It can be seen as truncating its

. A cantitruncation truncates both vertices and edges and replaces them with new facets. Cells are replaced by topologically expanded copies of themselves. (The composite term combines cantellation and truncation) #** There are higher cantellations also: bicantitruncation t_1,2,3 or t2r, tricantitruncation t_2,3,4 or t3r, quadricantitruncation t_3,4,5 or t4r, etc. # t_0,3: Runcination - applied to

Uniform 4-polytope In geometry, a uniform 4-polytope (or uniform polychoron) is a 4-dimensional polytope which is vertex-transitive and whose cells are uniform polyhedra, and faces are regular polygons. There are 47 non-prismatic convex uniform 4-polytopes. Th ...

and higher. Runcination truncates vertices, edges, and faces, replacing them each with new facets. 4-faces are replaced by topologically expanded copies of themselves. (The term, coined by Johnson, is derived from Latin ''runcina'' 'carpenter's plane'.) #* There are higher runcinations also: biruncination t_1,4, triruncination t_2,5, etc. # t_0,4 or 2r2r: Sterication - applied to

Uniform 5-polytope In geometry, a uniform 5-polytope is a five-dimensional uniform polytope. By definition, a uniform 5-polytope is vertex-transitive and constructed from uniform 4-polytope Facet (geometry), facets. The complete set of convex uniform 5-polytopes ...

s and higher. It can be seen as birectifying its birectification. Sterication truncates vertices, edges, faces, and cells, replacing each with new facets. 5-faces are replaced by topologically expanded copies of themselves. (The term, coined by Johnson, is derived from Greek ''stereos'' 'solid'.) #* There are higher sterications also: bisterication t_1,5 or 2r3r, tristerication t_2,6 or 2r4r, etc. #* t_0,2,4 or 2t2r: Stericantellation - applied to

s and higher. It can be seen as bitruncating its birectification. #** There are higher sterications also: bistericantellation t_1,3,5 or 2t3r, tristericantellation t_2,4,6 or 2t4r, etc. # t_0,5: Pentellation - applied to

Uniform 6-polytope In six-dimensional geometry, a uniform 6-polytope is a six-dimensional uniform polytope. A uniform polypeton is vertex-transitive, and all facets are uniform 5-polytopes. The complete set of convex uniform 6-polytopes has not been determined, bu ...

s and higher. Pentellation truncates vertices, edges, faces, cells, and 4-faces, replacing each with new facets. 6-faces are replaced by topologically expanded copies of themselves. (Pentellation is derived from Greek '' pente'' 'five'.) #* There are also higher pentellations: bipentellation t_1,6, tripentellation t_2,7, etc. # t_0,6 or 3r3r: Hexication - applied to

Uniform 7-polytope In seven-dimensional geometry, a 7-polytope is a polytope contained by 6-polytope facets. Each 5-polytope ridge being shared by exactly two 6-polytope facets. A uniform 7-polytope is one whose symmetry group is transitive on vertices and whose ...

s and higher. It can be seen as trirectifying its trirectification. Hexication truncates vertices, edges, faces, cells, 4-faces, and 5-faces, replacing each with new facets. 7-faces are replaced by topologically expanded copies of themselves. (Hexication is derived from Greek '' hex'' 'six'.) #* There are higher hexications also: bihexication: t_1,7 or 3r4r, trihexication: t_2,8 or 3r5r, etc. #* t_0,3,6 or 3t3r: Hexiruncinated - applied to

s and higher. It can be seen as tritruncating its trirectification. #** There are also higher hexiruncinations: bihexiruncinated: t_1,4,7 or 3t4r, trihexiruncinated: t_2,5,8 or 3t5r, etc. # t_0,7: Heptellation - applied to Uniform 8-polytopes and higher. Heptellation truncates vertices, edges, faces, cells, 4-faces, 5-faces, and 6-faces, replacing each with new facets. 8-faces are replaced by topologically expanded copies of themselves. (Heptellation is derived from Greek '' hepta'' 'seven'.) #* There are higher heptellations also: biheptellation t_1,8, triheptellation t_2,9, etc. # t_0,8 or 4r4r: Octellation - applied to

Uniform 9-polytope In nine-dimensional geometry, a nine-dimensional polytope or 9-polytope is a polytope contained by 8-polytope facets. Each 7-polytope ridge being shared by exactly two 8-polytope facets. A uniform 9-polytope is one which is vertex-transitive, a ...

s and higher. # t_0,9: Ennecation - applied to

Uniform 10-polytope In ten-dimensional geometry, a 10-polytope is a 10-dimensional polytope whose boundary consists of 9-polytope facets, exactly two such facets meeting at each 8-polytope ridge. A uniform 10-polytope is one which is vertex-transitive, and construc ...

s and higher. In addition combinations of truncations can be performed which also generate new uniform polytopes. For example, a ''runcitruncation'' is a ''runcination'' and ''truncation'' applied together. If all truncations are applied at once, the operation can be more generally called an

omnitruncation In geometry, an omnitruncation is an operation applied to a regular polytope (or honeycomb) in a Wythoff construction that creates a maximum number of facets. It is represented in a Coxeter–Dynkin diagram with all nodes ringed. It is a ''shor ...

Alternation

One special operation, called alternation, removes alternate vertices from a polytope with only even-sided faces. An alternated omnitruncated polytope is called a ''snub''. The resulting polytopes always can be constructed, and are not generally reflective, and also do not in general have ''uniform'' polytope solutions. The set of polytopes formed by alternating the

hypercube In geometry, a hypercube is an ''n''-dimensional analogue of a square () and a cube (). It is a closed, compact, convex figure whose 1-skeleton consists of groups of opposite parallel line segments aligned in each of the space's dimensions, p ...

s are known as demicubes. In three dimensions, this produces a

tetrahedron In geometry, a tetrahedron (plural: tetrahedra or tetrahedrons), also known as a triangular pyramid, is a polyhedron composed of four triangular faces, six straight edges, and four vertex corners. The tetrahedron is the simplest of all ...

; in four dimensions, this produces a

16-cell In geometry, the 16-cell is the regular convex 4-polytope (four-dimensional analogue of a Platonic solid) with Schläfli symbol . It is one of the six regular convex 4-polytopes first described by the Swiss mathematician Ludwig Schläfli in the ...

, or ''demitesseract''.

Vertex figure

Uniform polytopes can be constructed from their

, the arrangement of edges, faces, cells, etc. around each vertex. Uniform polytopes represented by a

, marking active mirrors by rings, have reflectional symmetry, and can be simply constructed by recursive reflections of the vertex figure. A smaller number of nonreflectional uniform polytopes have a single vertex figure but are not repeated by simple reflections. Most of these can be represented with operations like alternation of other uniform polytopes. Vertex figures for single-ringed Coxeter diagrams can be constructed from the diagram by removing the ringed node, and ringing neighboring nodes. Such vertex figures are themselves vertex-transitive. Multiringed polytopes can be constructed by a slightly more complicated construction process, and their topology is not a uniform polytope. For example, the vertex figure of a truncated regular polytope (with 2 rings) is a pyramid. An omnitruncated polytope (all nodes ringed) will always have an irregular

simplex In geometry, a simplex (plural: simplexes or simplices) is a generalization of the notion of a triangle or tetrahedron to arbitrary dimensions. The simplex is so-named because it represents the simplest possible polytope in any given dimension. ...

as its vertex figure.

Circumradius

Uniform polytopes have equal edge-lengths, and all vertices are an equal distance from the center, called the circumradius. Uniform polytopes whose circumradius is equal to the edge length can be used as

s for

s. For example, the regular

hexagon In geometry, a hexagon (from Greek , , meaning "six", and , , meaning "corner, angle") is a six-sided polygon. The total of the internal angles of any simple (non-self-intersecting) hexagon is 720°. Regular hexagon A '' regular hexagon'' has ...

divides into 6 equilateral triangles and is the vertex figure for the regular

triangular tiling In geometry, the triangular tiling or triangular tessellation is one of the three regular tilings of the Euclidean plane, and is the only such tiling where the constituent shapes are not parallelogons. Because the internal angle of the equilater ...

. Also the

cuboctahedron A cuboctahedron is a polyhedron with 8 triangular faces and 6 square faces. A cuboctahedron has 12 identical vertices, with 2 triangles and 2 squares meeting at each, and 24 identical edges, each separating a triangle from a square. As such, it ...

divides into 8 regular tetrahedra and 6 square pyramids (half

octahedron In geometry, an octahedron (plural: octahedra, octahedrons) is a polyhedron with eight faces. The term is most commonly used to refer to the regular octahedron, a Platonic solid composed of eight equilateral triangles, four of which meet at ea ...

), and it is the vertex figure for the

alternated cubic honeycomb The tetrahedral-octahedral honeycomb, alternated cubic honeycomb is a quasiregular space-filling tessellation (or honeycomb) in Euclidean 3-space. It is composed of alternating regular octahedra and tetrahedra in a ratio of 1:2. Other names i ...

Uniform polytopes by dimension

It is useful to classify the uniform polytopes by dimension. This is equivalent to the number of nodes on the Coxeter diagram, or the number of hyperplanes in the Wythoffian construction. Because (''n''+1)-dimensional polytopes are tilings of ''n''-dimensional spherical space, tilings of ''n''-dimensional Euclidean and

are also considered to be (''n''+1)-dimensional. Hence, the tilings of two-dimensional space are grouped with the three-dimensional solids.

One dimension

The only one-dimensional polytope is the line segment. It corresponds to the Coxeter family A₁.

Two dimensions

In two dimensions, there is an infinite family of convex uniform polytopes, the

s, the simplest being the equilateral

triangle A triangle is a polygon with three edges and three vertices. It is one of the basic shapes in geometry. A triangle with vertices ''A'', ''B'', and ''C'' is denoted \triangle ABC. In Euclidean geometry, any three points, when non- colline ...

. Truncated regular polygons become bicolored geometrically quasiregular polygons of twice as many sides, t=. The first few regular polygons (and quasiregular forms) are displayed below: There is also an infinite set of

s (one for each

rational number In mathematics, a rational number is a number that can be expressed as the quotient or fraction of two integers, a numerator and a non-zero denominator . For example, is a rational number, as is every integer (e.g. ). The set of all ra ...

greater than 2), but these are non-convex. The simplest example is the

pentagram A pentagram (sometimes known as a pentalpha, pentangle, or star pentagon) is a regular five-pointed star polygon, formed from the diagonal line segments of a convex (or simple, or non-self-intersecting) regular pentagon. Drawing a circle arou ...

, which corresponds to the rational number 5/2. Regular star polygons, , can be truncated into semiregular star polygons, t=t, but become double-coverings if ''q'' is even. A truncation can also be made with a reverse orientation polygon t=, for example t=. Regular polygons, represented by

for a p-gon. Regular polygons are self-dual, so the rectification produces the same polygon. The uniform truncation operation doubles the sides to . The snub operation, alternating the truncation, restores the original polygon . Thus all uniform polygons are also regular. The following operations can be performed on regular polygons to derive the uniform polygons, which are also regular polygons:

Three dimensions

In three dimensions, the situation gets more interesting. There are five convex regular polyhedra, known as the

Platonic solid In geometry, a Platonic solid is a convex, regular polyhedron in three-dimensional Euclidean space. Being a regular polyhedron means that the faces are congruent (identical in shape and size) regular polygons (all angles congruent and all e ...

s: In addition to these, there are also 13 semiregular polyhedra, or

Archimedean solid In geometry, an Archimedean solid is one of the 13 solids first enumerated by Archimedes. They are the convex uniform polyhedra composed of regular polygons meeting in identical vertices, excluding the five Platonic solids (which are compose ...

s, which can be obtained via

s, or by performing operations such as

truncation In mathematics and computer science, truncation is limiting the number of digits right of the decimal point. Truncation and floor function Truncation of positive real numbers can be done using the floor function. Given a number x \in \mathb ...

on the Platonic solids, as demonstrated in the following table: There is also the infinite set of

prisms Prism usually refers to: * Prism (optics), a transparent optical component with flat surfaces that refract light * Prism (geometry), a kind of polyhedron Prism may also refer to: Science and mathematics * Prism (geology), a type of sedimentar ...

, one for each regular polygon, and a corresponding set of

antiprism In geometry, an antiprism or is a polyhedron composed of two parallel direct copies (not mirror images) of an polygon, connected by an alternating band of triangles. They are represented by the Conway notation . Antiprisms are a subclass o ...

s. The uniform star polyhedra include a further 4 regular star polyhedra, the Kepler-Poinsot polyhedra, and 53 semiregular star polyhedra. There are also two infinite sets, the star prisms (one for each star polygon) and star antiprisms (one for each rational number greater than 3/2).

Constructions

The Wythoffian uniform polyhedra and tilings can be defined by their Wythoff symbol, which specifies the

fundamental region Given a topological space and a group acting on it, the images of a single point under the group action form an orbit of the action. A fundamental domain or fundamental region is a subset of the space which contains exactly one point from each o ...

of the object. An extension of Schläfli notation, also used by

Coxeter Harold Scott MacDonald "Donald" Coxeter, (9 February 1907 – 31 March 2003) was a British and later also Canadian geometer. He is regarded as one of the greatest geometers of the 20th century. Biography Coxeter was born in Kensington to ...

, applies to all dimensions; it consists of the letter 't', followed by a series of subscripted numbers corresponding to the ringed nodes of the

, and followed by the Schläfli symbol of the regular seed polytope. For example, the

truncated octahedron In geometry, the truncated octahedron is the Archimedean solid that arises from a regular octahedron by removing six pyramids, one at each of the octahedron's vertices. The truncated octahedron has 14 faces (8 regular hexagons and 6 squares), 36 ...

is represented by the notation: t_0,1.

Four dimensions

In four dimensions, there are 6

convex regular 4-polytope In mathematics, a regular 4-polytope is a regular four-dimensional polytope. They are the four-dimensional analogues of the regular polyhedra in three dimensions and the regular polygons in two dimensions. There are six convex and ten star reg ...

s, 17 prisms on the Platonic and Archimedean solids (excluding the cube-prism, which has already been counted as the

tesseract In geometry, a tesseract is the four-dimensional analogue of the cube; the tesseract is to the cube as the cube is to the square. Just as the surface of the cube consists of six square faces, the hypersurface of the tesseract consists of e ...

), and two infinite sets: the prisms on the convex antiprisms, and the

duoprism In geometry of 4 dimensions or higher, a double prism or duoprism is a polytope resulting from the Cartesian product of two polytopes, each of two dimensions or higher. The Cartesian product of an -polytope and an -polytope is an -polytope, wher ...

s. There are also 41 convex semiregular 4-polytope, including the

non-Wythoffian In geometry, a Wythoff construction, named after mathematician Willem Abraham Wythoff, is a method for constructing a uniform polyhedron or plane tiling. It is often referred to as Wythoff's kaleidoscopic construction. Construction process ...

and the

snub 24-cell In geometry, the snub 24-cell or snub disicositetrachoron is a convex uniform 4-polytope composed of 120 regular tetrahedral and 24 icosahedral cells. Five tetrahedra and three icosahedra meet at each vertex. In total it has 480 triangular face ...

. Both of these special 4-polytope are composed of subgroups of the vertices of the

600-cell In geometry, the 600-cell is the convex regular 4-polytope (four-dimensional analogue of a Platonic solid) with Schläfli symbol . It is also known as the C600, hexacosichoron and hexacosihedroid. It is also called a tetraplex (abbreviated from ...

. The four-dimensional uniform star polytopes have not all been enumerated. The ones that have include the 10 regular star (Schläfli-Hess) 4-polytopes and 57 prisms on the uniform star polyhedra, as well as three infinite families: the prisms on the star antiprisms, the duoprisms formed by multiplying two star polygons, and the duoprisms formed by multiplying an ordinary polygon with a star polygon. There is an unknown number of 4-polytope that do not fit into the above categories; over one thousand have been discovered so far. 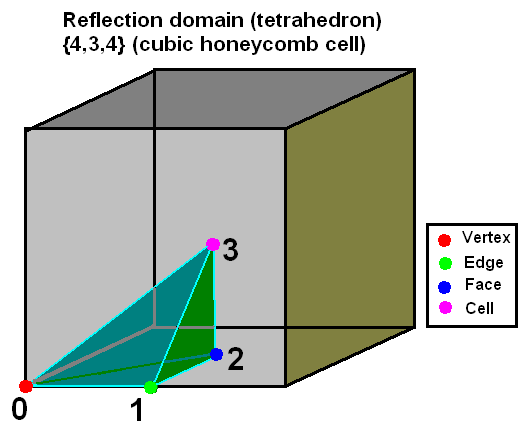Polychoron tetrahedral domain

Every regular polytope can be seen as the images of a

in a small number of mirrors. In a 4-dimensional polytope (or 3-dimensional cubic honeycomb) the fundamental region is bounded by four mirrors. A mirror in 4-space is a three-dimensional

hyperplane In geometry, a hyperplane is a subspace whose dimension is one less than that of its '' ambient space''. For example, if a space is 3-dimensional then its hyperplanes are the 2-dimensional planes, while if the space is 2-dimensional, its hyper ...

, but it is more convenient for our purposes to consider only its two-dimensional intersection with the three-dimensional surface of the

hypersphere In mathematics, an -sphere or a hypersphere is a topological space that is homeomorphic to a ''standard'' -''sphere'', which is the set of points in -dimensional Euclidean space that are situated at a constant distance from a fixed point, call ...

; thus the mirrors form an irregular

. Each of the sixteen

regular 4-polytopes In mathematics, a regular 4-polytope is a regular four-dimensional polytope. They are the four-dimensional analogues of the regular polyhedra in three dimensions and the regular polygons in two dimensions. There are six convex and ten star re ...

is generated by one of four symmetry groups, as follows: * group ,3,3 the

5-cell In geometry, the 5-cell is the convex 4-polytope with Schläfli symbol . It is a 5-vertex four-dimensional object bounded by five tetrahedral cells. It is also known as a C5, pentachoron, pentatope, pentahedroid, or tetrahedral pyramid. It ...

, which is self-dual; * group ,3,4

and its dual

; * group ,4,3 the

24-cell In geometry, the 24-cell is the convex regular 4-polytope (four-dimensional analogue of a Platonic solid) with Schläfli symbol . It is also called C24, or the icositetrachoron, octaplex (short for "octahedral complex"), icosatetrahedroid, o ...

, self-dual; * group ,3,5

, its dual

120-cell In geometry, the 120-cell is the convex regular 4-polytope (four-dimensional analogue of a Platonic solid) with Schläfli symbol . It is also called a C120, dodecaplex (short for "dodecahedral complex"), hyperdodecahedron, polydodecahedron, he ...

, and their ten regular stellations. * group ^1,1,1 contains only repeated members of the ,3,4family. (The groups are named in

Coxeter notation In geometry, Coxeter notation (also Coxeter symbol) is a system of classifying symmetry groups, describing the angles between fundamental reflections of a Coxeter group in a bracketed notation expressing the structure of a Coxeter-Dynkin diagra ...

.) Eight of the

s in Euclidean 3-space are analogously generated from the

cubic honeycomb The cubic honeycomb or cubic cellulation is the only proper regular space-filling tessellation (or honeycomb) in Euclidean 3-space made up of cubic cells. It has 4 cubes around every edge, and 8 cubes around each vertex. Its vertex figure is a r ...

, by applying the same operations used to generate the Wythoffian uniform 4-polytopes. For a given symmetry simplex, a generating point may be placed on any of the four vertices, 6 edges, 4 faces, or the interior volume. On each of these 15 elements there is a point whose images, reflected in the four mirrors, are the vertices of a uniform 4-polytope. The extended Schläfli symbols are made by a t followed by inclusion of one to four subscripts 0,1,2,3. If there is one subscript, the generating point is on a corner of the fundamental region, i.e. a point where three mirrors meet. These corners are notated as * 0: vertex of the parent 4-polytope (center of the dual's cell) * 1: center of the parent's edge (center of the dual's face) * 2: center of the parent's face (center of the dual's edge) * 3: center of the parent's cell (vertex of the dual) (For the two self-dual 4-polytopes, "dual" means a similar 4-polytope in dual position.) Two or more subscripts mean that the generating point is between the corners indicated.

Constructive summary

The 15 constructive forms by family are summarized below. The self-dual families are listed in one column, and others as two columns with shared entries on the symmetric

s. The final 10th row lists the snub 24-cell constructions. This includes all nonprismatic uniform 4-polytopes, except for the

, which has no Coxeter family.

Truncated forms

The following table defines all 15 forms. Each trunction form can have from one to four cell types, located in positions 0,1,2,3 as defined above. The cells are labeled by polyhedral truncation notation. * An n-gonal prism is represented as : ×. * The green background is shown on forms that are equivalent to either the parent or the dual. * The red background shows the truncations of the parent, and blue the truncations of the dual.

Half forms

Half constructions exist with ''holes'' rather than ringed nodes. Branches neighboring ''holes'' and inactive nodes must be even-order. Half construction have the vertices of an identically ringed construction.

Five and higher dimensions

In five and higher dimensions, there are 3 regular polytopes, the

and

cross-polytope In geometry, a cross-polytope, hyperoctahedron, orthoplex, or cocube is a regular, convex polytope that exists in ''n''- dimensional Euclidean space. A 2-dimensional cross-polytope is a square, a 3-dimensional cross-polytope is a regular octahe ...

. They are generalisations of the three-dimensional cube, tetrahedron and octahedron, respectively. There are no regular star polytopes in these dimensions. Most uniform higher-dimensional polytopes are obtained by modifying the regular polytopes, or by taking the Cartesian product of polytopes of lower dimensions. In six, seven and eight dimensions, the exceptional

simple Lie group In mathematics, a simple Lie group is a connected non-abelian Lie group ''G'' which does not have nontrivial connected normal subgroups. The list of simple Lie groups can be used to read off the list of simple Lie algebras and Riemannian symm ...

s, E₆, E₇ and E₈ come into play. By placing rings on a nonzero number of nodes of the

s, one can obtain 39 new 6-polytopes, 127 new 7-polytopes and 255 new 8-polytopes. A notable example is the 4₂₁ polytope.

Uniform honeycombs

Related to the subject of finite uniform polytopes are uniform honeycombs in Euclidean and hyperbolic spaces. Euclidean uniform honeycombs are generated by affine Coxeter groups and hyperbolic honeycombs are generated by the hyperbolic Coxeter groups. Two affine Coxeter groups can be multiplied together. There are two classes of hyperbolic Coxeter groups, compact and paracompact. Uniform honeycombs generated by compact groups have finite facets and vertex figures, and exist in 2 through 4 dimensions. Paracompact groups have affine or hyperbolic subgraphs, and infinite facets or vertex figures, and exist in 2 through 10 dimensions.

References

''The Beauty of Geometry: Twelve Essays'', Dover Publications, 1999, (Chapter 3: Wythoff's Construction for Uniform Polytopes) * Norman Johnson ''Uniform Polytopes'', Manuscript (1991) ** N.W. Johnson: ''The Theory of Uniform Polytopes and Honeycombs'', Ph.D. Dissertation, University of Toronto, 1966 * A. Boole Stott: ''Geometrical deduction of semiregular from regular polytopes and space fillings'', Verhandelingen of the Koninklijke academy van Wetenschappen width unit Amsterdam, Eerste Sectie 11,1, Amsterdam, 1910 * H.S.M. Coxeter: ** H.S.M. Coxeter, M.S. Longuet-Higgins and J.C.P. Miller: ''Uniform Polyhedra'', Philosophical Transactions of the Royal Society of London, Londne, 1954 ** H.S.M. Coxeter, ''Regular Polytopes'', 3rd Edition, Dover New York, 1973 *
Kaleidoscopes: Selected Writings of H.S.M. Coxeter
', edited by F. Arthur Sherk, Peter McMullen, Anthony C. Thompson, Asia Ivic Weiss, Wiley-Interscience Publication, 1995, ** (Paper 22) H.S.M. Coxeter
Regular and Semi Regular Polytopes I
ath. Zeit. 46 (1940) 380-407, MR 2,10** (Paper 23) H.S.M. Coxeter
Regular and Semi-Regular Polytopes II
ath. Zeit. 188 (1985) 559-591** (Paper 24) H.S.M. Coxeter
Regular and Semi-Regular Polytopes III
ath. Zeit. 200 (1988) 3-45*

, Longuet-Higgins, Miller, ''Uniform polyhedra'', Phil. Trans. 1954, 246 A, 401-50. (Extended Schläfli notation used) * Marco Möller, ''Vierdimensionale Archimedische Polytope'', Dissertation, Universität Hamburg, Hamburg (2004)

External links

*
uniform, convex polytopes in four dimensions:
Marco Möller {{Polytopes Polytopes