Probability four-current on:
[Wikipedia]
[Google]
[Amazon]
Probability is a branch of

 The sixteenth-century
The sixteenth-century
 For example, if two coins are flipped, then the chance of both being heads is
For example, if two coins are flipped, then the chance of both being heads is
Virtual Laboratories in Probability and Statistics (Univ. of Ala.-Huntsville)
*
Probability and Statistics EBook
*
HTML index with links to PostScript files
an
PDF
(first three chapters)
* ttp://www.economics.soton.ac.uk/staff/aldrich/Probability%20Earliest%20Uses.htm Probability and Statistics on the Earliest Uses Pages (Univ. of Southampton)
Earliest Uses of Symbols in Probability and Statistics
o
A tutorial on probability and Bayes' theorem devised for first-year Oxford University students
, by Charles Grinstead, Laurie Snel
Source
''(
Probabilità e induzione
', Bologna, CLUEB, 1993. (digital version)
{{Authority control
mathematics
Mathematics is a field of study that discovers and organizes methods, Mathematical theory, theories and theorems that are developed and Mathematical proof, proved for the needs of empirical sciences and mathematics itself. There are many ar ...
and statistics
Statistics (from German language, German: ', "description of a State (polity), state, a country") is the discipline that concerns the collection, organization, analysis, interpretation, and presentation of data. In applying statistics to a s ...
concerning events
Event may refer to:
Gatherings of people
* Ceremony, an event of ritual significance, performed on a special occasion
* Convention (meeting), a gathering of individuals engaged in some common interest
* Event management, the organization of eve ...
and numerical descriptions of how likely they are to occur. The probability of an event is a number between 0 and 1; the larger the probability, the more likely an event is to occur."Kendall's Advanced Theory of Statistics, Volume 1: Distribution Theory", Alan Stuart and Keith Ord, 6th ed., (2009), .William Feller, ''An Introduction to Probability Theory and Its Applications'', vol. 1, 3rd ed., (1968), Wiley, . This number is often expressed as a percentage (%), ranging from 0% to 100%. A simple example is the tossing of a fair (unbiased) coin. Since the coin is fair, the two outcomes ("heads" and "tails") are both equally probable; the probability of "heads" equals the probability of "tails"; and since no other outcomes are possible, the probability of either "heads" or "tails" is 1/2 (which could also be written as 0.5 or 50%).
These concepts have been given an axiomatic
An axiom, postulate, or assumption is a statement that is taken to be true, to serve as a premise or starting point for further reasoning and arguments. The word comes from the Ancient Greek word (), meaning 'that which is thought worthy or fi ...
mathematical formalization in ''probability theory
Probability theory or probability calculus is the branch of mathematics concerned with probability. Although there are several different probability interpretations, probability theory treats the concept in a rigorous mathematical manner by expre ...
'', which is used widely in areas of study such as statistics
Statistics (from German language, German: ', "description of a State (polity), state, a country") is the discipline that concerns the collection, organization, analysis, interpretation, and presentation of data. In applying statistics to a s ...
, mathematics
Mathematics is a field of study that discovers and organizes methods, Mathematical theory, theories and theorems that are developed and Mathematical proof, proved for the needs of empirical sciences and mathematics itself. There are many ar ...
, science
Science is a systematic discipline that builds and organises knowledge in the form of testable hypotheses and predictions about the universe. Modern science is typically divided into twoor threemajor branches: the natural sciences, which stu ...
, finance
Finance refers to monetary resources and to the study and Academic discipline, discipline of money, currency, assets and Liability (financial accounting), liabilities. As a subject of study, is a field of Business administration, Business Admin ...
, gambling
Gambling (also known as betting or gaming) is the wagering of something of Value (economics), value ("the stakes") on a Event (probability theory), random event with the intent of winning something else of value, where instances of strategy (ga ...
, artificial intelligence
Artificial intelligence (AI) is the capability of computer, computational systems to perform tasks typically associated with human intelligence, such as learning, reasoning, problem-solving, perception, and decision-making. It is a field of re ...
, machine learning
Machine learning (ML) is a field of study in artificial intelligence concerned with the development and study of Computational statistics, statistical algorithms that can learn from data and generalise to unseen data, and thus perform Task ( ...
, computer science
Computer science is the study of computation, information, and automation. Computer science spans Theoretical computer science, theoretical disciplines (such as algorithms, theory of computation, and information theory) to Applied science, ...
, game theory
Game theory is the study of mathematical models of strategic interactions. It has applications in many fields of social science, and is used extensively in economics, logic, systems science and computer science. Initially, game theory addressed ...
, and philosophy
Philosophy ('love of wisdom' in Ancient Greek) is a systematic study of general and fundamental questions concerning topics like existence, reason, knowledge, Value (ethics and social sciences), value, mind, and language. It is a rational an ...
to, for example, draw inferences about the expected frequency of events. Probability theory is also used to describe the underlying mechanics and regularities of complex systems
A complex system is a system composed of many components that may interact with one another. Examples of complex systems are Earth's global climate, organisms, the human brain, infrastructure such as power grid, transportation or communication s ...
.
Etymology
The word ''probability'' derives from the Latin , which can also mean " probity", a measure of theauthority
Authority is commonly understood as the legitimate power of a person or group of other people.
In a civil state, ''authority'' may be practiced by legislative, executive, and judicial branches of government,''The New Fontana Dictionary of M ...
of a witness
In law, a witness is someone who, either voluntarily or under compulsion, provides testimonial evidence, either oral or written, of what they know or claim to know.
A witness might be compelled to provide testimony in court, before a grand jur ...
in a legal case
Legal proceeding is an activity that seeks to invoke the power of a tribunal in order to enforce a law. Although the term may be defined more broadly or more narrowly as circumstances require, it has been noted that " e term ''legal proceedings'' ...
in Europe, and often correlated with the witness's nobility
Nobility is a social class found in many societies that have an aristocracy. It is normally appointed by and ranked immediately below royalty. Nobility has often been an estate of the realm with many exclusive functions and characteristics. T ...
. In a sense, this differs much from the modern meaning of ''probability'', which in contrast is a measure of the weight of empirical evidence
Empirical evidence is evidence obtained through sense experience or experimental procedure. It is of central importance to the sciences and plays a role in various other fields, like epistemology and law.
There is no general agreement on how the ...
, and is arrived at from inductive reasoning
Inductive reasoning refers to a variety of method of reasoning, methods of reasoning in which the conclusion of an argument is supported not with deductive certainty, but with some degree of probability. Unlike Deductive reasoning, ''deductive'' ...
and statistical inference
Statistical inference is the process of using data analysis to infer properties of an underlying probability distribution.Upton, G., Cook, I. (2008) ''Oxford Dictionary of Statistics'', OUP. . Inferential statistical analysis infers properties of ...
. Hacking, I. (2006) ''The Emergence of Probability: A Philosophical Study of Early Ideas about Probability, Induction and Statistical Inference'', Cambridge University Press,
Interpretations
When dealing with random experiments – i.e.,experiment
An experiment is a procedure carried out to support or refute a hypothesis, or determine the efficacy or likelihood of something previously untried. Experiments provide insight into cause-and-effect by demonstrating what outcome occurs whe ...
s that are random
In common usage, randomness is the apparent or actual lack of definite pattern or predictability in information. A random sequence of events, symbols or steps often has no order and does not follow an intelligible pattern or combination. ...
and well-defined
In mathematics, a well-defined expression or unambiguous expression is an expression (mathematics), expression whose definition assigns it a unique interpretation or value. Otherwise, the expression is said to be ''not well defined'', ill defined ...
– in a purely theoretical setting (like tossing a coin), probabilities can be numerically described by the number of desired outcomes, divided by the total number of all outcomes. This is referred to as theoretical probability (in contrast to empirical probability
In probability theory and statistics, the empirical probability, relative frequency, or experimental probability of an event is the ratio of the number of outcomes in which a specified event occurs to the total number of trials, i.e. by means no ...
, dealing with probabilities in the context of real experiments). The probability is a number between 0 and 1; the larger the probability, the more likely the desired outcome is to occur. For example, tossing a coin twice will yield "head-head", "head-tail", "tail-head", and "tail-tail" outcomes. The probability of getting an outcome of "head-head" is 1 out of 4 outcomes, or, in numerical terms, 1/4, 0.25 or 25%. The probability of getting an outcome of at least one head is 3 out of 4, or 0.75, and this event is more likely to occur. However, when it comes to practical application, there are two major competing categories of probability interpretations, whose adherents hold different views about the fundamental nature of probability:
* Objectivists
Objectivism is a philosophical system named and developed by Russian-American writer and philosopher Ayn Rand. She described it as "the concept of man as a heroic being, with his own happiness as the moral purpose of his life, with productive a ...
assign numbers to describe some objective or physical state of affairs. The most popular version of objective probability is frequentist probability
Frequentist probability or frequentism is an interpretation of probability; it defines an event's probability (the ''long-run probability'') as the limit of its relative frequency in infinitely many trials.
Probabilities can be found (in pr ...
, which claims that the probability of a random event denotes the '' relative frequency of occurrence'' of an experiment's outcome when the experiment is repeated indefinitely. This interpretation considers probability to be the relative frequency "in the long run" of outcomes. A modification of this is propensity probability
The propensity theory of probability is a probability interpretation in which the probability is thought of as a physical propensity, disposition, or tendency of a given type of situation to yield an outcome of a certain kind, or to yield a lon ...
, which interprets probability as the tendency of some experiment to yield a certain outcome, even if it is performed only once.
* Subjectivists assign numbers per subjective probability, that is, as a degree of belief. The degree of belief has been interpreted as "the price at which you would buy or sell a bet that pays 1 unit of utility if E, 0 if not E", although that interpretation is not universally agreed upon. The most popular version of subjective probability is Bayesian probability
Bayesian probability ( or ) is an interpretation of the concept of probability, in which, instead of frequency or propensity of some phenomenon, probability is interpreted as reasonable expectation representing a state of knowledge or as quant ...
, which includes expert knowledge as well as experimental data to produce probabilities. The expert knowledge is represented by some (subjective) prior probability distribution
A prior probability distribution of an uncertain quantity, simply called the prior, is its assumed probability distribution before some evidence is taken into account. For example, the prior could be the probability distribution representing the ...
. These data are incorporated in a likelihood function
A likelihood function (often simply called the likelihood) measures how well a statistical model explains observed data by calculating the probability of seeing that data under different parameter values of the model. It is constructed from the ...
. The product of the prior and the likelihood, when normalized, results in a posterior probability distribution
The posterior probability is a type of conditional probability that results from updating the prior probability with information summarized by the likelihood via an application of Bayes' rule. From an epistemological perspective, the posterior ...
that incorporates all the information known to date. By Aumann's agreement theorem
Aumann's agreement theorem states that two Bayesian agents with the same prior beliefs cannot "agree to disagree" about the probability of an event if their individual beliefs are common knowledge. In other words, if it is commonly known what eac ...
, Bayesian agents whose prior beliefs are similar will end up with similar posterior beliefs. However, sufficiently different priors can lead to different conclusions, regardless of how much information the agents share.
History
The scientific study of probability is a modern development of mathematics.Gambling
Gambling (also known as betting or gaming) is the wagering of something of Value (economics), value ("the stakes") on a Event (probability theory), random event with the intent of winning something else of value, where instances of strategy (ga ...
shows that there has been an interest in quantifying the ideas of probability throughout history, but exact mathematical descriptions arose much later. There are reasons for the slow development of the mathematics of probability. Whereas games of chance provided the impetus for the mathematical study of probability, fundamental issues are still obscured by superstitions.
According to Richard Jeffrey
Richard Carl Jeffrey (August 5, 1926 – November 9, 2002) was an American philosopher, logician, and probability theorist. He is best known for developing and championing the philosophy of radical probabilism and the associated heuristic of ...
, "Before the middle of the seventeenth century, the term 'probable' (Latin ''probabilis'') meant ''approvable'', and was applied in that sense, univocally, to opinion and to action. A probable action or opinion was one such as sensible people would undertake or hold, in the circumstances."Jeffrey, R.C., ''Probability and the Art of Judgment,'' Cambridge University Press. (1992). pp. 54–55 . However, in legal contexts especially, 'probable' could also apply to propositions for which there was good evidence.Franklin, J. (2001) ''The Science of Conjecture: Evidence and Probability Before Pascal,'' Johns Hopkins University Press. (pp. 22, 113, 127)

 The sixteenth-century
The sixteenth-century Italian
Italian(s) may refer to:
* Anything of, from, or related to the people of Italy over the centuries
** Italians, a Romance ethnic group related to or simply a citizen of the Italian Republic or Italian Kingdom
** Italian language, a Romance languag ...
polymath Gerolamo Cardano
Gerolamo Cardano (; also Girolamo or Geronimo; ; ; 24 September 1501– 21 September 1576) was an Italian polymath whose interests and proficiencies ranged through those of mathematician, physician, biologist, physicist, chemist, astrologer, as ...
demonstrated the efficacy of defining odds
In probability theory, odds provide a measure of the probability of a particular outcome. Odds are commonly used in gambling and statistics. For example for an event that is 40% probable, one could say that the odds are or
When gambling, o ...
as the ratio of favourable to unfavourable outcomes (which implies that the probability of an event is given by the ratio of favourable outcomes to the total number of possible outcomes).
Aside from the elementary work by Cardano, the doctrine of probabilities dates to the correspondence of Pierre de Fermat
Pierre de Fermat (; ; 17 August 1601 – 12 January 1665) was a French mathematician who is given credit for early developments that led to infinitesimal calculus, including his technique of adequality. In particular, he is recognized for his d ...
and Blaise Pascal
Blaise Pascal (19June 162319August 1662) was a French mathematician, physicist, inventor, philosopher, and Catholic Church, Catholic writer.
Pascal was a child prodigy who was educated by his father, a tax collector in Rouen. His earliest ...
(1654). Christiaan Huygens
Christiaan Huygens, Halen, Lord of Zeelhem, ( , ; ; also spelled Huyghens; ; 14 April 1629 – 8 July 1695) was a Dutch mathematician, physicist, engineer, astronomer, and inventor who is regarded as a key figure in the Scientific Revolution ...
(1657) gave the earliest known scientific treatment of the subject. Jakob Bernoulli
Jacob Bernoulli (also known as James in English or Jacques in French; – 16 August 1705) was a Swiss mathematician. He sided with Gottfried Wilhelm Leibniz during the Leibniz–Newton calculus controversy and was an early proponent of Leibniz ...
's ''Ars Conjectandi
(Latin for "The Art of Conjecturing") is a book on combinatorics and mathematical probability written by Jacob Bernoulli and published in 1713, eight years after his death, by his nephew, Nicolaus I Bernoulli. The seminal work consolidated, ap ...
'' (posthumous, 1713) and Abraham de Moivre
Abraham de Moivre FRS (; 26 May 166727 November 1754) was a French mathematician known for de Moivre's formula, a formula that links complex numbers and trigonometry, and for his work on the normal distribution and probability theory.
He move ...
's '' Doctrine of Chances'' (1718) treated the subject as a branch of mathematics. See Ian Hacking
Ian MacDougall Hacking (February 18, 1936 – May 10, 2023) was a Canadian philosopher specializing in the philosophy of science. Throughout his career, he won numerous awards, such as the Killam Prize for the Humanities and the Balzan Prize, ...
's ''The Emergence of Probability'' and James Franklin's ''The Science of Conjecture'' for histories of the early development of the very concept of mathematical probability.
The theory of errors
In statistics, propagation of uncertainty (or propagation of error) is the effect of variables' uncertainties (or errors, more specifically random errors) on the uncertainty of a function based on them. When the variables are the values of ex ...
may be traced back to Roger Cotes
Roger Cotes (10 July 1682 – 5 June 1716) was an English mathematician, known for working closely with Isaac Newton by proofreading the second edition of his famous book, the '' Principia'', before publication. He also devised the quadrature ...
's ''Opera Miscellanea'' (posthumous, 1722), but a memoir prepared by Thomas Simpson in 1755 (printed 1756) first applied the theory to the discussion of errors of observation. The reprint (1757) of this memoir lays down the axioms that positive and negative errors are equally probable, and that certain assignable limits define the range of all errors. Simpson also discusses continuous errors and describes a probability curve.
The first two laws of error that were proposed both originated with Pierre-Simon Laplace
Pierre-Simon, Marquis de Laplace (; ; 23 March 1749 – 5 March 1827) was a French polymath, a scholar whose work has been instrumental in the fields of physics, astronomy, mathematics, engineering, statistics, and philosophy. He summariz ...
. The first law was published in 1774, and stated that the frequency of an error could be expressed as an exponential function of the numerical magnitude of the errordisregarding sign. The second law of error was proposed in 1778 by Laplace, and stated that the frequency of the error is an exponential function of the square of the error.Wilson EB (1923) "First and second laws of error". Journal of the American Statistical Association
The ''Journal of the American Statistical Association'' is a quarterly peer-reviewed scientific journal published by Taylor & Francis on behalf of the American Statistical Association. It covers work primarily focused on the application of statis ...
, 18, 143 The second law of error is called the normal distribution or the Gauss law. "It is difficult historically to attribute that law to Gauss, who in spite of his well-known precocity had probably not made this discovery before he was two years old."
Daniel Bernoulli
Daniel Bernoulli ( ; ; – 27 March 1782) was a Swiss people, Swiss-France, French mathematician and physicist and was one of the many prominent mathematicians in the Bernoulli family from Basel. He is particularly remembered for his applicati ...
(1778) introduced the principle of the maximum product of the probabilities of a system of concurrent errors.
Adrien-Marie Legendre
Adrien-Marie Legendre (; ; 18 September 1752 – 9 January 1833) was a French people, French mathematician who made numerous contributions to mathematics. Well-known and important concepts such as the Legendre polynomials and Legendre transforma ...
(1805) developed the method of least squares
The method of least squares is a mathematical optimization technique that aims to determine the best fit function by minimizing the sum of the squares of the differences between the observed values and the predicted values of the model. The me ...
, and introduced it in his ''Nouvelles méthodes pour la détermination des orbites des comètes'' (''New Methods for Determining the Orbits of Comets''). In ignorance of Legendre's contribution, an Irish-American writer, Robert Adrain, editor of "The Analyst" (1808), first deduced the law of facility of error,
where is a constant depending on precision of observation, and is a scale factor ensuring that the area under the curve equals 1. He gave two proofs, the second being essentially the same as John Herschel
Sir John Frederick William Herschel, 1st Baronet (; 7 March 1792 – 11 May 1871) was an English polymath active as a mathematician, astronomer, chemist, inventor and experimental photographer who invented the blueprint and did botanical work. ...
's (1850). Gauss
Johann Carl Friedrich Gauss (; ; ; 30 April 177723 February 1855) was a German mathematician, astronomer, Geodesy, geodesist, and physicist, who contributed to many fields in mathematics and science. He was director of the Göttingen Observat ...
gave the first proof that seems to have been known in Europe (the third after Adrain's) in 1809. Further proofs were given by Laplace (1810, 1812), Gauss (1823), James Ivory
James Francis Ivory (born Richard Jerome Hazen June 7, 1928) is an American film director, producer, and screenwriter. He was a principal in Merchant Ivory Productions along with Indian film producer Ismail Merchant (his domestic and professio ...
(1825, 1826), Hagen (1837), Friedrich Bessel
Friedrich Wilhelm Bessel (; 22 July 1784 – 17 March 1846) was a German astronomer, mathematician, physicist, and geodesy, geodesist. He was the first astronomer who determined reliable values for the distance from the Sun to another star by th ...
(1838), W.F. Donkin (1844, 1856), and Morgan Crofton (1870). Other contributors were Ellis (1844), De Morgan (1864), Glaisher (1872), and Giovanni Schiaparelli
Giovanni Virginio Schiaparelli ( , , ; 14 March 1835 – 4 July 1910) was an Italian astronomer and science historian.
Biography
He studied at the University of Turin, graduating in 1854, and later did research at Berlin Observatory, unde ...
(1875). Peters's (1856) formula for ''r'', the probable error
In statistics, probable error defines the half-range of an interval about a central point for the distribution, such that half of the values from the distribution will lie within the interval and half outside.Dodge, Y. (2006) ''The Oxford Dictiona ...
of a single observation, is well known.
In the nineteenth century, authors on the general theory included Laplace
Pierre-Simon, Marquis de Laplace (; ; 23 March 1749 – 5 March 1827) was a French polymath, a scholar whose work has been instrumental in the fields of physics, astronomy, mathematics, engineering, statistics, and philosophy. He summariz ...
, Sylvestre Lacroix Sylvestre can refer to:
People Surname
Given name
Middle name
* Carlos Sylvestre Begnis (1903–1980), Argentine medical doctor and politician
* Philippe Sylvestre Dufour (1622–1687), French Protestant apothecary, banker, collector, an ...
(1816), Littrow (1833), Adolphe Quetelet
Lambert Adolphe Jacques Quetelet FRSF or FRSE (; 22 February 1796 – 17 February 1874) was a Belgian- French astronomer, mathematician, statistician and sociologist who founded and directed the Brussels Observatory and was influential ...
(1853), Richard Dedekind
Julius Wilhelm Richard Dedekind (; ; 6 October 1831 – 12 February 1916) was a German mathematician who made important contributions to number theory, abstract algebra (particularly ring theory), and the axiomatic foundations of arithmetic. H ...
(1860), Helmert (1872), Hermann Laurent (1873), Liagre, Didion and Karl Pearson
Karl Pearson (; born Carl Pearson; 27 March 1857 – 27 April 1936) was an English biostatistician and mathematician. He has been credited with establishing the discipline of mathematical statistics. He founded the world's first university ...
. Augustus De Morgan
Augustus De Morgan (27 June 1806 – 18 March 1871) was a British mathematician and logician. He is best known for De Morgan's laws, relating logical conjunction, disjunction, and negation, and for coining the term "mathematical induction", the ...
and George Boole
George Boole ( ; 2 November 1815 – 8 December 1864) was a largely self-taught English mathematician, philosopher and logician, most of whose short career was spent as the first professor of mathematics at Queen's College, Cork in Ireland. H ...
improved the exposition of the theory.
In 1906, Andrey Markov
Andrey Andreyevich Markov (14 June 1856 – 20 July 1922) was a Russian mathematician best known for his work on stochastic processes. A primary subject of his research later became known as the Markov chain. He was also a strong, close to mas ...
introduced the notion of Markov chains
In probability theory and statistics, a Markov chain or Markov process is a stochastic process describing a sequence of possible events in which the probability of each event depends only on the state attained in the previous event. Informally, ...
, which played an important role in stochastic process
In probability theory and related fields, a stochastic () or random process is a mathematical object usually defined as a family of random variables in a probability space, where the index of the family often has the interpretation of time. Sto ...
es theory and its applications. The modern theory of probability based on measure theory
In mathematics, the concept of a measure is a generalization and formalization of geometrical measures (length, area, volume) and other common notions, such as magnitude (mathematics), magnitude, mass, and probability of events. These seemingl ...
was developed by Andrey Kolmogorov
Andrey Nikolaevich Kolmogorov ( rus, Андре́й Никола́евич Колмого́ров, p=ɐnˈdrʲej nʲɪkɐˈlajɪvʲɪtɕ kəlmɐˈɡorəf, a=Ru-Andrey Nikolaevich Kolmogorov.ogg, 25 April 1903 – 20 October 1987) was a Soviet ...
in 1931.
On the geometric side, contributors to ''The Educational Times'' included Miller, Crofton, McColl, Wolstenholme, Watson, and Artemas Martin
Artemas Martin (August 3, 1835 – November 7, 1918) was a self-educated American mathematician.
Biography
Martin was born on August 3, 1835, in Steuben County, New York, grew up in Venango County, Pennsylvania, and spent most of his life in ...
. See integral geometry In mathematics, integral geometry is the theory of measures on a geometrical space invariant under the symmetry group of that space. In more recent times, the meaning has been broadened to include a view of invariant (or equivariant) transformati ...
for more information.
Theory
Like othertheories
A theory is a systematic and rational form of abstract thinking about a phenomenon, or the conclusions derived from such thinking. It involves contemplative and logical reasoning, often supported by processes such as observation, experimentation, ...
, the theory of probability
Probability theory or probability calculus is the branch of mathematics concerned with probability. Although there are several different probability interpretations, probability theory treats the concept in a rigorous mathematical manner by expre ...
is a representation of its concepts in formal termsthat is, in terms that can be considered separately from their meaning. These formal terms are manipulated by the rules of mathematics and logic, and any results are interpreted or translated back into the problem domain.
There have been at least two successful attempts to formalize probability, namely the Kolmogorov
Andrey Nikolaevich Kolmogorov ( rus, Андре́й Никола́евич Колмого́ров, p=ɐnˈdrʲej nʲɪkɐˈlajɪvʲɪtɕ kəlmɐˈɡorəf, a=Ru-Andrey Nikolaevich Kolmogorov.ogg, 25 April 1903 – 20 October 1987) was a Soviet ...
formulation and the Cox formulation. In Kolmogorov's formulation (see also probability space
In probability theory, a probability space or a probability triple (\Omega, \mathcal, P) is a mathematical construct that provides a formal model of a random process or "experiment". For example, one can define a probability space which models ...
), sets are interpreted as events
Event may refer to:
Gatherings of people
* Ceremony, an event of ritual significance, performed on a special occasion
* Convention (meeting), a gathering of individuals engaged in some common interest
* Event management, the organization of eve ...
and probability as a measure on a class of sets. In Cox's theorem
Cox's theorem, named after the physicist Richard Threlkeld Cox, is a derivation of the laws of probability theory from a certain set of postulates. This derivation justifies the so-called "logical" interpretation of probability, as the laws of pr ...
, probability is taken as a primitive (i.e., not further analyzed), and the emphasis is on constructing a consistent assignment of probability values to propositions. In both cases, the laws of probability are the same, except for technical details.
There are other methods for quantifying uncertainty, such as the Dempster–Shafer theory
The theory of belief functions, also referred to as evidence theory or Dempster–Shafer theory (DST), is a general framework for reasoning with uncertainty, with understood connections to other frameworks such as probability, possibility and ...
or possibility theory
Possibility theory is a mathematical theory for dealing with certain types of uncertainty and is an alternative to probability theory. It uses measures of possibility and necessity between 0 and 1, ranging from impossible to possible and unnecessa ...
, but those are essentially different and not compatible with the usually-understood laws of probability.
Applications
Probability theory is applied in everyday life inrisk
In simple terms, risk is the possibility of something bad happening. Risk involves uncertainty about the effects/implications of an activity with respect to something that humans value (such as health, well-being, wealth, property or the environ ...
assessment and modeling. The insurance industry and markets use actuarial science
Actuarial science is the discipline that applies mathematics, mathematical and statistics, statistical methods to Risk assessment, assess risk in insurance, pension, finance, investment and other industries and professions.
Actuary, Actuaries a ...
to determine pricing and make trading decisions. Governments apply probabilistic methods in environmental regulation
Environmental laws are laws that protect the environment. The term "environmental law" encompasses treaties, statutes, regulations, conventions, and policies designed to protect the natural environment and manage the impact of human activitie ...
, entitlement analysis, and financial regulation
Financial regulation is a broad set of policies that apply to the financial sector in most jurisdictions, justified by two main features of finance: systemic risk, which implies that the failure of financial firms involves public interest consi ...
.
An example of the use of probability theory in equity trading is the effect of the perceived probability of any widespread Middle East conflict on oil prices, which have ripple effects in the economy as a whole. An assessment by a commodity trader that a war is more likely can send that commodity's prices up or down, and signals other traders of that opinion. Accordingly, the probabilities are neither assessed independently nor necessarily rationally. The theory of behavioral finance
Behavioral economics is the study of the psychological (e.g. cognitive, behavioral, affective, social) factors involved in the decisions of individuals or institutions, and how these decisions deviate from those implied by traditional economi ...
emerged to describe the effect of such groupthink
Groupthink is a psychological phenomenon that occurs within a group of people in which the desire for harmony or conformity in the group results in an irrational or dysfunctional decision-making outcome. Cohesiveness, or the desire for cohesivenes ...
on pricing, on policy, and on peace and conflict.
In addition to financial assessment, probability can be used to analyze trends in biology (e.g., disease spread) as well as ecology (e.g., biological Punnett squares
The Punnett square is a square diagram that is used to predict the genotypes of a particular cross or breeding experiment. It is named after Reginald C. Punnett, who devised the approach in 1905. The diagram is used by biologists to determine ...
). (11 pages) As with finance, risk assessment can be used as a statistical tool to calculate the likelihood of undesirable events occurring, and can assist with implementing protocols to avoid encountering such circumstances. Probability is used to design games of chance
A game of chance is in contrast with a game of skill. It is a game whose outcome is strongly influenced by some randomizing device. Common devices used include dice, spinning tops, playing cards, roulette wheels, numbered balls, or in the case ...
so that casinos can make a guaranteed profit, yet provide payouts to players that are frequent enough to encourage continued play.
Another significant application of probability theory in everyday life is reliability
Reliability, reliable, or unreliable may refer to:
Science, technology, and mathematics Computing
* Data reliability (disambiguation), a property of some disk arrays in computer storage
* Reliability (computer networking), a category used to des ...
. Many consumer products, such as automobiles
A car, or an automobile, is a motor vehicle with wheels. Most definitions of cars state that they run primarily on roads, Car seat, seat one to eight people, have four wheels, and mainly transport private transport#Personal transport, peopl ...
and consumer electronics, use reliability theory in product design to reduce the probability of failure. Failure probability may influence a manufacturer's decisions on a product's warranty
In law, a warranty is an expressed or implied promise or assurance of some kind. The term's meaning varies across legal subjects. In property law, it refers to a covenant by the grantor of a deed. In insurance law, it refers to a promise by the ...
.
The cache language model A cache language model is a type of statistical language model. These occur in the natural language processing subfield of computer science and assign probabilities to given sequences of words by means of a probability distribution. Statistical lang ...
and other statistical language models that are used in natural language processing
Natural language processing (NLP) is a subfield of computer science and especially artificial intelligence. It is primarily concerned with providing computers with the ability to process data encoded in natural language and is thus closely related ...
are also examples of applications of probability theory.
Mathematical treatment
Consider an experiment that can produce a number of results. The collection of all possible results is called thesample space
In probability theory, the sample space (also called sample description space, possibility space, or outcome space) of an experiment or random trial is the set of all possible outcomes or results of that experiment. A sample space is usually den ...
of the experiment, sometimes denoted as . The power set
In mathematics, the power set (or powerset) of a set is the set of all subsets of , including the empty set and itself. In axiomatic set theory (as developed, for example, in the ZFC axioms), the existence of the power set of any set is po ...
of the sample space is formed by considering all different collections of possible results. For example, rolling a die can produce six possible results. One collection of possible results gives an odd number on the die. Thus, the subset is an element of the power set
In mathematics, the power set (or powerset) of a set is the set of all subsets of , including the empty set and itself. In axiomatic set theory (as developed, for example, in the ZFC axioms), the existence of the power set of any set is po ...
of the sample space of dice rolls. These collections are called "events". In this case, is the event that the die falls on some odd number. If the results that actually occur fall in a given event, the event is said to have occurred.
A probability is a way of assigning every event a value between zero and one, with the requirement that the event made up of all possible results (in our example, the event ) is assigned a value of one. To qualify as a probability, the assignment of values must satisfy the requirement that for any collection of mutually exclusive events (events with no common results, such as the events , , and ), the probability that at least one of the events will occur is given by the sum of the probabilities of all the individual events.
The probability of an event
Event may refer to:
Gatherings of people
* Ceremony, an event of ritual significance, performed on a special occasion
* Convention (meeting), a gathering of individuals engaged in some common interest
* Event management, the organization of eve ...
''A'' is written as , , or . This mathematical definition of probability can extend to infinite sample spaces, and even uncountable sample spaces, using the concept of a measure.
The ''opposite'' or ''complement'' of an event ''A'' is the event ot ''A''(that is, the event of ''A'' not occurring), often denoted as , , or ; its probability is given by . As an example, the chance of not rolling a six on a six-sided die is For a more comprehensive treatment, see Complementary event
In probability theory, the complement of any event ''A'' is the event ot ''A'' i.e. the event that ''A'' does not occur.Robert R. Johnson, Patricia J. Kuby: ''Elementary Statistics''. Cengage Learning 2007, , p. 229 () The event ''A'' and ...
.
If two events ''A'' and ''B'' occur on a single performance of an experiment, this is called the intersection or joint probability
A joint or articulation (or articular surface) is the connection made between bones, ossicles, or other hard structures in the body which link an animal's skeletal system into a functional whole.Saladin, Ken. Anatomy & Physiology. 7th ed. McGra ...
of ''A'' and ''B'', denoted as
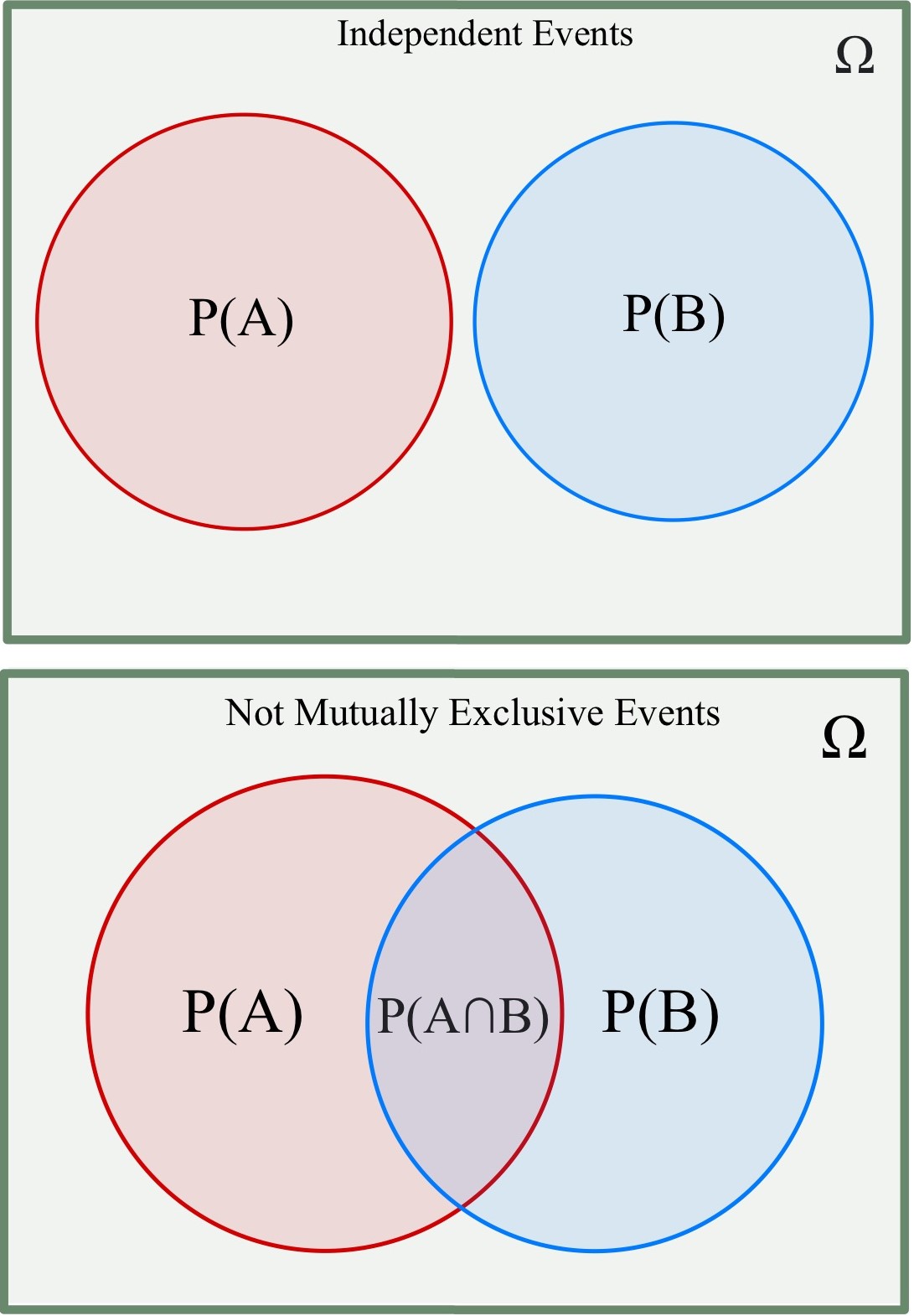
Independent events
If two events, ''A'' and ''B'' areindependent
Independent or Independents may refer to:
Arts, entertainment, and media Artist groups
* Independents (artist group), a group of modernist painters based in Pennsylvania, United States
* Independentes (English: Independents), a Portuguese artist ...
then the joint probability is
 For example, if two coins are flipped, then the chance of both being heads is
For example, if two coins are flipped, then the chance of both being heads is
Mutually exclusive events
If either event ''A'' or event ''B'' can occur but never both simultaneously, then they are called mutually exclusive events. If two events aremutually exclusive
In logic and probability theory, two events (or propositions) are mutually exclusive or disjoint if they cannot both occur at the same time. A clear example is the set of outcomes of a single coin toss, which can result in either heads or tails ...
, then the probability of ''both'' occurring is denoted as and If two events are mutually exclusive
In logic and probability theory, two events (or propositions) are mutually exclusive or disjoint if they cannot both occur at the same time. A clear example is the set of outcomes of a single coin toss, which can result in either heads or tails ...
, then the probability of ''either'' occurring is denoted as and
For example, the chance of rolling a 1 or 2 on a six-sided die is
Not (necessarily) mutually exclusive events
If the events are not (necessarily) mutually exclusive then Rewritten, For example, when drawing a card from a deck of cards, the chance of getting a heart or a face card (J, Q, K) (or both) is since among the 52 cards of a deck, 13 are hearts, 12 are face cards, and 3 are both: here the possibilities included in the "3 that are both" are included in each of the "13 hearts" and the "12 face cards", but should only be counted once. This can be expanded further for multiple not (necessarily) mutually exclusive events. For three events, this proceeds as follows:It can be seen, then, that this pattern can be repeated for any number of events.Conditional probability
''Conditional probability
In probability theory, conditional probability is a measure of the probability of an Event (probability theory), event occurring, given that another event (by assumption, presumption, assertion or evidence) is already known to have occurred. This ...
'' is the probability of some event ''A'', given the occurrence of some other event ''B''. Conditional probability is written , and is read "the probability of ''A'', given ''B''". It is defined by
If then is formally undefined
Undefined may refer to:
Mathematics
*Undefined (mathematics), with several related meanings
**Indeterminate form, in calculus
Computing
*Undefined behavior, computer code whose behavior is not specified under certain conditions
*Undefined valu ...
by this expression. In this case and are independent, since However, it is possible to define a conditional probability for some zero-probability events, for example by using a σ-algebra
In mathematical analysis and in probability theory, a σ-algebra ("sigma algebra") is part of the formalism for defining sets that can be measured. In calculus and analysis, for example, σ-algebras are used to define the concept of sets with a ...
of such events (such as those arising from a continuous random variable
In probability theory and statistics, a probability distribution is a function that gives the probabilities of occurrence of possible events for an experiment. It is a mathematical description of a random phenomenon in terms of its sample spa ...
).
For example, in a bag of 2 red balls and 2 blue balls (4 balls in total), the probability of taking a red ball is however, when taking a second ball, the probability of it being either a red ball or a blue ball depends on the ball previously taken. For example, if a red ball was taken, then the probability of picking a red ball again would be since only 1 red and 2 blue balls would have been remaining. And if a blue ball was taken previously, the probability of taking a red ball will be
Inverse probability
Inprobability theory
Probability theory or probability calculus is the branch of mathematics concerned with probability. Although there are several different probability interpretations, probability theory treats the concept in a rigorous mathematical manner by expre ...
and applications, ''Bayes' rule
Bayes' theorem (alternatively Bayes' law or Bayes' rule, after Thomas Bayes) gives a mathematical rule for inverting conditional probabilities, allowing one to find the probability of a cause given its effect. For example, if the risk of develo ...
'' relates the odds
In probability theory, odds provide a measure of the probability of a particular outcome. Odds are commonly used in gambling and statistics. For example for an event that is 40% probable, one could say that the odds are or
When gambling, o ...
of event to event before (prior to) and after (posterior to) conditioning Conditioning may refer to:
Science, computing, and technology
* Air conditioning, the removal of heat from indoor air for thermal comfort
** Automobile air conditioning, air conditioning in a vehicle
** Ice storage air conditioning, air conditio ...
on another event The odds on to event is simply the ratio of the probabilities of the two events. When arbitrarily many events are of interest, not just two, the rule can be rephrased as ''posterior is proportional to prior times likelihood'', where the proportionality symbol means that the left hand side is proportional to (i.e., equals a constant times) the right hand side as varies, for fixed or given (Lee, 2012; Bertsch McGrayne, 2012). In this form it goes back to Laplace (1774) and to Cournot (1843); see Fienberg (2005).
Summary of probabilities
Relation to randomness and probability in quantum mechanics
In adeterministic
Determinism is the metaphysical view that all events within the universe (or multiverse) can occur only in one possible way. Deterministic theories throughout the history of philosophy have developed from diverse and sometimes overlapping mo ...
universe, based on Newtonian concepts, there would be no probability if all conditions were known (Laplace's demon
In the history of science, Laplace's demon was a notable published articulation of causal determinism on a scientific basis by Pierre-Simon Laplace in 1814. According to determinism, if someone (the demon) knows the precise location and moment ...
) (but there are situations in which sensitivity to initial conditions exceeds our ability to measure them, i.e. know them). In the case of a roulette
Roulette (named after the French language, French word meaning "little wheel") is a casino game which was likely developed from the Italy, Italian game Biribi. In the game, a player may choose to place a bet on a single number, various grouping ...
wheel, if the force of the hand and the period of that force are known, the number on which the ball will stop would be a certainty (though as a practical matter, this would likely be true only of a roulette wheel that had not been exactly levelled – as Thomas A. Bass' Newtonian Casino revealed). This also assumes knowledge of inertia and friction of the wheel, weight, smoothness, and roundness of the ball, variations in hand speed during the turning, and so forth. A probabilistic description can thus be more useful than Newtonian mechanics for analyzing the pattern of outcomes of repeated rolls of a roulette wheel. Physicists face the same situation in the kinetic theory of gases
The kinetic theory of gases is a simple classical model of the thermodynamic behavior of gases. Its introduction allowed many principal concepts of thermodynamics to be established. It treats a gas as composed of numerous particles, too small ...
, where the system, while deterministic ''in principle'', is so complex (with the number of molecules typically the order of magnitude of the Avogadro constant
The Avogadro constant, commonly denoted or , is an SI defining constant with an exact value of when expressed in reciprocal moles.
It defines the ratio of the number of constituent particles to the amount of substance in a sample, where th ...
) that only a statistical description of its properties is feasible.
Probability theory
Probability theory or probability calculus is the branch of mathematics concerned with probability. Although there are several different probability interpretations, probability theory treats the concept in a rigorous mathematical manner by expre ...
is required to describe quantum phenomena. A revolutionary discovery of early 20th century physics
Physics is the scientific study of matter, its Elementary particle, fundamental constituents, its motion and behavior through space and time, and the related entities of energy and force. "Physical science is that department of knowledge whi ...
was the random character of all physical processes that occur at sub-atomic scales and are governed by the laws of quantum mechanics
Quantum mechanics is the fundamental physical Scientific theory, theory that describes the behavior of matter and of light; its unusual characteristics typically occur at and below the scale of atoms. Reprinted, Addison-Wesley, 1989, It is ...
. The objective wave function
In quantum physics, a wave function (or wavefunction) is a mathematical description of the quantum state of an isolated quantum system. The most common symbols for a wave function are the Greek letters and (lower-case and capital psi (letter) ...
evolves deterministically but, according to the Copenhagen interpretation
The Copenhagen interpretation is a collection of views about the meaning of quantum mechanics, stemming from the work of Niels Bohr, Werner Heisenberg, Max Born, and others. While "Copenhagen" refers to the Danish city, the use as an "interpretat ...
, it deals with probabilities of observing, the outcome being explained by a wave function collapse when an observation is made. However, the loss of determinism
Determinism is the Metaphysics, metaphysical view that all events within the universe (or multiverse) can occur only in one possible way. Deterministic theories throughout the history of philosophy have developed from diverse and sometimes ov ...
for the sake of instrumentalism
In philosophy of science and in epistemology, instrumentalism is a methodological view that ideas are useful instruments, and that the worth of an idea is based on how effective it is in explaining and predicting natural phenomena.
According to in ...
did not meet with universal approval. Albert Einstein
Albert Einstein (14 March 187918 April 1955) was a German-born theoretical physicist who is best known for developing the theory of relativity. Einstein also made important contributions to quantum mechanics. His mass–energy equivalence f ...
famously remarked in a letter to Max Born
Max Born (; 11 December 1882 – 5 January 1970) was a German-British theoretical physicist who was instrumental in the development of quantum mechanics. He also made contributions to solid-state physics and optics, and supervised the work of a ...
: "I am convinced that God does not play dice". Like Einstein, Erwin Schrödinger
Erwin Rudolf Josef Alexander Schrödinger ( ; ; 12 August 1887 – 4 January 1961), sometimes written as or , was an Austrian-Irish theoretical physicist who developed fundamental results in quantum field theory, quantum theory. In particul ...
, who discovered the wave function, believed quantum mechanics is a statistical
Statistics (from German language, German: ', "description of a State (polity), state, a country") is the discipline that concerns the collection, organization, analysis, interpretation, and presentation of data. In applying statistics to a s ...
approximation of an underlying deterministic reality
Reality is the sum or aggregate of everything in existence; everything that is not imagination, imaginary. Different Culture, cultures and Academic discipline, academic disciplines conceptualize it in various ways.
Philosophical questions abo ...
. In some modern interpretations of the statistical mechanics of measurement, quantum decoherence
Quantum decoherence is the loss of quantum coherence. It involves generally a loss of information of a system to its environment. Quantum decoherence has been studied to understand how quantum systems convert to systems that can be expla ...
is invoked to account for the appearance of subjectively probabilistic experimental outcomes.
See also
* Contingency * Equiprobability *Fuzzy logic
Fuzzy logic is a form of many-valued logic in which the truth value of variables may be any real number between 0 and 1. It is employed to handle the concept of partial truth, where the truth value may range between completely true and completely ...
* Heuristic (psychology)
Heuristics (from Ancient Greek εὑρίσκω, ''heurískō'', "I find, discover") is the process by which humans use mental shortcuts to arrive at decisions. Heuristics are simple strategies that humans, animals, organizations, and even machin ...
Notes
References
Bibliography
* Kallenberg, O. (2005) ''Probabilistic Symmetries and Invariance Principles''. Springer-Verlag, New York. 510 pp. * Kallenberg, O. (2002) ''Foundations of Modern Probability,'' 2nd ed. Springer Series in Statistics. 650 pp. * Olofsson, Peter (2005) ''Probability, Statistics, and Stochastic Processes'', Wiley-Interscience. 504 pp .External links
Virtual Laboratories in Probability and Statistics (Univ. of Ala.-Huntsville)
*
Probability and Statistics EBook
*
Edwin Thompson Jaynes
Edwin Thompson Jaynes (July 5, 1922 – April 30, 1998) was the Wayman Crow Distinguished Professor of Physics at Washington University in St. Louis. He wrote extensively on statistical mechanics and on foundations of probability and statistic ...
. ''Probability Theory: The Logic of Science''. Preprint: Washington University, (1996). �HTML index with links to PostScript files
an
(first three chapters)
* ttp://www.economics.soton.ac.uk/staff/aldrich/Probability%20Earliest%20Uses.htm Probability and Statistics on the Earliest Uses Pages (Univ. of Southampton)
Earliest Uses of Symbols in Probability and Statistics
o
A tutorial on probability and Bayes' theorem devised for first-year Oxford University students
, by Charles Grinstead, Laurie Snel
Source
''(
GNU Free Documentation License
The GNU Free Documentation License (GNU FDL or GFDL) is a copyleft license for free documentation, designed by the Free Software Foundation (FSF) for the GNU Project. It is similar to the GNU General Public License, giving readers the rights ...
)''
* Bruno de Finetti
Bruno de Finetti (13 June 1906 – 20 July 1985) was an Italian probabilist statistician and actuary, noted for the "operational subjective" conception of probability. The classic exposition of his distinctive theory is the 1937 , which discuss ...
, Probabilità e induzione
', Bologna, CLUEB, 1993. (digital version)
{{Authority control