Kepler–Poinsot polyhedron on:
[Wikipedia]
[Google]
[Amazon]
In
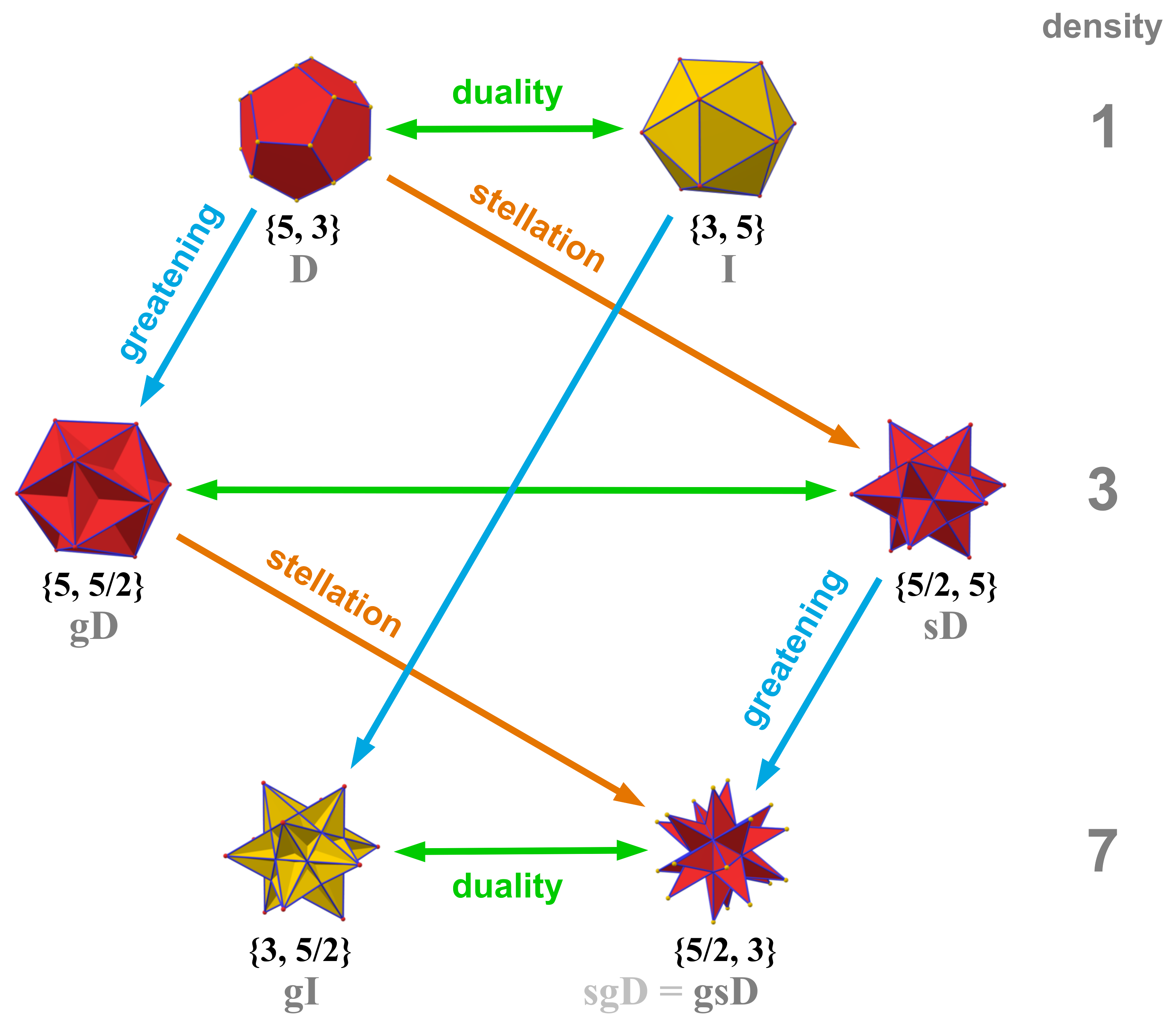
In his naming convention the
The three others are all the stellations of the
The three others are facetings of the icosahedron. If the intersections are treated as new edges and vertices, the figures obtained will not be regular, but they can still be considered
The pentagon faces of these cores are the invisible parts of the star polyhedra's pentagram faces.
For the small stellated dodecahedron the hull is times bigger than the core, and for the great it is times bigger.
(The midradius is a common measure to compare the size of different polyhedra.)
In the
** (Paper 1) H.S.M. Coxeter, ''The Nine Regular Solids'' roc. Can. Math. Congress 1 (1947), 252–264, MR 8, 482** (Paper 10) H.S.M. Coxeter, ''Star Polytopes and the Schlafli Function f(α,β,γ)'' lemente der Mathematik 44 (2) (1989) 25–36*
Paper models of Kepler–Poinsot polyhedraFree paper models (nets) of Kepler–Poinsot polyhedraThe Uniform Polyhedra
in Visual Polyhedra
Stella: Polyhedron Navigator
Software used to create many of the images on this page. {{DEFAULTSORT:Kepler-Poinsot Polyhedron Johannes Kepler Nonconvex polyhedra
geometry
Geometry (; ) is, with arithmetic, one of the oldest branches of mathematics. It is concerned with properties of space such as the distance, shape, size, and relative position of figures. A mathematician who works in the field of geometry is c ...
, a Kepler–Poinsot polyhedron is any of four regular star polyhedra
In geometry, a star polyhedron is a polyhedron which has some repetitive quality of nonconvexity giving it a star-like visual quality.
There are two general kinds of star polyhedron:
*Polyhedra which self-intersect in a repetitive way.
*Concave ...
.
They may be obtained by stellating the regular convex
Convex or convexity may refer to:
Science and technology
* Convex lens, in optics
Mathematics
* Convex set, containing the whole line segment that joins points
** Convex polygon, a polygon which encloses a convex set of points
** Convex polytop ...
dodecahedron
In geometry, a dodecahedron (Greek , from ''dōdeka'' "twelve" + ''hédra'' "base", "seat" or "face") or duodecahedron is any polyhedron with twelve flat faces. The most familiar dodecahedron is the regular dodecahedron with regular pentagon ...
and icosahedron
In geometry, an icosahedron ( or ) is a polyhedron with 20 faces. The name comes and . The plural can be either "icosahedra" () or "icosahedrons".
There are infinitely many non- similar shapes of icosahedra, some of them being more symmetrica ...
, and differ from these in having regular pentagram
A pentagram (sometimes known as a pentalpha, pentangle, or star pentagon) is a regular five-pointed star polygon, formed from the diagonal line segments of a convex (or simple, or non-self-intersecting) regular pentagon. Drawing a circle arou ...
mic face
The face is the front of an animal's head that features the eyes, nose and mouth, and through which animals express many of their emotions. The face is crucial for human identity, and damage such as scarring or developmental deformities may aff ...
s or vertex figure
In geometry, a vertex figure, broadly speaking, is the figure exposed when a corner of a polyhedron or polytope is sliced off.
Definitions
Take some corner or vertex of a polyhedron. Mark a point somewhere along each connected edge. Draw line ...
s. They can all be seen as three-dimensional analogues of the pentagram in one way or another.
Characteristics
Non-convexity
These figures havepentagram
A pentagram (sometimes known as a pentalpha, pentangle, or star pentagon) is a regular five-pointed star polygon, formed from the diagonal line segments of a convex (or simple, or non-self-intersecting) regular pentagon. Drawing a circle arou ...
s (star pentagons) as faces or vertex figures. The small
Small may refer to:
Science and technology
* SMALL, an ALGOL-like programming language
* Small (anatomy), the lumbar region of the back
* ''Small'' (journal), a nano-science publication
* <small>, an HTML element that defines smaller text ...
and great stellated dodecahedron
In geometry, the great stellated dodecahedron is a Kepler-Poinsot polyhedron, with Schläfli symbol . It is one of four nonconvex regular polyhedra.
It is composed of 12 intersecting pentagrammic faces, with three pentagrams meeting at each ve ...
have nonconvex regular pentagram
A pentagram (sometimes known as a pentalpha, pentangle, or star pentagon) is a regular five-pointed star polygon, formed from the diagonal line segments of a convex (or simple, or non-self-intersecting) regular pentagon. Drawing a circle arou ...
faces. The great dodecahedron
In geometry, the great dodecahedron is a Kepler–Poinsot polyhedron, with Schläfli symbol and Coxeter–Dynkin diagram of . It is one of four nonconvex regular polyhedra. It is composed of 12 pentagonal faces (six pairs of parallel pentagon ...
and great icosahedron
In geometry, the great icosahedron is one of four Kepler–Poinsot polyhedra (nonconvex regular polyhedra), with Schläfli symbol and Coxeter-Dynkin diagram of . It is composed of 20 intersecting triangular faces, having five triangles meeti ...
have convex
Convex or convexity may refer to:
Science and technology
* Convex lens, in optics
Mathematics
* Convex set, containing the whole line segment that joins points
** Convex polygon, a polygon which encloses a convex set of points
** Convex polytop ...
polygonal faces, but pentagrammic vertex figure
In geometry, a vertex figure, broadly speaking, is the figure exposed when a corner of a polyhedron or polytope is sliced off.
Definitions
Take some corner or vertex of a polyhedron. Mark a point somewhere along each connected edge. Draw line ...
s.
In all cases, two faces can intersect along a line that is not an edge of either face, so that part of each face passes through the interior of the figure. Such lines of intersection are not part of the polyhedral structure and are sometimes called false edges. Likewise where three such lines intersect at a point that is not a corner of any face, these points are false vertices. The images below show spheres at the true vertices, and blue rods along the true edges.
For example, the small stellated dodecahedron
In geometry, the small stellated dodecahedron is a Kepler-Poinsot polyhedron, named by Arthur Cayley, and with Schläfli symbol . It is one of four nonconvex regular polyhedra. It is composed of 12 pentagrammic faces, with five pentagrams meeti ...
has 12 pentagram
A pentagram (sometimes known as a pentalpha, pentangle, or star pentagon) is a regular five-pointed star polygon, formed from the diagonal line segments of a convex (or simple, or non-self-intersecting) regular pentagon. Drawing a circle arou ...
faces with the central pentagon
In geometry, a pentagon (from the Greek πέντε ''pente'' meaning ''five'' and γωνία ''gonia'' meaning ''angle'') is any five-sided polygon or 5-gon. The sum of the internal angles in a simple pentagon is 540°.
A pentagon may be sim ...
al part hidden inside the solid. The visible parts of each face comprise five isosceles triangle
In geometry, an isosceles triangle () is a triangle that has two sides of equal length. Sometimes it is specified as having ''exactly'' two sides of equal length, and sometimes as having ''at least'' two sides of equal length, the latter versio ...
s which touch at five points around the pentagon. We could treat these triangles as 60 separate faces to obtain a new, irregular polyhedron which looks outwardly identical. Each edge would now be divided into three shorter edges (of two different kinds), and the 20 false vertices would become true ones, so that we have a total of 32 vertices (again of two kinds). The hidden inner pentagons are no longer part of the polyhedral surface, and can disappear. Now Euler's formula
Euler's formula, named after Leonhard Euler, is a mathematical formula in complex analysis that establishes the fundamental relationship between the trigonometric functions and the complex exponential function. Euler's formula states that ...
holds: 60 − 90 + 32 = 2. However, this polyhedron is no longer the one described by the Schläfli symbol
In geometry, the Schläfli symbol is a notation of the form \ that defines regular polytopes and tessellations.
The Schläfli symbol is named after the 19th-century Swiss mathematician Ludwig Schläfli, who generalized Euclidean geometry to mo ...
, and so can not be a Kepler–Poinsot solid even though it still looks like one from outside.
Euler characteristic χ
A Kepler–Poinsot polyhedron covers its circumscribed sphere more than once, with the centers of faces acting as winding points in the figures which have pentagrammic faces, and the vertices in the others. Because of this, they are not necessarily topologically equivalent to the sphere as Platonic solids are, and in particular the Euler relation : does not always hold. Schläfli held that all polyhedra must have χ = 2, and he rejected the small stellated dodecahedron and great dodecahedron as proper polyhedra. This view was never widely held. A modified form of Euler's formula, usingdensity
Density (volumetric mass density or specific mass) is the substance's mass per unit of volume. The symbol most often used for density is ''ρ'' (the lower case Greek letter rho), although the Latin letter ''D'' can also be used. Mathematicall ...
(''D'') of the vertex figure
In geometry, a vertex figure, broadly speaking, is the figure exposed when a corner of a polyhedron or polytope is sliced off.
Definitions
Take some corner or vertex of a polyhedron. Mark a point somewhere along each connected edge. Draw line ...
s () and faces () was given by Arthur Cayley
Arthur Cayley (; 16 August 1821 – 26 January 1895) was a prolific British mathematician who worked mostly on algebra. He helped found the modern British school of pure mathematics.
As a child, Cayley enjoyed solving complex maths problem ...
, and holds both for convex polyhedra (where the correction factors are all 1), and the Kepler–Poinsot polyhedra:
:
Duality and Petrie polygons
The Kepler–Poinsot polyhedra exist in dual pairs. Duals have the samePetrie polygon
In geometry, a Petrie polygon for a regular polytope of dimensions is a skew polygon in which every consecutive sides (but no ) belongs to one of the facets. The Petrie polygon of a regular polygon is the regular polygon itself; that of a ...
, or more precisely, Petrie polygons with the same two dimensional projection.
The following images show the two dual compounds with the same edge radius. They also show that the Petrie polygons are skew.
Two relationships described in the article below are also easily seen in the images: That the violet edges are the same, and that the green faces lie in the same planes.
Summary
Relationships among the regular polyhedra

Conway's operational terminology
John Conway defines the Kepler–Poinsot polyhedra as ''greatenings'' and ''stellations'' of the convex solids.In his naming convention the
small stellated dodecahedron
In geometry, the small stellated dodecahedron is a Kepler-Poinsot polyhedron, named by Arthur Cayley, and with Schläfli symbol . It is one of four nonconvex regular polyhedra. It is composed of 12 pentagrammic faces, with five pentagrams meeti ...
is just the ''stellated dodecahedron''.
''Stellation'' changes pentagonal faces into pentagrams. (In this sense stellation is a unique operation, and not to be confused with the more general stellation
In geometry, stellation is the process of extending a polygon in two dimensions, polyhedron in three dimensions, or, in general, a polytope in ''n'' dimensions to form a new figure. Starting with an original figure, the process extends specif ...
described below.)
''Greatening'' maintains the type of faces, shifting and resizing them into parallel planes.
Stellations and facetings
Thegreat icosahedron
In geometry, the great icosahedron is one of four Kepler–Poinsot polyhedra (nonconvex regular polyhedra), with Schläfli symbol and Coxeter-Dynkin diagram of . It is composed of 20 intersecting triangular faces, having five triangles meeti ...
is one of the stellation
In geometry, stellation is the process of extending a polygon in two dimensions, polyhedron in three dimensions, or, in general, a polytope in ''n'' dimensions to form a new figure. Starting with an original figure, the process extends specif ...
s of the icosahedron
In geometry, an icosahedron ( or ) is a polyhedron with 20 faces. The name comes and . The plural can be either "icosahedra" () or "icosahedrons".
There are infinitely many non- similar shapes of icosahedra, some of them being more symmetrica ...
. (See The Fifty-Nine Icosahedra
''The Fifty-Nine Icosahedra'' is a book written and illustrated by H. S. M. Coxeter, P. Du Val, H. T. Flather and J. F. Petrie. It enumerates certain stellations of the regular convex or Platonic icosahedron, according to a set of rules put forw ...
)The three others are all the stellations of the
dodecahedron
In geometry, a dodecahedron (Greek , from ''dōdeka'' "twelve" + ''hédra'' "base", "seat" or "face") or duodecahedron is any polyhedron with twelve flat faces. The most familiar dodecahedron is the regular dodecahedron with regular pentagon ...
.
The great stellated dodecahedron
In geometry, the great stellated dodecahedron is a Kepler-Poinsot polyhedron, with Schläfli symbol . It is one of four nonconvex regular polyhedra.
It is composed of 12 intersecting pentagrammic faces, with three pentagrams meeting at each ve ...
is a faceting
Stella octangula as a faceting of the cube
In geometry, faceting (also spelled facetting) is the process of removing parts of a polygon, polyhedron or polytope, without creating any new vertices.
New edges of a faceted polyhedron may be ...
of the dodecahedron.The three others are facetings of the icosahedron. If the intersections are treated as new edges and vertices, the figures obtained will not be regular, but they can still be considered
stellation
In geometry, stellation is the process of extending a polygon in two dimensions, polyhedron in three dimensions, or, in general, a polytope in ''n'' dimensions to form a new figure. Starting with an original figure, the process extends specif ...
s.
(See also List of Wenninger polyhedron models
This is an indexed list of the uniform and stellated polyhedra from the book ''Polyhedron Models'', by Magnus Wenninger.
The book was written as a guide book to building polyhedra as physical models. It includes templates of face elements for cons ...
)
Shared vertices and edges
The great stellated dodecahedron shares its vertices with the dodecahedron. The other three Kepler–Poinsot polyhedra share theirs with the icosahedron.The stellated dodecahedra
Hull and core
Thesmall
Small may refer to:
Science and technology
* SMALL, an ALGOL-like programming language
* Small (anatomy), the lumbar region of the back
* ''Small'' (journal), a nano-science publication
* <small>, an HTML element that defines smaller text ...
and great
Great may refer to: Descriptions or measurements
* Great, a relative measurement in physical space, see Size
* Greatness, being divine, majestic, superior, majestic, or transcendent
People
* List of people known as "the Great"
*Artel Great (born ...
stellated dodecahedron
can be seen as a regular and a great dodecahedron
In geometry, the great dodecahedron is a Kepler–Poinsot polyhedron, with Schläfli symbol and Coxeter–Dynkin diagram of . It is one of four nonconvex regular polyhedra. It is composed of 12 pentagonal faces (six pairs of parallel pentagon ...
with their edges and faces extended until they intersect.The pentagon faces of these cores are the invisible parts of the star polyhedra's pentagram faces.
For the small stellated dodecahedron the hull is times bigger than the core, and for the great it is times bigger.
(The midradius is a common measure to compare the size of different polyhedra.)
Augmentations
Traditionally the two star polyhedra have been defined as ''augmentations'' (or ''cumulations''), Kepler calls the small stellation an ''augmented dodecahedron'' (then nicknaming it ''hedgehog''). Thesenaïve
Naivety (also spelled naïvety), naiveness, or naïveté is the state of being naive. It refers to an apparent or actual lack of experience and sophistication, often describing a neglect of pragmatism in favor of moral idealism. A ''naïve'' may ...
definitions are still used.
E.g. MathWorld
''MathWorld'' is an online mathematics reference work, created and largely written by Eric W. Weisstein. It is sponsored by and licensed to Wolfram Research, Inc. and was partially funded by the National Science Foundation's National Science Di ...
states that the two star polyhedra can be constructed by adding pyramids to the faces of the Platonic solids.
Symmetry
All Kepler–Poinsot polyhedra have fullicosahedral symmetry
In mathematics, and especially in geometry, an object has icosahedral symmetry if it has the same symmetries as a regular icosahedron. Examples of other polyhedra with icosahedral symmetry include the regular dodecahedron (the dual polyhedr ...
, just like their convex hulls.
The great icosahedron
In geometry, the great icosahedron is one of four Kepler–Poinsot polyhedra (nonconvex regular polyhedra), with Schläfli symbol and Coxeter-Dynkin diagram of . It is composed of 20 intersecting triangular faces, having five triangles meeti ...
and its dual resemble the icosahedron and its dual in that they have faces and vertices on the 3-fold (yellow) and 5-fold (red) symmetry axes.In the
great dodecahedron
In geometry, the great dodecahedron is a Kepler–Poinsot polyhedron, with Schläfli symbol and Coxeter–Dynkin diagram of . It is one of four nonconvex regular polyhedra. It is composed of 12 pentagonal faces (six pairs of parallel pentagon ...
and its dual all faces and vertices are on 5-fold symmetry axes (so there are no yellow elements in these images).
The following table shows the solids in pairs of duals. In the top row they are shown with pyritohedral symmetry
image:tetrahedron.jpg, 150px, A regular tetrahedron, an example of a solid with full tetrahedral symmetry
A regular tetrahedron has 12 rotational (or orientation-preserving) symmetries, and a symmetry order of 24 including transformations that c ...
, in the bottom row with icosahedral symmetry (to which the mentioned colors refer).
The table below shows orthographic projection
Orthographic projection (also orthogonal projection and analemma) is a means of representing three-dimensional objects in two dimensions. Orthographic projection is a form of parallel projection in which all the projection lines are orthogona ...
s from the 5-fold (red), 3-fold (yellow) and 2-fold (blue) symmetry axes.
History
Most, if not all, of the Kepler-Poinsot polyhedra were known of in some form or other before Kepler. A small stellated dodecahedron appears in a marble tarsia (inlay panel) on the floor ofSt. Mark's Basilica
The Patriarchal Cathedral Basilica of Saint Mark ( it, Basilica Cattedrale Patriarcale di San Marco), commonly known as St Mark's Basilica ( it, Basilica di San Marco; vec, Baxéłega de San Marco), is the cathedral church of the Catholic Pat ...
, Venice
Venice ( ; it, Venezia ; vec, Venesia or ) is a city in northeastern Italy and the capital of the Veneto Regions of Italy, region. It is built on a group of 118 small islands that are separated by canals and linked by over 400 ...
, Italy. It dates from the 15th century and is sometimes attributed to Paolo Uccello.
In his ''Perspectiva corporum regularium
(from Latin: ''Perspective of the Regular Solids'') is a book of perspective drawings of polyhedra by German Renaissance goldsmith Wenzel Jamnitzer, with engravings by Jost Amman, published in 1568.
Despite its Latin title, is written mainly ...
'' (''Perspectives of the regular solids''), a book of woodcuts published in 1568, Wenzel Jamnitzer
Wenzel Jamnitzer (sometimes Jamitzer, or Wenzel ''Gemniczer'') (1507/1508 – 19 December 1585) was a Northern Mannerist goldsmith, artist, and printmaker in etching, who worked in Nuremberg. He was the best known German goldsmith of his e ...
depicts the great stellated dodecahedron
In geometry, the great stellated dodecahedron is a Kepler-Poinsot polyhedron, with Schläfli symbol . It is one of four nonconvex regular polyhedra.
It is composed of 12 intersecting pentagrammic faces, with three pentagrams meeting at each ve ...
and a great dodecahedron
In geometry, the great dodecahedron is a Kepler–Poinsot polyhedron, with Schläfli symbol and Coxeter–Dynkin diagram of . It is one of four nonconvex regular polyhedra. It is composed of 12 pentagonal faces (six pairs of parallel pentagon ...
(both shown below). There is also a truncated version of the small stellated dodecahedron
In geometry, the small stellated dodecahedron is a Kepler-Poinsot polyhedron, named by Arthur Cayley, and with Schläfli symbol . It is one of four nonconvex regular polyhedra. It is composed of 12 pentagrammic faces, with five pentagrams meeti ...
. It is clear from the general arrangement of the book that he regarded only the five Platonic solids as regular.
The small and great stellated dodecahedra, sometimes called the Kepler polyhedra, were first recognized as regular by Johannes Kepler
Johannes Kepler (; ; 27 December 1571 – 15 November 1630) was a German astronomer, mathematician, astrologer, natural philosopher and writer on music. He is a key figure in the 17th-century Scientific Revolution, best known for his laws ...
around 1619.H.S.M. Coxeter,P. Du Val, H.T. Flather and J.F. Petrie; ''The Fifty-Nine Icosahedra'', 3rd Edition, Tarquin, 1999. p.11 He obtained them by stellating the regular convex dodecahedron, for the first time treating it as a surface rather than a solid. He noticed that by extending the edges or faces of the convex dodecahedron until they met again, he could obtain star pentagons. Further, he recognized that these star pentagons are also regular. In this way he constructed the two stellated dodecahedra. Each has the central convex region of each face "hidden" within the interior, with only the triangular arms visible. Kepler's final step was to recognize that these polyhedra fit the definition of regularity, even though they were not convex
Convex or convexity may refer to:
Science and technology
* Convex lens, in optics
Mathematics
* Convex set, containing the whole line segment that joins points
** Convex polygon, a polygon which encloses a convex set of points
** Convex polytop ...
, as the traditional Platonic solid
In geometry, a Platonic solid is a convex, regular polyhedron in three-dimensional Euclidean space. Being a regular polyhedron means that the faces are congruent (identical in shape and size) regular polygons (all angles congruent and all e ...
s were.
In 1809, Louis Poinsot
Louis Poinsot (3 January 1777 – 5 December 1859) was a French mathematician and physicist. Poinsot was the inventor of geometrical mechanics, showing how a system of forces acting on a rigid body could be resolved into a single force and a ...
rediscovered Kepler's figures, by assembling star pentagons around each vertex. He also assembled convex polygons around star vertices to discover two more regular stars, the great icosahedron and great dodecahedron. Some people call these two the Poinsot polyhedra. Poinsot did not know if he had discovered all the regular star polyhedra.
Three years later, Augustin Cauchy proved the list complete by stellating the Platonic solid
In geometry, a Platonic solid is a convex, regular polyhedron in three-dimensional Euclidean space. Being a regular polyhedron means that the faces are congruent (identical in shape and size) regular polygons (all angles congruent and all e ...
s, and almost half a century after that, in 1858, Bertrand
Bertrand may refer to:
Places
* Bertrand, Missouri, US
* Bertrand, Nebraska, US
* Bertrand, New Brunswick, Canada
* Bertrand Township, Michigan, US
* Bertrand, Michigan
* Bertrand, Virginia, US
* Bertrand Creek, state of Washington
* Saint-Ber ...
provided a more elegant proof by faceting
Stella octangula as a faceting of the cube
In geometry, faceting (also spelled facetting) is the process of removing parts of a polygon, polyhedron or polytope, without creating any new vertices.
New edges of a faceted polyhedron may be ...
them.
The following year, Arthur Cayley
Arthur Cayley (; 16 August 1821 – 26 January 1895) was a prolific British mathematician who worked mostly on algebra. He helped found the modern British school of pure mathematics.
As a child, Cayley enjoyed solving complex maths problem ...
gave the Kepler–Poinsot polyhedra the names by which they are generally known today.
A hundred years later, John Conway developed a systematic terminology for stellations in up to four dimensions. Within this scheme the small stellated dodecahedron
In geometry, the small stellated dodecahedron is a Kepler-Poinsot polyhedron, named by Arthur Cayley, and with Schläfli symbol . It is one of four nonconvex regular polyhedra. It is composed of 12 pentagrammic faces, with five pentagrams meeti ...
is just the ''stellated dodecahedron''.
Regular star polyhedra in art and culture
Adissection
Dissection (from Latin ' "to cut to pieces"; also called anatomization) is the dismembering of the body of a deceased animal or plant to study its anatomical structure. Autopsy is used in pathology and forensic medicine to determine the cause o ...
of the great dodecahedron was used for the 1980s puzzle Alexander's Star
Alexander's Star is a puzzle similar to the Rubik's Cube, in the shape of a great dodecahedron.
History
Alexander's Star was invented by Adam Alexander, an American mathematician, in 1982. It was patented on 26 March 1985, with US patent num ...
.
Regular star polyhedra first appear in Renaissance art. A small stellated dodecahedron is depicted in a marble tarsia on the floor of St. Mark's Basilica, Venice, Italy, dating from ca. 1430 and sometimes attributed to Paulo Ucello.
In the 20th Century, Artist M. C. Escher
Maurits Cornelis Escher (; 17 June 1898 – 27 March 1972) was a Dutch graphic artist who made mathematically inspired woodcuts, lithographs, and mezzotints.
Despite wide popular interest, Escher was for most of his life neglected in t ...
's interest in geometric forms often led to works based on or including regular solids; ''Gravitation
In physics, gravity () is a fundamental interaction which causes mutual attraction between all things with mass or energy. Gravity is, by far, the weakest of the four fundamental interactions, approximately 1038 times weaker than the stron ...
'' is based on a small stellated dodecahedron.
Norwegian artist Vebjørn Sand
Vebjørn Sand (born March 11, 1966, in Bærum) is a Norway, Norwegian painter and artist. He is known for his paintings as well as his public arts projects, such as the Vebjørn Sand Da Vinci Project, Da Vinci Project, and the Kepler–Poinsot po ...
's sculpture ''The Kepler Star'' is displayed near Oslo Airport, Gardermoen
Oslo Airport ( no, Oslo lufthavn; ), alternatively referred to as Oslo Gardermoen Airport or simply Gardermoen, is the international airport serving Oslo, Norway, the capital and most populous city in the country. A hub for Flyr, Norse Atl ...
. The star spans 14 meters, and consists of an icosahedron
In geometry, an icosahedron ( or ) is a polyhedron with 20 faces. The name comes and . The plural can be either "icosahedra" () or "icosahedrons".
There are infinitely many non- similar shapes of icosahedra, some of them being more symmetrica ...
and a dodecahedron
In geometry, a dodecahedron (Greek , from ''dōdeka'' "twelve" + ''hédra'' "base", "seat" or "face") or duodecahedron is any polyhedron with twelve flat faces. The most familiar dodecahedron is the regular dodecahedron with regular pentagon ...
inside a great stellated dodecahedron.
See also
*Regular polytope
In mathematics, a regular polytope is a polytope whose symmetry group acts transitively on its flags, thus giving it the highest degree of symmetry. All its elements or -faces (for all , where is the dimension of the polytope) — cells, ...
* Regular polyhedron
A regular polyhedron is a polyhedron whose symmetry group acts transitively on its flags. A regular polyhedron is highly symmetrical, being all of edge-transitive, vertex-transitive and face-transitive. In classical contexts, many different equival ...
* List of regular polytopes
This article lists the regular polytopes and regular polytope compounds in Euclidean, spherical and hyperbolic spaces.
The Schläfli symbol describes every regular tessellation of an ''n''-sphere, Euclidean and hyperbolic spaces. A Schläfli ...
* Uniform polyhedron
In geometry, a uniform polyhedron has regular polygons as Face (geometry), faces and is vertex-transitive (i.e., there is an isometry mapping any vertex onto any other). It follows that all vertices are congruence (geometry), congruent.
Unifor ...
* Uniform star polyhedron
In geometry, a uniform star polyhedron is a self-intersecting uniform polyhedron. They are also sometimes called nonconvex polyhedra to imply self-intersecting. Each polyhedron can contain either star polygon faces, star polygon vertex figures, ...
* Polyhedral compound
In geometry, a polyhedral compound is a figure that is composed of several polyhedra sharing a common centre. They are the three-dimensional analogs of polygonal compounds such as the hexagram.
The outer vertices of a compound can be connec ...
* Regular star 4-polytope
In mathematics, a regular 4-polytope is a regular four-dimensional polytope. They are the four-dimensional analogues of the regular polyhedra in three dimensions and the regular polygons in two dimensions.
There are six convex and ten star reg ...
– the ten regular star 4-polytope
In geometry, a 4-polytope (sometimes also called a polychoron, polycell, or polyhedroid) is a four-dimensional polytope. It is a connected and closed figure, composed of lower-dimensional polytopal elements: vertices, edges, faces (polygons), an ...
s, 4-dimensional analogues of the Kepler–Poinsot polyhedra
References
Notes
Bibliography
* J. Bertrand, Note sur la théorie des polyèdres réguliers, ''Comptes rendus des séances de l'Académie des Sciences'', 46 (1858), pp. 79–82, 117. *Augustin-Louis Cauchy
Baron Augustin-Louis Cauchy (, ; ; 21 August 178923 May 1857) was a French mathematician, engineer, and physicist who made pioneering contributions to several branches of mathematics, including mathematical analysis and continuum mechanics. H ...
, ''Recherches sur les polyèdres.'' J. de l'École Polytechnique 9, 68–86, 1813.
* Arthur Cayley
Arthur Cayley (; 16 August 1821 – 26 January 1895) was a prolific British mathematician who worked mostly on algebra. He helped found the modern British school of pure mathematics.
As a child, Cayley enjoyed solving complex maths problem ...
, On Poinsot's Four New Regular Solids. ''Phil. Mag.'' 17, pp. 123–127 and 209, 1859.
* John H. Conway
John Horton Conway (26 December 1937 – 11 April 2020) was an English mathematician active in the theory of finite groups, knot theory, number theory, combinatorial game theory and coding theory. He also made contributions to many branches o ...
, Heidi Burgiel, Chaim Goodman-Strauss, ''The Symmetry of Things'' 2008, (Chapter 24, Regular Star-polytopes, pp. 404–408)
* ''Kaleidoscopes: Selected Writings of H. S. M. Coxeter
Harold Scott MacDonald "Donald" Coxeter, (9 February 1907 – 31 March 2003) was a British and later also Canadian geometer. He is regarded as one of the greatest geometers of the 20th century.
Biography
Coxeter was born in Kensington t ...
'', edited by F. Arthur Sherk, Peter McMullen, Anthony C. Thompson, Asia Ivic Weiss, Wiley-Interscience Publication, 1995,** (Paper 1) H.S.M. Coxeter, ''The Nine Regular Solids'' roc. Can. Math. Congress 1 (1947), 252–264, MR 8, 482** (Paper 10) H.S.M. Coxeter, ''Star Polytopes and the Schlafli Function f(α,β,γ)'' lemente der Mathematik 44 (2) (1989) 25–36*
Theoni Pappas
Theoni Pappas (born 1944) is an American mathematics teacher known for her books and calendars concerning popular mathematics.
Pappas is a graduate of the University of California, Berkeley, and earned a master's degree at Stanford University
...
, (The Kepler–Poinsot Solids) ''The Joy of Mathematics''. San Carlos, CA: Wide World Publ./Tetra, p. 113, 1989.
* Louis Poinsot
Louis Poinsot (3 January 1777 – 5 December 1859) was a French mathematician and physicist. Poinsot was the inventor of geometrical mechanics, showing how a system of forces acting on a rigid body could be resolved into a single force and a ...
, Memoire sur les polygones et polyèdres. ''J. de l'École Polytechnique'' 9, pp. 16–48, 1810.
* Lakatos, Imre; ''Proofs and Refutations'', Cambridge University Press (1976) - discussion of proof of Euler characteristic
* , pp. 39–41.
* John H. Conway
John Horton Conway (26 December 1937 – 11 April 2020) was an English mathematician active in the theory of finite groups, knot theory, number theory, combinatorial game theory and coding theory. He also made contributions to many branches o ...
, Heidi Burgiel, Chaim Goodman-Strauss, ''The Symmetries of Things'' 2008, (Chapter 26. pp. 404: Regular star-polytopes Dimension 3)
* Chapter 8: Kepler Poisot polyhedra
External links
*Paper models of Kepler–Poinsot polyhedra
in Visual Polyhedra
Software used to create many of the images on this page. {{DEFAULTSORT:Kepler-Poinsot Polyhedron Johannes Kepler Nonconvex polyhedra