Cylindrical symmetry on:
[Wikipedia]
[Google]
[Amazon]
In
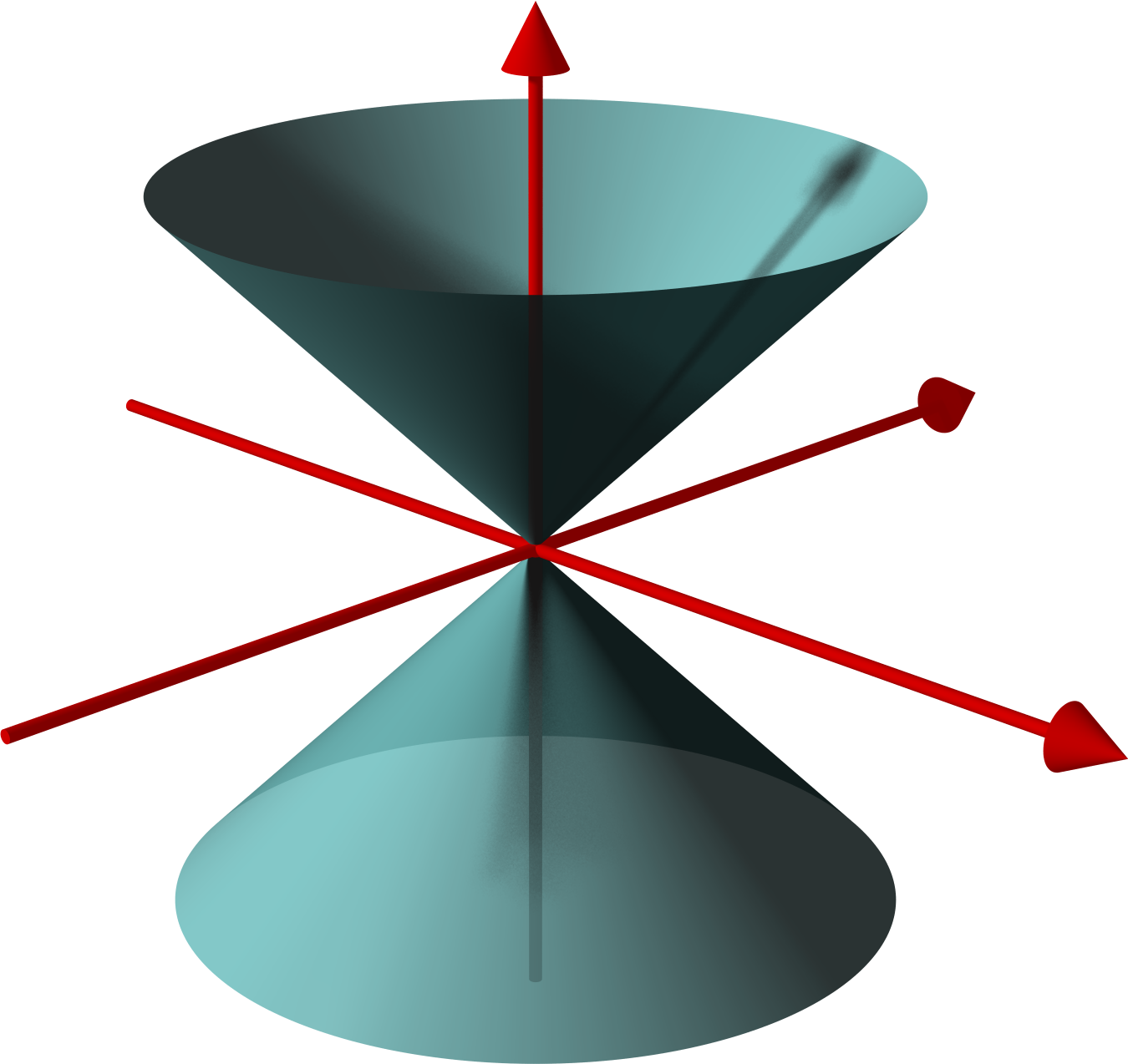In 3-dimensions, a
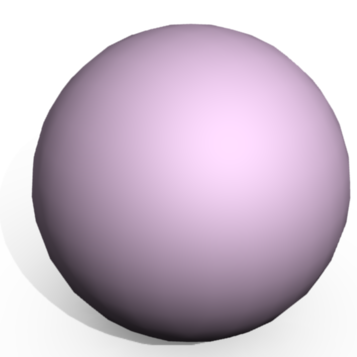An analogous 3-dimensional equivalent term is spherical symmetry.
Rotational spherical symmetry is isomorphic with the
geometry
Geometry (; ) is, with arithmetic, one of the oldest branches of mathematics. It is concerned with properties of space such as the distance, shape, size, and relative position of figures. A mathematician who works in the field of geometry is ...
, circular symmetry is a type of continuous symmetry
In mathematics, continuous symmetry is an intuitive idea corresponding to the concept of viewing some symmetries as motions, as opposed to discrete symmetry, e.g. reflection symmetry, which is invariant under a kind of flip from one state to ano ...
for a planar object that can be rotated by any arbitrary angle
In Euclidean geometry, an angle is the figure formed by two rays, called the '' sides'' of the angle, sharing a common endpoint, called the '' vertex'' of the angle.
Angles formed by two rays lie in the plane that contains the rays. Angles a ...
and map onto itself.
Rotational circular symmetry is isomorphic with the circle group in the complex plane, or the special orthogonal group SO(2), and unitary group
In mathematics, the unitary group of degree ''n'', denoted U(''n''), is the group of unitary matrices, with the group operation of matrix multiplication. The unitary group is a subgroup of the general linear group . Hyperorthogonal group is ...
U(1). Reflective circular symmetry is isomorphic with the orthogonal group O(2).
Two dimensions
A 2-dimensional object with circular symmetry would consist ofconcentric circle
In geometry, two or more objects are said to be concentric, coaxal, or coaxial when they share the same center or axis. Circles, regular polygons and regular polyhedra, and spheres may be concentric to one another (sharing the same center point ...
s and annular
Annulus (or anulus) or annular indicates a ring- or donut-shaped area or structure. It may refer to:
Human anatomy
* '' Anulus fibrosus disci intervertebralis'', spinal structure
* Annulus of Zinn, a.k.a. annular tendon or ''anulus tendineus co ...
domains.
Rotational circular symmetry has all cyclic symmetry, Z''n'' as subgroup symmetries. Reflective circular symmetry has all dihedral symmetry
In mathematics, a dihedral group is the group of symmetries of a regular polygon, which includes rotations and reflections. Dihedral groups are among the simplest examples of finite groups, and they play an important role in group theory, g ...
, Dih''n'' as subgroup symmetries.
Three dimensions
In 3-dimensions, a surface
A surface, as the term is most generally used, is the outermost or uppermost layer of a physical object or space. It is the portion or region of the object that can first be perceived by an observer using the senses of sight and touch, and is ...
or solid of revolution has circular symmetry around an axis, also called cylindrical symmetry or axial symmetry. An example is a right circular cone
A cone is a three-dimensional geometric shape that tapers smoothly from a flat base (frequently, though not necessarily, circular) to a point called the apex or vertex.
A cone is formed by a set of line segments, half-lines, or lines con ...
. Circular symmetry in 3 dimensions has all pyramidal symmetry, C''n''v as subgroups.
A double-cone, bicone
In geometry, a bicone or dicone (from la, bi-, and Greek: ''di-'', both meaning "two") is the three-dimensional surface of revolution of a rhombus around one of its axes of symmetry. Equivalently, a bicone is the surface created by joining ...
, cylinder
A cylinder (from ) has traditionally been a three-dimensional solid, one of the most basic of curvilinear geometric shapes. In elementary geometry, it is considered a prism with a circle as its base.
A cylinder may also be defined as an infin ...
, toroid
In mathematics, a toroid is a surface of revolution with a hole in the middle. The axis of revolution passes through the hole and so does not intersect the surface. For example, when a rectangle is rotated around an axis parallel to one of its ...
and spheroid
A spheroid, also known as an ellipsoid of revolution or rotational ellipsoid, is a quadric surface obtained by rotating an ellipse about one of its principal axes; in other words, an ellipsoid with two equal semi-diameters. A spheroid has ...
have circular symmetry, and in addition have a bilateral symmetry perpendular to the axis of system (or half cylindrical symmetry). These reflective circular symmetries have all discrete prismatic symmetries, D''n''h as subgroups.
Four dimensions
In four dimensions, an object can have circular symmetry, on two orthogonal axis planes, or duocylindrical symmetry. For example, theduocylinder
The duocylinder, also called the double cylinder or the bidisc, is a geometric object embedded in 4- dimensional Euclidean space, defined as the Cartesian product of two disks of respective radii ''r''1 and ''r''2:
:D = \left\
It is analogo ...
and Clifford torus have circular symmetry in two orthogonal axes. A spherinder
In four-dimensional geometry, the spherinder, or spherical cylinder or spherical prism, is a geometric object, defined as the Cartesian product of a 3- ball (or solid 2-sphere) of radius ''r''1 and a line segment of length 2''r''2:
:D = \
Lik ...
has spherical symmetry in one 3-space, and circular symmetry in the orthogonal direction.
Spherical symmetry
An analogous 3-dimensional equivalent term is spherical symmetry.
Rotational spherical symmetry is isomorphic with the rotation group SO(3)
In mechanics and geometry, the 3D rotation group, often denoted SO(3), is the group of all rotations about the origin of three-dimensional Euclidean space \R^3 under the operation of composition.
By definition, a rotation about the origin is a ...
, and can be parametrized by the Davenport chained rotations pitch, yaw, and roll. Rotational spherical symmetry has all the discrete chiral 3D point groups as subgroups. Reflectional spherical symmetry is isomorphic with the orthogonal group O(3) and has the 3-dimensional discrete point groups as subgroups.
A scalar field has spherical symmetry if it depends on the distance to the origin only, such as the potential
Potential generally refers to a currently unrealized ability. The term is used in a wide variety of fields, from physics to the social sciences to indicate things that are in a state where they are able to change in ways ranging from the simple r ...
of a central force
In classical mechanics, a central force on an object is a force that is directed towards or away from a point called center of force.
: \vec = \mathbf(\mathbf) = \left\vert F( \mathbf ) \right\vert \hat
where \vec F is the force, F is a vecto ...
. A vector field has spherical symmetry if it is in radially inward or outward direction with a magnitude and orientation (inward/outward) depending on the distance to the origin only, such as a central force.
See also
*Isotropy
Isotropy is uniformity in all orientations; it is derived . Precise definitions depend on the subject area. Exceptions, or inequalities, are frequently indicated by the prefix ' or ', hence ''anisotropy''. ''Anisotropy'' is also used to describe ...
* Rotational symmetry
Rotational symmetry, also known as radial symmetry in geometry, is the property a shape has when it looks the same after some rotation by a partial turn. An object's degree of rotational symmetry is the number of distinct orientations in which i ...
* Particle in a spherically symmetric potential
In the quantum mechanics description of a particle in spherical coordinates, a spherically symmetric potential, is a potential that depends only on the distance between the particle and a defined centre point. One example of a spherical potential ...
* Gauss's theorem
References
* * *{{springer, title=Orthogonal group, id=p/o070300 Symmetry Rotation