|
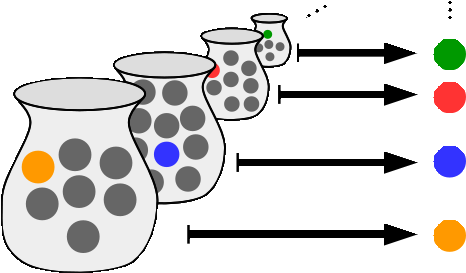
Surjection
In mathematics, a surjective function (also known as surjection, or onto function) is a function that every element can be mapped from element so that . In other words, every element of the function's codomain is the image of one element of its domain. It is not required that be unique; the function may map one or more elements of to the same element of . The term ''surjective'' and the related terms ''injective'' and ''bijective'' were introduced by Nicolas Bourbaki, a group of mainly French 20th-century mathematicians who, under this pseudonym, wrote a series of books presenting an exposition of modern advanced mathematics, beginning in 1935. The French word '' sur'' means ''over'' or ''above'', and relates to the fact that the image of the domain of a surjective function completely covers the function's codomain. Any function induces a surjection by restricting its codomain to the image of its domain. Every surjective function has a right inverse assuming the axiom ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Bijective Function
In mathematics, a bijection, also known as a bijective function, one-to-one correspondence, or invertible function, is a function between the elements of two sets, where each element of one set is paired with exactly one element of the other set, and each element of the other set is paired with exactly one element of the first set. There are no unpaired elements. In mathematical terms, a bijective function is a one-to-one (injective) and onto (surjective) mapping of a set ''X'' to a set ''Y''. The term ''one-to-one correspondence'' must not be confused with ''one-to-one function'' (an injective function; see figures). A bijection from the set ''X'' to the set ''Y'' has an inverse function from ''Y'' to ''X''. If ''X'' and ''Y'' are finite sets, then the existence of a bijection means they have the same number of elements. For infinite sets, the picture is more complicated, leading to the concept of cardinal number—a way to distinguish the various sizes of infinite sets. ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Function (mathematics)
In mathematics, a function from a set to a set assigns to each element of exactly one element of .; the words map, mapping, transformation, correspondence, and operator are often used synonymously. The set is called the domain of the function and the set is called the codomain of the function.Codomain ''Encyclopedia of Mathematics'Codomain. ''Encyclopedia of Mathematics''/ref> The earliest known approach to the notion of function can be traced back to works of Persian mathematicians Al-Biruni and Sharaf al-Din al-Tusi. Functions were originally the idealization of how a varying quantity depends on another quantity. For example, the position of a planet is a ''function'' of time. Historically, the concept was elaborated with the infinitesimal calculus at the end of the 17th century, and, until the 19th century, the functions that were considered were differentiable (that is, they had a high degree of regularity). The concept of a function was formalized at the end of ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Axiom Of Choice
In mathematics, the axiom of choice, or AC, is an axiom of set theory equivalent to the statement that ''a Cartesian product of a collection of non-empty sets is non-empty''. Informally put, the axiom of choice says that given any collection of sets, each containing at least one element, it is possible to construct a new set by arbitrarily choosing one element from each set, even if the collection is infinite. Formally, it states that for every indexed family (S_i)_ of nonempty sets, there exists an indexed set (x_i)_ such that x_i \in S_i for every i \in I. The axiom of choice was formulated in 1904 by Ernst Zermelo in order to formalize his proof of the well-ordering theorem. In many cases, a set arising from choosing elements arbitrarily can be made without invoking the axiom of choice; this is, in particular, the case if the number of sets from which to choose the elements is finite, or if a canonical rule on how to choose the elements is available – some distinguis ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Projection (set Theory)
In set theory, a projection is one of two closely related types of functions or operations, namely: * A set-theoretic operation typified by the ''j''th projection map, written \mathrm_, that takes an element \vec = (x_1,\ \ldots,\ x_j,\ \ldots,\ x_k) of the Cartesian product (X_1 \times \cdots \times X_j \times \cdots \times X_k) to the value \mathrm_(\vec) = x_j. * A function that sends an element ''x'' to its equivalence class under a specified equivalence relation ''E'', or, equivalently, a surjection from a set to another set.. The function from elements to equivalence classes is a surjection, and every surjection corresponds to an equivalence relation under which two elements are equivalent when they have the same image. The result of the mapping is written as 'x''when ''E'' is understood, or written as 'x''sub>''E'' when it is necessary to make ''E'' explicit. See also * Cartesian product * Projection (relational algebra) * Projection (mathematics) In mathematics, a proj ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Injective Function
In mathematics, an injective function (also known as injection, or one-to-one function) is a function that maps distinct elements of its domain to distinct elements; that is, implies . (Equivalently, implies in the equivalent contrapositive statement.) In other words, every element of the function's codomain is the image of one element of its domain. The term must not be confused with that refers to bijective functions, which are functions such that each element in the codomain is an image of exactly one element in the domain. A homomorphism between algebraic structures is a function that is compatible with the operations of the structures. For all common algebraic structures, and, in particular for vector spaces, an is also called a . However, in the more general context of category theory, the definition of a monomorphism differs from that of an injective homomorphism. This is thus a theorem that they are equivalent for algebraic structures; see for more details. ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Codomain
In mathematics, the codomain or set of destination of a function is the set into which all of the output of the function is constrained to fall. It is the set in the notation . The term range is sometimes ambiguously used to refer to either the codomain or image of a function. A codomain is part of a function if is defined as a triple where is called the ''domain'' of , its ''codomain'', and its '' graph''. The set of all elements of the form , where ranges over the elements of the domain , is called the ''image'' of . The image of a function is a subset of its codomain so it might not coincide with it. Namely, a function that is not surjective has elements in its codomain for which the equation does not have a solution. A codomain is not part of a function if is defined as just a graph. For example in set theory it is desirable to permit the domain of a function to be a proper class , in which case there is formally no such thing as a triple . With such a d ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Codomain2
In mathematics, the codomain or set of destination of a Function (mathematics), function is the Set (mathematics), set into which all of the output of the function is constrained to fall. It is the set in the notation . The term Range of a function, range is sometimes ambiguously used to refer to either the codomain or Image (mathematics), image of a function. A codomain is part of a function if is defined as a triple where is called the ''Domain of a function, domain'' of , its ''codomain'', and its ''Graph of a function, graph''. The set of all elements of the form , where ranges over the elements of the domain , is called the ''Image (mathematics), image'' of . The image of a function is a subset of its codomain so it might not coincide with it. Namely, a function that is not Surjective function, surjective has elements in its codomain for which the equation does not have a solution. A codomain is not part of a function if is defined as just a graph. For ex ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Image (mathematics)
In mathematics, the image of a function is the set of all output values it may produce. More generally, evaluating a given function f at each element of a given subset A of its domain produces a set, called the "image of A under (or through) f". Similarly, the inverse image (or preimage) of a given subset B of the codomain of f, is the set of all elements of the domain that map to the members of B. Image and inverse image may also be defined for general binary relations, not just functions. Definition The word "image" is used in three related ways. In these definitions, f : X \to Y is a function from the set X to the set Y. Image of an element If x is a member of X, then the image of x under f, denoted f(x), is the value of f when applied to x. f(x) is alternatively known as the output of f for argument x. Given y, the function f is said to "" or "" if there exists some x in the function's domain such that f(x) = y. Similarly, given a set S, f is said to "" if there ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Domain Of A Function
In mathematics, the domain of a function is the set of inputs accepted by the function. It is sometimes denoted by \operatorname(f) or \operatornamef, where is the function. More precisely, given a function f\colon X\to Y, the domain of is . Note that in modern mathematical language, the domain is part of the definition of a function rather than a property of it. In the special case that and are both subsets of \R, the function can be graphed in the Cartesian coordinate system. In this case, the domain is represented on the -axis of the graph, as the projection of the graph of the function onto the -axis. For a function f\colon X\to Y, the set is called the codomain, and the set of values attained by the function (which is a subset of ) is called its range or image. Any function can be restricted to a subset of its domain. The restriction of f \colon X \to Y to A, where A\subseteq X, is written as \left. f \_A \colon A \to Y. Natural domain If a real function ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Image (mathematics)
In mathematics, the image of a function is the set of all output values it may produce. More generally, evaluating a given function f at each element of a given subset A of its domain produces a set, called the "image of A under (or through) f". Similarly, the inverse image (or preimage) of a given subset B of the codomain of f, is the set of all elements of the domain that map to the members of B. Image and inverse image may also be defined for general binary relations, not just functions. Definition The word "image" is used in three related ways. In these definitions, f : X \to Y is a function from the set X to the set Y. Image of an element If x is a member of X, then the image of x under f, denoted f(x), is the value of f when applied to x. f(x) is alternatively known as the output of f for argument x. Given y, the function f is said to "" or "" if there exists some x in the function's domain such that f(x) = y. Similarly, given a set S, f is said to "" if there ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Domain Of A Function
In mathematics, the domain of a function is the set of inputs accepted by the function. It is sometimes denoted by \operatorname(f) or \operatornamef, where is the function. More precisely, given a function f\colon X\to Y, the domain of is . Note that in modern mathematical language, the domain is part of the definition of a function rather than a property of it. In the special case that and are both subsets of \R, the function can be graphed in the Cartesian coordinate system. In this case, the domain is represented on the -axis of the graph, as the projection of the graph of the function onto the -axis. For a function f\colon X\to Y, the set is called the codomain, and the set of values attained by the function (which is a subset of ) is called its range or image. Any function can be restricted to a subset of its domain. The restriction of f \colon X \to Y to A, where A\subseteq X, is written as \left. f \_A \colon A \to Y. Natural domain If a real function ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
France
France (), officially the French Republic ( ), is a country primarily located in Western Europe. It also comprises of overseas regions and territories in the Americas and the Atlantic, Pacific and Indian Oceans. Its metropolitan area extends from the Rhine to the Atlantic Ocean and from the Mediterranean Sea to the English Channel and the North Sea; overseas territories include French Guiana in South America, Saint Pierre and Miquelon in the North Atlantic, the French West Indies, and many islands in Oceania and the Indian Ocean. Due to its several coastal territories, France has the largest exclusive economic zone in the world. France borders Belgium, Luxembourg, Germany, Switzerland, Monaco, Italy, Andorra, and Spain in continental Europe, as well as the Netherlands, Suriname, and Brazil in the Americas via its overseas territories in French Guiana and Saint Martin. Its eighteen integral regions (five of which are overseas) span a combined area of and contain clos ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |