alternating group on:
[Wikipedia]
[Google]
[Amazon]
In

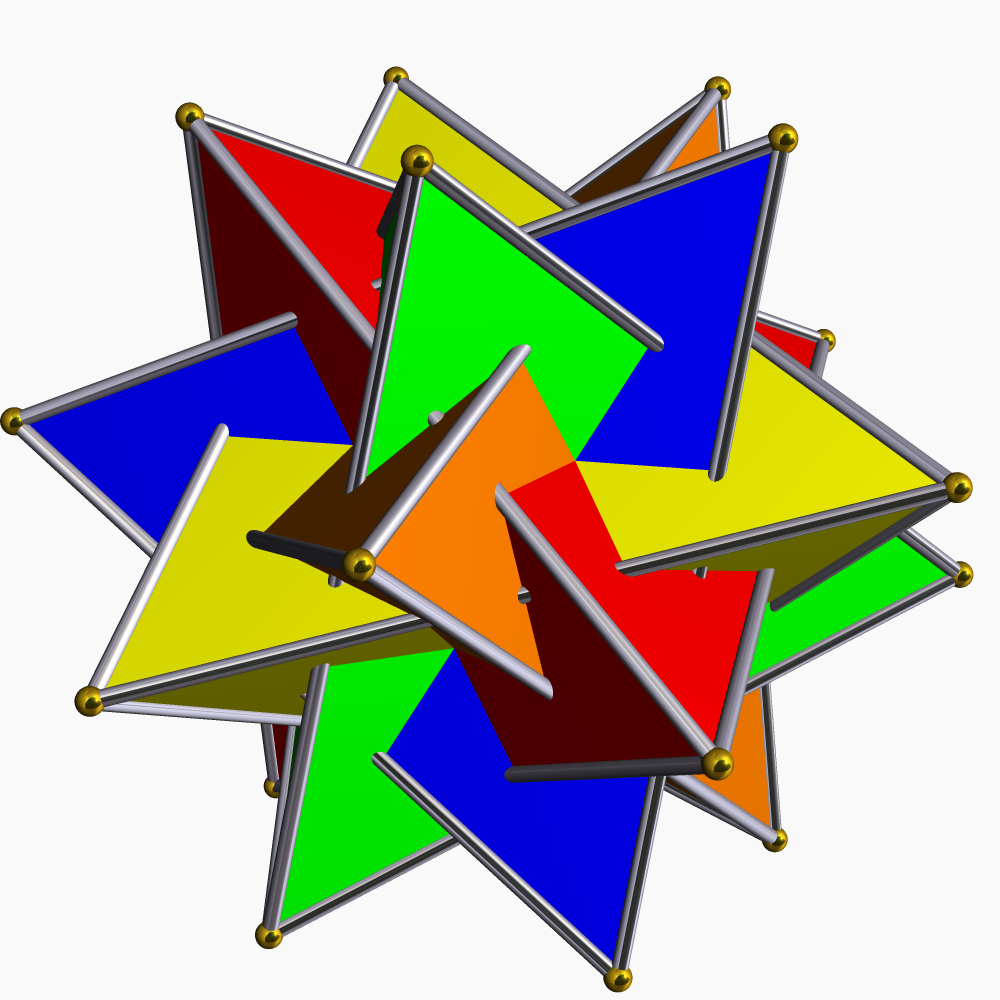 A5 is the group of isometries of a dodecahedron in 3-space, so there is a representation .
In this picture the vertices of the polyhedra represent the elements of the group, with the center of the sphere representing the identity element. Each vertex represents a rotation about the axis pointing from the center to that vertex, by an angle equal to the distance from the origin, in radians. Vertices in the same polyhedron are in the same conjugacy class. Since the conjugacy class equation for A5 is , we obtain four distinct (nontrivial) polyhedra.
The vertices of each polyhedron are in bijective correspondence with the elements of its conjugacy class, with the exception of the conjugacy class of (2,2)-cycles, which is represented by an icosidodecahedron on the outer surface, with its antipodal vertices identified with each other. The reason for this redundancy is that the corresponding rotations are by radians, and so can be represented by a vector of length in either of two directions. Thus the class of (2,2)-cycles contains 15 elements, while the icosidodecahedron has 30 vertices.
The two conjugacy classes of twelve 5-cycles in A5 are represented by two icosahedra, of radii 2/5 and 4/5, respectively. The nontrivial outer automorphism in interchanges these two classes and the corresponding icosahedra.
A5 is the group of isometries of a dodecahedron in 3-space, so there is a representation .
In this picture the vertices of the polyhedra represent the elements of the group, with the center of the sphere representing the identity element. Each vertex represents a rotation about the axis pointing from the center to that vertex, by an angle equal to the distance from the origin, in radians. Vertices in the same polyhedron are in the same conjugacy class. Since the conjugacy class equation for A5 is , we obtain four distinct (nontrivial) polyhedra.
The vertices of each polyhedron are in bijective correspondence with the elements of its conjugacy class, with the exception of the conjugacy class of (2,2)-cycles, which is represented by an icosidodecahedron on the outer surface, with its antipodal vertices identified with each other. The reason for this redundancy is that the corresponding rotations are by radians, and so can be represented by a vector of length in either of two directions. Thus the class of (2,2)-cycles contains 15 elements, while the icosidodecahedron has 30 vertices.
The two conjugacy classes of twelve 5-cycles in A5 are represented by two icosahedra, of radii 2/5 and 4/5, respectively. The nontrivial outer automorphism in interchanges these two classes and the corresponding icosahedra.
 It can be proved that the
It can be proved that the
mathematics
Mathematics is an area of knowledge that includes the topics of numbers, formulas and related structures, shapes and the spaces in which they are contained, and quantities and their changes. These topics are represented in modern mathematics ...
, an alternating group is the group of even permutation
In mathematics, when ''X'' is a finite set with at least two elements, the permutations of ''X'' (i.e. the bijective functions from ''X'' to ''X'') fall into two classes of equal size: the even permutations and the odd permutations. If any total o ...
s of a finite set. The alternating group on a set of elements is called the alternating group of degree , or the alternating group on letters and denoted by or
Basic properties
For , the group A''n'' is the commutator subgroup of the symmetric group S''n'' withindex
Index (or its plural form indices) may refer to:
Arts, entertainment, and media Fictional entities
* Index (''A Certain Magical Index''), a character in the light novel series ''A Certain Magical Index''
* The Index, an item on a Halo megastru ...
2 and has therefore ''n''!/2 elements. It is the kernel of the signature group homomorphism explained under symmetric group.
The group A''n'' is abelian
Abelian may refer to:
Mathematics Group theory
* Abelian group, a group in which the binary operation is commutative
** Category of abelian groups (Ab), has abelian groups as objects and group homomorphisms as morphisms
* Metabelian group, a grou ...
if and only if
In logic and related fields such as mathematics and philosophy, "if and only if" (shortened as "iff") is a biconditional logical connective between statements, where either both statements are true or both are false.
The connective is bic ...
and simple
Simple or SIMPLE may refer to:
*Simplicity, the state or quality of being simple
Arts and entertainment
* ''Simple'' (album), by Andy Yorke, 2008, and its title track
* "Simple" (Florida Georgia Line song), 2018
* "Simple", a song by Johnn ...
if and only if or . A5 is the smallest non-abelian simple group, having order
Order, ORDER or Orders may refer to:
* Categorization, the process in which ideas and objects are recognized, differentiated, and understood
* Heterarchy, a system of organization wherein the elements have the potential to be ranked a number of ...
60, and the smallest non- solvable group.
The group A4 has the Klein four-group
In mathematics, the Klein four-group is a group with four elements, in which each element is self-inverse (composing it with itself produces the identity)
and in which composing any two of the three non-identity elements produces the third one ...
V as a proper normal subgroup
In abstract algebra, a normal subgroup (also known as an invariant subgroup or self-conjugate subgroup) is a subgroup that is invariant under conjugation by members of the group of which it is a part. In other words, a subgroup N of the group G ...
, namely the identity and the double transpositions , that is the kernel of the surjection of A4 onto . We have the exact sequence . In Galois theory, this map, or rather the corresponding map , corresponds to associating the Lagrange resolvent cubic to a quartic, which allows the quartic polynomial
In algebra, a quartic function is a function of the form
:f(x)=ax^4+bx^3+cx^2+dx+e,
where ''a'' is nonzero,
which is defined by a polynomial of degree four, called a quartic polynomial.
A ''quartic equation'', or equation of the fourth deg ...
to be solved by radicals, as established by Lodovico Ferrari.
Conjugacy classes
As in the symmetric group, any two elements of A''n'' that are conjugate by an element of A''n'' must have the same cycle shape. The converse is not necessarily true, however. If the cycle shape consists only of cycles of odd length with no two cycles the same length, where cycles of length one are included in the cycle type, then there are exactly two conjugacy classes for this cycle shape . Examples: *The twopermutation
In mathematics, a permutation of a set is, loosely speaking, an arrangement of its members into a sequence or linear order, or if the set is already ordered, a rearrangement of its elements. The word "permutation" also refers to the act or pro ...
s (123) and (132) are not conjugates in A3, although they have the same cycle shape, and are therefore conjugate in S3.
*The permutation (123)(45678) is not conjugate to its inverse (132)(48765) in A8, although the two permutations have the same cycle shape, so they are conjugate in S8.
Relation with symmetric group
:''See Symmetric group''. As finite symmetric groups are the groups of all permutations of a set with finite elements, and the alternating groups are groups of even permutations, alternating groups are subgroups of finite symmetric groups.Generators and relations
For ''n'' ≥ 3, A''n'' is generated by 3-cycles, since 3-cycles can be obtained by combining pairs of transpositions. This generating set is often used to prove that A''n'' is simple for .Automorphism group
For , except for , the automorphism group of A''n'' is the symmetric group S''n'', with inner automorphism group A''n'' andouter automorphism group In mathematics, the outer automorphism group of a group, , is the quotient, , where is the automorphism group of and ) is the subgroup consisting of inner automorphisms. The outer automorphism group is usually denoted . If is trivial and has a ...
Z2; the outer automorphism comes from conjugation by an odd permutation.
For and 2, the automorphism group is trivial. For the automorphism group is Z2, with trivial inner automorphism group and outer automorphism group Z2.
The outer automorphism group of A6 is the Klein four-group , and is related to the outer automorphism of S6. The extra outer automorphism in A6 swaps the 3-cycles (like (123)) with elements of shape 32 (like ).
Exceptional isomorphisms
There are some exceptional isomorphisms between some of the small alternating groups and small groups of Lie type, particularlyprojective special linear group
In mathematics, especially in the group theoretic area of algebra, the projective linear group (also known as the projective general linear group or PGL) is the induced action of the general linear group of a vector space ''V'' on the associa ...
s. These are:
* A4 is isomorphic to PSL2(3)Robinson (1996), p. 78/ref> and the symmetry group
In group theory, the symmetry group of a geometric object is the group of all transformations under which the object is invariant, endowed with the group operation of composition. Such a transformation is an invertible mapping of the amb ...
of chiral tetrahedral symmetry.
* A5 is isomorphic to PSL2(4), PSL2(5), and the symmetry group of chiral icosahedral symmetry. (See for an indirect isomorphism of using a classification of simple groups of order 60, and here
Here is an adverb that means "in, on, or at this place". It may also refer to:
Software
* Here Technologies, a mapping company
* Here WeGo (formerly Here Maps), a mobile app and map website by Here
Television
* Here TV (formerly "here!"), a ...
for a direct proof).
* A6 is isomorphic to PSL2(9) and PSp4(2)'.
* A8 is isomorphic to PSL4(2).
More obviously, A3 is isomorphic to the cyclic group Z3, and A0, A1, and A2 are isomorphic to the trivial group
In mathematics, a trivial group or zero group is a group consisting of a single element. All such groups are isomorphic, so one often speaks of the trivial group. The single element of the trivial group is the identity element and so it is usuall ...
(which is also for any ''q'').
Examples S4 and A4
Example A5 as a subgroup of 3-space rotations

 A5 is the group of isometries of a dodecahedron in 3-space, so there is a representation .
In this picture the vertices of the polyhedra represent the elements of the group, with the center of the sphere representing the identity element. Each vertex represents a rotation about the axis pointing from the center to that vertex, by an angle equal to the distance from the origin, in radians. Vertices in the same polyhedron are in the same conjugacy class. Since the conjugacy class equation for A5 is , we obtain four distinct (nontrivial) polyhedra.
The vertices of each polyhedron are in bijective correspondence with the elements of its conjugacy class, with the exception of the conjugacy class of (2,2)-cycles, which is represented by an icosidodecahedron on the outer surface, with its antipodal vertices identified with each other. The reason for this redundancy is that the corresponding rotations are by radians, and so can be represented by a vector of length in either of two directions. Thus the class of (2,2)-cycles contains 15 elements, while the icosidodecahedron has 30 vertices.
The two conjugacy classes of twelve 5-cycles in A5 are represented by two icosahedra, of radii 2/5 and 4/5, respectively. The nontrivial outer automorphism in interchanges these two classes and the corresponding icosahedra.
A5 is the group of isometries of a dodecahedron in 3-space, so there is a representation .
In this picture the vertices of the polyhedra represent the elements of the group, with the center of the sphere representing the identity element. Each vertex represents a rotation about the axis pointing from the center to that vertex, by an angle equal to the distance from the origin, in radians. Vertices in the same polyhedron are in the same conjugacy class. Since the conjugacy class equation for A5 is , we obtain four distinct (nontrivial) polyhedra.
The vertices of each polyhedron are in bijective correspondence with the elements of its conjugacy class, with the exception of the conjugacy class of (2,2)-cycles, which is represented by an icosidodecahedron on the outer surface, with its antipodal vertices identified with each other. The reason for this redundancy is that the corresponding rotations are by radians, and so can be represented by a vector of length in either of two directions. Thus the class of (2,2)-cycles contains 15 elements, while the icosidodecahedron has 30 vertices.
The two conjugacy classes of twelve 5-cycles in A5 are represented by two icosahedra, of radii 2/5 and 4/5, respectively. The nontrivial outer automorphism in interchanges these two classes and the corresponding icosahedra.
Example: the 15 puzzle
15 puzzle
The 15 puzzle (also called Gem Puzzle, Boss Puzzle, Game of Fifteen, Mystic Square and many others) is a sliding puzzle having 15 square tiles numbered 1–15 in a frame that is 4 tiles high and 4 tiles wide, leaving one unoccupied tile positio ...
, a famous example of the sliding puzzle, can be represented by the alternating group A15, because the combinations of the 15 puzzle can be generated by 3-cycles. In fact, any sliding puzzle with square tiles of equal size can be represented by A2''k''−1.
Subgroups
A4 is the smallest group demonstrating that the converse of Lagrange's theorem is not true in general: given a finite group ''G'' and a divisor ''d'' of , there does not necessarily exist a subgroup of ''G'' with order ''d'': the group , of order 12, has no subgroup of order 6. A subgroup of three elements (generated by a cyclic rotation of three objects) with any distinct nontrivial element generates the whole group. For all , A''n'' has no nontrivial (that is, proper)normal subgroup
In abstract algebra, a normal subgroup (also known as an invariant subgroup or self-conjugate subgroup) is a subgroup that is invariant under conjugation by members of the group of which it is a part. In other words, a subgroup N of the group G ...
s. Thus, A''n'' is a simple group for all . A5 is the smallest non-solvable group.
Group homology
The group homology of the alternating groups exhibits stabilization, as instable homotopy theory
In mathematics, stable homotopy theory is the part of homotopy theory (and thus algebraic topology) concerned with all structure and phenomena that remain after sufficiently many applications of the suspension functor. A founding result was the F ...
: for sufficiently large ''n'', it is constant. However, there are some low-dimensional exceptional homology. Note that the homology of the symmetric group exhibits similar stabilization, but without the low-dimensional exceptions (additional homology elements).
''H''1: Abelianization
The first homology group coincides with abelianization, and (since A''n'' is perfect, except for the cited exceptions) is thus: :''H''1(A''n'', Z) = Z1 for ''n'' = 0, 1, 2; :''H''1(A3, Z) = A = A3 = Z3; :''H''1(A4, Z) = A = Z3; :''H''1(A''n'', Z) = Z1 for ''n'' ≥ 5. This is easily seen directly, as follows. A''n'' is generated by 3-cycles – so the only non-trivial abelianization maps are since order-3 elements must map to order-3 elements – and for all 3-cycles are conjugate, so they must map to the same element in the abelianization, since conjugation is trivial in abelian groups. Thus a 3-cycle like (123) must map to the same element as its inverse (321), but thus must map to the identity, as it must then have order dividing 2 and 3, so the abelianization is trivial. For , A''n'' is trivial, and thus has trivial abelianization. For A3 and A4 one can compute the abelianization directly, noting that the 3-cycles form two conjugacy classes (rather than all being conjugate) and there are non-trivial maps (in fact an isomorphism) and .''H''2: Schur multipliers
TheSchur multiplier
In mathematical group theory, the Schur multiplier or Schur multiplicator is the second homology group H_2(G, \Z) of a group ''G''. It was introduced by in his work on projective representations.
Examples and properties
The Schur multiplier \ope ...
s of the alternating groups A''n'' (in the case where ''n'' is at least 5) are the cyclic groups of order 2, except in the case where ''n'' is either 6 or 7, in which case there is also a triple cover. In these cases, then, the Schur multiplier is (the cyclic group) of order 6. These were first computed in .
:''H''2(A''n'', Z) = Z1 for ''n'' = 1, 2, 3;
:''H''2(A''n'', Z) = Z2 for ''n'' = 4, 5;
:''H''2(A''n'', Z) = Z6 for ''n'' = 6, 7;
:''H''2(A''n'', Z) = Z2 for ''n'' ≥ 8.
Notes
References
* * *External links
* * {{DEFAULTSORT:Alternating Group Finite groups Permutation groups